?Mathematical formulae have been encoded as MathML and are displayed in this HTML version using MathJax in order to improve their display. Uncheck the box to turn MathJax off. This feature requires Javascript. Click on a formula to zoom.
?Mathematical formulae have been encoded as MathML and are displayed in this HTML version using MathJax in order to improve their display. Uncheck the box to turn MathJax off. This feature requires Javascript. Click on a formula to zoom.Abstract
Let be a commutative ring with identity. The nilpotent graph of
, denoted by
, is a graph with vertex set
, and two vertices
and
are adjacent if and only if
is nilpotent, where
. In this paper, we characterize finite rings (up to isomorphism) with identity whose nilpotent graphs can be embedded in the projective plane or Klein bottle. Also, we classify finite rings whose nilpotent graphs are ring graph or outerplanarity index 1,2.
1 Introduction
Throughout, rings are commutative with . The zero-divisor of a ring
is denoted by
and
. The zero-divisor graph of
, denoted by
, is an undirected graph whose vertices are elements of
and two distinct vertices
and
are adjacent if and only if
. The zero-divisor graph of a commutative ring was first investigated by Beck [Citation1]. He was interested in coloring of the graph
.
Later, it was modified as in present form by Anderson and Livingston [Citation2]. This paper deals with the nilpotent graph of rings. Recall that the nilpotent graph of , denoted by
, is a graph with vertex set
, and two vertices
and
are adjacent if and only if
is nilpotent, where
. It was first investigated by Chen [Citation3]. He consoders all the elements of ring
as the vertices of the graph and two vertices
and
are adjacent if and only if
is nilpotent. However, in 2010, Li [Citation4] modified and studied the nilpotent graph
of
. Note that the usual zerodivisor graph
is a subgraph of the graph
. If
,where each
is a field and
, then
.
The focus of this paper is on finding non-orientable genus(crosscap) of the graph . This work is motivated by the following result of Hsieh [Citation5] who completely determined the finite commutative rings whose zero-divisor graphs are projective planar. This paper is organized as follows: In Section 2, we characterize finite rings (up to isomorphism) with identity whose nilpotent graphs can be embedded in the projective plane or Klein bottle. In Section 3, we classify finite rings whose nilpotent graphs are ring graph or outerplanar.
One can refer to [Citation6] for the following: We denote the ring of integers modulo by
and the field with
elements by
. If
is a subset of
,we denote
set of units of
and Spec(R) is the set of all prime ideals of
. If
is a direct product of local rings, then
denotes the zero element of
. We use
for the complete graph with
vertices and
for the complete bipartite graph.
One can refer to [Citation7] for the following: By a surface, we mean a connected two-dimensional real manifold, i.e., a connected topological space such that each point has a neighborhood homeomorphic to an open disc. Every non-orientable compact surface is a connected sum of finite copies of
projective planes (see [Citation7]). The number of copies of projective planes is called the crosscap number (non-orientable genus) of this surface. The crosscap of a graph
, denoted
, is the smallest integer
such that the graph can be embedded in
. For example, the projective plane is of crosscap one and the Klein bottle is of crosscap two. A non-planar graph is said to be projective if it can be embedded into a projective plane. The following results are used in the subsequent section.
Lemma 1
[Citation7]
Let be integers and for a real number
, let
denote the smallest integer that is greater than or equal to
. Then
| (i) | |||||
| (ii) |
| ||||
Theorem 1
[Citation8, Kuratowski’s]
The graph is planar if and only if it contains no subgraph isomorphic to
or
or a subdivision of one of these.
Theorem 2
[Citation9, Theorem 3.1]
Let be a finite local ring with
for some prime
and some positive integer
. Then
is planar if and only if
is isomorphic to one of the following rings:
or
.
Theorem 3
[Citation9, Theorem 3.2]
Let be a finite commutative ring with identity, where each
is a local ring and
is a field,
and
. Then
is planar if and only if
is isomorphic to one of the following rings:
or
.
Theorem 4
[Citation9]
Let be a finite ring, where each
is a field and
. Then
is planar if and only if
is isomorphic to one of the following rings:
or
, where
is a finite field.
Theorem 5
[Citation5]
Let be a finite ring such that
. Then
.
Theorem 6
[Citation9]
Let be a finite local ring with
for some prime
and some positive integer
. Then
, where
is the set of all units in
.
Theorem 7
[Citation5]
Let be a finite ring such that
. Then
if and only if
is isomorphic to one of the following 16 rings:
.
Theorem 8
[Citation5]
Let be a finite ring such that
. Then
if and only if
is isomorphic to one of the following 6 rings:
.
Theorem 9
[Citation5]
Let be a finite ring such that
. Then
if and only if
is isomorphic to
.
Theorem 10
[Citation10]
Suppose , and
. The non-orientable genus of
is given by
Theorem 11
[Citation11]
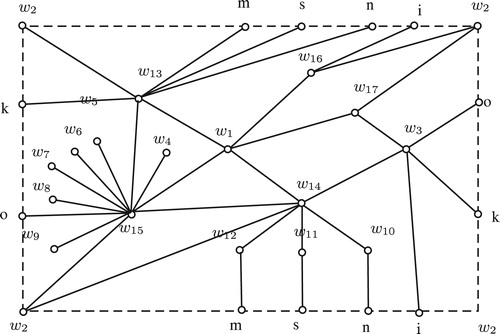
The complete bipartite graph admits the unique embedding into the Klein bottle, up to congruence, as shown in .
2 Crosscap of 

In this section, we classify all finite rings (up to isomorphism) with identity whose nilpotent graphs can be embedded in a projective plane or Klein bottle.
The following Lemma gives the general formula for the crosscap of the graph in the case of local ring.
Lemma 2
If is a finite local ring with
and
, then
.
Proof
By Theorem 6, . Also
and
. By Theorem 10,
.
Lemma 3
If is a finite commutative ring with identity, where
’s are local rings which are not fields, then
.
Proof
Since is a subgraph of
,
contains a copy of
which is a subgraph of the graph induced by the set of vertices
. By Lemma 1
,
. Thus the result follows.□
We consider the finite ring and so
where
is a local ring. On the observation of Lemma 3, at least one
is a field, for some
.
Lemma 4
Let be a finite commutative ring with identity, where each
is a field,
and
. Then
if one of the following holds:
and
, and either
or
and
and
and
.
Proof
| (i) | Follows from Theorem 5. | ||||
| (ii) | Suppose | ||||
| (iii) | Suppose | ||||
| (iv) | Suppose | ||||
| (v) | Suppose | ||||
| (vi) | Suppose | ||||
The following Theorems characterize the ring for which
and
.
Theorem 12
Let be a finite ring. Then
if and only if
is isomorphic to one of the following rings:
or
.
Proof
Assume that . Then by Theorem 5,
. Since
is finite,
, where
is a local ring for each
. Without loss of generality, assume that
. If
, then by Lemma 4
,
.
Case 1: .
If is a field for all
, then
and so by Theorem 8,
is isomorphic to one of the following rings:
. If not, without loss of generality, let
be a local ring but not a field. Then
is a subgraph of
. So
contains a copy of
which is the subgraph of a graph induced by the set of vertices
. It then follows from Lemma 1
that
, a contradiction.
Case 2: .
If is a field, then
and so by Theorem 7,
or
. If not, without loss of generality, let
be a local ring but not a field. We claim that
and
.
Suppose that . Then let
and
, where
for
. Then
contains a copy of
which is the subgraph of the graph induced by set of vertices
and so
, a contradiction. Hence
and so
.
Suppose that . Then let
and
where
and
for all
. Then
contains a copy of
which is the subgraph of the graph induced by set of vertices
. It then follows from Lemma 1
that
, a contradiction. Hence
.
Thus we get . By Theorem 3,
is planar for
. Hence we conclude that
or
.
Case 3: .
If , then by Theorem 2,
is planar. Hence
. Now by Lemma 2,
, where
and
. Since
,
and
. So
is isomorphic to one of the following rings:
or
.
Conversely, suppose is isomorphic to one of the following rings:
or
. Then by Lemma 2,
.
If or
, then by Theorem 3,
is non-planar. Moreover shows one explicit embedding of
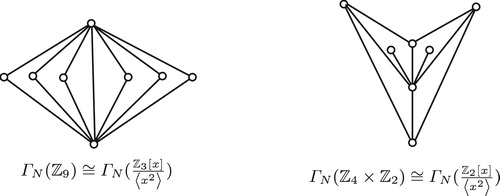
in the projective plane. Thus
.
Suppose is isomorphic to one of the following rings:
or
. Then by Theorems 7 – 9,
. This completes the proof.□
Theorem 13
Let be a finite ring. Then
if and only if
is isomorphic to one of the following rings:
or
.
Proof
Assume that . Then by Theorem 5,
. Since
is finite,
, where
’s are local ring for each
. Without loss of generality, assume that
.
Case 1: .
Then by Lemma 4 ,
. Also, by Theorem 12,
. Thus there is no ring available in this case.
Case 2: .
If is a field for all
, then by Lemma 4
–
,
is isomorphic to one of the following rings:
.
If
is isomorphic to
, then
and so by Theorem 4,
is planar.
If
is isomorphic to one of the following rings:
, then by Theorem 12,
.
Thus is isomorphic to the following rings:
.
Suppose that at least one is not a field. Without loss of generality, let
be a local ring but not a field. Then
is a subgraph of
. So
contains a copy of
which is the subgraph of a graph induced by the set of vertices
. It then follows from Lemma 1
that
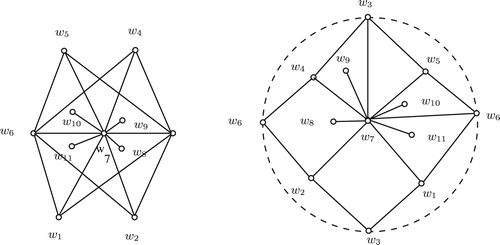
. We claim that
. By Theorem 11,
has a unique embedding in
and it has six 4-faces and one 6-face. Hence we can find a unique embedding of
in
that contains nine 4-faces. Let
be a partition of
for
. Then each face contains exactly two vertices from
. Also each
from
lie in exactly 3 faces. So there may be at most one face that contains the vertices
. Suppose there exists a face that contains the vertices
. Since
and
are adjacent to these vertices in
, there must be one edge crossing in the embedding of
. Suppose not. Then we cannot insert that vertices without crossing. Hence we cannot have the embedding of
in the non-orientable surface of crosscap 2. Thus
, a contradiction.
Case 3: .
If is a field, then
. Since
or
. Hence
or
. If not, then at least one
is not a field. Without loss of generality, let
be a local ring but not a field. We claim that
and
.
Suppose that . Then
or
. Let
. Let
and
, where
for
. Then
contains a copy of
which is the subgraph of the graph induced by set of vertices
and so
, a contradiction. Hence
or
.
Suppose that . Then
contains a copy of
which consists of vertices of
. It then follows from Lemma 1
that
, which contradicts our assumption. Therefore
and so
.
Suppose that . Then let
and
where
and
for all
. Then
contains a copy of
which is the subgraph of the graph induced by set of vertices
. It then follows from Lemma 1
that
, a contradiction. Hence
.
Thus we get is isomorphic to one of the following rings:
. By Theorem 2,
is planar for
. Also, by Theorem 12,
for
. Hence we conclude that
or
.
Conversely, suppose that or
. Then since
, by Lemma 1
,
.
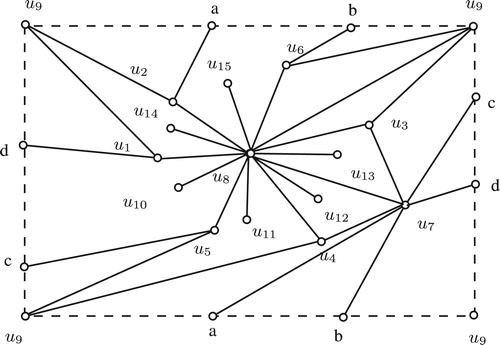
Assume that is isomorphic to one of the following rings:
or
.
Then since ,
contains a copy of
which is the subgraph of the graph induced by the set of vertices
. By Lemma 1
,
. Moreover shows one explicit embedding of
in
. Thus
.
Assume that is isomorphic to
. Then let
and
for
. Then
contains a copy of
which consists of vertices
. It then follows from Lemma 1
that
. Moreover shows the embedding in the non-orientable surface of crosscap 2. Thus
.
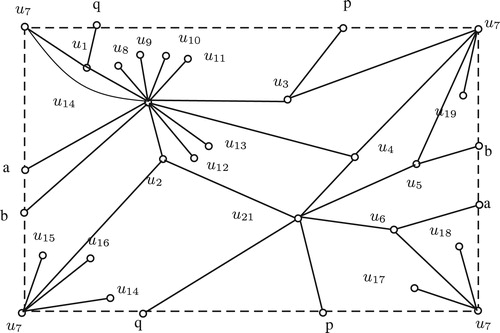
Suppose that . Then let
for
. Then
contains a copy of
which consists of vertices
. It then follows from Lemma 1
that
. Moreover shows one explicit embedding of
in the non-orientable surface of crosscap 2. Thus
. This completes the proof.□
3 Outerplanarity of 

Let be a graph with
vertices and
edges. A chord is any edge of
joining two nonadjacent vertices in a cycle of
. Let
be a cycle of
. We say
is a primitive cycle if it has no chords. Also, a graph
has the primitive cycle property (PCP) if any two primitive cycles intersect in at most one edge. The number
is called the free rank of
and it is the number of primitive cycles of
. Also, the number
is called the cycle rank of
, where
is the number of connected components of
. The cycle rank of
can be expressed as the dimension of the cycle space of
. By [Citation12, Proposition 2.2], we have
. A graph
is called a ring graph if it satisfies one of the following equivalent conditions (see [Citation9]).
rank(G) = frank(G),
satisfies the PCP and
does not contain a subdivision of
as a subgraph.
The following concepts were defined in [Citation13]. An embedding of a planar graph is 1-outerplanar if it is outerplanar (i.e.) all vertices are incident on the outerface in
. An embedding of a planar graph is
-outerplanar if removing all vertices on the outerface (together with their incident edges) yields a
-outerplanar embedding. A graph is
-outerplanar if it has a
-outerplanar embedding. The outerplanarity index of a graph
is the smallest
such that
is
-outerplanar. There is a characterization for outerplanar graphs that says a graph is outerplanar if and only if it does not contain a subdivision of
or
. Clearly, every outerplanar graph is a ring graph or every ring graph is a planar graph. In this section, we characterize all rings R such that
is a ring graph and outerplanar graph with index 1,2.
Theorem 14
Let be a finite ring. Then
is a ring graph if and only if
is isomorphic to one of the following rings:
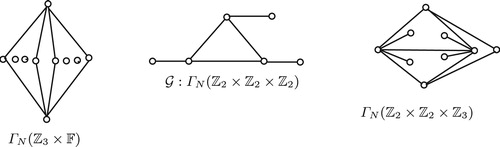
or
.
Proof
Assume that is a ring graph. Then since every ring graph is planar, it is enough to check rings in Theorems 2–4, whether it is a ring graph or not. Now the nilpotent graph of rings
we have that
, and so they are ring graphs. Also for rings
, where
,
contains a subdivision of
or does not satisfy PCP (see ) and so they are not ring graphs. The converse is obvious.□
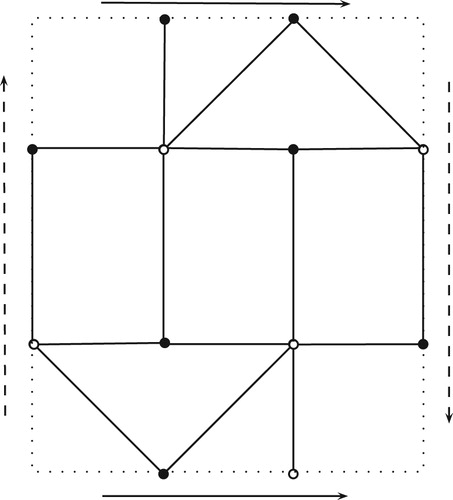
Theorem 15
Let be a finite ring. Then
is an outerplanar if and only if
is isomorphic to one of the following rings:
Proof
Assume that is an outerplanar. Then since
is a ring graph, by Theorem 14, we need to check rings
are outerplanar or not. By , we can find the subdivision of
in
for rings
and so are not outerplanar. Also, the nilpotent graph for rings
, is
or
(see ) respectively and so are outerplanar. The converse is obvious.□
Theorem 16
Let be a finite ring. Then
has an outerplanarity index 2 if and only if
is isomorphic to one of the following rings:
.
Proof
Assume that has an outerplanarity index 2. Then
is planar, by Theorems 2–4, we need only to check rings:
. are 2-outerplanar and is minimum or not. Since
is not 1-outerplanar, by Theorem 15,
is not isomorphic to the following rings:
. By Figs. , , the nilpotent graph for rings
, are 2-outerplanar, as the removal of vertices in the outer face remains the graph
or totally disconnected. The converse is obvious.□
Corollary 1
Let be a finite ring. Then
has an outerplanarity index at most two.
Notes
Peer review under responsibility of Kalasalingam University.
References
- Beck I. Coloring of commutative rings J. Algebra 116 1988 208 226
- Anderson D.F. Livingston P.S. The zero-divisor graph of a commutative ring J. Algebra 217 1999 434 447
- Chen P.W. A kind of graph structure of rings Algebra Colloq. 10 2 2003 229 238
- Li A. Li Q. A kind of graph of structure on von-Neumann regular rings Int. J. Algebra 4 6 2010 291 302
- Chiang-Hsieh H.J. Classification of rings with projective zero-divisor graphs J. Algebra 319 2008 2789 2802
- Atiyah M.F. Macdonald I.G. Introduction to Commutative Algebra 1969 Addison–Wesley Reading, MA
- White T. Graphs, Groups and Surfaces North-Holland Mathematics Studies vol. 188 (1984) North-Holland. Amsterdam.
- Chartrand G. Lesniak L. Graphs and Digraphs 1986 Wadsworth and Brooks/Cole Monterey, CA
- Kala R. Kavitha S. On nilpotent graph of genus one Discrete Math. Algorithms Appl. 63 2014 1450037(1–10)
- Ellingham M.N. Stephens C. The nonorientable genus of joins of complete graphs with large edgeless graphs J. Combin. Theory Ser. B 97 2007 827 845
- Negami Seiya Suzuki Yusuke The 2-extendability of 5-connected graphs on the Klein bottle Discrete Math. 310 2010 2510 2518
- Gitler I. Reyes E. Villarreal R.H. Ring graphs and complete intersection toric ideals Discrete Math. 310 3 2010 430 441
- Kammer Frank Determining the smallest k such that G is k-outerplanar Lecture Notes in Comput. Sci. 4698 2007 359 370