 ?Mathematical formulae have been encoded as MathML and are displayed in this HTML version using MathJax in order to improve their display. Uncheck the box to turn MathJax off. This feature requires Javascript. Click on a formula to zoom.
?Mathematical formulae have been encoded as MathML and are displayed in this HTML version using MathJax in order to improve their display. Uncheck the box to turn MathJax off. This feature requires Javascript. Click on a formula to zoom.Abstract
Let be a commutative ring with a proper ideal
. A generalization of total graph is introduced and investigated. It is the (undirected) graph with all elements of
as vertices, that two distinct vertices
are adjacent if and only if
where
for some
and
is a multiplicatively closed subset of
. This version of total graph is denoted by
. We in addition characterize certain lower and upper bounds for the genus of the total graph, and compute genus
on finite ring
, with respect to some special ideal
.
1 Introduction
Throughout, all rings will be commutative with non-zero identity. Let be a ring and
a proper ideal of
. The
of a commutative ring
, denoted by
, was introduced by Anderson and Badawi in [Citation1] and studied by several authors ([Citation2–4], etc.), where the authors in [Citation3,4] obtained some facts on the genus of total graphs. They considered a total graph with all elements of
as vertices, that two distinct vertices
are adjacent if and only if
where
denotes the set of all zero-divisors of
. The total graph is then extended in joint papers [Citation5,6] of the second author in rings and modules, respectively. Furthermore, a generalized total graph was introduced in [Citation7]. For a proper submodule
of
, there is a generalization of the graph of modules relative
under multiplicatively closed subset
denoted by
which was studied by present authors in [Citation8]. The vertex set of
is
, that two distinct vertices
and
are adjacent if and only if
where
for some
and
is a multiplicatively closed subset of
, i.e.
for all
. As
is a proper submodule of
and
,
is not empty.
We define a generalized total graph over ring , denoted by
, with all elements of
as vertices, that two distinct vertices
are adjacent if and only if
where
for some
,
is an ideal of
and
is a multiplicatively closed subset of
.
It follows from the definition that if , (for example, if
,
,
,
or
, by [Citation8]), then
is complete; so we suppose that
. We denote by
and
the (induced) subgraphs of
with vertices in
and
, respectively. Based on our assumption,
and so
is always nontrivial.
Let be a simple graph. We say that
is
if none of two vertices of
are adjacent. We use
to denote complete graph with
vertices. A
is a graph whose vertex set
can be partitioned into subsets
and
such that the edge set consists of precisely those edges which join vertices in
to vertices of
. In particular, if
consists of all possible such edges, then
is called the
and denoted by
when
and
. Two subgraphs
and
of
are
if
and
have no common vertices and no vertex of
(resp.,
) is adjacent (in
) to any vertex not in
(resp.,
). The
of two graphs
and
is the graph
whose vertex set is
and the edge set is
. The
of graphs
and
is defined as the graph
which the vertex set is
and the edge set is the set of all pairs
such that either
and
or
and
. Two graphs
and
are said to be
to each another, written
, if there exists a bijection
such that for each pair
of vertices of
,
if and only if
. For a vertex
of graph
,
is the degree of vertex
and
:
is a vertex of
. For a nonnegative integer
, a graph
is called
if every vertex of
has degree
. The
of a graph
, denoted by
, is the minimal integer
such that the graph can be embedded in
, where
denotes a sphere with
handles. Intuitively,
is embedded in a surface if it can be drawn in the surface so that its edges intersect only at their common vertices. A
is a graph that can be embedded in the plane, i.e., it can be drawn on the plane in such a way that its edges intersect only at their endpoints. For such graphs the genus is zero. A graph with genus one is called a
graph. If
is a subgraph of
, then
. For details on the notion of embedding of a graph in a surface, see White [Citation9, Chapter 6].
In Section 2, we remind some facts and give a lower bound for genus of the graph . We proceed in Section 3 by determining all isomorphism classes of finite rings
whose
has genus at most one (i.e. a planar or toroidal graph). Also, we compute genus of the graph over
under some well-known multiplicatively closed subsets of
.
2 Background problem and some comments
Throughout, denotes the least integer that is greater than or equal to
. In the following theorem we give some well-known formulas, see, e.g., [Citation9–11]:
Theorem 2.1
The following statements hold:
| (1) | For | ||||
| (2) | For | ||||
| (3) | Let | ||||
| (4) | The genus of a graph is the sum of the genuses of its components. | ||||
According to Theorem 2.1 we have for
,
for
and
, for other value of
.
Corollary 2.2
If is a graph with
vertices, then
.
In the following of the section, we characterize a lower bound for the genus of the graph . Considering the fact that
is in the form of
(see [Citation8, Remark 3.1]), in view of Theorem 2.1, it is enough for us to obtain a lower bound for genus of the graph
.
Theorem 2.3
[Citation8, Corollary 3.7] Let ,
, and
.Then
is a disjoint union of
copies of
.
Theorem 2.4
[Citation8, Theorem 3.10] Let and
. If
, a multiplicatively closed subset of
containing some even elements, then
is a disjoint union of
copies of
.
Corollary 2.5
Let and
. Then the following hold:
| 1. | If | ||||
| 2. | If | ||||
Proof
It is obvious by Theorems 2.3 and 2.4.
Lemma 2.6
[12 , Proposition .2.1] If is a graph with
vertices and genus
, then
.
Theorem 2.7
[Citation8 , Theorem 3.13] Suppose that the edge set of is not empty and
is a vertex of the graph. Then the degree of
is either
or
.
Proposition 2.8
Let with
vertices have a nonempty edge set, and let
. Then
.
Proof
By Lemma 2.6 and Theorem 2.7, , so
. Then
. Hence
.
Corollary 2.9
If is infinite, then
is infinite for all ideals
and all closed subsets
of
.
3 The genus of 

Considering Corollary 2.9, the genus will be infinite if is not a finite ring. In order to compute the genus, we consider a finite ring
.
In view of Theorem 2.1, it is enough for us to study the genus of .
Remark 3.1
If , then
and
is trivial so, in the following, we suppose that
. It should be noted that if
, then
.
Theorem 3.2
Let be a finite ring such that
with
,
and
be a multiplicatively closed subset of
. Then
.
Proof
It is enough to show that there is a subgraph of
with
; this implies that
. So, we proceed for
.
| 1. | Let
| ||||
| 2. | Let Claim. Let there exists (i) If (ii) Suppose ( ( Hence, | ||||
Remark 3.3
It should be noted that, Theorem 3.2 is satisfied for every with
and
.
Theorem 3.4
Let where every
is a finite ring for
,
and
be a multiplicatively closed subset of
.
| 1. | Let (i) If (ii) If | ||||
| 2. | Let there exists | ||||
Proof
| 1. | (i) Consider (ii) For a non zero element | ||||
| 2. | If there is | ||||
Corollary 3.5
Let where every
is a finite ring for
,
and
be a multiplicatively closed subset of
.
It is easily proved that the following statements hold.
| 1. | Let (i) If (ii) If | ||||
| 2. | Let there exists | ||||
Proof
It is obvious by Theorem 3.4.
Example 3.6
Let ,
and
be a multiplicatively closed subset of
. Then
. If
, then
. Let
and let
(so for every
,
, otherwise
). Then
. Considering the vertices of
in the form of
, one has
is a
.
Theorem 3.7
Let ,
where
is a ring with
,
is a field with
elements and let
be a multiplicatively closed subset of
.
| 1. | Let | ||||
| 2. | If for every | ||||
Proof
Claim; for every ,
. By way of contradiction, let there exists
such that
for some
. So
which implies that
; so
, a contradiction.
| 1. | Let (a) If (b) Let | ||||
| 2. | Let for all | ||||
Theorem 3.8
Let be a finite ring for which
with
,
,
and let
. If
, then
is isomorphic to the one of the following rings:
Proof
Note that , by Theorem 3.2.
| 1′. | Let If | ||||
| 2′. | Let For every non zero element (i) Let (ii) Let Hence, for | ||||
Proposition 3.9
Let be a finite ring for which
with
,
,
and let
. If
, then
is isomorphic to the one of the following rings:
Proof
Note that , by Theorem 3.2. Put
, by
) and
.
| 1. | Let Since (a) Let ( ( Suppose that Hence, if So, Therefore, if (b) Let | ||||
| 2. | Let ( If ( Let Hence, Thus, in this case, ( ( Hence, in this case, | ||||
Remark 3.10
Let be a ring with
and
be a multiplicatively closed subset of
. Then
is a subgraph of
, since
for some
. Recall that
is the graph with all elements of
as vertices, and two distinct vertices
are adjacent if and only if
where
denotes the set of all zero-divisors of
, so
.
For finite ring with ideal
,
and multiplicatively closed subset
of
,
is a subgraph of
, since
. For the recent inclusion, let
. Then
for some
, so
for every
. If for every
,
, then
, a contradiction, so there exists
for
. Then
implies that
, hence
.
Theorem 3.11
Let with
and
.
| 1. | Let (i) If (ii) If | ||||
| 2. | If | ||||
Proof
We at first show that . There are
such that
, so
. Hence
. We claim that
. By way of contradiction, if there exists
such that
for some
, then
. But
( since
), so
, a contradiction. Hence
| 1. | Let (i) If Let
. . .
Hence, (ii) Let
. . .
Hence, | ||||
| 2. | Let | ||||
Fig. 1 The total graph of Theorem 3.11.
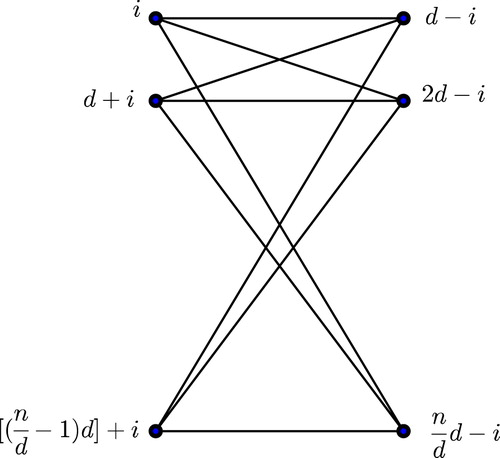
Example 3.12
Consider ,
and
. Then
is a disjoint union of
and two copies of
. Then
.
Theorem 3.13
Let ,
and
. Then:
| 1. | If | ||||
| 2. | If | ||||
Proof
| 1. | Let | ||||
| 2. | If | ||||
Example 3.14
Consider ,
and
. Then
is a disjoint union of
and 3 copies of
. Hence
.
Theorem 3.15
Let ,
where
is a prime number with
,
and
.
| 1. | If | ||||
| 2. | Let | ||||
Proof
By the proof of Theorem 3.11, , where
.
| 1. | If | ||||
| 2. | Let Let | ||||
Example 3.16
Consider ,
and
. Then
is a disjoint union of
and
such that
.
Theorem 3.17
Let ,
such that
with
,
and
.
| 1. | If there is at least one even number in | ||||
| 2. | Let there be no even number in (
| ||||
Proof
Note that by the proof of Theorem 3.11, .
| 1. | If there is at least one even element in | ||||
| 2. | Let there be no even element in Conversely, we show that Let (
| ||||
Example 3.18
Consider ,
and
. Then
is a disjoint union of
copies of
and
.
Theorem 3.19
Let ,
with
,
and
.
| 1. | If | ||||
| 2. | let
( | ||||
Proof
| 1. | Let | ||||
| 2. | By the proof of Theorem 3.11, ( ( | ||||
Example 3.20
Consider ,
and
. Then
is a disjoint union of two copies of
and
.
Theorem 3.21
Let ,
,
with
,
and
.
| 1. | If | ||||
| 2. | Let (i) If (ii) If | ||||
Proof
| 1. | If | ||||
| 2. | Let | ||||
Example 3.22
Consider ,
and
. Then
is a disjoint union of
copies of
and
.
Theorem 3.23
Let denote the field of
elements where
is a prime number,
where
and
. Let
be one of the following sets:
, …,
.
| 1. | If | ||||
| 2. | If | ||||
Proof
We note that for every positive integer
and all cases of
. Otherwise, there exists
such that
and
for some
, where
. Because
, so
, a contradiction. Hence, in all cases,
.
| 1. | If | ||||
| 2. | In the same way of the case 1, for | ||||
Fig. 2 The total graph of Theorem 3.23 (1).
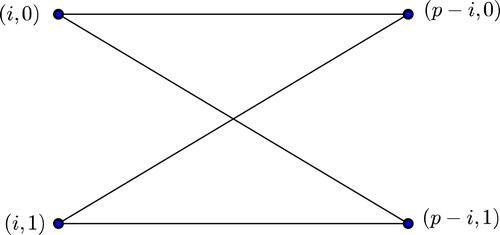
References
- AndersonD.F.BadawiA., The total graph of a commutative ring J. Algebra 320 7 2008 2706–2719
- AkbariS.KianiD.MohammadiF.MoradiS., The total graph and regular graph of a commutative ring J. Pure Appl. Algebra 213 12 2009 2224–2228
- MaimaniH.R.WickhamC.YassemiS., Rings whose total graphs have genus at most one Rocky Mountain J. Math. 42 5 2010 1551–1560
- Tamizh ChelvamT.AsirT., On the genus of the total graph of a commutative ring Comm. Algebra 412013 142–153
- AbbasiA.Sh. Habibi., The total graph of a commutative ring with respect to proper ideals J. Korean Math. Soc. 49 1 2012 85–98
- AbbasiA.Sh. Habibi., The total graph of a module over a commutative ring with respect to proper submodules J. Algebra Appl. 11 3 2012 125004813 pages
- AndersonD.F.BadawiA., The generalized total graph of a commutative ring J. Algebra Appl. 12 5 2013 125021218 pages
- AbbasiA.Hamidian JahromiL., A generalization of total graphs of modules over commutative rings under multiplicatively closed subsets J. Math. Ext. 11 3 2017 87–102
- WhiteA.T., Graphs, Groups and Surfaces1973North-HollandAmsterdam
- BattleJ.HararyF.KodamaY.YoungsJ.W.T., Additivity of the genus of a graph Bull. Amer. Math. Soc. 68 6 1962 565–568
- WhiteA.T., The genus of the Cartesian product of two graphs J. Combin. Theory Ser. B 11 2 1971 89–94
- WickhamC., Classification of rings with genus one zero-divisor graphs Comm. Algebra 36 2 2008 325–345
Further reading
- GodsilC.RoyleG. Algebraic Graph Theory 2001 SpringerNew York
