Molecular theory of the genetic code

Abstract

This article honours Michael Baer on the occasion of his 80th birthday and celebrates his scientific contributions to non-adiabatic chemical physics. This undertaking prompts the presentation of a first principles molecular theory of the genetic code. Jacques Monod’s classic essay, ‘Chance and Necessity’, is exercised as a platform for this discussion. In particular the controversial concept of teleonomy is considered and evaluated in relation to modern developments in chemical physics.

GRAPHICAL ABSTRACT

KEYWORDS:

• Density matrix
• non-adiabatic theory
• genetic code
• Poisson distribution
• correlated dissipative structure

1. Special remarks

I first met Michael Baer during one of Per-Olov Löwdin’s International Summer Institutes in Quantum Chemistry in the late sixties [1], held both in Uppsala, Sweden, and in Beito, Norway. These gatherings were all very popular and drew more than 100 participants each year with typically a large contingent of members from Israel – an effect of the conscription not the least due to one of the most popular lecturers of the institute, Ruben Pauncz [2].

This was an outstanding group of creative, noisy and wonderful people, making long-lasting contributions to the timely evolution of the School. Not only were these significant events in the history of quantum chemistry, they also stamped on our memory with historic significance. In 1967, some of the students came to the institute directly from the six-day war, changing their army boots back to sneakers, all things considered an arduous experience for those involved, almost impossible to fully and empathetically comprehend. At the end of the institute, the transport from Norway back to Uppsala travelled under special permission, since Sweden did change from left-hand to right-hand traffic during that night, a ‘danger’ of a different sort. In 1969, the institute commenced a few days after Neil Armstrong announced, ‘the Eagle has landed’, which conferred a special preamble for the optimism that permeated the almost unlimited promises of scientific and technological progress.

In this milieu, Michael Baer excelled as an especially creative and most inquisitive student later heading for an excellent career in chemical physics, culminating as research leader and Head of the Department of Physics and Applied Mathematics at Soreq, Yavne, and then, after retirement, associated with the Fritz Haber Center for Molecular Dynamics, Institute of Chemistry, The Hebrew University of Jerusalem.

His pioneering research tackled front-line, innovative contributions to contemporary and still prevalent topics like conical intersections, complex-absorbing potentials and recently to the study of molecular Maxwell–Lorentz Fields. In the book ‘Beyond Born-Oppenheimer: Electronic Nonadiabatic Coupling Terms and Conical Intersections’ [3], Michael Baer developed powerful approaches to non-adiabatic theories. His deep-seated work, in particular with Daniel Neuhauser, on molecular scattering theory, did, e.g. draw more than 20 references in the review by Muga et al. [4], acknowledging the importance of his research. Yet, the most adequate impact for this celebratory article is his inadvertent contribution to the fundamental theme that is the subject of this study.

2. Introduction

The genetic code, well known today, entrenched in the molecular structure, discovered by Watson and Crick [5], constitutes a basic axiom of biology. Yet, there does not exist a fundamental theory that translates a molecular structure into some kind of code for communication and information processing. Jacques Monod, in his timeless essay, Chance and Necessity [6], struggled from the beginning with this conundrum. Already at the start, he averred a possible distinction between living beings and all other objects, through three quantitative properties, teleonomy, automorphous morphogenesis and reproductive invariance. He explained that with a teleonomic structure, he meant the following, viz, ‘all its performances can be regarded as corresponding to a certain quantity of information, which must be transmitted for these structures to be realized and the performances accomplished’.

Principally, he singled out invariant reproduction as devoid of teleonomy, linking the genetic organisation exclusively to that of nucleic acids, while the proteins were responsible for all the teleonomic structures. He ranked teleonomy as a secondary concept derived from primary invariance alone, which he found consistent with the postulate of objective science. Unfortunately, the story does not end there, since the primary character of living organisms admits teleonomic features, and quoting Monod: ‘Here therefore, at best in appearance, lies a profound epistemological contradiction. In fact the central problem of biology lies with this very contradiction’.

The great evolutionist Ernst Mayr [7] did put teleonomic processes on par, but thus far unaccounted for, with the known physical laws, characterising them as follows: ‘a teleonomic process or behaviour is one that owes its goal-directedness to the influence of an evolved program’. All things considered Jacques Monod in 1969 was pessimistic: ‘I believe that we can assert today that a universal theory, however completely successful in other domains, could never encompass the biosphere, its structure, and its evolution as phenomena deducible from first principles’.

The obvious conclusion befalls that there stands a vital gap in the basic understanding of Nature. A lot has become known since the sixties, see e.g. the development of Prigogine’s theory of subdynamics [8], with its time-directed, probabilistic star-unitary transformation approach to non-equilibrium statistical mechanics, instigating, from first principles, irreversibility and self-organisation in so-called dissipative systems, and (see e.g. [9]) the progress of treating unstable states in the continuous spectra on a rigorous mathematical basis. Nevertheless, the inconsistency formulated above remains, and to formulate a relevant theoretical agenda requires several makings. Among many qualities, biological systems are sensitive to temperatures and exhibit long-range correlations. Within this framework we will proceed in six steps:

1. Combine strongly quantum correlated situations with thermal fluctuations;

2. Establish correlated dissipative systems and their transformation properties;

3. Generate the time evolution, the appropriate statistics and microscopic self-organisation;

4. Translate stored information from the lower micro- to the higher mesoscopic level;

5. Establish the correlated dissipative ensemble (CDE) and its transformation properties;

6. Establish evolution of the biosphere from first principles.

Note that steps 1–3 concern reproductive invariance on the level of nucleic acids, while 4 and 5 refer to structural teleology on the level of proteins, and point 6 to a wide-ranging formulation that integrates a hierarchy, from the molecular to the cosmological rank.

3. Theoretical framework

One of the most basic molecular interactions, of great significance in the biosphere, is the hydrogen bond. Its history goes back a long way as already revealed in Pauling’s classic The Nature of the Chemical Bond [10]. It provides the molecular understanding of the structure of DNA/RNA – its build-up and the properties of our genes. The universality of the code and its translation mechanism, however, is a tall riddle. In consonance with Monod one can devise two main possibilities: (1) chemical – or stereo chemical – reasons account for the structure of the code, or (2) this structure is chemically arbitrary. While (2) prompts speculation, (1) is more appealing since it would be verifiable. Hence one needs to establish a constructive principle that is commensurate with (1) without being in conflict with the laws of physics.

As step 1, see the previous section, we will consider the emergence of quantum and thermal correlations in an open system, i.e. a system where there exists a flow of entropy, due to exchanges of energy or matter with the environment. Such theories do exist [8], as said above, but they are not specific enough to confront biological processes in complex enough systems. One very important aspect is the query whether pioneering quantum mechanics would be a sufficiently general framework for delivering the first step? Obviously the answer is no, since thermal systems in equilibrium are not isolated. Furthermore, one needs to embark on a density matrix formulation, which is flexible enough to extend molecular dynamics beyond the adiabatic approximation – and moreover exhibiting original time irreversibility, a characterising trait of non-equilibrium biological systems.

The specifics of this elaboration will not be presented here, but we will give some abbreviated guidance in the Appendices; for more theoretical details, see also [11]. Some relevant condensed matter applications, where quantum and thermal correlations become constructive, are presented in [12], propagating spin waves above the Curie point in ferromagnetic systems; in [13], proton transfer in aqueous solutions and the anomalous decrease of proton mobility in H₂O/D₂O mixtures, and molar conductivities and relaxation times in molten alkali chlorides; and finally in establishing the equivalence between the BCS theory of superconductivity and Yang’s concept of ODLRO (Off-Diagonal Long-Range Order) in [14], with extensions to the treatment of alternant cuprate- and iron-based lattices [15].

As already mentioned, a higher-order dynamics formulation, based on density matrices, rather than wave functions, for dissipative (open) systems is indispensible. To accommodate solutions violating time reversibility, it is required to make extensions to non-Hermitian quantum mechanics, decidedly to make use of the mathematical theorems associated with the class of dilatation analytic potentials [9,16] – an aspect, which today has become more or less standard in modern theoretical chemical physics. The formulation displays merging quantum and thermal correlations, at the same time supporting long-range correlations, all together operating at precise temperatures. Another requirement is the need to go beyond the Born–Oppenheimer approximation [3], and to treat electrons and nuclei on a more or less equal footing [17], as prompted by the traditional, yet mostly unknown, mirror theorem [18].

4. Quantum – thermal correlations

There is a vital distinction between an ordinary thermal system at equilibrium, represented by a set of molecular vibrations, represented by harmonic oscillators, fine-tuned at a given absolute temperature T characterised by the timescale with at 310 K, and those occurring collectively in correlated systems specifically including biological self-organisational complex enough structures. In superfluids and superconductors, the generation of ODLRO [14] can be diagnosed by a large eigenvalue of the second-order reduced density matrix. However, while in conventional superconductors, the electrons cooperate through the ‘assistance’ of the attendant nuclear vibrations to provide the former with a resistant-free passage through the system, provided the temperature is low enough, the correlations, in soft condensed matter, like in melts or liquids, become more complicated as the emergence between quantum and thermal motion produce another type of long-range order (see below). The interplay between nuclear motion and electron dynamics might become ‘constructive’, which under specific conditions generate relaxation processes controlled by a higher-order timescale , usually many times larger than .

In these cases, the setting up authorises thermalisation of an apposite strongly correlated second-order reduced density matrix of particle states, exhibiting Yang’s ODLRO, through the Bloch equation (see Appendix 1 for details). (1) In Equation (1) above, is the Prigogine energy super-operator expressed in terms of a suitable Hamiltonian H properly extended to the non-Hermitian case and with , where k is the Boltzmann constant; for more details, see also [19] and references therein.

More specifically, one may find it a bit surprising that a very simple, easy to interpret, long-range correlated will emerge at precise temperatures T, originally represented in a physical basis of molecular constituents, remembering the mirroring relationship between electron and nuclear dynamics. In particular the dimension n, of correlated localised sites in the actual system – for instance reflecting the double proton motion associated with the relevant hydrogen bonds in DNA – is linked to the inherent timescales of the system, imparted by the Bloch thermalisation boundary condition [19], and linking the temperature with the appropriate timescales of the system (see also Appendix 2). (2) In this physical basis, one obtains after thermalisation [11,19] the following density matrix , with , and represented by the irreducible matrix , in the basis (3) with (4) Surprisingly turns out to be a complex symmetric (irreducible) Jordan block, which cannot be diagonalised – an accessible possibility offered by dissipative dynamics [19,20]. Under the transformation , given by, with (5) one obtains the transition matrix [21,22] (6) i.e. the transformation of the basis brings the complex symmetric Jordan block to classical canonical form with the matrix given by (7) i.e. a matrix with zeros everywhere except with ‘ones’ above the diagonal.

The thermal excitation of the system, represented by in Equation (7) above, reveals that the system under consideration converts from a standard representation in terms of physical states to a Correlated Dissipative Structure (CDS) represented by the transition density two-matrix as given by Equation (6). It is important to appreciate that the presently appearing non-equilibrium situation, i.e. the system undergoing transitions between n delocalised sites, specified by the states , depends explicitly on the temperature and, through the Bloch thermalisation, on the appropriate timescales (Equation (2)). As expected, the time evolution of Equation (6), for some , is neither unitary nor even decaying; rather, as can be easily demonstrated, it is Poissonian (with ), where below is the ‘quantity’ at time t, defined in Equation (17) below. (8) It is easy to demonstrate that microscopic self-organisation is a consequence of Equation (8) (see e.g. [11] and Appendix 2).

Since the change of the physical basis is given by (or since it is unitary), one obtains the classical canonical Jordan form of , i.e. (9) with the transformations (and ) becoming key quantities in the ensuing sections, as will be clear below. In terms of the standard algebraic form notation, the covariant transformation here turns out to be (or ) (see Equations (6) and (7)), and with the contravariant one becoming . The latter exhibits a remarkable factoring characteristic, while the columns of reveal a systematic passing of the equidistant points on the unit circle in the complex phase plane.

In summary, we have derived from first principles a correlated dissipative form of dynamics and their transformation properties, merging quantum and thermal fluctuations, and being subject to Poisson dynamics, including microscopic self-organisation. Note also the early applications to soft condensed matter, where in particular a delicate temperature dependence of pH in aqueous solutions related to excess mobility of hydrogen (and hydroxyl) ions [11,13] might be of particular relevance. The first three points, documented in the introduction, have now been accounted for.

5. Communication pure and simple

While moving from the level of our genes to the protein machinery, one cannot avoid mentioning that certain DNA/RNA structures play a role that must be considered teleonomic. The interaction between proteins and nucleic acids prompts an analysis and interpretation of teleonomic molecular interactions. This is further underlined by the fact that the structure of the transformation B (see Equation (5)) is already implicitly attached to the points 1–3. The piecing together will be clear further below.

The mechanisms that translate the genetic code are fundamentally far more complex than anything up till now experienced, since they combine different levels of organisation. For instance, a given non-covalent protein has a precise structure and shape that constitutes particular stereospecific discriminations on a substrate of complexity, on which its function depends, while the hydrogen bonded building blocks of DNA yield a basic chemical understanding of its helical structure, yet subtly dependent on the nucleotide sequence. Monod solved this dilemma by making clear his position in relation to physical laws, i.e. attempting to separate ‘the two essential properties that characterize living organisms: reproductive invariance and structural teleonomy’.

The non-covalent traits of folded proteins do constitute a coherent and integrated functional unit, whose inner workings is an elaborate apparatus that feeds on teleonomy, eventually leading to the primitive cell and to wit the central nervous system of living beings. The applied mathematician George Hall, noted in his Recollection and Reflections, published in a Special Issue of the International Journal of Quantum Chemistry to his honour [23], from earlier studies of his research group, on phenethylalamine and amphetamine [24], ‘that their protonated forms had the side chain folded over the benzene ring’ and concluded that ‘this folding helps protect the charge during the motion of the molecule through the liquid, and so could have important implications for the biological action of these molecules’. Protein dynamics does require a new physical principle that accounts for its non-covalent details.

To continue with the steps 4–5, we will employ the CDS as basis entities for a higher-level Liouville formulation (for details, see e.g. Appendix 2 and [19,20]) (10) For instance, applying above to the CDS (see e.g. Equation (6)) gives, before the non-Hermitian extension, the sum of the energy differences , with the final outcome becoming the overall change , which is nothing but a vibrational frequency of a thermal oscillation, described by the energy super-operator (see Equation (1)), and associated with the timescale . Hence, the present Liouville picture characterises the CDS by this frequency and with the typical lifetime . A precise analytic continuation of is given in [20]. A recent review of the ingredients of the theoretical constituents can also be found in [25]. Here, in contrast to the construction in Equation (1), the real part of the eigenvalue of the Liouville operator in Equation (10) appears as an energy difference, while the imaginary part consists of widths added up to be consistent with the timescales of Equation (2).

As a consequence, one obtains a higher-order entity, e.g. from base pairs to genes, chromosomes or cells, which will be denoted as a CDE. The latter integrates a principal basis set of CDS’s defined by Equation (6), and which will be denoted by (in analogy with above), commensurate with the timescales and . Each CDS unit is, as described above, characterised by Equation (2) with its dimension, n, defining an index , serving as a quality factor for the base units of the whole ensemble, representing, e.g. a protein-based cellular component.

The higher-level CDE can be shown (see Appendix 2) to exhibit an analogical irreducible unit Q referring, as said above, to the CDS basis , and the transformed , cf. the parallel relations between and , but generally with a distinct dimension m. One key difference is that the characteristic frequency of the newly defined entity now plays the role of the shorter timescale. For instance, if we are interested in gamma wave patterns of neural oscillations in the brain, a characteristic timescale would be of the order 25 ms at human body temperatures, conceivably corresponding to perception and awareness, the phases of cognition, affection and conation demanding longer time scales (see e.g. [19] for more details).

The Liouville super-propagator, with the resolution of the identity, , now can be written as (11) (12) (13) promoting the general picture of a causal propagator and its resolvent defined by (14) and which in traditional cases relate through the standard Fourier transform. In the present situation, the extension entails the separation of positive and negative times directing our interest on the retarded–advanced propagator with integration contours running in the upper-lower complex energy plane. A detailed exposition of the connection between the correlation function and the corresponding spectrum, including analyticity requirements and appropriate integration contours, have been further discussed in the appendix of [26].

With the Liouvillian in Equation (11) inserted in Equation (14), one gets (15) (16) noting that the expansions are limited by the dimension m. The occurrence of higher order poles in Equation (16) is responsible for the presence of the polynomial in front of the decay factor in Equation (15). Hence, the conventional microscopic law of decay, i.e. , modifies according to the highest power m − 1 of . For a given k, one obtains the time-dependent transition probability from (17) which is close to maximum at . For the term with the largest value of , one gets (18) Hence N(t) increases according to Equation (18) and a new timescale, , emerges as a result of the irreducible perturbation in Equation (11). For reasons to be evident below or adding the decay time, will establish a new basic communication time scale.

Before ending the section, we will take a brief look at the matrices , and . The CDS at a given temperature T, releasing a thermal oscillation into the biological complex enough system, subject to a CDE, amounts to the transformation from the ‘local’ preferred basis to the canonical one and analogously for and , as given by or . The symmetries of the rows and columns of were shown in [27], with significant parity relations between pairs of rows and remarkable factorisation properties of its columns. In fact, any interaction between, e.g. a CDS and another CDS or CDE, like, e.g. a perception in the case of neurons in our brain, should bear the ‘parity-induced’ structure of the covariant vectors of (see Equation (9)). Adjusting to the ‘molecular’ cognition–affection–conation phase amounts to coming around to ‘translating’ the cyclic contravariant vectors of the matrix via the transformation back to the ‘physical’ basis or localised, e.g. in the cell and in the organism respectively. One might therefore regard the translation of well-matched molecular information via the communication carrying transformation as approved ‘messages’ between intra- and inter-cell entities, in particular between the molecular-DNA-RNA-gene level and the higher-level hierarchical organisation, leading to teleonomic cellular function and biological order, inciting molecular communication protocols.

It is essential to recognise that the information bearing configurations, embedded in the CDS and transduced to a CDE, are contingent on a subtle interplay between the orderly distribution of the phases of the covariant vectors and their complex conjugate pairing symmetry, and the above-mentioned factoring structure of the contravariant transformation. Moreover, the transition density matrix (Equation (6)), expressed by the representation of the molecular basis, for instance the paired proton tunnelling transfer in a base pair located in a given DNA frame, covers consecutively all the columns of defining the canonical vectors. Since through Equation (A7) in Appendix 1 can be interpreted as a successive time-unit propagation consistent with the applied phase correlation of the molecular sites, each column follows spatiotemporally in sequence. Hence, every phase point situated on the unit circle in the complex plane, commensurate with the dimension of the CDS, provides the structure also of the columns of . The latter, however, contains anticlockwise cyclic correlations of the vital locations for possible coding–encoding purposes. Obviously, the concatenated feature of the vectors of the CDS does incorporate all the canonical vectors of , which by default must contain the subspace corresponding to the non-partitioned columns of , i.e. those with no apparent coding information.

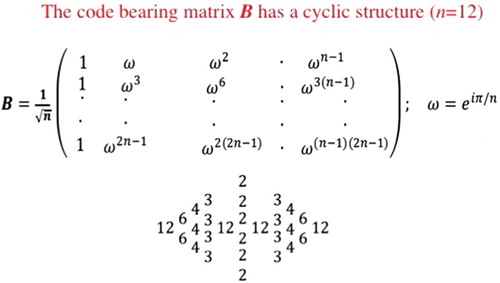
The transformation is a hidden treasure that conveys the statistical formulation of both the base units, defining the CDS, and the higher-level entities, the CDE. The unitary transformation of dimension n, given in Equation (5), displays as already said above, an important cyclic structure of its columns, given by the occurrence of the various powers of . For a given quality factor n, the matrix shows a unique factorisation property of its associated canonical basis vectors, incorporating the possibility of encryption and self-similar, recursive structures. For instance, the case n = 12 yields, removing the first column of ones (19) Rewriting the graph above as seven non-commuting factors, including the first row of (1)’s and leaving out the final 5 symmetric repetitions in Equation (19), i.e. One notes in particular the explicit factorisation structure of the transformation (Equation (5)), with unambiguous phase relations distributed repeatedly all over the CDS. Between the total number of n = 12 columns, there are 4 columns that are of the type 1 × (12), i.e. that one third of the columns cannot be factored further in contrast to the remaining two thirds. Obviously, the present choice has a particular relevance in connection with the genetic code, since the diagram above promotes codon triplets of a four-letter alphabet in various configurations of the code translation. To extend the genetic alphabet to incorporate 20 amino acids, the choice n = 12 × 5 = 60 will add columns divisible by 5, 15, 20 and 30. One observes that of a total of 60 columns, 40 cannot be factored, increasing the abundance of the latter (n = 12) from 1/3 to 2/3, a number rapidly increasing with n. Finally, one might speculate what the consequences of adding to the presence of the factors 2, 3, 4, 5, 12, 15, 20, 30 and 60 the inevitable choice of the prime number 23 proportionate with the number of human chromosomes, possibly yielding appropriate quality factors , for cellular communication and differentiation. In passing one notes that our closest living relative, the chimpanzee that branched out from a common ancestor about some five million years ago, in contrast contains 24 chromosomes. Obviously, the fusion of two chimpanzee chromosomes provided a momentous selective advantage in the process of evolution. One might speculate whether this naive number game may be of any relevance for hypotheses regarding the abundance and locations of untranslated regions, UTR’s that connect protein-coding DNA sequences – the latter often abbreviated CDS not to be confused with the current definition of a correlated dissipative structure. Yet, the concept of introns and also inteins, correspondingly for proteins, suggests a significantly integrated importance in the present view.

In previous work [19], we have further examined the significance of extending the present formulation to a higher level of neural correlates of consciousness, NCC’s. The conjecture is that the Poisson form, commensurate with the Q_f-factor representation of the cell (Equation (18)) promotes a synchronisation structure of the emerging spike trains where classes of statistical distributions with λ = l − 1 for l = 2,3, … n -1, and provides the necessary channel for communication. This protocol promotes several application areas, e.g. the interpretation of Trehub’s retinoid model for visual perceptions [28], the call centre model for neuron dynamics, and suggests interpretations for the exon–intron puzzle [19]. Furthermore, the cited property of , with every column being mirrored with its complex conjugate, gives a simple and direct explanation of the Necker-cube illusion, while the properties of the contravariant matrix elucidates the well-known fact that the memory storage in our brain miraculously inverts the image on the retina, refracted by the lens and hence flipped upside-down.

If the CDE dimensions m and the CDS quality factor n as defined above are related – they do not have to be the same just having many common divisors, not being relatively prime (one might speculate whether the appearance of large primes might lead to anomalous growth) – then there might be a trans-level communication, for instance appropriate in geno-phenotypic translations. This evokes a teleonomic principle, based on the structure of the CDS and its authority relation with the next level of description, the CDE, instigating an objective physical channel for Communication Simpliciter or Communication Pure and Simple. The previous lack of a physical rule for the gradual emergence of teleonomic systems has been a daring problem for the integration of a self-organisational status of the unicellular state, providing grounds for longstanding disagreements between molecular and evolutionary biologists.

6. Conclusion

One is still left with step 6 above, i.e. to establish, if possible, a biosphere evolution from first principles, cf. Monod’s statement in the introduction. This is a highly problematic demand, if taken literally. Nevertheless, it is possible to make some recommendations and explanations, but first a few key observations. The non-Hermitian formulation, as referred to here, requires a complex symmetric ansatz in terms of bilinear forms and extended scalar products [25]. It is possible to identify the latter with a non-positive definite metric, which sets up a direct relation with the theory of relativity [29,30], establishing the explicit correlation between space–time and momentum–matter. Since all matrices can be brought to complex symmetric form (see e.g. [21,22]), it follows that such matrices may exhibit so-called Jordan blocks.

The appearance of irreducible matrix blocks is usually a problem, which one wants avoid, but here it becomes a blessing in disguise. In addition to the possibility to map a CDS or a CDE onto a Jordan block, displaying the information bearing transformation , one can show that the appearing multi-curve-crossing structures prohibit decoherence, commensurate with its thermal structure, providing a self-referential formulation of some basic properties of general relativity, like a simple derivation of the Schwarzschild line element, evading the cosmological constant, establishing background independence and black hole-like objects. The self-referential characteristic exhibits a surprising relation to Gödel’s incompleteness theorems [19,29,30] via a conversion of an extended logical negation table to a linear algebra vernacular. All in all, it arranges for fundamental quantum theory to be applicable from the microscopic domain to the macroscopic and cosmological ranks, including teleonomic structures in biological complex enough systems.

Returning to the question of biological processes being guided by an evolved program, it is essential to develop the options given to complex enough systems in a Poisson environment. In particular, we have emphasised two important aspects, viz. the possibility of molecular aggregates to recognise each other and to communicate, both horizontally and vertically. Note that , defined above, serves as a quality index for protein processing and cellular recognition. This is in concert with certain aspects of modern signal processing techniques for communication systems, e.g. quality aspects of cavity resonators like a tuning fork on a resonance box or a musical instrument. This quality value applied, e.g. to somatic cells in a multicellular organism, provides cell recognition through the process of molecular communication, including various types of short and long range and auto signalling. It is interesting that the origin of the genetic code, through the inter-level property of molecular communication, is connected and reproduced in analogy with the dynamics of the neurons in our brain and vice versa.

At the end of his classic work [6], Monod examines the objectivity of science, ascertaining that ‘knowledge in itself is exclusive of all value judgement (all save that of ‘epistemological value’) whereas ethics, in essence non-objective, is forever barred from the sphere of knowledge’. Although there are modern propositions to use science to identify values (we will not go into this issue here), Monod continues: ‘no discourse or action is to be considered meaningful, authentic, unless – or only insofar – it makes explicit and preserves the distinction between the two categories it combines’. Despite the impossibility to formulate a ‘first commandment’ ensuring the foundation of objective knowledge, since it ends up in a self-referential statement, Monod concludes that what remains is an axiomatic value, an ethical guideline and a rule for conduct.

Rephrasing slightly, one might say that every effort to do research, i.e. to accumulate knowing by systematic observation and deliberate experiments and rational theory [31] is a priori devoid of ethical value, yet it cannot be grounded in anything else than upon a value judgement. True knowledge, ignorant of values, becomes a Gödelian statement or in the present mathematical language identified by a higher-order singularity.

In order to separate teleonomy, as a secondary concept, from objective science, one needs to examine the concept by the basic terminology of authentic knowledge of Nature. While the general concept of semiotics incorporates syntax, semantics and pragmatics, it is imperative to stress that ‘communication’ here has been defined in a restrictive sense, since it concerns no more than an objective physical channel with an unbiased syntax, for potential use in semantic and pragmatic interchange of information. As a final conclusion, it is conceivable to recover the term teleonomy as a basic biological concept, by its relation to ‘communication’, as expressed above, strictly identified as Communication Simpliciter, separate from other interpretations, which incorporate semantic and pragmatic steps in its characterisation. Since Communication Pure and Simple embeds Gödelian self-references as an arbitrary choice it subsumes axiomatic and deductive value, embodying teleonomic Darwinian evolution with a new non-probabilistic and non-deterministic paradigm.

Disclosure statement

No potential conflict of interest was reported by the author.

Appendix 1

The CDS structure

A suitable starting point is the second-order reduced density matrix for N fermions, described by the space-spin coordinates , and normalised to the number of pairings , i.e. (A1) which for the wave function ψ and some appropriate two-body potential give the energy expression (A2) An immediate conclusion from Equation (A2) is that all many-particle correlations are ‘hiding’ in as it relates back to the wave function via Equation (1). The Canadian mathematician, A. John Coleman [32,33], devoted a large part of his life to the problem of examining the basic properties of reduced density matrices. In particular, he derived the celebrated wave function representable two-matrix denoted the extreme state degenerate configuration. This reading, uniquely requiring strongly correlated conditions, cf. Yang’s concept of ODLRO [14], has a very simple form – a basic statistical argument is given in Appendix 2. (A3) with the eigenfunction corresponding to the large eigenvalue given by , and The n-dimensional matrix is represented in the space of the preferred basis , e.g. in the theory of superconductivity, they will refer to appropriate pairs of light carriers, i.e. electrons, delocalised as a superposition of basis functions centred at various nuclear centres. It is important that the density matrix should describe the full dynamics of the system, including also the nuclear motion, although we have here only indicated the electronic variables. In passing, one should observe the possibility to take the projection on the light carriers by taking the trace over the nuclear degrees of freedom, providing a Liouvillian subdynamics approach for the full dynamical description. The extreme state mentioned above corresponds to a degenerate state with one large eigenvalue, , approaching (for large n) the number of physical pairs, N/2, and the (n − 1)-degenerate small eigenvalue , approaching zero, leaving out the uninteresting unpaired background. For n equal to the number of physical pairs, N/2, one finds the independent particle model with , while for finite n the eigenvalues might be far from these limits. Utilising the transformation B (see Equation (5) above and [20,21]), can be represented in diagonal form as (A4) Employing Bloch thermalisation to the nuclear motion, utilising the mirroring relations between the light carriers and the nuclear skeleton, one obtains the representation of the complex symmetric density matrix below, with the usual energy notation, given by the standard relation between widths and lifetimes of the resonance, . Since the reference energy can be set at , and with the individual fluctuations at nuclear site k, denoted and its lifetime , one obtains (A5) One may now ask under what conditions the size (dimension) of the CDS has its maximal value, exhibiting the longest survival time. Straightforward examination tells (A6) yielding the relation (A7) and (A8) In Equation (A8), one recognizes the matrix Q defined in Equation (6). Completing the present scenario, one gets (A9) with the conditions , (A10) Note the dependence of on the dimension n, which may be significant in comparing situations involving various types of cell communications, e.g. the appearance of spike trains in neurons. Before continuing with the higher-level discussion, one observes that the Jordan block (see Equation (17)) is the fundamental reason why one obtains a time evolution that entails Poisson distributions.

Appendix 2

The CDE structure

In order to determine the higher-order dynamics, it is important to give a simplified proof of Equation (A3). This entails a quantum logical argument, whose validity depends on the interpretation of Coleman’s extreme situation (see Equation (A3)). Consider first the density operator, as above, for a general system of paired fermions described by the preferred localised basis of dimension , since we neglect the unpaired contributions the normalisation becomes as in Equation (A11) below: (A11) The matrix element defines the probability p to find the paired fermion particle at the state k (or at site k) and the probability for making the transition from site k to site l. Hence the matrix becomes (A12) or in block diagonal form as (A13) with one nondegenerate eigenvalue and a (n − 1)-degenerate eigenvalue , i.e. (A14) The representation shown above, in Equations (A13) and (A14), is essentially identical to those presented in Appendix 1 – a more exact determination of would match the extreme state exactly, but this is not essential here. Completing the model, one might consider an elementary set-up for a scattering experiment, imagining a system (I), comprising n bosonic (or paired fermionic) degrees of freedom being ‘scattered’ or correlated with system (II), the nuclear part, on a relaxation timescale given by , generally much larger than the thermal timescale. In this situation, the system (I) is open, i.e. it exchanges energy and/or entropy with its environment here system (II) and vice versa. The model defines an incoming beam of light carriers impinging on a region occupied by correlated nuclei, equal to a spherically averaged total cross-section, , consistent with the physical parameters of the system. The experiment is prepared so that on average one will detect one particle degree of freedom in the differential solid-angle element during the timescale . Hence the incident flux, of the number of particles/degrees of freedom per unit area and time comes to be (A15) The number of particles scattered into per unit time is (A16) yielding the total cross-section (A17) and the following relation between our physical parameters of the model (A18) Developing a correlated cluster of harmonic oscillators with the energies , it is possible to identify the relations (A6)–(A8).

A higher-level theory will utilise the CDS units as input, representing more complex entities, like genes, proteins, cells, and so on, with, in analogy with the model above, nucleic acids being targeted by transfer-RNA, enzymes making cells repairs and cells programmed to find their positions in a given organism. To set up such a scenario, analogous with CDS dynamics, one must be able to demonstrate the appearance of a corresponding irreducible unit Q in the CDS basis . An examination of the associated system operators reveals a simple analogy with Equations (A11) and (A18) in that its diagonal elements can be determined by a general probability measure [34], let us say , with an appropriate density operator and P(A) a suitable self-adjoint projection on Hilbert Space, and (1 − p(A))p(A) equivalently for the off-diagonal elements.

A similar analysis, cf. the derivation leading up to the CDS, constitutes through diagonalisation and thermalisation an equivalent Q (cf. Equation (6)), but generally with a distinct dimension m, in terms of and the transformed (cf. comparable relations between and ). The key difference is that the characteristic time scale for the CDS is . The CDE given by the expression (A19) contains three time scales, and a new one as obtained from Equations (17) and (18) in section 5. Owing to the factorisation property of the transformation B, the relation between the dimensions (n, m) will be crucial for the transfer of coded information between the molecular level and the higher order rank. In addition to the property of a higher-order entity, defined by its quality factor , molecular recognition, cellular communication and neuronal dynamics prompts teleonomy, as an essential biological concept.

As emphasised in the main text, Communication Simpliciter concerns communication in a restrictive sense, as stated in the main text above, founded on an unbiased syntax and an objective stochastic information channel, observing Poisson statistics, and derived from first principles. It does not by fiat contradict the objectivity of the physical laws of nature.