The spectral radius of signless Laplacian matrix and sum-connectivity index of graphs

Abstract

The sum-connectivity index of a graph G is defined as the sum of weights ${(d_u+d_v)}^{-1/2}$ over all edges uv of G, where d_u and d_v are the degrees of the vertices u and v in G, respectively. The sum-connectivity index is one of the most important indices in chemical and mathematical fields. The spectral radius of a square matrix M is the maximum among the absolute values of the eigenvalues of M. Let q(G) be the spectral radius of the signless Laplacian matrix $Q(G)=D(G)+A(G),$ where D(G) is the diagonal matrix having degrees of the vertices on the main diagonal and A(G) is the (0, 1) adjacency matrix of G. The sum-connectivity index of a graph G and the spectral radius of the matrix Q(G) have been extensively studied. We investigate the relationship between the sum-connectivity index of a graph G and the spectral radius of the matrix Q(G). We prove that for some connected graphs with n vertices and m edges, $$\frac{q(G)}{\mathit{SCI}(G)}\le \frac{n\left(n+\frac{2m}{n-1}-2\right)}{\sqrt{n}(n-1)+2m-2n+2}.$$

Keywords:

• Sum-connectivity index
• spectral radius
• signless Laplacian matrix

AMS subject classification:

• 05C50

1. Introduction

Let G be a simple connected graph with vertex set V(G) and edge set E(G). The open neighborhood of vertex v is $N_G(v)=N(v)=\{u\in V(G)|uv\in E(G)\}$ and the degree of v is $d_G(v)=d_v=|N(v)|.$ A pendant vertex is a vertex of degree one. The maximum degree of G is denoted by $\Delta (G).$ Denote by, as usual, P_n, S_n, K_n and C_n the path, the star, the complete and the cycle on n vertices, respectively. A unicyclic graph is a connected graph with n vertices and n edges. A bicyclic graph is a connected graph with n vertices and n + 1 edges. The spectral radius of a square matrix M is the maximum among the absolute values of the eigenvalues of M. Let q(G) be the spectral radius of the signless Laplacian matrix $Q(G)=D(G)+A(G),$ where D(G) is the diagonal matrix having degrees of the vertices on the main diagonal and A(G) is the (0, 1) adjacency matrix of G, see [13]. We denote the spectral radius (of the adjacency matrix A(G)) of a graph G by ${\lambda }_1(G).$ A single number that characterizes the properties of the graph of a molecular is called a graph-theoretical invariant or topological index. The structure-property relationship quantity the connection between the structure and properties of molecules.

Topological indices have been used to give a high degree of predictability of pharmaceutical properties was tasted with the help of topological indices.

The Randić connectivity index [14], $R=R(G)$ of a graph G is defined as $$R(G)=\sum _{uv\in E(G)}\frac{1}{\sqrt{d_u{d}_v}}.$$

This topological index has been successfully related to chemical and physical properties of organic molecules, and become one of the most important molecular descriptors. The sum-connectivity index of a graph G was proposed in [16], which defined as $$\mathit{SCI}(G)=\sum _{uv\in E(G)}\frac{1}{\sqrt{d_u+d_v}}.$$

The sum-connectivity index has been found to correlate well with π-electronic energy of benzenoid hydrocarbons. The Randić index and the sum-connectivity index found good approximate rather accurately the π-electron energy ($E_{\pi }$) of benzenoid hydrocarbons. The correlation coefficients between SCI(G) and $E_{\pi },$ and R(G) and $E_{\pi }$ are 0.9999 and 0.9992, respectively. The value of the correlation coefficient is 0.99088 for 136 trees representing the lower alkanes taken from Ivanciuc et al. [10], it shows that the sum-connectivity index and original Randić connectivity index are highly intercorrelated molecular descriptors. The applications of the sum-connectivity index have been investigated in [11, 12].

Some basic mathematical properties of the sum-connectivity index have been established in [1, 2, 5, 11, 12, 15, 16].

Using the AutoGraphiX system, Hansen and Lucas [8] gave a conjecture saying that if G is a connected graph having $n\ge 4$ vertices, then $$\frac{q(G)}{R(G)}\le \{\begin{array}{cc}\frac{4n-4}{n}\hfill & \hspace{1em}\text{if}\mathrm{}4\le n\le 12,\hfill \\ \frac{n}{\sqrt{n-1}}\hfill & \hspace{1em}\text{if}\mathrm{}n\ge 13,\hfill \end{array}$$ with equality if and only if G is K_n for $4\le n\le 12$ and G is S_n for $n\ge 13.$

Motivated by the works of [3, 8], we study the relationship between the sum-connectivity index SCI(G) of a graph G and the spectral radius of the signless Laplacian matrix Q(G). In particular, we prove the following theorems.

Theorem 1.

Let G be a connected graph with $n\ge 9$ vertices and m edges. If $\mathit{SCI}(G)\ge \frac{n-1}{\sqrt{n}}+\frac{2(m-n+1)}{n}$, then $$\frac{q(G)}{\mathit{SCI}(G)}\le \frac{n\left(n+\frac{2m}{n-1}-2\right)}{\sqrt{n}(n-1)+2m-2n+2}$$ with equality if and only if $G\cong S_n.$

2. Preliminaries

In this section, we present known results, which will be used in the proofs of our theorems.

The following lemma due to [7] gives an upper bound of the spectral radius of the signless Laplacian matrix, which is the main tool of our proof.

Lemma 1.

[7] Let G be a connected graph with n vertices, m edges and let q(G) be the spectral radius of the signless Laplacian matrix of G. Then $q(G)\le \frac{2m}{n-1}+n-2$ with equality if and only if G is K_n or S_n.

The following lemma due to [9] gives an upper bound of the spectral radius of the adjacency matrix.

Theorem 2.

[9] Let G be a connected graph G with n vertices, m edges and let ${\lambda }_1(G)$ be the spectral radius of the adjacency matrix of G. Then $${\lambda }_1(G)\le \sqrt{2m-n+1}$$ with equality if and only if G is K_n or S_n.

The following result comes from [6].

Theorem 3.

[6] Among the set of n-vertex unicyclic graphs with $n\ge 5,$ $$\mathit{SCI}(G)\ge \frac{2}{\sqrt{n+1}}+\frac{n-3}{\sqrt{n}}+\frac{1}{2}$$ with equality if and only if $G\cong S_n(n-1,2,2).$

The following bounds of the sum-connectivity index is well-known.

Theorem 4.

[4] Among the set of n-vertex bicyclic graphs with $n\ge 5,$ $$\mathit{SCI}(G)\ge \frac{1}{\sqrt{n+2}}+\frac{n-4}{\sqrt{n}}+\frac{2}{\sqrt{n+1}}+\frac{2}{\sqrt{5}}$$ with equality if and only if $G\cong B_n(n-1,3).$

The following results come from [16].

Theorem 5.

[16] If $n\ge 5$, then the star S_n is the unique graph with minimum sum-connectivity index among n-vertex graphs without isolated vertices.

Proposition 1.

[16] Let G be a triangle-free graph with n vertices and $m\ge 1$ edges. Then $\mathit{SCI}(G)\ge \frac{m}{\sqrt{n}}$

3. Proof of Theorem 1

In this section we prove our main result.

Now we consider graphs with $n\ge 9$ vertices, m edges with the sum-connectivity index at least $\frac{n-1}{\sqrt{n}}+\frac{2(m-n+1)}{n}.$

Theorem 6.

Let G be a connected graph with $n\ge 9$ vertices and m edges. If $\mathit{SCI}(G)\ge \frac{n-1}{\sqrt{n}}+\frac{2(m-n+1)}{n}$, then $$\frac{q(G)}{\mathit{SCI}(G)}\le \frac{n\left(n+\frac{2m}{n-1}-2\right)}{\sqrt{n}(n-1)+2m-2n+2}$$ with equality if and only if $G\cong S_n.$

Proof.

Since $\mathit{SCI}(G)\ge \frac{n-1}{\sqrt{n}}+\frac{2(m-n+1)}{n},$ we deduce from Lemma 1 that $q(G)\le \frac{2m}{n-1}+n-2$ with equality if and only if G is either K_n or S_n. Hence, $$\frac{q(G)}{\mathit{SCI}(G)}\le \frac{\frac{2m}{n-1}+n-2}{\frac{n-1}{\sqrt{n}}+\frac{2(m-n+1)}{n}}=\frac{\left(\frac{n-1}{\sqrt{n}}+\frac{2(m-n+1)}{n}\right)\left(\frac{n\left(n+\frac{2m}{n-1}-2\right)}{\sqrt{n}(n-1)+2m-2n+2}\right)}{\frac{n-1}{\sqrt{n}}+\frac{2(m-n+1)}{n}}=\frac{n\left(n+\frac{2m}{n-1}-2\right)}{\sqrt{n}(n-1)+2m-2n+2}.$$

Since for K_n we have $m=\frac{n(n-1)}{2},$ therefore, we get $\frac{n-1}{\sqrt{n}}+\frac{2(m-n+1)}{n}=n+\sqrt{n}-3-\frac{1}{\sqrt{n}}+\frac{2}{n}.$ Note that $\mathit{SCI}(K_n)=\frac{n\sqrt{2n-2}}{4}>n+\sqrt{n}-3-\frac{1}{\sqrt{n}}+\frac{2}{n}$ for $n\ge 9,$ hence, $\frac{q(K_n)}{\mathit{SCI}(K_n)}<\frac{n\left(n+\frac{2m}{n-1}-2\right)}{\sqrt{n}(n-1)+2m-2n+2}.$ For the graph S_n we have $m=n-1.$ Hence, $\mathit{SCI}(S_n)=\frac{n-1}{\sqrt{n}}=\frac{n-1}{\sqrt{n}}+\frac{2(m-n+1)}{n},$ therefore, $\frac{q(S_n)}{\mathit{SCI}(S_n)}=\frac{n^{3/2}}{n-1}$ which implies that $\frac{q(G)}{\mathit{SCI}(G)}\le \frac{n\left(n+\frac{2m}{n-1}-2\right)}{\sqrt{n}(n-1)+2m-2n+2}$ with equality if and only if $G\cong S_n.$ □

In the next result, we will show that our theorem holds for trees and graphs satisfying the inequality $m\ge n+1+\frac{6}{n-4}.$

Theorem 7.

Let G be a triangle-free graph with $n\ge 9$ vertices. Then

1. If $m=n-1$, then $\frac{q(G)}{\mathit{SCI}(G)}\le \frac{n\left(n+\frac{2m}{n-1}-2\right)}{\sqrt{n}(n-1)+2m-2n+2}$ with equality if and only if $G\cong S_n.$

2. If $m\ge n+1+\frac{6}{n-4}$, then $$\frac{q(G)}{\mathit{SCI}(G)}<\frac{n\left(n+\frac{2m}{n-1}-2\right)}{\sqrt{n}(n-1)+2m-2n+2}.$$

Proof.

By Proposition 1, we have $\mathit{SCI}(G)\ge \frac{m}{\sqrt{n}}.$ Assuming that $g(m,n)=\mathit{SCI}(G)-\frac{n-1}{\sqrt{n}}-\frac{2(m-n+1)}{n},$ we get $$\begin{array}{c}g(m,n)\ge \frac{m}{\sqrt{n}}-\frac{n-1}{\sqrt{n}}-\frac{2(m-n+1)}{n}=\frac{m\sqrt{n}-(n-1)\sqrt{n}-2(m-n+1)}{n}\\ =\frac{f(m,n)}{n}.\end{array}$$

We consider some partial derivatives of f(m, n), i.e. (1) $$\frac{\partial f(m,n)}{\partial m}=\sqrt{n}-2\ge 0.$$(1)

If $m=n-1,$ then $f(m,n)=0$ and $g(m,n)\ge 0,$ hence $\mathit{SCI}(G)\ge \frac{n-1}{\sqrt{n}}+\frac{2(m-n+1)}{n}.$ Therefore, by Theorem 6, we have $\frac{q(G)}{\mathit{SCI}(G)}\le \frac{n\left(n+\frac{2m}{n-1}-2\right)}{\sqrt{n}(n-1)+2m-2n+2}$ and equality holds if and only if $G\cong S_n.$

If $m\ge n+1+\frac{6}{n-4},$ then by Inequality (1) we can write $$\frac{m\sqrt{n}-(n-1)\sqrt{n}-2(m-n+1)}{n}\ge \frac{2}{\sqrt{n}}+\frac{6\sqrt{n}-4n-8}{n^2-n}>0,$$ hence, we get $g(m,n)\ge 0.$ Therefore, we have $\mathit{SCI}(G)\ge \frac{n-1}{\sqrt{n}}-\frac{2(m-n+1)}{n}.$ Since G is not S_n, by Theorem 6, we obtain $\frac{q(G)}{\mathit{SCI}(G)}<\frac{n\left(n+\frac{2m}{n-1}-2\right)}{\sqrt{n}(n-1)+2m-2n+2}.$□

Here, we solve our problem for the unicyclic graph.

Theorem 8.

Let G be a connected graph with $n\ge 9$ vertices and m = n edges. Then $$\frac{q(G)}{\mathit{SCI}(G)}<\frac{n\left(n+\frac{2m}{n-1}-2\right)}{\sqrt{n}(n-1)+2m-2n+2}.$$

Proof.

If m = n, then G is a unicyclic graph and by Theorem 3 we have $$\mathit{SCI}(G)\ge \frac{2}{\sqrt{n+1}}+\frac{n-3}{\sqrt{n}}+\frac{1}{2}=\frac{4\sqrt{n}+2(n-1)\sqrt{n+1}+\sqrt{n^2+n}}{2\sqrt{n^2+n}}.$$

It can be checked that $\frac{4\sqrt{n}+2(n-1)\sqrt{n+1}+\sqrt{n^2+n}}{2\sqrt{n^2+n}}>\frac{n-1}{\sqrt{n}}+\frac{2}{n}$ holds for $n\ge 2.$ Then from Theorem 6, we obtain $\frac{q(G)}{\mathit{SCI}(G)}<\frac{n\left(n+\frac{2m}{n-1}-2\right)}{\sqrt{n}(n-1)+2m-2n+2}.$□

Now, we solve our problem for the bicyclic graph.

Theorem 9.

Let G be a connected graph with $n\ge 9$ vertices and $m=n+1$ edges. Then $$\frac{q(G)}{\mathit{SCI}(G)}<\frac{n\left(n+\frac{2m}{n-1}-2\right)}{\sqrt{n}(n-1)+2m-2n+2}.$$

Proof.

If $m=n+1,$ then G is a bicyclic graph and by Theorem 4 we have $$\mathit{SCI}(G)\ge \frac{1}{\sqrt{n+2}}+\frac{n-4}{\sqrt{n}}+\frac{2}{\sqrt{n+1}}+\frac{2}{\sqrt{5}}.$$

It can be checked that $\frac{1}{\sqrt{n+2}}+\frac{n-4}{\sqrt{n}}+\frac{2}{\sqrt{n+1}}+\frac{2}{\sqrt{5}}>\frac{n-1}{\sqrt{n}}+\frac{4}{n}$ holds for $n\ge 6.$ Then from Theorem 6, we obtain $\frac{q(G)}{\mathit{SCI}(G)}<\frac{n\left(n+\frac{2m}{n-1}-2\right)}{\sqrt{n}(n-1)+2m-2n+2}.$□

From Theorems 7, 8 and 9 we obtain the best possible bound on $\frac{q(G)}{\mathit{SCI}(G)}$ for a connected graph G having $n\ge 10$ vertices.

Corollary 1.

Let G be a connected graph with $n\ge 10$ vertices. Then $$\frac{q(G)}{\mathit{SCI}(G)}\le \frac{n\left(n+\frac{2m}{n-1}-2\right)}{\sqrt{n}(n-1)+2m-2n+2}$$ with equality if and only if $G\cong S_n.$

Proof.

Note that $n+2\ge n+1+\frac{6}{n-4}$ for $n\ge 10.$ From Theorem 7, the result holds if $n\ge 10$ and $m\ge n+2.$ By Theorems 8 and 9, the result holds for graphs G with m = n and $m=n+1,$ and by Theorem 7, the result holds for graphs G with $m=n-1$ with equality if and only if G is S_n.□

From Theorems 7, 8 and 9 we also know that if $6\le n\le 9,$ then the only cases which remain unsolved are:

1. n = 6 and m = 8, 9, 10;

2. n = 7 and m = 9, 10;

3. n = 8 and m = 10;

4. n = 9 and m = 11.

Theorem 10.

Let G be a connected graph with n vertices and m edges. If

1. n = 6 and m = 8, 9, 10;

2. n = 7 and m = 9, 10;

3. n = 8 and m = 10;

4. n = 9 and m = 11,

then $$\frac{q(G)}{\mathit{SCI}(G)}<\frac{n\left(n+\frac{2m}{n-1}-2\right)}{\sqrt{n}(n-1)+2m-2n+2}.$$

Proof.

From Theorem 5, we obtain the lower bounds on the sum-connectivity index of G. Hence, $\mathit{SCI}(G)>2.3674542494839$ if n = 6 and m = 8, $\mathit{SCI}(G)>2.5600575949894$ if n = 7 and m = 9, $\mathit{SCI}(G)>2.7465084177844$ if n = 8 and m = 10, $\mathit{SCI}(G)>2.925$ if n = 9 and m = 11, $\mathit{SCI}(G)>2.2521355971461$ if n = 6 and m = 9, $\mathit{SCI}(G)>2.1575507653593$ if n = 6 and m = 10 and $\mathit{SCI}(G)>2.443331341521$ if n = 7 and m = 10.

By Lemma 1, we obtain upper bounds on q(G). We get $q(G)\le \frac{36}{5}$ if n = 6 and m = 8, $q(G)\le 8$ if n = 7 and m = 9, $q(G)\le \frac{62}{7}$ if n = 8 and m = 10, $q(G)\le \frac{39}{4}$ if n = 9 and m = 11, $q(G)\le \frac{38}{5}$ if n = 6 and m = 9, $q(G)\le 8,$ if n = 7 and m = 10 and $q(G)\le \frac{25}{3},$ if n = 6 and m = 10.

It is easy to verify that $\frac{q(G)}{\mathit{SCI}(G)}<\frac{n\left(n+\frac{2m}{n-1}-2\right)}{\sqrt{n}(n-1)+2m-2n+2}$ for all these cases.□

From Theorems 7, 8, 9 and 10, we get the next result.

Corollary 2.

Let G be a connected graph with n vertices for $6\le n\le 9$. Then $$\frac{q(G)}{\mathit{SCI}(G)}<\frac{n\left(n+\frac{2m}{n-1}-2\right)}{\sqrt{n}(n-1)+2m-2n+2}.$$

Finally, we solve our problem for connected graph with $n=3,4,5.$

Theorem 11.

Let G be a connected graph with 3 vertices. Then $$\frac{q(G)}{\mathit{SCI}(G)}\le \frac{8}{3}$$ with equality if and only if $G\cong K_3.$

Proof.

For n = 3, there are only two non-isomorphic graphs: K₃ and $K_3-\left\{e\right\},$ where $e\in E(K_3).$ We have $\mathit{SCI}(K_3)=\frac{3}{2}$ and by Lemma 1, $q(K_3)=4,$ thus $\frac{q(K_3)}{\mathit{SCI}(K_3)}=\frac{8}{3}.$

For $K_3-\left\{e\right\}$ we obtain $\mathit{SCI}(K_3-\{e\})=\frac{2}{\sqrt{3}}$ and by Lemma 1, $q(K_3-\{e\})\le 3,$ so $\frac{q(K_3-\{e\})}{\mathit{SCI}(K_3-\{e\})}=\frac{3\sqrt{3}}{2}.$ Hence $\frac{q(G)}{\mathit{SCI}(G)}\le \frac{8}{3}$ for any graph G having 3 vertices with equality if and only if G is K₃.□

Here, we solve our problem for connected graphs on 4 vertices.

Theorem 12.

Let G be a connected graph with 4 vertices. Then $$\frac{q(G)}{\mathit{SCI}(G)}\le \frac{8}{3}$$ with equality if and only if $G\cong S_4.$

Proof.

The only graph with 4 vertices and 6 edges is K₄, and the only graph with 4 vertices and 5 edges is $K_4-\left\{e\right\}.$ Since $\mathit{SCI}(K_4)=\sqrt{6}$ and $q(K_4)=6$ (by Lemma 1), we get $\frac{q(K_4)}{\mathit{SCI}(K_4)}=\sqrt{6}<\frac{8}{3}.$ For $K_4-\left\{e\right\}$ where $e\in E(K_4-\{e\}),$ we obtain $\mathit{SCI}(K_4-\{e\})=\frac{\sqrt{6}}{6}+\frac{4\sqrt{5}}{5},$ and from Lemma 1 we have $q(K_4-\{e\})\le \frac{16}{3},$ which gives $\frac{q(K_4-\{e\})}{\mathit{SCI}(K_4-\{e\})}=\frac{128\sqrt{5}}{91}-\frac{80\sqrt{6}}{273}<\frac{8}{3}.$ We have two non-isomorphic graphs for m = 4, namely C₄ and $S_4+\left\{e\right\}.$ We get $\mathit{SCI}(C_4)=2$ and $q(C_4)\le \frac{14}{3}$ (by Lemma 1), so $\frac{q(C_4)}{\mathit{SCI}(C_4)}\le \frac{7}{3}<\frac{8}{3}.$ Similarly, $\mathit{SCI}(S_4+\{e\})=\frac{2\sqrt{5}}{5}+1$ and $q(S_4+\{e\})\le \frac{14}{3},$ thus $\frac{q(S_4+\{e\})}{\mathit{SCI}(S_4+\{e\})}\le \frac{70-28\sqrt{5}}{3}<\frac{8}{3}.$ 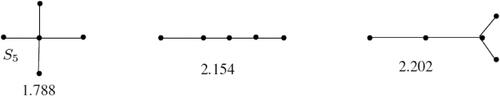There are two non-isomorphic graphs for m = 3, namely S₄ and P₄. We have $\mathit{SCI}(S_4)=\frac{3}{2}$ and $q(S_4)=4$ (by Lemma 1), thus $\frac{q(S_4)}{\mathit{SCI}(S_4)}=\frac{8}{3}.$ Also $\mathit{SCI}(P_4)=\frac{2\sqrt{3}}{3}+\frac{1}{2}$ and $q(P_4)\le 4$ (by Lemma 1), thus $\frac{q(P_4)}{\mathit{SCI}(P_4)}\le \frac{32\sqrt{3}-24}{13}<\frac{8}{3}.$□

Here, we solve our problem for connected graphs on 5 vertices.

Theorem 13.

Let G be a connected graph with 5 vertices. Then $$\frac{q(G)}{\mathit{SCI}(G)}\le \frac{5\sqrt{5}}{4}$$ with equality if and only if $G\cong S_5.$

Proof.

We consider the cases $m=7,8,9,10.$ The only graph with 5 vertices and 10 edges are K₅. Since $\mathit{SCI}(K_5)=\frac{5\sqrt{2}}{2}$ and $q(K_5)=8$ (by Lemma 1), we get $\frac{q(K_5)}{\mathit{SCI}(K_5)}=\frac{8\sqrt{2}}{5}.$

The only graph with 5 vertices and 9 edges is $K_5-\left\{e\right\}$ where $e\in E(K_5).$ We have $\mathit{SCI}(K_5-\{e\})=\frac{3\sqrt{2}}{4}+\frac{6\sqrt{7}}{7}$ and from Lemma 1 we obtain $q(K_5-\{e\})\le \frac{15}{2},$ which gives $\frac{q(K_5-\{e\})}{\mathit{SCI}(K_5-\{e\})}\le \frac{8\sqrt{7}-7\sqrt{2}}{5}<\frac{8\sqrt{2}}{5}.$

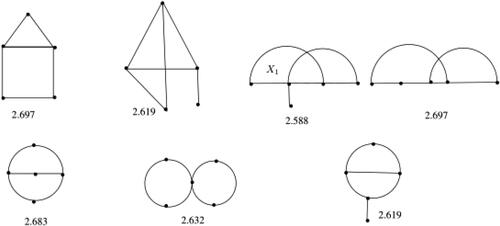
For m = 8 we have $G=K_5-\{e_1,e_2\}$ where $e_1,e_2\in E(K_5).$ There are two nonisomorphic graphs having 8 edges. If e₁ and e₂ are adjacent, then $\mathit{SCI}(K_5-\{e_1,e_2\})=\frac{\sqrt{2}}{4}+\frac{\sqrt{6}}{2}+\frac{4\sqrt{7}}{7}$ and if e₁ and e₂ are not adjacent, then $\mathit{SCI}(K_5-\{e_1,e_2\})=\frac{4\sqrt{6}}{3}.$ So, $\mathit{SCI}(K_5-\{e_1,e_2\})\ge \frac{\sqrt{2}}{4}+\frac{\sqrt{6}}{2}+\frac{4\sqrt{7}}{7}$ and from Lemma 1 we obtain $q(K_5-\{e_1,e_2\})\le 7,$ which gives $$\frac{q(K_5-\{e_1,e_2\})}{\mathit{SCI}(K_5-\{e_1,e_2\})}\le \frac{7}{\frac{\sqrt{2}}{4}+\frac{\sqrt{6}}{2}+\frac{4\sqrt{7}}{7}}=\frac{1}{\frac{\sqrt{7}}{28}+\frac{\sqrt{6}}{14}+\frac{4\sqrt{7}}{49}}<\frac{8\sqrt{2}}{5}.$$

For m = 7 we have $G=K_5-\{e_1,e_2,e_3\}$ where $e_1,e_2,e_3\in E(K_5).$ There are four non-isomorphic graphs having 7 edges. If $G[e_1,e_2,e_3]$ is K₃, then $\mathit{SCI}(K_5-\{e_1,e_2,e_3\})=\sqrt{6}+\frac{\sqrt{2}}{4}.$ If $G[e_1,e_2,e_3]$ is S₄, then $\mathit{SCI}(K_5-\{e_1,e_2,e_3\})=\frac{\sqrt{5}}{5}+\frac{\sqrt{6}}{2}+\frac{3\sqrt{7}}{7}.$ If $G[e_1,e_2,e_3]$ is a path, then $\mathit{SCI}(K_5-\{e_1,e_2,e_3\})=\frac{\sqrt{6}}{2}+\frac{2\sqrt{5}}{5}+\frac{2\sqrt{7}}{7}.$ If $G[e_1,e_2,e_3]$ is not connected ($G[e_1,e_2,e_3]$ is $K_2\cup P_3),$ then $\mathit{SCI}(K_5-\{e_1,e_2,e_3\})=\frac{2\sqrt{5}}{5}+\frac{5\sqrt{6}}{6}.$ Thus $\mathit{SCI}(K_5-\{e_1,e_2,e_3\})\ge \sqrt{6}+\frac{\sqrt{2}}{4}$ and from Lemma 1 we obtain $q(K_5-\{e_1,e_2,e_3\})\le \frac{13}{2},$ which gives $$\frac{q(K_5-\{e_1,e_2,e_3\})}{\mathit{SCI}(K_5-\{e_1,e_2,e_3\})}\le \frac{52\sqrt{6}-13\sqrt{2}}{47}<\frac{8\sqrt{2}}{5}<\frac{5\sqrt{5}}{4}.$$

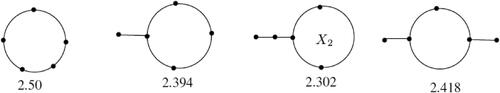
For m = 6 we have $G=K_5-\{e_1,e_2,e_3,e_4\}$ where $e_1,e_2,e_3,e_4\in E(K_5).$ There are three non-isomorphic graphs having 6 edges. If $G[e_1,e_2,e_3,e_4]$ is C₄, then $\mathit{SCI}(K_5-\{e_1,e_2,e_3,e_4\})=1+\frac{2\sqrt{6}}{3}.$ If $G[e_1,e_2,e_3,e_4]$ is a path, then $\mathit{SCI}(K_5-\{e_1,e_2,e_3,e_4\})=\frac{\sqrt{3}}{3}+\frac{\sqrt{6}}{6}+\frac{4\sqrt{5}}{5}.$ If $G[e_1,e_2,e_3,e_4]$ is not connected ($G[e_1,e_2,e_3,e_4]$ is $K_3\cup P_2),$ then $\mathit{SCI}(K_5-\{e_1,e_2,e_3,e_4\})=\frac{6\sqrt{5}}{5}.$ Hence, by Figure 1, we can see that $\mathit{SCI}(K_5-\{e_1,e_2,e_3,e_4\})\ge \frac{\sqrt{6}}{2}+\frac{2\sqrt{5}}{5}+\frac{1}{2}=X_1$ and from Lemma 1 we obtain $q(K_5-\{e_1,e_2,e_3,e_4\})\le 6,$ which gives $$\frac{q(K_5-\{e_1,e_2,e_3,e_4\})}{\mathit{SCI}(K_5-\{e_1,e_2,e_3,e_4\})}\le \frac{1}{\frac{\sqrt{5}}{15}+\frac{\sqrt{6}}{12}+\frac{1}{12}}<\frac{5\sqrt{5}}{4}.$$

Figure 1. Graphs with 5 vertices and 6 edges.

For m = 5 we have $G=K_5-\{e_1,e_2,e_3,e_4,e_5\}$ where $e_1,e_2,e_3,e_4,e_5\in E(K_5).$ There are three non-isomorphic graphs having 5 edges. By Figure 2, we can see $\mathit{SCI}(K_5-\{e_1,e_2,e_3,,e_4,e_5\})\ge \frac{\sqrt{6}}{6}+\frac{2\sqrt{5}}{5}+1=X_2$ and from Lemma 1 we obtain $q(K_5-\{e_1,e_2,e_3,e_4,e_5\})\le \frac{11}{2},$ which gives $$\frac{q(K_5-\{e_1,e_2,e_3,e_4,e_5\})}{\mathit{SCI}(K_5-\{e_1,e_2,e_3,e_4,e_5\})}\le \frac{1}{\frac{\sqrt{6}}{33}+\frac{4\sqrt{5}}{55}+\frac{2}{11}}<\frac{5\sqrt{5}}{4}.$$

Figure 2. Graphs with 5 vertices and 5 edges.

For m = 4 we have $G=K_5-\{e_1,e_2,e_3,e_4,e_5,e_6\}$ where $e_1,e_2,e_3,e_4,e_5,e_6\in E(K_5).$ There are three non-isomorphic graphs having 4 edges (see Figure 3). We can see $\mathit{SCI}(K_5-\{e_1,e_2,e_3,,e_4,e_5,e_6\})\ge \frac{4\sqrt{5}}{5}$ and from Lemma 1 we obtain $q(K_5-\{e_1,e_2,e_3,e_4,e_5,e_6\})=5,$ which gives $$\frac{q(K_5-\{e_1,e_2,e_3,e_4,e_5,e_6\})}{\mathit{SCI}(K_5-\{e_1,e_2,e_3,e_4,e_5,e_6\})}=\frac{5\sqrt{5}}{4}.$$

Figure 3. Graphs with 5 vertices and 4 edges.

□