Asymptotic analysis of the nonsteady micropolar fluid flow through a curved pipe

ABSTRACT

We consider the nonsteady flow of a micropolar fluid in a thin (or long) curved pipe via rigorous asymptotic analysis. Germano's reference system is employed to describe the pipe's geometry. After writing the governing equations in curvilinear coordinates, we construct the asymptotic expansion up to a second order. Obtained in the explicit form, the asymptotic approximation clearly demonstrates the effects of pipe's distortion, micropolarity and the time derivative. A detailed study of the boundary layers in space is provided as well as the construction of the divergence correction. Finally, a rigorous justification of the proposed effective model is given by proving the error estimates.

COMMUNICATED BY:

• Grigory Panasenko

KEYWORDS:

• Micropolar fluid
• curved pipe
• nonsteady flow
• asymptotic expansion
• error analysis

2010 MATHEMATICS SUBJECT CLASSIFICATIONS:

• 35C20
• 35Q35
• 76M45

1. Introduction

The model of micropolar fluids was first introduced in 1960s by Eringen in his well-known paper [1], providing a generalization of the classical Navier–Stokes model. The main advantage of the micropolar fluid model is due to the fact that it takes into account the microstructure of the fluid particles and effects such as rotation and shrinking. Consequently, numerous non-Newtonian fluids such as liquid crystals, animal blood, muddy fluids and even water in small scales can be described in a more precise and realistic manner. In order to describe the rotation of the particles, a new vector field is introduced – the angular velocity field of rotation (microrotation), and accordingly a new equation coming from the conservation of the angular momentum. In this way, we obtain a coupled system of partial differential equations with four new viscosity coefficients. The model of micropolar fluids has been a subject of research in both the mathematical and engineering community: a comprehensive survey of the underlying mathematical theory can be found in the monograph by Lukaszezwicz [2], whereas recent results concerning engineering applications in biomedicine and blood flow modelling can be found in [3–8].

In last two decades, one can also find a number of papers providing a rigorous derivation of new asymptotic models for steady-state flows in thin domains. The model describing the flow of Newtonian fluid in a three-dimensional curved pipe has been formally derived and justified via error estimate by Marušić–Paloka [9]. The asymptotic behaviour of a steady flow in a system of thin pipes has been considered by the same author in [10]. The steady flow of a micropolar fluid has been investigated by Dupuy, Panasenko and Stavre [11,12] in two-dimensional domains such as a periodically constricted tubes and curvilinear channels. A three-dimensional setting including an undeformed pipe and a curved pipe has been addressed by Pažanin [13,14], while multiple pipe system has been treated by Beneš and Pažanin [15].

Most recently, new asymptotic models for the nonsteady flows have been proposed and mathematically justified in case of classical, Newtonian fluid by Panasenko and Pileckas [16–18] in an infinite cylinder and a system of thin pipes. The asymptotic behaviour of nonsteady Newtonian fluid flow in a curved three-dimensional thin pipe with moving walls has been considered by Castineira et al. [19,20]). A higher-order model describing the nonsteady flow of a micropolar fluid through a straight pipe has been rigorously derived in [21,22] by the authors of this paper. In view of that and inspired by the applications, our aim here is to investigate a nonsteady micropolar flow through a pipe with an arbitrary (generic) central curve.

The paper is organized as follows. In Section 2, we formally describe the geometry of the curved pipe using Germano's frame of reference (introduced in [23,24]) and consider the equations describing the nonsteady micropolar fluid flow. In Section 3, we introduce the curvilinear coordinates and rewrite the governing problem in an undeformed pipe. In Section 4, using two-scale expansion technique, we compute the asymptotic approximation up to a second-order in order to capture the effects of the pipe's geometry, micropolarity as well as the time derivative. It should be emphasized that the asymptotic solution is provided in the form of the explicit formulae, which is particularly important with regards to numerical simulations. We also conduct a detailed boundary layer analysis in the vicinity of pipe's ends and construct the divergence correction to improve the order of accuracy of our model. In Section 5, we prove the error estimates in suitable norms evaluating the difference between the exact solution of the problem (which cannot be found due to the complexity of the flow domain and the equations) and our second-order asymptotic approximation. By doing that, we justify the usage of the proposed effective model and that represents our main contribution. We strongly believe that the presented result can be useful in the practice, especially one related to blood flow modelling. Finally, in the appendix, we provide complete expressions computed for the higher-order regular part correctors as well as for the exponentially decreasing functions appearing in the boundary layers problems.

2. The setting of the problem

2.1. Description of the pipe's geometry

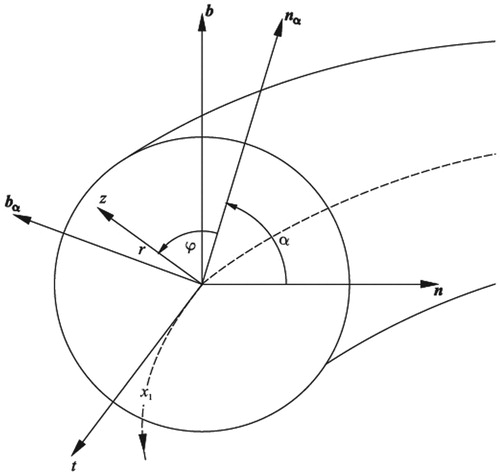
In the following, we define our thin (or long) curved pipe ${\mathrm{\Omega }}_{\epsilon }^{\alpha }$ with a smooth central curve γ and a circular cross-section. We suppose that the curve γ is a generic curve in ${\mathbb{R}}^3$ parameterized by its arc length $x_1\in [0,l]$. We denote by $\mathit{\pi }\in C^3\left(\right[0,l];{\mathbb{R}}^3)$ its natural parametrization such that ${\mathit{\pi }}^{\prime }\left(x_1\right)\ne 0$, for every $x_1\in [0,l]$. At each point, $\mathit{\pi }\left(x_1\right)$ of the curve γ we define its curvature (flexion) as $\kappa \left(x_1\right)=\left|{\mathit{\pi }}^{″}\right(x_1\left)\right|$ and introduce Frenet's basis by $\mathbf{\text{t}}={\mathit{\pi }}^{\prime },\mathbf{\text{n}}=\frac{1}{\kappa }{\mathbf{\text{t}}}^{\prime },\mathbf{\text{b}}=\mathbf{\text{t}}\times \mathbf{\text{n}},$ where $\mathbf{\text{t}}$ is the tangent, $\mathbf{\text{n}}$ the normal and $\mathbf{\text{b}}$ the binormal. The torsion of γ is denoted by $\tau \left(x_1\right)=-\left|{\mathbf{\text{b}}}^{\prime }\right(x_1\left)\right|$. It is known that the Frenet's basis $(\mathbf{\text{t}},\mathbf{\text{n}},\mathbf{\text{b}})$ satisfies the system (1) ${\mathbf{\text{t}}}^{\prime }=\kappa \mathbf{\text{n}},{\mathbf{\text{n}}}^{\prime }=-\kappa \mathbf{\text{t}}+\tau \mathbf{\text{b}},{\mathbf{\text{b}}}^{\prime }=-\tau \mathbf{\text{n}}.$(1) Let $0<\epsilon \ll 1$ be a small parameter and $B=B(0,1)\subset {\mathbb{R}}^2$ a unit circle. We define the undeformed thin pipe as ${\mathrm{\Omega }}_{\epsilon }=\{\mathit{x}=(x_1,x_2,x_3)\in {\mathbb{R}}^3:x_1\in ⟨0,l⟩,{\mathit{x}}_{\ast }=(x_2,x_3)\in \epsilon B\}=⟨0,l⟩\times B^{\epsilon }.$ Next, we introduce the mapping ${\mathrm{\Phi }}_{\epsilon }^{\alpha }:{\mathrm{\Omega }}_{\epsilon }\to {\mathbb{R}}^3$ by (2) ${\mathrm{\Phi }}_{\epsilon }^{\alpha }\left(\mathit{x}\right)=\mathit{\pi }\left(x_1\right)+x_2{\mathbf{\text{n}}}_{\alpha }\left(x_1\right)+x_3{\mathbf{\text{b}}}_{\alpha }\left(x_1\right),$(2) where (3) $\begin{array}{rl}& {\mathbf{\text{n}}}_{\alpha }\left(x_1\right)=\cos \alpha \left(x_1\right)\mathbf{\text{n}}\left(x_1\right)+\sin \alpha \left(x_1\right)\mathbf{\text{b}}\left(x_1\right),\\ & {\mathbf{\text{b}}}_{\alpha }\left(x_1\right)=-\sin \alpha \left(x_1\right)\mathbf{\text{n}}\left(x_1\right)+\cos \alpha \left(x_1\right)\mathbf{\text{b}}\left(x_1\right),\end{array}$(3) and (4) $\alpha \left(x_1\right)=-{\int }_{x_0}^{x_1}\tau \left(\xi \right)\mathrm{d}\xi +{\alpha }_0,$(4) with $x_0$ and ${\alpha }_0$ being arbitrary constants. The local injectivity of ${\mathrm{\Phi }}_{\epsilon }^{\alpha }$ can be easily established assuming ε is sufficiently small (see [14, Section 3.1] for details).

Figure 1. Germano's frame of reference.

We can now define the curved pipe with the central curve γ and circular cross-section $B^{\epsilon }$ by putting ${\mathrm{\Omega }}_{\epsilon }^{\alpha }={\mathrm{\Phi }}_{\epsilon }^{\alpha }\left({\mathrm{\Omega }}_{\epsilon }\right).$ We also denote by ${\mathrm{\Sigma }}_{\epsilon }^i={\mathrm{\Phi }}_{\epsilon }^{\alpha }\left(\right\{i\}\times B^{\epsilon }),i=0,l$ the ends of the pipe and by ${\mathrm{\Gamma }}_{\epsilon }^{\alpha }={\mathrm{\Phi }}_{\epsilon }^{\alpha }(⟨0,l⟩\times \mathrm{\partial }{B}^{\epsilon })$ its lateral boundary.

2.2. The flow equations

The equations describing the nonsteady flow of a micropolar fluid in a thin curved pipe ${\mathrm{\Omega }}_{\epsilon }^{\alpha }$ read: (5) $\begin{array}{rl}& \frac{\mathrm{\partial }{\mathbf{\text{u}}}_{\epsilon }}{\mathrm{\partial }t}-\mu \mathrm{\Delta }{\mathbf{\text{u}}}_{\epsilon }+\mathrm{\nabla }{p}_{\epsilon }=a\text{rot}{\mathbf{\text{w}}}_{\epsilon }+\mathbf{\text{F}},\\ & \text{div}{\mathbf{\text{u}}}_{\epsilon }=0,\text{in}{\mathrm{\Omega }}_{\epsilon }^{\alpha },\\ & \frac{\mathrm{\partial }{\mathbf{\text{w}}}_{\epsilon }}{\mathrm{\partial }t}-\delta \mathrm{\Delta }{\mathbf{\text{w}}}_{\epsilon }-\beta \text{div}{\mathbf{\text{w}}}_{\epsilon }+2a{\mathbf{\text{w}}}_{\epsilon }=a\text{rot}{\mathbf{\text{u}}}_{\epsilon }+\mathbf{\text{G}}.\end{array}$(5) Here ${\mathbf{\text{u}}}_{\epsilon }$ is the velocity field, $p_{\epsilon }$ is the pressure and ${\mathbf{\text{w}}}_{\epsilon }$ is the microrotation field. The external sources of linear and angular momentum are given by the functions $\mathbf{\text{F}}(t,\tilde{\mathit{x}})=\mathbf{\text{f}}(t,x_1)$ and $\mathbf{\text{G}}(t,\stackrel{\mathbf{~}}{\mathit{x}})=\mathbf{\text{g}}(t,x_1)$, where $\stackrel{\mathbf{~}}{\mathit{x}}={\mathrm{\Phi }}_{\epsilon }^{\alpha }\left(\mathit{x}\right)$. We employ the notation $\mu =\nu +{\nu }_r$, $a=2{\nu }_r$, $\delta =c_a+c_d$ and $\beta =c_0+c_d-c_a$, where ν is the Newtonian viscosity, ${\nu }_r$ is the microrotation viscosity, while $c_0,c_a$ and $c_d$ are the coefficients of angular viscosities.

We prescribe the following boundary and initial condition: (6) $\begin{array}{rl}& {\mathbf{\text{u}}}_{\epsilon }=0\text{on}{\mathrm{\Gamma }}_{\epsilon }^{\alpha },{\mathbf{\text{u}}}_{\epsilon }={\epsilon }^2{\mathbf{\text{h}}}_i^{\epsilon }\text{on}{\mathrm{\Sigma }}_{\epsilon }^i,i=0,l,\\ & {\mathbf{\text{w}}}_{\epsilon }=0\text{on}\mathrm{\partial }{\mathrm{\Omega }}_{\epsilon }^{\alpha },\\ & {\mathbf{\text{u}}}_{\epsilon }(0,\tilde{\mathit{x}})=0,{\mathbf{\text{w}}}_{\epsilon }(0,\tilde{\mathit{x}})=0,\end{array}$(6) where we suppose that the prescribed velocities ${\mathbf{\text{h}}}_0^{\epsilon },{\mathbf{\text{h}}}_l^{\epsilon }$ are given in the form (7) ${\mathbf{\text{h}}}_0^{\epsilon }(t,\tilde{\mathit{x}})=h_0^t(t,\frac{{\mathit{x}}_{\ast }}{\epsilon })\mathbf{\text{t}}\left(0\right)+h_0^n(t,\frac{{\mathit{x}}_{\ast }}{\epsilon })\mathbf{\text{n}}\left(0\right)+h_0^b(t,\frac{{\mathit{x}}_{\ast }}{\epsilon })\mathbf{\text{b}}\left(0\right),$(7) where $\stackrel{\mathbf{~}}{\mathit{x}}=\mathrm{\Phi }(0,{\mathit{x}}_{\ast })$, and (8) ${\mathbf{\text{h}}}_l^{\epsilon }(t,\tilde{\mathit{x}})=h_l^t(t,\frac{{\mathit{x}}_{\ast }}{\epsilon })\mathbf{\text{t}}\left(l\right)+h_l^n(t,\frac{{\mathit{x}}_{\ast }}{\epsilon })\mathbf{\text{n}}\left(l\right)+h_l^b(t,\frac{{\mathit{x}}_{\ast }}{\epsilon })\mathbf{\text{b}}\left(l\right),$(8) where $\stackrel{\mathbf{~}}{\mathit{x}}=\mathrm{\Phi }(l,{\mathit{x}}_{\ast })$. To assure the well-posedness of the governing problem, the following compatibility condition needs to be fulfilled: (9) $F\left(t\right)={\int }_{B^{\epsilon }}{h}_i^t(t,\frac{{\mathit{x}}_{\ast }}{\epsilon })={\epsilon }^2{\int }_B{h}_i^t(t,{\mathit{y}}_{\ast })={\epsilon }^2{F}_0^{\ast }\left(t\right),i=0,l.$(9) Since the initial velocity equals to zero, there also needs to hold (10) $F\left(0\right)=0.$(10) In the following, we suppose that ${\mathbf{\text{h}}}_0^{\epsilon },{\mathbf{\text{h}}}_l^{\epsilon },\mathbf{\text{F}}$ and $\mathbf{\text{G}}$ vanish in the neighbourhood of t=0, i.e. (11) ${\mathbf{\text{h}}}_0^{\epsilon }(t,\cdot ),{\mathbf{\text{h}}}_l^{\epsilon }(t,\cdot ),\mathbf{\text{F}}(t,\cdot ),\mathbf{\text{G}}(t,\cdot ),\mathrm{\forall }t\in [0,T^{\ast }],T^{\ast }>0.$(11) Let ${\displaystyle \frac{{\mathrm{\partial }}^j{\mathbf{\text{h}}}_0^{\epsilon }}{\mathrm{\partial }{t}^j}}\in L^2(0,T;H^{1/2}({\mathrm{\Sigma }}_{\epsilon }^0\left)\right)$ and ${\displaystyle \frac{{\mathrm{\partial }}^j{\mathbf{\text{h}}}_l^{\epsilon }}{\mathrm{\partial }{t}^j}}\in L^2(0,T;H^{1/2}({\mathrm{\Sigma }}_{\epsilon }^l\left)\right)$ for j=0,1 and let ${\mathbf{\text{h}}}_{\epsilon }\in L^2(0,T;W^{1,2}({\mathrm{\Omega }}_{\epsilon }^{\alpha }\left)\right)$ with ${\displaystyle \frac{\mathrm{\partial }{\mathbf{\text{h}}}_{\epsilon }}{\mathrm{\partial }t}}\in L^2(0,T;W^{1,2}({\mathrm{\Omega }}_{\epsilon }^{\alpha }\left)\right)$ be the solution of the following problem (see Lemma 5.2): $\begin{array}{rl}& \text{div}{\mathbf{\text{h}}}_{\epsilon }=0\text{in}{\mathrm{\Omega }}_{\epsilon }^{\alpha },\\ & {\mathbf{\text{h}}}_{\epsilon }=0\text{on}{\mathrm{\Gamma }}_{\epsilon }^{\alpha },\\ & {\mathbf{\text{h}}}_{\epsilon }={\epsilon }^2{\mathbf{\text{h}}}_i^{\epsilon }\text{on}{\mathrm{\Sigma }}_{\epsilon }^i,i=0,l.\end{array}$ A weak solution of our problem (5(5) $\begin{array}{rl}& \frac{\mathrm{\partial }{\mathbf{\text{u}}}_{\epsilon }}{\mathrm{\partial }t}-\mu \mathrm{\Delta }{\mathbf{\text{u}}}_{\epsilon }+\mathrm{\nabla }{p}_{\epsilon }=a\text{rot}{\mathbf{\text{w}}}_{\epsilon }+\mathbf{\text{F}},\\ & \text{div}{\mathbf{\text{u}}}_{\epsilon }=0,\text{in}{\mathrm{\Omega }}_{\epsilon }^{\alpha },\\ & \frac{\mathrm{\partial }{\mathbf{\text{w}}}_{\epsilon }}{\mathrm{\partial }t}-\delta \mathrm{\Delta }{\mathbf{\text{w}}}_{\epsilon }-\beta \text{div}{\mathbf{\text{w}}}_{\epsilon }+2a{\mathbf{\text{w}}}_{\epsilon }=a\text{rot}{\mathbf{\text{u}}}_{\epsilon }+\mathbf{\text{G}}.\end{array}$(5) )–(11(11) ${\mathbf{\text{h}}}_0^{\epsilon }(t,\cdot ),{\mathbf{\text{h}}}_l^{\epsilon }(t,\cdot ),\mathbf{\text{F}}(t,\cdot ),\mathbf{\text{G}}(t,\cdot ),\mathrm{\forall }t\in [0,T^{\ast }],T^{\ast }>0.$(11) ) is a pair $({\mathbf{\text{u}}}_{\epsilon },{\mathbf{\text{w}}}_{\epsilon })=({\mathbf{\text{v}}}_{\epsilon }+{\mathbf{\text{h}}}_{\epsilon },{\mathbf{\text{w}}}_{\epsilon })$ such that $\text{div}{\mathbf{\text{v}}}_{\epsilon }=0,{\mathbf{\text{v}}}_{\epsilon }(0,\stackrel{\mathbf{~}}{\mathit{x}})=0,{\mathbf{\text{w}}}_{\epsilon }(0,\stackrel{\mathbf{~}}{\mathit{x}})=0,{\mathbf{\text{v}}}_{\epsilon },{\mathbf{\text{w}}}_{\epsilon }\in L^2(0,T;{\stackrel{\circ }{W}}^{1,2}({\mathrm{\Omega }}_{\epsilon }^{\alpha }\left)\right)\cap L^{\mathrm{\infty }}(0,T;L^2({\mathrm{\Omega }}_{\epsilon }^{\alpha }\left)\right),{\mathbf{\text{v}}}_t,{\mathbf{\text{w}}}_t\in L^2(0,T;L^2({\mathrm{\Omega }}_{\epsilon }^{\alpha }\left)\right)$, and $({\mathbf{\text{v}}}_{\epsilon },{\mathbf{\text{w}}}_{\epsilon })$ satisfy the integral identities (12) $\begin{array}{rl}& {\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}\frac{\mathrm{\partial }{\mathbf{\text{v}}}_{\epsilon }}{\mathrm{\partial }t}\mathit{\varphi }+\mu {\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}\mathrm{\nabla }{\mathbf{\text{v}}}_{\epsilon }\mathrm{\nabla }\mathit{\varphi }=a{\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}\text{rot}{\mathbf{\text{w}}}_{\epsilon }\mathit{\varphi }+{\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}\mathbf{\text{F}}\mathit{\varphi }\\ & -{\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}\frac{\mathrm{\partial }{\mathbf{\text{h}}}_{\epsilon }}{\mathrm{\partial }t}\mathit{\varphi }-{\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}\mathrm{\nabla }{\mathbf{\text{h}}}_{\epsilon }\mathrm{\nabla }\mathit{\varphi },\mathrm{\forall }\mathit{\varphi }\in {\stackrel{\circ }{W}}^{1,2}\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right),\text{div}\mathit{\varphi }=0,\\ & {\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}\frac{\mathrm{\partial }{\mathbf{\text{w}}}_{\epsilon }}{\mathrm{\partial }t}\mathit{\psi }+\delta {\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}\mathrm{\nabla }{\mathbf{\text{w}}}_{\epsilon }\mathrm{\nabla }\mathit{\psi }+\beta {\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}\text{div}{\mathbf{\text{w}}}_{\epsilon }\text{div}\mathit{\psi }+2a{\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}{\mathbf{\text{w}}}_{\epsilon }\mathit{\psi }=a{\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}\text{rot}({\mathbf{\text{v}}}_{\epsilon }+{\mathbf{\text{h}}}_{\epsilon })\mathit{\psi }\\ & +{\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}\mathbf{\text{G}}\mathit{\psi },\mathrm{\forall }\mathit{\psi }\in {\stackrel{\circ }{W}}^{1,2}\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right).\end{array}$(12) Let $\mathbf{\text{F}}$, $\mathbf{\text{G}}\in L^2(0,T;L^2({\mathrm{\Omega }}_{\epsilon }^{\alpha }\left)\right)$. There then exists a unique weak solution $({\mathbf{\text{v}}}_{\epsilon },{\mathbf{\text{w}}}_{\epsilon })$ satisfying (12) for a.e. $t\in [0,T]$. The proof follows the same arguments as the proof of Theorem 2.1.1 from [2, Chapter III, Section 2] which can be straighforwardly adapted to our setting.

3. Rewriting the problem

In this section, we will provide the geometric tools necessary to write the problem (5(5) $\begin{array}{rl}& \frac{\mathrm{\partial }{\mathbf{\text{u}}}_{\epsilon }}{\mathrm{\partial }t}-\mu \mathrm{\Delta }{\mathbf{\text{u}}}_{\epsilon }+\mathrm{\nabla }{p}_{\epsilon }=a\text{rot}{\mathbf{\text{w}}}_{\epsilon }+\mathbf{\text{F}},\\ & \text{div}{\mathbf{\text{u}}}_{\epsilon }=0,\text{in}{\mathrm{\Omega }}_{\epsilon }^{\alpha },\\ & \frac{\mathrm{\partial }{\mathbf{\text{w}}}_{\epsilon }}{\mathrm{\partial }t}-\delta \mathrm{\Delta }{\mathbf{\text{w}}}_{\epsilon }-\beta \text{div}{\mathbf{\text{w}}}_{\epsilon }+2a{\mathbf{\text{w}}}_{\epsilon }=a\text{rot}{\mathbf{\text{u}}}_{\epsilon }+\mathbf{\text{G}}.\end{array}$(5) )–(11(11) ${\mathbf{\text{h}}}_0^{\epsilon }(t,\cdot ),{\mathbf{\text{h}}}_l^{\epsilon }(t,\cdot ),\mathbf{\text{F}}(t,\cdot ),\mathbf{\text{G}}(t,\cdot ),\mathrm{\forall }t\in [0,T^{\ast }],T^{\ast }>0.$(11) ) on the reference domain ${\mathrm{\Omega }}_{\epsilon }$, allowing us to perform the asymptotic analysis.

3.1. Covariant and contravariant basis

Let us consider the following mapping: $\begin{array}{rl}& {\tilde{\mathrm{\Phi }}}_{\epsilon }^{\alpha }:[0,T]\times {\mathrm{\Omega }}_{\epsilon }\to [0,T]\times {\mathrm{\Omega }}_{\epsilon }^{\alpha },\\ & {\tilde{\mathrm{\Phi }}}_{\epsilon }^{\alpha }(t,\mathit{x})=(\tilde{t},\tilde{\mathit{x}})=(\tilde{t},{\mathrm{\Phi }}_{\epsilon }^{\alpha }(\mathit{x}\left)\right),\end{array}$ We introduce the covariant basis as the gradient of the mapping ${\mathrm{\Phi }}_{\epsilon }^{\alpha }$ consisting of vectors ${\mathbf{\text{a}}}_i\left(\mathit{x}\right)=\frac{\mathrm{\partial }{\mathrm{\Phi }}_{\epsilon }^{\alpha }\left(\mathit{x}\right)}{\mathrm{\partial }{x}_i},i=1,2,3.$ Using (2(2) ${\mathrm{\Phi }}_{\epsilon }^{\alpha }\left(\mathit{x}\right)=\mathit{\pi }\left(x_1\right)+x_2{\mathbf{\text{n}}}_{\alpha }\left(x_1\right)+x_3{\mathbf{\text{b}}}_{\alpha }\left(x_1\right),$(2) )–(3(3) $\begin{array}{rl}& {\mathbf{\text{n}}}_{\alpha }\left(x_1\right)=\cos \alpha \left(x_1\right)\mathbf{\text{n}}\left(x_1\right)+\sin \alpha \left(x_1\right)\mathbf{\text{b}}\left(x_1\right),\\ & {\mathbf{\text{b}}}_{\alpha }\left(x_1\right)=-\sin \alpha \left(x_1\right)\mathbf{\text{n}}\left(x_1\right)+\cos \alpha \left(x_1\right)\mathbf{\text{b}}\left(x_1\right),\end{array}$(3) ) and Frenet's relations (1(1) ${\mathbf{\text{t}}}^{\prime }=\kappa \mathbf{\text{n}},{\mathbf{\text{n}}}^{\prime }=-\kappa \mathbf{\text{t}}+\tau \mathbf{\text{b}},{\mathbf{\text{b}}}^{\prime }=-\tau \mathbf{\text{n}}.$(1) ), one can easily obtain $\begin{array}{rl}& {\mathbf{\text{a}}}_1=(1-\kappa ({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{x}}_{\ast }\left)\right)\mathbf{\text{t}}-({\alpha }^{\prime }+\tau )({\mathbf{\text{e}}}_{\alpha }^{\perp }\cdot {\mathit{x}}_{\ast })\mathbf{\text{n}}+({\alpha }^{\prime }+\tau )({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{x}}_{\ast })\mathbf{\text{b}},\\ & {\mathbf{\text{a}}}_2=\cos \alpha \mathbf{\text{n}}+\sin \alpha \mathbf{\text{b}},{\mathbf{\text{a}}}_3=-\sin \alpha \mathbf{\text{n}}+\cos \alpha \mathbf{\text{b}},\end{array}$ where (13) ${\mathbf{\text{e}}}_{\alpha }=(\cos \alpha ,-\sin \alpha ),{\mathbf{\text{e}}}_{\alpha }^{\perp }=(\sin \alpha ,\cos \alpha ).$(13) From (4(4) $\alpha \left(x_1\right)=-{\int }_{x_0}^{x_1}\tau \left(\xi \right)\mathrm{d}\xi +{\alpha }_0,$(4) ), it directly follows that ${\alpha }^{\prime }=-\tau$, so we have ${\mathbf{\text{a}}}_1=(1-\kappa ({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{x}}_{\ast }\left)\right)\mathbf{\text{t}}.$ The contravariant basis is the dual basis to the covariant basis defined by the relation ${\mathbf{\text{a}}}^i\cdot {\mathbf{\text{a}}}_j={\delta }_{ij},i,j=1,2,3.$ It can be easily verified that ${\mathbf{\text{a}}}^1=\frac{1}{1-\kappa ({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{x}}_{\ast })}\mathbf{\text{t}},{\mathbf{\text{a}}}^2=\cos \alpha \mathbf{\text{n}}+\sin \alpha \mathbf{\text{b}},{\mathbf{\text{a}}}^3=-\sin \alpha \mathbf{\text{n}}+\cos \alpha \mathbf{\text{b}}.$ More details can be found in [14, Section 3.1].

3.2. Micropolar equations in the reference domain

Let us denote the scalar field $P_{\epsilon }=p_{\epsilon }\circ {\tilde{\mathrm{\Phi }}}_{\epsilon }^{\alpha },$ where ${\tilde{\mathrm{\Phi }}}_{\epsilon }^{\alpha }:[0,T]\times {\mathrm{\Omega }}_{\epsilon }\to [0,T]\times {\mathrm{\Omega }}_{\epsilon }^{\alpha },p_{\epsilon }:[0,T]\times {\mathrm{\Omega }}_{\epsilon }^{\alpha }\to \mathbb{R}$ and $P_{\epsilon }:[0,T]\times {\mathrm{\Omega }}_{\epsilon }\to \mathbb{R}$.

We also introduce the vector fields $\begin{array}{rl}& {\mathbf{\text{U}}}_{\epsilon }={\mathbf{\text{u}}}_{\epsilon }\circ {\tilde{\mathrm{\Phi }}}_{\epsilon }^{\alpha }=V_{\epsilon }^1{\mathbf{\text{a}}}^1+V_{\epsilon }^2{\mathbf{\text{a}}}^2+V_{\epsilon }^3{\mathbf{\text{a}}}^3,\\ & {\mathbf{\text{W}}}_{\epsilon }={\mathbf{\text{w}}}_{\epsilon }\circ {\tilde{\mathrm{\Phi }}}_{\epsilon }^{\alpha }=W_{\epsilon }^1{\mathbf{\text{a}}}^1+W_{\epsilon }^2{\mathbf{\text{a}}}^2+W_{\epsilon }^3{\mathbf{\text{a}}}^3,\end{array}$ where ${\mathbf{\text{u}}}_{\epsilon },{\mathbf{\text{w}}}_{\epsilon }:[0,T]\times {\mathrm{\Omega }}_{\epsilon }^{\alpha }\to {\mathbb{R}}^3$ and ${\mathbf{\text{U}}}_{\epsilon },{\mathbf{\text{W}}}_{\epsilon }:[0,T]\times {\mathrm{\Omega }}_{\epsilon }\to {\mathbb{R}}^3$. By $V_{\epsilon }^i={\mathbf{\text{U}}}_{\epsilon }\cdot {\mathbf{\text{a}}}_i,W_{\epsilon }^i={\mathbf{\text{W}}}_{\epsilon }\cdot {\mathbf{\text{a}}}_i$, we denote the corresponding covariant components.

Using the expressions for the differential operators in curvilinear coordinates derived in [14, Section 3.3], we can write the equations (5(5) $\begin{array}{rl}& \frac{\mathrm{\partial }{\mathbf{\text{u}}}_{\epsilon }}{\mathrm{\partial }t}-\mu \mathrm{\Delta }{\mathbf{\text{u}}}_{\epsilon }+\mathrm{\nabla }{p}_{\epsilon }=a\text{rot}{\mathbf{\text{w}}}_{\epsilon }+\mathbf{\text{F}},\\ & \text{div}{\mathbf{\text{u}}}_{\epsilon }=0,\text{in}{\mathrm{\Omega }}_{\epsilon }^{\alpha },\\ & \frac{\mathrm{\partial }{\mathbf{\text{w}}}_{\epsilon }}{\mathrm{\partial }t}-\delta \mathrm{\Delta }{\mathbf{\text{w}}}_{\epsilon }-\beta \text{div}{\mathbf{\text{w}}}_{\epsilon }+2a{\mathbf{\text{w}}}_{\epsilon }=a\text{rot}{\mathbf{\text{u}}}_{\epsilon }+\mathbf{\text{G}}.\end{array}$(5) ) on the reference domain ${\mathrm{\Omega }}_{\epsilon }$.

The equation (5(5) $\begin{array}{rl}& \frac{\mathrm{\partial }{\mathbf{\text{u}}}_{\epsilon }}{\mathrm{\partial }t}-\mu \mathrm{\Delta }{\mathbf{\text{u}}}_{\epsilon }+\mathrm{\nabla }{p}_{\epsilon }=a\text{rot}{\mathbf{\text{w}}}_{\epsilon }+\mathbf{\text{F}},\\ & \text{div}{\mathbf{\text{u}}}_{\epsilon }=0,\text{in}{\mathrm{\Omega }}_{\epsilon }^{\alpha },\\ & \frac{\mathrm{\partial }{\mathbf{\text{w}}}_{\epsilon }}{\mathrm{\partial }t}-\delta \mathrm{\Delta }{\mathbf{\text{w}}}_{\epsilon }-\beta \text{div}{\mathbf{\text{w}}}_{\epsilon }+2a{\mathbf{\text{w}}}_{\epsilon }=a\text{rot}{\mathbf{\text{u}}}_{\epsilon }+\mathbf{\text{G}}.\end{array}$(5) )₁ expressing the balance of momentum in the reference domain ${\mathrm{\Omega }}_{\epsilon }$ takes the following form: $\begin{array}{rl}& \frac{\mathrm{\partial }{V}_{\epsilon }^1}{\mathrm{\partial }t}(1+\kappa ({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{x}}_{\ast })+\cdots )\mathbf{\text{t}}+\frac{\mathrm{\partial }{V}_{\epsilon }^2}{\mathrm{\partial }t}{\mathbf{\text{a}}}^2+\frac{\mathrm{\partial }{V}_{\epsilon }^3}{\mathrm{\partial }t}{\mathbf{\text{a}}}^3-\mu (\mathrm{\Delta }{V}_{\epsilon }^1+\kappa \cos \alpha (\frac{\mathrm{\partial }{V}_{\epsilon }^1}{\mathrm{\partial }{x}_2}-\frac{\mathrm{\partial }{V}_{\epsilon }^2}{\mathrm{\partial }{x}_1})\\ & +\kappa \sin \alpha (\frac{\mathrm{\partial }{V}_{\epsilon }^3}{\mathrm{\partial }{x}_1}-\frac{\mathrm{\partial }{V}_{\epsilon }^1}{\mathrm{\partial }{x}_3}))\mathbf{\text{t}}\\ & +\mu (\frac{\mathrm{\partial }}{\mathrm{\partial }{x}_1}(V_{\epsilon }^2\kappa \cos \alpha -V_{\epsilon }^3\kappa \sin \alpha -{\kappa }^{\prime }(V_{\epsilon }^2\cos \alpha +V_{\epsilon }^3\sin \alpha ))+{\kappa }^2{V}_{\epsilon }^1-\kappa ({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{x}}_{\ast })\\ & (\mathrm{\Delta }{V}_{\epsilon }^1-\kappa \cos \alpha \frac{\mathrm{\partial }{V}_{\epsilon }^1}{\mathrm{\partial }{x}_2}))\mathbf{\text{t}}\\ & -\mu ({\kappa }^2({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{x}}_{\ast })\sin \alpha \frac{\mathrm{\partial }{V}_{\epsilon }^1}{\mathrm{\partial }{x}_3}+{\kappa }^2({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{x}}_{\ast }{)}^2\mathrm{\Delta }{V}_{\epsilon }^1)\mathbf{\text{t}}-\mu (\mathrm{\Delta }{V}_{\epsilon }^2-\kappa \cos \alpha \frac{\mathrm{\partial }{V}_{\epsilon }^2}{\mathrm{\partial }{x}_2}+\kappa \sin \alpha \frac{\mathrm{\partial }{V}_{\epsilon }^2}{\mathrm{\partial }{x}_3}){\mathbf{\text{a}}}^2\\ & -\mu (2\kappa ({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{x}}_{\ast })(-\kappa \cos \alpha \frac{\mathrm{\partial }{V}_{\epsilon }^2}{\mathrm{\partial }{x}_2}+\kappa \sin \alpha \frac{\mathrm{\partial }{V}_{\epsilon }^2}{\mathrm{\partial }{x}_3})\\ & +(2\kappa \frac{\mathrm{\partial }{V}_{\epsilon }^1}{\mathrm{\partial }{x}_1}+{\kappa }^{\prime }{V}_{\epsilon }^1-{\kappa }^2{V}_{\epsilon }^2)\cos \alpha +\kappa \tau V_{\epsilon }^1\sin \alpha ){\mathbf{\text{a}}}^2\\ & -\mu ({\kappa }^2\cos \alpha ({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{x}}_{\ast })\frac{\mathrm{\partial }{V}_{\epsilon }^2}{\mathrm{\partial }{x}_2}-{\kappa }^2\sin \alpha ({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{x}}_{\ast })\frac{\mathrm{\partial }{V}_{\epsilon }^2}{\mathrm{\partial }{x}_3}){\mathbf{\text{a}}}^2\\ & -\mu (\mathrm{\Delta }{V}_{\epsilon }^3+\kappa \sin \alpha \frac{\mathrm{\partial }{V}_{\epsilon }^3}{\mathrm{\partial }{x}_3}-\kappa \cos \alpha \frac{\mathrm{\partial }{V}_{\epsilon }^3}{\mathrm{\partial }{x}_2}){\mathbf{\text{a}}}^3\\ & -\mu (2\kappa ({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{x}}_{\ast })(\kappa \sin \alpha \frac{\mathrm{\partial }{V}_{\epsilon }^3}{\mathrm{\partial }{x}_3}-\kappa \cos \alpha \frac{\mathrm{\partial }{V}_{\epsilon }^3}{\mathrm{\partial }{x}_2})+\kappa \tau V_{\epsilon }^1\cos \alpha \\ & -(2\kappa \frac{\mathrm{\partial }{V}_{\epsilon }^1}{\mathrm{\partial }{x}_1}+{\kappa }^{\prime }{V}_{\epsilon }^1-{\kappa }^2{V}_{\epsilon }^2)\sin \alpha ){\mathbf{\text{a}}}^3\end{array}$ (14) $\begin{array}{rl}& -\mu ({\kappa }^2\cos \alpha ({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{x}}_{\ast })\frac{\mathrm{\partial }{V}_{\epsilon }^3}{\mathrm{\partial }{x}_2}-{\kappa }^2\sin \alpha ({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{x}}_{\ast })\frac{\mathrm{\partial }{V}_{\epsilon }^3}{\mathrm{\partial }{x}_3}){\mathbf{\text{a}}}^3\\ & +\frac{\mathrm{\partial }{P}_{\epsilon }}{\mathrm{\partial }{x}_1}(1+\kappa ({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{x}}_{\ast })+\cdots )\mathbf{\text{t}}+\frac{\mathrm{\partial }{P}_{\epsilon }}{\mathrm{\partial }{x}_2}{\mathbf{\text{a}}}^2+\frac{\mathrm{\partial }{P}_{\epsilon }}{\mathrm{\partial }{x}_3}{\mathbf{\text{a}}}^3\\ & =a(\frac{\mathrm{\partial }{W}_{\epsilon }^3}{\mathrm{\partial }{x}_2}-\frac{\mathrm{\partial }{W}_{\epsilon }^2}{\mathrm{\partial }{x}_3})\mathbf{\text{t}}+a(1+\kappa ({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{x}}_{\ast })+\cdots )(\frac{\mathrm{\partial }{W}_{\epsilon }^1}{\mathrm{\partial }{x}_3}-\frac{\mathrm{\partial }{W}_{\epsilon }^3}{\mathrm{\partial }{x}_1}){\mathbf{\text{a}}}^2+a(1+\kappa ({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{x}}_{\ast })+\cdots )\\ & (\frac{\mathrm{\partial }{W}_{\epsilon }^2}{\mathrm{\partial }{x}_1}-\frac{\mathrm{\partial }{W}_{\epsilon }^1}{\mathrm{\partial }{x}_2}){\mathbf{\text{a}}}^3\\ & +f_1\mathbf{\text{t}}+(f_2\cos \alpha +f_3\sin \alpha ){\mathbf{\text{a}}}^2+(f_3\cos \alpha -f_2\sin \alpha ){\mathbf{\text{a}}}^3.\end{array}$(14) The equation (5(5) $\begin{array}{rl}& \frac{\mathrm{\partial }{\mathbf{\text{u}}}_{\epsilon }}{\mathrm{\partial }t}-\mu \mathrm{\Delta }{\mathbf{\text{u}}}_{\epsilon }+\mathrm{\nabla }{p}_{\epsilon }=a\text{rot}{\mathbf{\text{w}}}_{\epsilon }+\mathbf{\text{F}},\\ & \text{div}{\mathbf{\text{u}}}_{\epsilon }=0,\text{in}{\mathrm{\Omega }}_{\epsilon }^{\alpha },\\ & \frac{\mathrm{\partial }{\mathbf{\text{w}}}_{\epsilon }}{\mathrm{\partial }t}-\delta \mathrm{\Delta }{\mathbf{\text{w}}}_{\epsilon }-\beta \text{div}{\mathbf{\text{w}}}_{\epsilon }+2a{\mathbf{\text{w}}}_{\epsilon }=a\text{rot}{\mathbf{\text{u}}}_{\epsilon }+\mathbf{\text{G}}.\end{array}$(5) )₂ expressing the balance of mass can be written in the reference domain ${\mathrm{\Omega }}_{\epsilon }$ as: (15) $\begin{array}{rl}& [\frac{1}{1-\kappa ({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{x}}_{\ast })}{]}^2(\frac{\mathrm{\partial }{V}_{\epsilon }^1}{\mathrm{\partial }{x}_1}-\kappa (V_{\epsilon }^2\cos \alpha -V_{\epsilon }^3\sin \alpha )+\left({\kappa }^{\prime }\right({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{x}}_{\ast })+\kappa \tau ({\mathbf{\text{e}}}_{\alpha }^{\perp }\cdot {\mathit{x}}_{\ast }\left)\right)V_{\epsilon }^1\\ & +\kappa ({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{x}}_{\ast })\left({\kappa }^{\prime }\right({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{x}}_{\ast })+\kappa \tau ({\mathbf{\text{e}}}_{\alpha }^{\perp }\cdot {\mathit{x}}_{\ast }\left)\right)V_{\epsilon }^1+{\kappa }^2({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{x}}_{\ast })\cos \alpha V_{\epsilon }^2-{\kappa }^2({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{x}}_{\ast })\sin \alpha V_{\epsilon }^3)\\ & +\frac{\mathrm{\partial }{V}_{\epsilon }^2}{\mathrm{\partial }{x}_2}+\frac{\mathrm{\partial }{V}_{\epsilon }^3}{\mathrm{\partial }{x}_3}=\frac{\mathrm{\partial }{V}_{\epsilon }^1}{\mathrm{\partial }{x}_1}-\kappa (V_{\epsilon }^2\cos \alpha -V_{\epsilon }^3\sin \alpha )+\left({\kappa }^{\prime }\right({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{x}}_{\ast })+\kappa \tau ({\mathbf{\text{e}}}_{\alpha }^{\perp }\cdot {\mathit{x}}_{\ast }\left)\right)V_{\epsilon }^1\\ & +\kappa ({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{x}}_{\ast })\left({\kappa }^{\prime }\right({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{x}}_{\ast })+\kappa \tau ({\mathbf{\text{e}}}_{\alpha }^{\perp }\cdot {\mathit{x}}_{\ast }\left)\right)V_{\epsilon }^1+{\kappa }^2({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{x}}_{\ast })\cos \alpha V_{\epsilon }^2-{\kappa }^2({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{x}}_{\ast })\sin \alpha V_{\epsilon }^3\\ & +\frac{\mathrm{\partial }{V}_{\epsilon }^2}{\mathrm{\partial }{x}_2}+\frac{\mathrm{\partial }{V}_{\epsilon }^3}{\mathrm{\partial }{x}_3}+2\kappa ({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{x}}_{\ast })(\frac{\mathrm{\partial }{V}_{\epsilon }^1}{\mathrm{\partial }{x}_1}-\kappa (V_{\epsilon }^2\cos \alpha -V_{\epsilon }^3\sin \alpha )+\left({\kappa }^{\prime }\right({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{x}}_{\ast })+\kappa \tau ({\mathbf{\text{e}}}_{\alpha }^{\perp }\cdot {\mathit{x}}_{\ast }\left)\right)V_{\epsilon }^1\\ & +{\kappa }^2({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{x}}_{\ast })\cos \alpha V_{\epsilon }^2-{\kappa }^2({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{x}}_{\ast })\sin \alpha V_{\epsilon }^3)+3{\kappa }^2({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{x}}_{\ast }{)}^2(\frac{\mathrm{\partial }{V}_{\epsilon }^1}{\mathrm{\partial }{x}_1}-\kappa (V_{\epsilon }^2\cos \alpha -V_{\epsilon }^3\sin \alpha ))\\ & +4{\kappa }^3({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{x}}_{\ast }{)}^3\frac{\mathrm{\partial }{V}_{\epsilon }^1}{\mathrm{\partial }{x}_1}+\mathcal{O}({\epsilon }^3)=0.\end{array}$(15) Finally, the equation (5(5) $\begin{array}{rl}& \frac{\mathrm{\partial }{\mathbf{\text{u}}}_{\epsilon }}{\mathrm{\partial }t}-\mu \mathrm{\Delta }{\mathbf{\text{u}}}_{\epsilon }+\mathrm{\nabla }{p}_{\epsilon }=a\text{rot}{\mathbf{\text{w}}}_{\epsilon }+\mathbf{\text{F}},\\ & \text{div}{\mathbf{\text{u}}}_{\epsilon }=0,\text{in}{\mathrm{\Omega }}_{\epsilon }^{\alpha },\\ & \frac{\mathrm{\partial }{\mathbf{\text{w}}}_{\epsilon }}{\mathrm{\partial }t}-\delta \mathrm{\Delta }{\mathbf{\text{w}}}_{\epsilon }-\beta \text{div}{\mathbf{\text{w}}}_{\epsilon }+2a{\mathbf{\text{w}}}_{\epsilon }=a\text{rot}{\mathbf{\text{u}}}_{\epsilon }+\mathbf{\text{G}}.\end{array}$(5) )₃ describing the balance of angular momentum in the reference domain ${\mathrm{\Omega }}_{\epsilon }$ reads: $\begin{array}{rl}& \frac{\mathrm{\partial }{W}_{\epsilon }^1}{\mathrm{\partial }t}(1+\kappa ({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{x}}_{\ast })+\cdots )\mathbf{\text{t}}+\frac{\mathrm{\partial }{W}_{\epsilon }^2}{\mathrm{\partial }t}{\mathbf{\text{a}}}^2+\frac{\mathrm{\partial }{W}_{\epsilon }^3}{\mathrm{\partial }t}{\mathbf{\text{a}}}^3\\ & -\delta (\mathrm{\Delta }{W}_{\epsilon }^1+\kappa \cos \alpha (\frac{\mathrm{\partial }{W}_{\epsilon }^1}{\mathrm{\partial }{x}_2}-\frac{\mathrm{\partial }{W}_{\epsilon }^2}{\mathrm{\partial }{x}_1})+\kappa \sin \alpha (\frac{\mathrm{\partial }{W}_{\epsilon }^3}{\mathrm{\partial }{x}_1}-\frac{\mathrm{\partial }{W}_{\epsilon }^1}{\mathrm{\partial }{x}_3}))\mathbf{\text{t}}\\ & +\delta (\frac{\mathrm{\partial }}{\mathrm{\partial }{x}_1}(W_{\epsilon }^2\kappa \cos \alpha -W_{\epsilon }^3\kappa \sin \alpha -{\kappa }^{\prime }(W_{\epsilon }^2\cos \alpha -W_{\epsilon }^3\sin \alpha \left)\right)\\ & +{\kappa }^2{W}_{\epsilon }^1-\kappa ({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{x}}_{\ast })(\mathrm{\Delta }{W}_{\epsilon }^1-\kappa \cos \alpha \frac{\mathrm{\partial }{W}_{\epsilon }^1}{\mathrm{\partial }{x}_2}))\mathbf{\text{t}}\\ & -\delta ({\kappa }^2({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{x}}_{\ast })\sin \alpha \frac{\mathrm{\partial }{W}_{\epsilon }^1}{\mathrm{\partial }{x}_3}+{\kappa }^2({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{x}}_{\ast }{)}^2\mathrm{\Delta }{W}_{\epsilon }^1)\mathbf{\text{t}}\\ & -\delta (\mathrm{\Delta }{W}_{\epsilon }^2-\kappa \cos \alpha \frac{\mathrm{\partial }{W}_{\epsilon }^2}{\mathrm{\partial }{x}_2}+\kappa \sin \alpha \frac{\mathrm{\partial }{W}_{\epsilon }^2}{\mathrm{\partial }{x}_3}){\mathbf{\text{a}}}^2\\ & -\delta (2\kappa ({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{x}}_{\ast })(-\kappa \cos \alpha \frac{\mathrm{\partial }{W}_{\epsilon }^2}{\mathrm{\partial }{x}_2}+\kappa \sin \alpha \frac{\mathrm{\partial }{W}_{\epsilon }^2}{\mathrm{\partial }{x}_3})\\ & +(2\kappa \frac{\mathrm{\partial }{W}_{\epsilon }^1}{\mathrm{\partial }{x}_1}+{\kappa }^{\prime }{W}_{\epsilon }^1-{\kappa }^2{W}_{\epsilon }^2)\cos \alpha +\kappa \tau W_{\epsilon }^1\sin \alpha )){\mathbf{\text{a}}}^2\\ & -\delta ({\kappa }^2\cos \alpha ({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{x}}_{\ast })\frac{\mathrm{\partial }{W}_{\epsilon }^2}{\mathrm{\partial }{x}_2}-{\kappa }^2\sin \alpha ({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{x}}_{\ast })\frac{\mathrm{\partial }{W}_{\epsilon }^2}{\mathrm{\partial }{x}_3}){\mathbf{\text{a}}}^2\end{array}$ $\begin{array}{rl}& -\delta (\mathrm{\Delta }{W}_{\epsilon }^3+\kappa \sin \alpha \frac{\mathrm{\partial }{W}_{\epsilon }^3}{\mathrm{\partial }{x}_3}-\kappa \cos \alpha \frac{\mathrm{\partial }{W}_{\epsilon }^3}{\mathrm{\partial }{x}_2}){\mathbf{\text{a}}}^3\\ & -\delta (2\kappa ({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{x}}_{\ast })(\kappa \sin \alpha \frac{\mathrm{\partial }{W}_{\epsilon }^3}{\mathrm{\partial }{x}_3}-\kappa \cos \alpha \frac{\mathrm{\partial }{W}_{\epsilon }^3}{\mathrm{\partial }{x}_2})+\kappa \tau W_{\epsilon }^1\cos \alpha \\ & -(2\kappa \frac{\mathrm{\partial }{W}_{\epsilon }^1}{\mathrm{\partial }{x}_1}+{\kappa }^{\prime }{W}_{\epsilon }^1-{\kappa }^2{W}_{\epsilon }^2)\sin \alpha ){\mathbf{\text{a}}}^3\\ & -\delta ({\kappa }^2\cos \alpha ({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{x}}_{\ast })\frac{\mathrm{\partial }{W}_{\epsilon }^3}{\mathrm{\partial }{x}_2}-{\kappa }^2\sin \alpha ({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{x}}_{\ast })\frac{\mathrm{\partial }{W}_{\epsilon }^3}{\mathrm{\partial }{x}_3}){\mathbf{\text{a}}}^3\\ & -\beta ((1+\kappa ({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{x}}_{\ast }\left)\right)(\frac{{\mathrm{\partial }}^2{W}_{\epsilon }^1}{\mathrm{\partial }{x}_1^2}-(\kappa \cos \alpha {)}^{\prime }{W}_{\epsilon }^2)\mathbf{\text{t}}\\ & -\beta (1+\kappa ({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{x}}_{\ast }\left)\right)(-\kappa \cos \alpha \frac{\mathrm{\partial }{W}_{\epsilon }^2}{\mathrm{\partial }{x}_1}\\ & +(\kappa \sin \alpha {)}^{\prime }{W}_{\epsilon }^3+\kappa \sin \alpha \frac{\mathrm{\partial }{W}_{\epsilon }^3}{\mathrm{\partial }{x}_1}+\frac{{\mathrm{\partial }}^2{W}_{\epsilon }^2}{\mathrm{\partial }{x}_1\mathrm{\partial }{x}_2}+\frac{{\mathrm{\partial }}^2{W}_{\epsilon }^3}{\mathrm{\partial }{x}_1\mathrm{\partial }{x}_3})\mathbf{\text{t}}\\ & -\beta (\frac{{\mathrm{\partial }}^2{W}_{\epsilon }^1}{\mathrm{\partial }{x}_1\mathrm{\partial }{x}_2}-\kappa (\frac{\mathrm{\partial }{W}_{\epsilon }^2}{\mathrm{\partial }{x}_2}\cos \alpha -\frac{\mathrm{\partial }{W}_{\epsilon }^3}{\mathrm{\partial }{x}_2}\sin \alpha )+({\kappa }^{\prime }{W}_{\epsilon }^1+{\kappa }^2\cos \alpha W_{\epsilon }^2-{\kappa }^2\sin \alpha W_{\epsilon }^3)\cos \alpha \\ & +2\kappa \cos \alpha \frac{\mathrm{\partial }{W}_{\epsilon }^1}{\mathrm{\partial }{x}_1}){\mathbf{\text{a}}}^2\\ & -\beta (-2{\kappa }^2(W_{\epsilon }^2\cos \alpha -W_{\epsilon }^3\sin \alpha )\cos \alpha \\ & +\kappa \tau W_{\epsilon }^1\sin \alpha +\left({\kappa }^{\prime }\right({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{x}}_{\ast })+\kappa \tau ({\mathbf{\text{e}}}_{\alpha }^{\perp }\cdot {\mathit{x}}_{\ast }\left)\right)\frac{\mathrm{\partial }{W}_{\epsilon }^1}{\mathrm{\partial }{x}_2}+{\kappa }^2({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{x}}_{\ast })\frac{\mathrm{\partial }{W}_{\epsilon }^2}{\mathrm{\partial }{x}_2}\cos \alpha ){\mathbf{\text{a}}}^2\\ & -\beta (-{\kappa }^2({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{x}}_{\ast })\frac{\mathrm{\partial }{W}_{\epsilon }^3}{\mathrm{\partial }{x}_2}\sin \alpha +\frac{{\mathrm{\partial }}^2{W}_{\epsilon }^2}{\mathrm{\partial }{x}_2^2}+\frac{{\mathrm{\partial }}^2{W}_{\epsilon }^3}{\mathrm{\partial }{x}_2\mathrm{\partial }{x}_3}+2\kappa ({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{x}}_{\ast })\\ & (\frac{{\mathrm{\partial }}^2{W}_{\epsilon }^1}{\mathrm{\partial }{x}_1\mathrm{\partial }{x}_2}-\kappa (\frac{\mathrm{\partial }{W}_{\epsilon }^2}{\mathrm{\partial }{x}_2}\cos \alpha -\frac{\mathrm{\partial }{W}_{\epsilon }^3}{\mathrm{\partial }{x}_2}\sin \alpha ))){\mathbf{\text{a}}}^2\end{array}$ (16) $\begin{array}{rl}& -\beta (\frac{{\mathrm{\partial }}^2{W}_{\epsilon }^1}{\mathrm{\partial }{x}_1\mathrm{\partial }{x}_3}-\kappa (\frac{\mathrm{\partial }{W}_{\epsilon }^2}{\mathrm{\partial }{x}_3}\cos \alpha -\frac{\mathrm{\partial }{W}_{\epsilon }^3}{\mathrm{\partial }{x}_3}\sin \alpha )+\kappa \tau W_{\epsilon }^1\cos \alpha \\ & -({\kappa }^{\prime }{W}_{\epsilon }^1+{\kappa }^2\cos \alpha W_{\epsilon }^2-{\kappa }^2\sin \alpha W_{\epsilon }^3)\sin \alpha ){\mathbf{\text{a}}}^3\\ & -\beta (2\kappa (\frac{\mathrm{\partial }{W}_{\epsilon }^1}{\mathrm{\partial }{x}_1}-\kappa (W_{\epsilon }^2\cos \alpha -W_{\epsilon }^3\sin \alpha ))\sin \alpha \\ & +{\kappa }^{\prime }({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{x}}_{\ast })\frac{\mathrm{\partial }{W}_{\epsilon }^1}{\mathrm{\partial }{x}_3}+\kappa \tau ({\mathbf{\text{e}}}_{\alpha }^{\perp }\cdot {\mathit{x}}_{\ast })\frac{\mathrm{\partial }{W}_{\epsilon }^1}{\mathrm{\partial }{x}_3}+{\kappa }^2({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{x}}_{\ast })\cos \alpha \frac{\mathrm{\partial }{W}_{\epsilon }^2}{\mathrm{\partial }{x}_3}){\mathbf{\text{a}}}^3\\ & -\beta (-{\kappa }^2({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{x}}_{\ast })\frac{\mathrm{\partial }{W}_{\epsilon }^3}{\mathrm{\partial }{x}_3}\sin \alpha +\frac{{\mathrm{\partial }}^2{W}_{\epsilon }^2}{\mathrm{\partial }{x}_2\mathrm{\partial }{x}_3}+\frac{{\mathrm{\partial }}^2{W}_{\epsilon }^3}{\mathrm{\partial }{x}_3^2}+2\kappa ({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{x}}_{\ast })\\ & (\frac{{\mathrm{\partial }}^2{W}_{\epsilon }^1}{\mathrm{\partial }{x}_1\mathrm{\partial }{x}_3}-\kappa (\frac{\mathrm{\partial }{W}_{\epsilon }^2}{\mathrm{\partial }{x}_3}\cos \alpha -\frac{\mathrm{\partial }{W}_{\epsilon }^3}{\mathrm{\partial }{x}_3}\sin \alpha ))]{\mathbf{\text{a}}}^3\\ & +2a(1+\kappa ({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{x}}_{\ast })+\cdots )W_{\epsilon }^1\mathbf{\text{t}}+2a{W}_{\epsilon }^2{\mathbf{\text{a}}}^2+2a{W}_{\epsilon }^3{\mathbf{\text{a}}}^3\\ & =a(\frac{\mathrm{\partial }{V}_{\epsilon }^3}{\mathrm{\partial }{x}_2}-\frac{\mathrm{\partial }{V}_{\epsilon }^2}{\mathrm{\partial }{x}_3})\mathbf{\text{t}}+a((1+\kappa ({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{x}}_{\ast })+\cdots )(\frac{\mathrm{\partial }{V}_{\epsilon }^1}{\mathrm{\partial }{x}_3}-\frac{\mathrm{\partial }{V}_{\epsilon }^3}{\mathrm{\partial }{x}_1})){\mathbf{\text{a}}}^2\\ & +a((1+\kappa ({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{x}}_{\ast })+\cdots )(\frac{\mathrm{\partial }{V}_{\epsilon }^2}{\mathrm{\partial }{x}_1}-\frac{\mathrm{\partial }{V}_{\epsilon }^1}{\mathrm{\partial }{x}_2})){\mathbf{\text{a}}}^3+g_1\mathbf{\text{t}}+(g_2\cos \alpha +g_3\sin \alpha ){\mathbf{\text{a}}}^2\\ & +(g_3\cos \alpha -g_2\sin \alpha ){\mathbf{\text{a}}}^3.\end{array}$(16) Note that $\mathbf{\text{f}}=f_1\mathbf{\text{t}}+f_2\mathbf{\text{n}}+f_3\mathbf{\text{b}},\mathbf{\text{g}}=g_1\mathbf{\text{t}}+g_2\mathbf{\text{n}}+g_3\mathbf{\text{b}}.$

4. Asymptotic expansion

4.1. Regular part

In this section, we construct the regular part of the asymptotic approximation of the solution $({\mathbf{\text{u}}}_{\epsilon },{\mathbf{\text{w}}}_{\epsilon },p_{\epsilon })$. In view of that, let us introduce $\begin{array}{rl}& {\mathbf{\text{U}}}_{\epsilon }={\mathbf{\text{u}}}_{\epsilon }\circ {\tilde{\mathrm{\Phi }}}_{\epsilon }^{\alpha }=V_{\epsilon }^1{\mathbf{\text{a}}}^1+V_{\epsilon }^2{\mathbf{\text{a}}}^2+V_{\epsilon }^3{\mathbf{\text{a}}}^3,\\ & {\mathbf{\text{W}}}_{\epsilon }={\mathbf{\text{w}}}_{\epsilon }\circ {\tilde{\mathrm{\Phi }}}_{\epsilon }^{\alpha }=W_{\epsilon }^1{\mathbf{\text{a}}}^1+W_{\epsilon }^2{\mathbf{\text{a}}}^2+W_{\epsilon }^3{\mathbf{\text{a}}}^3,\\ & P_{\epsilon }=p_{\epsilon }\circ {\tilde{\mathrm{\Phi }}}_{\epsilon }^{\alpha }\end{array}$ and formally expand (for i=1,2,3) (17) $\begin{array}{rl}& V_{\epsilon }^i(t,\mathit{x})={\epsilon }^2{V}_0^i(t,x_1,\frac{x_2}{\epsilon },\frac{x_3}{\epsilon })+{\epsilon }^3{V}_1^i(t,x_1,\frac{x_2}{\epsilon },\frac{x_3}{\epsilon })+{\epsilon }^4{V}_2^i(t,x_1,\frac{x_2}{\epsilon },\frac{x_3}{\epsilon })+\cdots \\ & W_{\epsilon }^i(t,\mathit{x})={\epsilon }^2{W}_0^i(t,x_1,\frac{x_2}{\epsilon },\frac{x_3}{\epsilon })+{\epsilon }^3{W}_1^i(t,x_1,\frac{x_2}{\epsilon },\frac{x_3}{\epsilon })+{\epsilon }^4{W}_2^i(t,x_1,\frac{x_2}{\epsilon },\frac{x_3}{\epsilon })+\cdots \\ & P_{\epsilon }(t,\mathit{x})=P_0(t,x_1)+\epsilon P_1(t,x_1,\frac{x_2}{\epsilon },\frac{x_3}{\epsilon })+{\epsilon }^2{P}_2(t,x_1,\frac{x_2}{\epsilon },\frac{x_3}{\epsilon })+{\epsilon }^3{P}_3(t,x_1,\frac{x_2}{\epsilon },\frac{x_3}{\epsilon })+\cdots \end{array}$(17)

4.1.1. Zero-order approximation

We first compute the zero-order approximation for the velocity ${\mathbf{\text{V}}}_0=(V_0^1,V_0^2,V_0^3)$ and the microrotation ${\mathbf{\text{W}}}_0=(W_0^1,W_0^2,W_0^3)$. Plugging the expansions (17(16) $\begin{array}{rl}& -\beta (\frac{{\mathrm{\partial }}^2{W}_{\epsilon }^1}{\mathrm{\partial }{x}_1\mathrm{\partial }{x}_3}-\kappa (\frac{\mathrm{\partial }{W}_{\epsilon }^2}{\mathrm{\partial }{x}_3}\cos \alpha -\frac{\mathrm{\partial }{W}_{\epsilon }^3}{\mathrm{\partial }{x}_3}\sin \alpha )+\kappa \tau W_{\epsilon }^1\cos \alpha \\ & -({\kappa }^{\prime }{W}_{\epsilon }^1+{\kappa }^2\cos \alpha W_{\epsilon }^2-{\kappa }^2\sin \alpha W_{\epsilon }^3)\sin \alpha ){\mathbf{\text{a}}}^3\\ & -\beta (2\kappa (\frac{\mathrm{\partial }{W}_{\epsilon }^1}{\mathrm{\partial }{x}_1}-\kappa (W_{\epsilon }^2\cos \alpha -W_{\epsilon }^3\sin \alpha ))\sin \alpha \\ & +{\kappa }^{\prime }({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{x}}_{\ast })\frac{\mathrm{\partial }{W}_{\epsilon }^1}{\mathrm{\partial }{x}_3}+\kappa \tau ({\mathbf{\text{e}}}_{\alpha }^{\perp }\cdot {\mathit{x}}_{\ast })\frac{\mathrm{\partial }{W}_{\epsilon }^1}{\mathrm{\partial }{x}_3}+{\kappa }^2({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{x}}_{\ast })\cos \alpha \frac{\mathrm{\partial }{W}_{\epsilon }^2}{\mathrm{\partial }{x}_3}){\mathbf{\text{a}}}^3\\ & -\beta (-{\kappa }^2({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{x}}_{\ast })\frac{\mathrm{\partial }{W}_{\epsilon }^3}{\mathrm{\partial }{x}_3}\sin \alpha +\frac{{\mathrm{\partial }}^2{W}_{\epsilon }^2}{\mathrm{\partial }{x}_2\mathrm{\partial }{x}_3}+\frac{{\mathrm{\partial }}^2{W}_{\epsilon }^3}{\mathrm{\partial }{x}_3^2}+2\kappa ({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{x}}_{\ast })\\ & (\frac{{\mathrm{\partial }}^2{W}_{\epsilon }^1}{\mathrm{\partial }{x}_1\mathrm{\partial }{x}_3}-\kappa (\frac{\mathrm{\partial }{W}_{\epsilon }^2}{\mathrm{\partial }{x}_3}\cos \alpha -\frac{\mathrm{\partial }{W}_{\epsilon }^3}{\mathrm{\partial }{x}_3}\sin \alpha ))]{\mathbf{\text{a}}}^3\\ & +2a(1+\kappa ({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{x}}_{\ast })+\cdots )W_{\epsilon }^1\mathbf{\text{t}}+2a{W}_{\epsilon }^2{\mathbf{\text{a}}}^2+2a{W}_{\epsilon }^3{\mathbf{\text{a}}}^3\\ & =a(\frac{\mathrm{\partial }{V}_{\epsilon }^3}{\mathrm{\partial }{x}_2}-\frac{\mathrm{\partial }{V}_{\epsilon }^2}{\mathrm{\partial }{x}_3})\mathbf{\text{t}}+a((1+\kappa ({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{x}}_{\ast })+\cdots )(\frac{\mathrm{\partial }{V}_{\epsilon }^1}{\mathrm{\partial }{x}_3}-\frac{\mathrm{\partial }{V}_{\epsilon }^3}{\mathrm{\partial }{x}_1})){\mathbf{\text{a}}}^2\\ & +a((1+\kappa ({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{x}}_{\ast })+\cdots )(\frac{\mathrm{\partial }{V}_{\epsilon }^2}{\mathrm{\partial }{x}_1}-\frac{\mathrm{\partial }{V}_{\epsilon }^1}{\mathrm{\partial }{x}_2})){\mathbf{\text{a}}}^3+g_1\mathbf{\text{t}}+(g_2\cos \alpha +g_3\sin \alpha ){\mathbf{\text{a}}}^2\\ & +(g_3\cos \alpha -g_2\sin \alpha ){\mathbf{\text{a}}}^3.\end{array}$(16) ) into the equations (14(13) ${\mathbf{\text{e}}}_{\alpha }=(\cos \alpha ,-\sin \alpha ),{\mathbf{\text{e}}}_{\alpha }^{\perp }=(\sin \alpha ,\cos \alpha ).$(13) )–(15(14) $\begin{array}{rl}& -\mu ({\kappa }^2\cos \alpha ({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{x}}_{\ast })\frac{\mathrm{\partial }{V}_{\epsilon }^3}{\mathrm{\partial }{x}_2}-{\kappa }^2\sin \alpha ({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{x}}_{\ast })\frac{\mathrm{\partial }{V}_{\epsilon }^3}{\mathrm{\partial }{x}_3}){\mathbf{\text{a}}}^3\\ & +\frac{\mathrm{\partial }{P}_{\epsilon }}{\mathrm{\partial }{x}_1}(1+\kappa ({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{x}}_{\ast })+\cdots )\mathbf{\text{t}}+\frac{\mathrm{\partial }{P}_{\epsilon }}{\mathrm{\partial }{x}_2}{\mathbf{\text{a}}}^2+\frac{\mathrm{\partial }{P}_{\epsilon }}{\mathrm{\partial }{x}_3}{\mathbf{\text{a}}}^3\\ & =a(\frac{\mathrm{\partial }{W}_{\epsilon }^3}{\mathrm{\partial }{x}_2}-\frac{\mathrm{\partial }{W}_{\epsilon }^2}{\mathrm{\partial }{x}_3})\mathbf{\text{t}}+a(1+\kappa ({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{x}}_{\ast })+\cdots )(\frac{\mathrm{\partial }{W}_{\epsilon }^1}{\mathrm{\partial }{x}_3}-\frac{\mathrm{\partial }{W}_{\epsilon }^3}{\mathrm{\partial }{x}_1}){\mathbf{\text{a}}}^2+a(1+\kappa ({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{x}}_{\ast })+\cdots )\\ & (\frac{\mathrm{\partial }{W}_{\epsilon }^2}{\mathrm{\partial }{x}_1}-\frac{\mathrm{\partial }{W}_{\epsilon }^1}{\mathrm{\partial }{x}_2}){\mathbf{\text{a}}}^3\\ & +f_1\mathbf{\text{t}}+(f_2\cos \alpha +f_3\sin \alpha ){\mathbf{\text{a}}}^2+(f_3\cos \alpha -f_2\sin \alpha ){\mathbf{\text{a}}}^3.\end{array}$(14) ) and collecting the ${\epsilon }^0$ terms, we obtain the following: $\begin{array}{rl}& -\mu ({\mathrm{\Delta }}_{{\mathit{y}}_{\ast }}{V}_0^1\mathbf{\text{t}}+{\mathrm{\Delta }}_{{\mathit{y}}_{\ast }}{V}_0^2{\mathbf{\text{a}}}^2+{\mathrm{\Delta }}_{{\mathit{y}}_{\ast }}{V}_0^3{\mathbf{\text{a}}}^3)+\frac{\mathrm{\partial }{P}_0}{\mathrm{\partial }{x}_1}\mathbf{\text{t}}+\frac{\mathrm{\partial }{P}_1}{\mathrm{\partial }{y}_2}{\mathbf{\text{a}}}^2+\frac{\mathrm{\partial }{P}_1}{\mathrm{\partial }{y}_3}{\mathbf{\text{a}}}^3\\ & =f_1\mathbf{\text{t}}+(\cos \alpha f_2+\sin \alpha f_3){\mathbf{\text{a}}}^2+(-\sin \alpha f_2+\cos \alpha f_3){\mathbf{\text{a}}}^3.\end{array}$ where ${\mathit{y}}_{\ast }=\frac{{\mathit{x}}_{\ast }}{\epsilon }$. In this way, we obtain the problem for the velocity and pressure zero-order approximation posed in $\mathrm{\Omega }=⟨0,l⟩\times B$: (18) $\begin{array}{rl}& -\mu ({\mathrm{\Delta }}_{{\mathit{y}}_{\ast }}{V}_0^1,{\mathrm{\Delta }}_{{\mathit{y}}_{\ast }}{V}_0^2,{\mathrm{\Delta }}_{{\mathit{y}}_{\ast }}{V}_0^3)+(\frac{\mathrm{\partial }{P}_0}{\mathrm{\partial }{x}_1},\frac{\mathrm{\partial }{P}_1}{\mathrm{\partial }{y}_2},\frac{\mathrm{\partial }{P}_1}{\mathrm{\partial }{y}_3})=(f_1,f_2\cos \alpha +f_3\sin \alpha ,f_3\cos \alpha -f_2\sin \alpha )\text{in}\mathrm{\Omega },\\ & {\text{div}}_{{\mathit{y}}_{\ast }}{\mathbf{\text{V}}}_0=0\text{in}\mathrm{\Omega },{\mathbf{\text{V}}}_0=\mathbf{\text{0}}\text{on}\mathrm{\Gamma }=⟨0,l⟩\times \mathrm{\partial }B.\end{array}$(18) The system (18(18) $\begin{array}{rl}& -\mu ({\mathrm{\Delta }}_{{\mathit{y}}_{\ast }}{V}_0^1,{\mathrm{\Delta }}_{{\mathit{y}}_{\ast }}{V}_0^2,{\mathrm{\Delta }}_{{\mathit{y}}_{\ast }}{V}_0^3)+(\frac{\mathrm{\partial }{P}_0}{\mathrm{\partial }{x}_1},\frac{\mathrm{\partial }{P}_1}{\mathrm{\partial }{y}_2},\frac{\mathrm{\partial }{P}_1}{\mathrm{\partial }{y}_3})=(f_1,f_2\cos \alpha +f_3\sin \alpha ,f_3\cos \alpha -f_2\sin \alpha )\text{in}\mathrm{\Omega },\\ & {\text{div}}_{{\mathit{y}}_{\ast }}{\mathbf{\text{V}}}_0=0\text{in}\mathrm{\Omega },{\mathbf{\text{V}}}_0=\mathbf{\text{0}}\text{on}\mathrm{\Gamma }=⟨0,l⟩\times \mathrm{\partial }B.\end{array}$(18) ) can be solved by taking (19) $\begin{array}{rl}& V_0^1(t,x_1,{\mathit{y}}_{\ast })=\frac{1}{4\mu }(1-|{\mathit{y}}_{\ast }{|}^2\left)(f_1\right(t,x_1)-\frac{\mathrm{\partial }{P}_0(x_1,t)}{\mathrm{\partial }{x}_1}),V_0^2=V_0^3=0,\\ & P_1(t,x_1,{\mathit{y}}_{\ast })=f_2(t,x_1)\left({\mathbf{\text{e}}}_{\alpha }\right(x_1)\cdot {\mathit{y}}_{\ast })+f_3(t,x_1)\left({\mathbf{\text{e}}}_{\alpha }^{\perp }\right(x_1)\cdot {\mathit{y}}_{\ast }),\end{array}$(19) where ${\mathbf{\text{e}}}_{\alpha }\left(x_1\right)$ and ${\mathbf{\text{e}}}_{\alpha }^{\perp }\left(x_1\right)$ are given by (13(13) ${\mathbf{\text{e}}}_{\alpha }=(\cos \alpha ,-\sin \alpha ),{\mathbf{\text{e}}}_{\alpha }^{\perp }=(\sin \alpha ,\cos \alpha ).$(13) ).

To determine the zero-order pressure approximation, we collect the ${\epsilon }^2$ terms in the divergence equation to obtain: (20) $\frac{\mathrm{\partial }{V}_0^1}{\mathrm{\partial }{x}_1}+{\text{div}}_{y\ast }{\mathbf{\text{V}}}_1=0\text{in}\mathrm{\Omega }.$(20) Integrating (20(20) $\frac{\mathrm{\partial }{V}_0^1}{\mathrm{\partial }{x}_1}+{\text{div}}_{y\ast }{\mathbf{\text{V}}}_1=0\text{in}\mathrm{\Omega }.$(20) ) over B and using the divergence theorem yields $\frac{\mathrm{\partial }}{\mathrm{\partial }{x}_1}{\int }_B{V}_0^1=0.$ The boundary conditions give ${\int }_B{V}_0^1(t,0,{\mathit{y}}_{\ast })={\int }_B{V}_0^1(t,l,{\mathit{y}}_{\ast })=F_0^{\ast }\left(t\right),$ (justified in Section 4.2) implying (21) ${\int }_B{V}_0^1=F_0^{\ast }\left(t\right).$(21) From (19(19) $\begin{array}{rl}& V_0^1(t,x_1,{\mathit{y}}_{\ast })=\frac{1}{4\mu }(1-|{\mathit{y}}_{\ast }{|}^2\left)(f_1\right(t,x_1)-\frac{\mathrm{\partial }{P}_0(x_1,t)}{\mathrm{\partial }{x}_1}),V_0^2=V_0^3=0,\\ & P_1(t,x_1,{\mathit{y}}_{\ast })=f_2(t,x_1)\left({\mathbf{\text{e}}}_{\alpha }\right(x_1)\cdot {\mathit{y}}_{\ast })+f_3(t,x_1)\left({\mathbf{\text{e}}}_{\alpha }^{\perp }\right(x_1)\cdot {\mathit{y}}_{\ast }),\end{array}$(19) ) and (21(21) ${\int }_B{V}_0^1=F_0^{\ast }\left(t\right).$(21) ), we now get $\frac{\pi }{8\mu }(f_1(t,x_1)-\frac{\mathrm{\partial }{P}_0(t,x_1)}{\mathrm{\partial }{x}_1})=F_0^{\ast }\left(t\right)$ to obtain (22) $P_0(t,x_1)=-\frac{8\mu }{\pi }{F}_0^{\ast }\left(t\right)x_1+{\int }_0^{x_1}{f}_1(t,\xi )d\xi +p_0\left(t\right),$(22) where $p_0\left(t\right)$ is an arbitrary function of time. It now follows from (19(19) $\begin{array}{rl}& V_0^1(t,x_1,{\mathit{y}}_{\ast })=\frac{1}{4\mu }(1-|{\mathit{y}}_{\ast }{|}^2\left)(f_1\right(t,x_1)-\frac{\mathrm{\partial }{P}_0(x_1,t)}{\mathrm{\partial }{x}_1}),V_0^2=V_0^3=0,\\ & P_1(t,x_1,{\mathit{y}}_{\ast })=f_2(t,x_1)\left({\mathbf{\text{e}}}_{\alpha }\right(x_1)\cdot {\mathit{y}}_{\ast })+f_3(t,x_1)\left({\mathbf{\text{e}}}_{\alpha }^{\perp }\right(x_1)\cdot {\mathit{y}}_{\ast }),\end{array}$(19) )₁ and (22(22) $P_0(t,x_1)=-\frac{8\mu }{\pi }{F}_0^{\ast }\left(t\right)x_1+{\int }_0^{x_1}{f}_1(t,\xi )d\xi +p_0\left(t\right),$(22) ) that (23) $V_0^1(t,{\mathit{y}}_{\ast })=\frac{2}{\pi }(1-|{\mathit{y}}_{\ast }{|}^2\left)F_0^{\ast }\right(t).$(23) We now turn our attention to computing the microrotation zero-order approximation ${\mathbf{\text{W}}}_0=(W_0^1,W_0^2,W_0^3)$. Plugging the expansions (17(17) $\begin{array}{rl}& V_{\epsilon }^i(t,\mathit{x})={\epsilon }^2{V}_0^i(t,x_1,\frac{x_2}{\epsilon },\frac{x_3}{\epsilon })+{\epsilon }^3{V}_1^i(t,x_1,\frac{x_2}{\epsilon },\frac{x_3}{\epsilon })+{\epsilon }^4{V}_2^i(t,x_1,\frac{x_2}{\epsilon },\frac{x_3}{\epsilon })+\cdots \\ & W_{\epsilon }^i(t,\mathit{x})={\epsilon }^2{W}_0^i(t,x_1,\frac{x_2}{\epsilon },\frac{x_3}{\epsilon })+{\epsilon }^3{W}_1^i(t,x_1,\frac{x_2}{\epsilon },\frac{x_3}{\epsilon })+{\epsilon }^4{W}_2^i(t,x_1,\frac{x_2}{\epsilon },\frac{x_3}{\epsilon })+\cdots \\ & P_{\epsilon }(t,\mathit{x})=P_0(t,x_1)+\epsilon P_1(t,x_1,\frac{x_2}{\epsilon },\frac{x_3}{\epsilon })+{\epsilon }^2{P}_2(t,x_1,\frac{x_2}{\epsilon },\frac{x_3}{\epsilon })+{\epsilon }^3{P}_3(t,x_1,\frac{x_2}{\epsilon },\frac{x_3}{\epsilon })+\cdots \end{array}$(17) ) into (16(16) $\begin{array}{rl}& -\beta (\frac{{\mathrm{\partial }}^2{W}_{\epsilon }^1}{\mathrm{\partial }{x}_1\mathrm{\partial }{x}_3}-\kappa (\frac{\mathrm{\partial }{W}_{\epsilon }^2}{\mathrm{\partial }{x}_3}\cos \alpha -\frac{\mathrm{\partial }{W}_{\epsilon }^3}{\mathrm{\partial }{x}_3}\sin \alpha )+\kappa \tau W_{\epsilon }^1\cos \alpha \\ & -({\kappa }^{\prime }{W}_{\epsilon }^1+{\kappa }^2\cos \alpha W_{\epsilon }^2-{\kappa }^2\sin \alpha W_{\epsilon }^3)\sin \alpha ){\mathbf{\text{a}}}^3\\ & -\beta (2\kappa (\frac{\mathrm{\partial }{W}_{\epsilon }^1}{\mathrm{\partial }{x}_1}-\kappa (W_{\epsilon }^2\cos \alpha -W_{\epsilon }^3\sin \alpha ))\sin \alpha \\ & +{\kappa }^{\prime }({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{x}}_{\ast })\frac{\mathrm{\partial }{W}_{\epsilon }^1}{\mathrm{\partial }{x}_3}+\kappa \tau ({\mathbf{\text{e}}}_{\alpha }^{\perp }\cdot {\mathit{x}}_{\ast })\frac{\mathrm{\partial }{W}_{\epsilon }^1}{\mathrm{\partial }{x}_3}+{\kappa }^2({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{x}}_{\ast })\cos \alpha \frac{\mathrm{\partial }{W}_{\epsilon }^2}{\mathrm{\partial }{x}_3}){\mathbf{\text{a}}}^3\\ & -\beta (-{\kappa }^2({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{x}}_{\ast })\frac{\mathrm{\partial }{W}_{\epsilon }^3}{\mathrm{\partial }{x}_3}\sin \alpha +\frac{{\mathrm{\partial }}^2{W}_{\epsilon }^2}{\mathrm{\partial }{x}_2\mathrm{\partial }{x}_3}+\frac{{\mathrm{\partial }}^2{W}_{\epsilon }^3}{\mathrm{\partial }{x}_3^2}+2\kappa ({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{x}}_{\ast })\\ & (\frac{{\mathrm{\partial }}^2{W}_{\epsilon }^1}{\mathrm{\partial }{x}_1\mathrm{\partial }{x}_3}-\kappa (\frac{\mathrm{\partial }{W}_{\epsilon }^2}{\mathrm{\partial }{x}_3}\cos \alpha -\frac{\mathrm{\partial }{W}_{\epsilon }^3}{\mathrm{\partial }{x}_3}\sin \alpha ))]{\mathbf{\text{a}}}^3\\ & +2a(1+\kappa ({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{x}}_{\ast })+\cdots )W_{\epsilon }^1\mathbf{\text{t}}+2a{W}_{\epsilon }^2{\mathbf{\text{a}}}^2+2a{W}_{\epsilon }^3{\mathbf{\text{a}}}^3\\ & =a(\frac{\mathrm{\partial }{V}_{\epsilon }^3}{\mathrm{\partial }{x}_2}-\frac{\mathrm{\partial }{V}_{\epsilon }^2}{\mathrm{\partial }{x}_3})\mathbf{\text{t}}+a((1+\kappa ({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{x}}_{\ast })+\cdots )(\frac{\mathrm{\partial }{V}_{\epsilon }^1}{\mathrm{\partial }{x}_3}-\frac{\mathrm{\partial }{V}_{\epsilon }^3}{\mathrm{\partial }{x}_1})){\mathbf{\text{a}}}^2\\ & +a((1+\kappa ({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{x}}_{\ast })+\cdots )(\frac{\mathrm{\partial }{V}_{\epsilon }^2}{\mathrm{\partial }{x}_1}-\frac{\mathrm{\partial }{V}_{\epsilon }^1}{\mathrm{\partial }{x}_2})){\mathbf{\text{a}}}^3+g_1\mathbf{\text{t}}+(g_2\cos \alpha +g_3\sin \alpha ){\mathbf{\text{a}}}^2\\ & +(g_3\cos \alpha -g_2\sin \alpha ){\mathbf{\text{a}}}^3.\end{array}$(16) ), we arrive at the following problem: $\begin{array}{rl}& -\delta {\mathrm{\Delta }}_{{\mathit{y}}_{\ast }}{\mathbf{\text{W}}}_0-\beta {\mathrm{\nabla }}_{{\mathit{y}}_{\ast }}\left({\text{div}}_{{\mathit{y}}_{\ast }}{\mathbf{\text{W}}}_0\right)=(g_1,g_2\cos \alpha +g_3\sin \alpha ,g_3\cos \alpha -g_2\sin \alpha )\text{in}\mathrm{\Omega },\\ & {\mathbf{\text{W}}}_0=0\text{on}\mathrm{\Gamma }\end{array}$ leading to (24) $\begin{array}{rl}& W_0^1(t,x_1,{\mathit{y}}_{\ast })=\frac{1}{4\delta }(1-|{\mathit{y}}_{\ast }{|}^2\left)g_1\right(t,x_1),\\ & W_0^2(t,x_1,{\mathit{y}}_{\ast })=\frac{1}{2(2\delta +\beta )}(1-|{\mathit{y}}_{\ast }{|}^2\left)\right(g_2(t,x_1)\cos \alpha \left(x_1\right)+g_3(t,x_1)\sin \alpha \left(x_1\right)),\\ & W_0^3(t,x_1,{\mathit{y}}_{\ast })=\frac{1}{2(2\delta +\beta )}(1-|{\mathit{y}}_{\ast }{|}^2\left)\right(g_3(t,x_1)\cos \alpha \left(x_1\right)-g_2(t,x_1)\sin \alpha \left(x_1\right)).\end{array}$(24) As expected, the zero-order velocity and microrotation approximation given by (23(23) $V_0^1(t,{\mathit{y}}_{\ast })=\frac{2}{\pi }(1-|{\mathit{y}}_{\ast }{|}^2\left)F_0^{\ast }\right(t).$(23) ) and (24(24) $\begin{array}{rl}& W_0^1(t,x_1,{\mathit{y}}_{\ast })=\frac{1}{4\delta }(1-|{\mathit{y}}_{\ast }{|}^2\left)g_1\right(t,x_1),\\ & W_0^2(t,x_1,{\mathit{y}}_{\ast })=\frac{1}{2(2\delta +\beta )}(1-|{\mathit{y}}_{\ast }{|}^2\left)\right(g_2(t,x_1)\cos \alpha \left(x_1\right)+g_3(t,x_1)\sin \alpha \left(x_1\right)),\\ & W_0^3(t,x_1,{\mathit{y}}_{\ast })=\frac{1}{2(2\delta +\beta )}(1-|{\mathit{y}}_{\ast }{|}^2\left)\right(g_3(t,x_1)\cos \alpha \left(x_1\right)-g_2(t,x_1)\sin \alpha \left(x_1\right)).\end{array}$(24) ) do not feel the effects of curvature, torsion, micropolarity or the time derivative so we need to compute the first- and second-order correctors to capture those effects.

4.1.2. First-order corrector

Again, substituting (17(17) $\begin{array}{rl}& V_{\epsilon }^i(t,\mathit{x})={\epsilon }^2{V}_0^i(t,x_1,\frac{x_2}{\epsilon },\frac{x_3}{\epsilon })+{\epsilon }^3{V}_1^i(t,x_1,\frac{x_2}{\epsilon },\frac{x_3}{\epsilon })+{\epsilon }^4{V}_2^i(t,x_1,\frac{x_2}{\epsilon },\frac{x_3}{\epsilon })+\cdots \\ & W_{\epsilon }^i(t,\mathit{x})={\epsilon }^2{W}_0^i(t,x_1,\frac{x_2}{\epsilon },\frac{x_3}{\epsilon })+{\epsilon }^3{W}_1^i(t,x_1,\frac{x_2}{\epsilon },\frac{x_3}{\epsilon })+{\epsilon }^4{W}_2^i(t,x_1,\frac{x_2}{\epsilon },\frac{x_3}{\epsilon })+\cdots \\ & P_{\epsilon }(t,\mathit{x})=P_0(t,x_1)+\epsilon P_1(t,x_1,\frac{x_2}{\epsilon },\frac{x_3}{\epsilon })+{\epsilon }^2{P}_2(t,x_1,\frac{x_2}{\epsilon },\frac{x_3}{\epsilon })+{\epsilon }^3{P}_3(t,x_1,\frac{x_2}{\epsilon },\frac{x_3}{\epsilon })+\cdots \end{array}$(17) ) into the Equation (14(14) $\begin{array}{rl}& -\mu ({\kappa }^2\cos \alpha ({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{x}}_{\ast })\frac{\mathrm{\partial }{V}_{\epsilon }^3}{\mathrm{\partial }{x}_2}-{\kappa }^2\sin \alpha ({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{x}}_{\ast })\frac{\mathrm{\partial }{V}_{\epsilon }^3}{\mathrm{\partial }{x}_3}){\mathbf{\text{a}}}^3\\ & +\frac{\mathrm{\partial }{P}_{\epsilon }}{\mathrm{\partial }{x}_1}(1+\kappa ({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{x}}_{\ast })+\cdots )\mathbf{\text{t}}+\frac{\mathrm{\partial }{P}_{\epsilon }}{\mathrm{\partial }{x}_2}{\mathbf{\text{a}}}^2+\frac{\mathrm{\partial }{P}_{\epsilon }}{\mathrm{\partial }{x}_3}{\mathbf{\text{a}}}^3\\ & =a(\frac{\mathrm{\partial }{W}_{\epsilon }^3}{\mathrm{\partial }{x}_2}-\frac{\mathrm{\partial }{W}_{\epsilon }^2}{\mathrm{\partial }{x}_3})\mathbf{\text{t}}+a(1+\kappa ({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{x}}_{\ast })+\cdots )(\frac{\mathrm{\partial }{W}_{\epsilon }^1}{\mathrm{\partial }{x}_3}-\frac{\mathrm{\partial }{W}_{\epsilon }^3}{\mathrm{\partial }{x}_1}){\mathbf{\text{a}}}^2+a(1+\kappa ({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{x}}_{\ast })+\cdots )\\ & (\frac{\mathrm{\partial }{W}_{\epsilon }^2}{\mathrm{\partial }{x}_1}-\frac{\mathrm{\partial }{W}_{\epsilon }^1}{\mathrm{\partial }{x}_2}){\mathbf{\text{a}}}^3\\ & +f_1\mathbf{\text{t}}+(f_2\cos \alpha +f_3\sin \alpha ){\mathbf{\text{a}}}^2+(f_3\cos \alpha -f_2\sin \alpha ){\mathbf{\text{a}}}^3.\end{array}$(14) ) and collecting the terms of order ε, we get the following equation for the first component of the velocity first-order corrector $V_1^1$: (25) $\begin{array}{rl}& -\mu ({\mathrm{\Delta }}_{{\mathit{y}}_{\ast }}{V}_1^1+\kappa \cos \alpha \frac{\mathrm{\partial }{V}_0^1}{\mathrm{\partial }{y}_2}-\kappa \sin \alpha \frac{\mathrm{\partial }{V}_0^1}{\mathrm{\partial }{y}_3}+\kappa ({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast }){\mathrm{\Delta }}_{{\mathit{y}}_{\ast }}{V}_0^1)+\kappa ({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast })\frac{\mathrm{\partial }{P}_0}{\mathrm{\partial }{x}_1}+\frac{\mathrm{\partial }{P}_1}{\mathrm{\partial }{x}_1}\\ & =a(\frac{\mathrm{\partial }{W}_0^3}{\mathrm{\partial }{y}_2}-\frac{\mathrm{\partial }{W}_0^2}{\mathrm{\partial }{y}_3})\text{in}\mathrm{\Omega }.\end{array}$(25) Substituting the expressions for $V_0^1,P_0,P_1,W_0^2$ and $W_0^3$ derived in the previous section and given by (19(19) $\begin{array}{rl}& V_0^1(t,x_1,{\mathit{y}}_{\ast })=\frac{1}{4\mu }(1-|{\mathit{y}}_{\ast }{|}^2\left)(f_1\right(t,x_1)-\frac{\mathrm{\partial }{P}_0(x_1,t)}{\mathrm{\partial }{x}_1}),V_0^2=V_0^3=0,\\ & P_1(t,x_1,{\mathit{y}}_{\ast })=f_2(t,x_1)\left({\mathbf{\text{e}}}_{\alpha }\right(x_1)\cdot {\mathit{y}}_{\ast })+f_3(t,x_1)\left({\mathbf{\text{e}}}_{\alpha }^{\perp }\right(x_1)\cdot {\mathit{y}}_{\ast }),\end{array}$(19) ), (22(22) $P_0(t,x_1)=-\frac{8\mu }{\pi }{F}_0^{\ast }\left(t\right)x_1+{\int }_0^{x_1}{f}_1(t,\xi )d\xi +p_0\left(t\right),$(22) ), (23(23) $V_0^1(t,{\mathit{y}}_{\ast })=\frac{2}{\pi }(1-|{\mathit{y}}_{\ast }{|}^2\left)F_0^{\ast }\right(t).$(23) ) and (24(24) $\begin{array}{rl}& W_0^1(t,x_1,{\mathit{y}}_{\ast })=\frac{1}{4\delta }(1-|{\mathit{y}}_{\ast }{|}^2\left)g_1\right(t,x_1),\\ & W_0^2(t,x_1,{\mathit{y}}_{\ast })=\frac{1}{2(2\delta +\beta )}(1-|{\mathit{y}}_{\ast }{|}^2\left)\right(g_2(t,x_1)\cos \alpha \left(x_1\right)+g_3(t,x_1)\sin \alpha \left(x_1\right)),\\ & W_0^3(t,x_1,{\mathit{y}}_{\ast })=\frac{1}{2(2\delta +\beta )}(1-|{\mathit{y}}_{\ast }{|}^2\left)\right(g_3(t,x_1)\cos \alpha \left(x_1\right)-g_2(t,x_1)\sin \alpha \left(x_1\right)).\end{array}$(24) ) into (25(25) $\begin{array}{rl}& -\mu ({\mathrm{\Delta }}_{{\mathit{y}}_{\ast }}{V}_1^1+\kappa \cos \alpha \frac{\mathrm{\partial }{V}_0^1}{\mathrm{\partial }{y}_2}-\kappa \sin \alpha \frac{\mathrm{\partial }{V}_0^1}{\mathrm{\partial }{y}_3}+\kappa ({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast }){\mathrm{\Delta }}_{{\mathit{y}}_{\ast }}{V}_0^1)+\kappa ({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast })\frac{\mathrm{\partial }{P}_0}{\mathrm{\partial }{x}_1}+\frac{\mathrm{\partial }{P}_1}{\mathrm{\partial }{x}_1}\\ & =a(\frac{\mathrm{\partial }{W}_0^3}{\mathrm{\partial }{y}_2}-\frac{\mathrm{\partial }{W}_0^2}{\mathrm{\partial }{y}_3})\text{in}\mathrm{\Omega }.\end{array}$(25) ), we obtain the following problem for $V_1^1$: (26) $\begin{array}{rl}& -\mu {\mathrm{\Delta }}_{{\mathit{y}}_{\ast }}{V}_1^1=y_2{H}_1(t,x_1)+y_3{H}_2(t,x_2)\text{in}\mathrm{\Omega },\\ & V_1^1=0\text{on}\mathrm{\Gamma },\end{array}$(26) where the functions $H_1(t,x_1)$ and $H_2(t,x_1)$ are provided in the appendix (see (A1(A1) $\begin{array}{rl}{H}_1(t,x_1)& =\cos \alpha (-\frac{4\mu \kappa }{\pi }{F}_0^{\ast }-\kappa f_1-\frac{\mathrm{\partial }{f}_2}{\mathrm{\partial }{x}_1}+\tau f_3-\frac{a}{2\delta +\beta }{g}_3)+\sin \alpha (-\tau f_2-\frac{\mathrm{\partial }{f}_3}{\mathrm{\partial }{x}_1}+\frac{a}{2\delta +\beta }{g}_2),\\ H_2(t,x_1)& =\sin \alpha (\frac{4\mu \kappa }{\pi }{F}_0^{\ast }+\kappa f_1+\frac{\mathrm{\partial }{f}_2}{\mathrm{\partial }{x}_1}-\tau f_3-\frac{a}{2\delta +\beta }{g}_3)-\cos \alpha (\tau f_2+\frac{\mathrm{\partial }{f}_3}{\mathrm{\partial }{x}_1}+\frac{a}{2\delta +\beta }{g}_2).\end{array}$(A1) )).

It can be easily shown that the solution of problem (26(26) $\begin{array}{rl}& -\mu {\mathrm{\Delta }}_{{\mathit{y}}_{\ast }}{V}_1^1=y_2{H}_1(t,x_1)+y_3{H}_2(t,x_2)\text{in}\mathrm{\Omega },\\ & V_1^1=0\text{on}\mathrm{\Gamma },\end{array}$(26) ) is given by (27) $V_1^1(t,x_1,{\mathit{y}}_{\ast })=\frac{1}{8\mu }(1-|{\mathit{y}}_{\ast }{|}^2\left)\right(y_2{H}_1(t,x_1)+y_3{H}_2(t,x_1)).$(27) In a similar manner, we obtain the equations for the two other velocity components ${\mathbf{\text{V}}}_1^{\ast }=(V_1^2,V_1^3)$: (28) $\begin{array}{rl}& -\mu ({\mathrm{\Delta }}_{{\mathit{y}}_{\ast }}{V}_1^2-\kappa \cos \alpha \frac{\mathrm{\partial }{V}_0^2}{\mathrm{\partial }{y}_2}+\kappa \sin \alpha \frac{\mathrm{\partial }{V}_0^2}{\mathrm{\partial }{y}_3},{\mathrm{\Delta }}_{{\mathit{y}}_{\ast }}{V}_1^3+\kappa \sin \alpha \frac{\mathrm{\partial }{V}_0^3}{\mathrm{\partial }{y}_3}-\kappa \cos \alpha \frac{\mathrm{\partial }{V}_0^3}{\mathrm{\partial }{y}_2})\\ & +(\frac{\mathrm{\partial }{P}_2}{\mathrm{\partial }{y}_2},\frac{\mathrm{\partial }{P}_2}{\mathrm{\partial }{y}_3})=a(\frac{\mathrm{\partial }{W}_0^1}{\mathrm{\partial }{y}_3},-\frac{\mathrm{\partial }{W}_0^1}{\mathrm{\partial }{y}_2})\text{in}\mathrm{\Omega },\\ & \frac{\mathrm{\partial }{V}_0^1}{\mathrm{\partial }{x}_1}-\kappa V_0^2+\frac{\mathrm{\partial }{V}_1^2}{\mathrm{\partial }{y}_2}+\frac{\mathrm{\partial }{V}_1^3}{\mathrm{\partial }{y}_3}=0\text{in}\mathrm{\Omega },{\mathbf{\text{V}}}_1^{\ast }=\mathbf{\text{0}}\text{on}\mathrm{\Gamma }.\end{array}$(28) Applying the expressions for $V_0^1,V_0^2,V_0^3$ and $W_0^1$ given by (19(19) $\begin{array}{rl}& V_0^1(t,x_1,{\mathit{y}}_{\ast })=\frac{1}{4\mu }(1-|{\mathit{y}}_{\ast }{|}^2\left)(f_1\right(t,x_1)-\frac{\mathrm{\partial }{P}_0(x_1,t)}{\mathrm{\partial }{x}_1}),V_0^2=V_0^3=0,\\ & P_1(t,x_1,{\mathit{y}}_{\ast })=f_2(t,x_1)\left({\mathbf{\text{e}}}_{\alpha }\right(x_1)\cdot {\mathit{y}}_{\ast })+f_3(t,x_1)\left({\mathbf{\text{e}}}_{\alpha }^{\perp }\right(x_1)\cdot {\mathit{y}}_{\ast }),\end{array}$(19) ), (23(23) $V_0^1(t,{\mathit{y}}_{\ast })=\frac{2}{\pi }(1-|{\mathit{y}}_{\ast }{|}^2\left)F_0^{\ast }\right(t).$(23) ) and (24(24) $\begin{array}{rl}& W_0^1(t,x_1,{\mathit{y}}_{\ast })=\frac{1}{4\delta }(1-|{\mathit{y}}_{\ast }{|}^2\left)g_1\right(t,x_1),\\ & W_0^2(t,x_1,{\mathit{y}}_{\ast })=\frac{1}{2(2\delta +\beta )}(1-|{\mathit{y}}_{\ast }{|}^2\left)\right(g_2(t,x_1)\cos \alpha \left(x_1\right)+g_3(t,x_1)\sin \alpha \left(x_1\right)),\\ & W_0^3(t,x_1,{\mathit{y}}_{\ast })=\frac{1}{2(2\delta +\beta )}(1-|{\mathit{y}}_{\ast }{|}^2\left)\right(g_3(t,x_1)\cos \alpha \left(x_1\right)-g_2(t,x_1)\sin \alpha \left(x_1\right)).\end{array}$(24) ) into the equation (28), we arrive at: (29) $\begin{array}{rl}& -\mu {\mathrm{\Delta }}_{{\mathit{y}}_{\ast }}{\mathbf{\text{V}}}_1^{\ast }+{\mathrm{\nabla }}_{{\mathit{y}}_{\ast }}{P}_2=-\frac{a}{2\delta }{g}_1(y_3,-y_2)\text{in}\mathrm{\Omega },\\ & {\text{div}}_{{\mathit{y}}_{\ast }}{\mathbf{\text{V}}}_1^{\ast }=0\text{in}\mathrm{\Omega },{\mathbf{\text{V}}}_1^{\ast }=\mathbf{\text{0}}\text{on}\mathrm{\Gamma }.\end{array}$(29) The solution of the problem (29(29) $\begin{array}{rl}& -\mu {\mathrm{\Delta }}_{{\mathit{y}}_{\ast }}{\mathbf{\text{V}}}_1^{\ast }+{\mathrm{\nabla }}_{{\mathit{y}}_{\ast }}{P}_2=-\frac{a}{2\delta }{g}_1(y_3,-y_2)\text{in}\mathrm{\Omega },\\ & {\text{div}}_{{\mathit{y}}_{\ast }}{\mathbf{\text{V}}}_1^{\ast }=0\text{in}\mathrm{\Omega },{\mathbf{\text{V}}}_1^{\ast }=\mathbf{\text{0}}\text{on}\mathrm{\Gamma }.\end{array}$(29) ) reads: (30) $\begin{array}{rl}& V_1^2(t,x_1,{\mathit{y}}_{\ast })=-\frac{a}{16\mu \delta }{g}_1(t,x_1)(1-|{\mathit{y}}_{\ast }{|}^2)y_3,V_1^3(t,x_1,{\mathit{y}}_{\ast })=\frac{a}{16\mu \delta }{g}_1(t,x_1\left)\right(1-\left|{\mathit{y}}_{\ast }{|}^2\right)y_2,\\ & P_2(t,x_1)=x_1{p}_1\left(t\right)+\frac{1}{2}{x}_1^2(\frac{p_2\left(t\right)-p_1\left(t\right)}{l}),\end{array}$(30) where $p_1\left(t\right)$ and $p_2\left(t\right)$ will be determined in Section 4.2.1 from the compatibility conditions related to the second-order boundary layer correctors for the velocity and pressure.

We will now determine the expressions for the microrotation first-order correctors. Plugging (17(17) $\begin{array}{rl}& V_{\epsilon }^i(t,\mathit{x})={\epsilon }^2{V}_0^i(t,x_1,\frac{x_2}{\epsilon },\frac{x_3}{\epsilon })+{\epsilon }^3{V}_1^i(t,x_1,\frac{x_2}{\epsilon },\frac{x_3}{\epsilon })+{\epsilon }^4{V}_2^i(t,x_1,\frac{x_2}{\epsilon },\frac{x_3}{\epsilon })+\cdots \\ & W_{\epsilon }^i(t,\mathit{x})={\epsilon }^2{W}_0^i(t,x_1,\frac{x_2}{\epsilon },\frac{x_3}{\epsilon })+{\epsilon }^3{W}_1^i(t,x_1,\frac{x_2}{\epsilon },\frac{x_3}{\epsilon })+{\epsilon }^4{W}_2^i(t,x_1,\frac{x_2}{\epsilon },\frac{x_3}{\epsilon })+\cdots \\ & P_{\epsilon }(t,\mathit{x})=P_0(t,x_1)+\epsilon P_1(t,x_1,\frac{x_2}{\epsilon },\frac{x_3}{\epsilon })+{\epsilon }^2{P}_2(t,x_1,\frac{x_2}{\epsilon },\frac{x_3}{\epsilon })+{\epsilon }^3{P}_3(t,x_1,\frac{x_2}{\epsilon },\frac{x_3}{\epsilon })+\cdots \end{array}$(17) ) into the Equation (16(16) $\begin{array}{rl}& -\beta (\frac{{\mathrm{\partial }}^2{W}_{\epsilon }^1}{\mathrm{\partial }{x}_1\mathrm{\partial }{x}_3}-\kappa (\frac{\mathrm{\partial }{W}_{\epsilon }^2}{\mathrm{\partial }{x}_3}\cos \alpha -\frac{\mathrm{\partial }{W}_{\epsilon }^3}{\mathrm{\partial }{x}_3}\sin \alpha )+\kappa \tau W_{\epsilon }^1\cos \alpha \\ & -({\kappa }^{\prime }{W}_{\epsilon }^1+{\kappa }^2\cos \alpha W_{\epsilon }^2-{\kappa }^2\sin \alpha W_{\epsilon }^3)\sin \alpha ){\mathbf{\text{a}}}^3\\ & -\beta (2\kappa (\frac{\mathrm{\partial }{W}_{\epsilon }^1}{\mathrm{\partial }{x}_1}-\kappa (W_{\epsilon }^2\cos \alpha -W_{\epsilon }^3\sin \alpha ))\sin \alpha \\ & +{\kappa }^{\prime }({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{x}}_{\ast })\frac{\mathrm{\partial }{W}_{\epsilon }^1}{\mathrm{\partial }{x}_3}+\kappa \tau ({\mathbf{\text{e}}}_{\alpha }^{\perp }\cdot {\mathit{x}}_{\ast })\frac{\mathrm{\partial }{W}_{\epsilon }^1}{\mathrm{\partial }{x}_3}+{\kappa }^2({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{x}}_{\ast })\cos \alpha \frac{\mathrm{\partial }{W}_{\epsilon }^2}{\mathrm{\partial }{x}_3}){\mathbf{\text{a}}}^3\\ & -\beta (-{\kappa }^2({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{x}}_{\ast })\frac{\mathrm{\partial }{W}_{\epsilon }^3}{\mathrm{\partial }{x}_3}\sin \alpha +\frac{{\mathrm{\partial }}^2{W}_{\epsilon }^2}{\mathrm{\partial }{x}_2\mathrm{\partial }{x}_3}+\frac{{\mathrm{\partial }}^2{W}_{\epsilon }^3}{\mathrm{\partial }{x}_3^2}+2\kappa ({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{x}}_{\ast })\\ & (\frac{{\mathrm{\partial }}^2{W}_{\epsilon }^1}{\mathrm{\partial }{x}_1\mathrm{\partial }{x}_3}-\kappa (\frac{\mathrm{\partial }{W}_{\epsilon }^2}{\mathrm{\partial }{x}_3}\cos \alpha -\frac{\mathrm{\partial }{W}_{\epsilon }^3}{\mathrm{\partial }{x}_3}\sin \alpha ))]{\mathbf{\text{a}}}^3\\ & +2a(1+\kappa ({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{x}}_{\ast })+\cdots )W_{\epsilon }^1\mathbf{\text{t}}+2a{W}_{\epsilon }^2{\mathbf{\text{a}}}^2+2a{W}_{\epsilon }^3{\mathbf{\text{a}}}^3\\ & =a(\frac{\mathrm{\partial }{V}_{\epsilon }^3}{\mathrm{\partial }{x}_2}-\frac{\mathrm{\partial }{V}_{\epsilon }^2}{\mathrm{\partial }{x}_3})\mathbf{\text{t}}+a((1+\kappa ({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{x}}_{\ast })+\cdots )(\frac{\mathrm{\partial }{V}_{\epsilon }^1}{\mathrm{\partial }{x}_3}-\frac{\mathrm{\partial }{V}_{\epsilon }^3}{\mathrm{\partial }{x}_1})){\mathbf{\text{a}}}^2\\ & +a((1+\kappa ({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{x}}_{\ast })+\cdots )(\frac{\mathrm{\partial }{V}_{\epsilon }^2}{\mathrm{\partial }{x}_1}-\frac{\mathrm{\partial }{V}_{\epsilon }^1}{\mathrm{\partial }{x}_2})){\mathbf{\text{a}}}^3+g_1\mathbf{\text{t}}+(g_2\cos \alpha +g_3\sin \alpha ){\mathbf{\text{a}}}^2\\ & +(g_3\cos \alpha -g_2\sin \alpha ){\mathbf{\text{a}}}^3.\end{array}$(16) ) and collecting the terms of order ε, we obtain: (31) $\begin{array}{rl}& -\delta ({\mathrm{\Delta }}_{{\mathit{y}}_{\ast }}{W}_1^1+\kappa \cos \alpha \frac{\mathrm{\partial }{W}_0^1}{\mathrm{\partial }{y}_2}-\kappa \sin \frac{\mathrm{\partial }{W}_0^1}{\mathrm{\partial }{y}_3}+\kappa ({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast }){\mathrm{\Delta }}_{{\mathit{y}}_{\ast }}{W}_0^1)-\beta (\frac{{\mathrm{\partial }}^2{W}_0^2}{\mathrm{\partial }{x}_1\mathrm{\partial }{y}_2}+\frac{{\mathrm{\partial }}^2{W}_0^3}{\mathrm{\partial }{x}_1\mathrm{\partial }{y}_3})\\ & =a(\frac{\mathrm{\partial }{V}_0^3}{\mathrm{\partial }{y}_2}-\frac{\mathrm{\partial }{V}_0^2}{\mathrm{\partial }{y}_3}).\end{array}$(31) Employing the expressions for $V_0^2,V_0^3,W_0^1,W_0^2$ and $W_0^3$ given by (19(19) $\begin{array}{rl}& V_0^1(t,x_1,{\mathit{y}}_{\ast })=\frac{1}{4\mu }(1-|{\mathit{y}}_{\ast }{|}^2\left)(f_1\right(t,x_1)-\frac{\mathrm{\partial }{P}_0(x_1,t)}{\mathrm{\partial }{x}_1}),V_0^2=V_0^3=0,\\ & P_1(t,x_1,{\mathit{y}}_{\ast })=f_2(t,x_1)\left({\mathbf{\text{e}}}_{\alpha }\right(x_1)\cdot {\mathit{y}}_{\ast })+f_3(t,x_1)\left({\mathbf{\text{e}}}_{\alpha }^{\perp }\right(x_1)\cdot {\mathit{y}}_{\ast }),\end{array}$(19) ) and (24(24) $\begin{array}{rl}& W_0^1(t,x_1,{\mathit{y}}_{\ast })=\frac{1}{4\delta }(1-|{\mathit{y}}_{\ast }{|}^2\left)g_1\right(t,x_1),\\ & W_0^2(t,x_1,{\mathit{y}}_{\ast })=\frac{1}{2(2\delta +\beta )}(1-|{\mathit{y}}_{\ast }{|}^2\left)\right(g_2(t,x_1)\cos \alpha \left(x_1\right)+g_3(t,x_1)\sin \alpha \left(x_1\right)),\\ & W_0^3(t,x_1,{\mathit{y}}_{\ast })=\frac{1}{2(2\delta +\beta )}(1-|{\mathit{y}}_{\ast }{|}^2\left)\right(g_3(t,x_1)\cos \alpha \left(x_1\right)-g_2(t,x_1)\sin \alpha \left(x_1\right)).\end{array}$(24) ) in the Equation (31(31) $\begin{array}{rl}& -\delta ({\mathrm{\Delta }}_{{\mathit{y}}_{\ast }}{W}_1^1+\kappa \cos \alpha \frac{\mathrm{\partial }{W}_0^1}{\mathrm{\partial }{y}_2}-\kappa \sin \frac{\mathrm{\partial }{W}_0^1}{\mathrm{\partial }{y}_3}+\kappa ({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast }){\mathrm{\Delta }}_{{\mathit{y}}_{\ast }}{W}_0^1)-\beta (\frac{{\mathrm{\partial }}^2{W}_0^2}{\mathrm{\partial }{x}_1\mathrm{\partial }{y}_2}+\frac{{\mathrm{\partial }}^2{W}_0^3}{\mathrm{\partial }{x}_1\mathrm{\partial }{y}_3})\\ & =a(\frac{\mathrm{\partial }{V}_0^3}{\mathrm{\partial }{y}_2}-\frac{\mathrm{\partial }{V}_0^2}{\mathrm{\partial }{y}_3}).\end{array}$(31) ), we get the following problem for $W_1^1$: (32) $\begin{array}{rl}& -\delta {\mathrm{\Delta }}_{{\mathit{y}}_{\ast }}{W}_1^1=y_2{H}_3(t,x_1)+y_3{H}_4(t,x_1)\text{in}\mathrm{\Omega },\\ & W_1^1=0\text{on}\mathrm{\Gamma },\end{array}$(32) where $H_3(t,x_1)$ and $H_4(t,x_1)$ are given in the appendix (see (A2(A2) $\begin{array}{rl}{H}_3(t,x_1)& =-\frac{3\kappa }{2}{g}_1\cos \alpha +\frac{\beta }{2\delta +\beta }((\tau g_3-\frac{\mathrm{\partial }{g}_2}{\mathrm{\partial }{x}_1})\cos \alpha -(\tau g_2+\frac{\mathrm{\partial }{g}_3}{\mathrm{\partial }{x}_1})\sin \alpha ),\\ H_4(t,x_1)& =\frac{3\kappa }{2}{g}_1\sin \alpha -\frac{\beta }{2\delta +\beta }((\tau g_2+\frac{\mathrm{\partial }{g}_3}{\mathrm{\partial }{x}_1})\cos \alpha +(\tau g_3-\frac{\mathrm{\partial }{g}_2}{\mathrm{\partial }{x}_1})\sin \alpha ).\end{array}$(A2) )).

It follows (33) $W_1^1(t,x_1,{\mathit{y}}_{\ast })=\frac{1}{8\mu }(1-|{\mathit{y}}_{\ast }{|}^2\left)\right(y_2{H}_3(t,x_1)+y_3{H}_4(t,x_1)).$(33) Substituting the expansions (17(17) $\begin{array}{rl}& V_{\epsilon }^i(t,\mathit{x})={\epsilon }^2{V}_0^i(t,x_1,\frac{x_2}{\epsilon },\frac{x_3}{\epsilon })+{\epsilon }^3{V}_1^i(t,x_1,\frac{x_2}{\epsilon },\frac{x_3}{\epsilon })+{\epsilon }^4{V}_2^i(t,x_1,\frac{x_2}{\epsilon },\frac{x_3}{\epsilon })+\cdots \\ & W_{\epsilon }^i(t,\mathit{x})={\epsilon }^2{W}_0^i(t,x_1,\frac{x_2}{\epsilon },\frac{x_3}{\epsilon })+{\epsilon }^3{W}_1^i(t,x_1,\frac{x_2}{\epsilon },\frac{x_3}{\epsilon })+{\epsilon }^4{W}_2^i(t,x_1,\frac{x_2}{\epsilon },\frac{x_3}{\epsilon })+\cdots \\ & P_{\epsilon }(t,\mathit{x})=P_0(t,x_1)+\epsilon P_1(t,x_1,\frac{x_2}{\epsilon },\frac{x_3}{\epsilon })+{\epsilon }^2{P}_2(t,x_1,\frac{x_2}{\epsilon },\frac{x_3}{\epsilon })+{\epsilon }^3{P}_3(t,x_1,\frac{x_2}{\epsilon },\frac{x_3}{\epsilon })+\cdots \end{array}$(17) ) into the Equation (16(16) $\begin{array}{rl}& -\beta (\frac{{\mathrm{\partial }}^2{W}_{\epsilon }^1}{\mathrm{\partial }{x}_1\mathrm{\partial }{x}_3}-\kappa (\frac{\mathrm{\partial }{W}_{\epsilon }^2}{\mathrm{\partial }{x}_3}\cos \alpha -\frac{\mathrm{\partial }{W}_{\epsilon }^3}{\mathrm{\partial }{x}_3}\sin \alpha )+\kappa \tau W_{\epsilon }^1\cos \alpha \\ & -({\kappa }^{\prime }{W}_{\epsilon }^1+{\kappa }^2\cos \alpha W_{\epsilon }^2-{\kappa }^2\sin \alpha W_{\epsilon }^3)\sin \alpha ){\mathbf{\text{a}}}^3\\ & -\beta (2\kappa (\frac{\mathrm{\partial }{W}_{\epsilon }^1}{\mathrm{\partial }{x}_1}-\kappa (W_{\epsilon }^2\cos \alpha -W_{\epsilon }^3\sin \alpha ))\sin \alpha \\ & +{\kappa }^{\prime }({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{x}}_{\ast })\frac{\mathrm{\partial }{W}_{\epsilon }^1}{\mathrm{\partial }{x}_3}+\kappa \tau ({\mathbf{\text{e}}}_{\alpha }^{\perp }\cdot {\mathit{x}}_{\ast })\frac{\mathrm{\partial }{W}_{\epsilon }^1}{\mathrm{\partial }{x}_3}+{\kappa }^2({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{x}}_{\ast })\cos \alpha \frac{\mathrm{\partial }{W}_{\epsilon }^2}{\mathrm{\partial }{x}_3}){\mathbf{\text{a}}}^3\\ & -\beta (-{\kappa }^2({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{x}}_{\ast })\frac{\mathrm{\partial }{W}_{\epsilon }^3}{\mathrm{\partial }{x}_3}\sin \alpha +\frac{{\mathrm{\partial }}^2{W}_{\epsilon }^2}{\mathrm{\partial }{x}_2\mathrm{\partial }{x}_3}+\frac{{\mathrm{\partial }}^2{W}_{\epsilon }^3}{\mathrm{\partial }{x}_3^2}+2\kappa ({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{x}}_{\ast })\\ & (\frac{{\mathrm{\partial }}^2{W}_{\epsilon }^1}{\mathrm{\partial }{x}_1\mathrm{\partial }{x}_3}-\kappa (\frac{\mathrm{\partial }{W}_{\epsilon }^2}{\mathrm{\partial }{x}_3}\cos \alpha -\frac{\mathrm{\partial }{W}_{\epsilon }^3}{\mathrm{\partial }{x}_3}\sin \alpha ))]{\mathbf{\text{a}}}^3\\ & +2a(1+\kappa ({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{x}}_{\ast })+\cdots )W_{\epsilon }^1\mathbf{\text{t}}+2a{W}_{\epsilon }^2{\mathbf{\text{a}}}^2+2a{W}_{\epsilon }^3{\mathbf{\text{a}}}^3\\ & =a(\frac{\mathrm{\partial }{V}_{\epsilon }^3}{\mathrm{\partial }{x}_2}-\frac{\mathrm{\partial }{V}_{\epsilon }^2}{\mathrm{\partial }{x}_3})\mathbf{\text{t}}+a((1+\kappa ({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{x}}_{\ast })+\cdots )(\frac{\mathrm{\partial }{V}_{\epsilon }^1}{\mathrm{\partial }{x}_3}-\frac{\mathrm{\partial }{V}_{\epsilon }^3}{\mathrm{\partial }{x}_1})){\mathbf{\text{a}}}^2\\ & +a((1+\kappa ({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{x}}_{\ast })+\cdots )(\frac{\mathrm{\partial }{V}_{\epsilon }^2}{\mathrm{\partial }{x}_1}-\frac{\mathrm{\partial }{V}_{\epsilon }^1}{\mathrm{\partial }{x}_2})){\mathbf{\text{a}}}^3+g_1\mathbf{\text{t}}+(g_2\cos \alpha +g_3\sin \alpha ){\mathbf{\text{a}}}^2\\ & +(g_3\cos \alpha -g_2\sin \alpha ){\mathbf{\text{a}}}^3.\end{array}$(16) ), we deduce the equations for $(W_1^2,W_1^3)$: (34) $\begin{array}{rl}& -\delta ({\mathrm{\Delta }}_{{\mathit{y}}_{\ast }}{W}_1^2-\kappa \cos \alpha \frac{\mathrm{\partial }{W}_0^2}{\mathrm{\partial }{y}_2}+\kappa \sin \alpha \frac{\mathrm{\partial }{W}_0^2}{\mathrm{\partial }{y}_3})\\ & -\beta (\frac{{\mathrm{\partial }}^2{W}_0^1}{\mathrm{\partial }{x}_1\mathrm{\partial }{y}_2}-\kappa \cos \alpha \frac{\mathrm{\partial }{W}_0^2}{\mathrm{\partial }{y}_2}+\kappa \sin \alpha \frac{\mathrm{\partial }{W}_0^3}{\mathrm{\partial }{y}_2}+\frac{{\mathrm{\partial }}^2{W}_1^2}{\mathrm{\partial }{y}_2^2}+\frac{{\mathrm{\partial }}^2{W}_1^3}{\mathrm{\partial }{y}_2\mathrm{\partial }{y}_3})=a\frac{\mathrm{\partial }{V}_0^1}{\mathrm{\partial }{y}_3},\\ & -\delta ({\mathrm{\Delta }}_{{\mathit{y}}_{\ast }}{W}_1^3+\kappa \sin \alpha \frac{\mathrm{\partial }{W}_0^3}{\mathrm{\partial }{y}_3}-\kappa \cos \alpha \frac{\mathrm{\partial }{W}_0^3}{\mathrm{\partial }{y}_2})\\ & -\beta (\frac{{\mathrm{\partial }}^2{W}_0^1}{\mathrm{\partial }{x}_1\mathrm{\partial }{y}_3}-\kappa \cos \alpha \frac{\mathrm{\partial }{W}_0^2}{\mathrm{\partial }{y}_3}+\kappa \sin \alpha \frac{\mathrm{\partial }{W}_0^3}{\mathrm{\partial }{y}_3}+\frac{{\mathrm{\partial }}^2{W}_1^3}{\mathrm{\partial }{y}_3^2}+\frac{{\mathrm{\partial }}^2{W}_1^2}{\mathrm{\partial }{y}_2\mathrm{\partial }{y}_3})=-a\frac{\mathrm{\partial }{V}_0^1}{\mathrm{\partial }{y}_2}.\end{array}$(34) In view of the expressions for $V_0^1,W_0^1,W_0^2$ and $W_0^3$ given by (19(19) $\begin{array}{rl}& V_0^1(t,x_1,{\mathit{y}}_{\ast })=\frac{1}{4\mu }(1-|{\mathit{y}}_{\ast }{|}^2\left)(f_1\right(t,x_1)-\frac{\mathrm{\partial }{P}_0(x_1,t)}{\mathrm{\partial }{x}_1}),V_0^2=V_0^3=0,\\ & P_1(t,x_1,{\mathit{y}}_{\ast })=f_2(t,x_1)\left({\mathbf{\text{e}}}_{\alpha }\right(x_1)\cdot {\mathit{y}}_{\ast })+f_3(t,x_1)\left({\mathbf{\text{e}}}_{\alpha }^{\perp }\right(x_1)\cdot {\mathit{y}}_{\ast }),\end{array}$(19) ), (23(23) $V_0^1(t,{\mathit{y}}_{\ast })=\frac{2}{\pi }(1-|{\mathit{y}}_{\ast }{|}^2\left)F_0^{\ast }\right(t).$(23) ) and (24(24) $\begin{array}{rl}& W_0^1(t,x_1,{\mathit{y}}_{\ast })=\frac{1}{4\delta }(1-|{\mathit{y}}_{\ast }{|}^2\left)g_1\right(t,x_1),\\ & W_0^2(t,x_1,{\mathit{y}}_{\ast })=\frac{1}{2(2\delta +\beta )}(1-|{\mathit{y}}_{\ast }{|}^2\left)\right(g_2(t,x_1)\cos \alpha \left(x_1\right)+g_3(t,x_1)\sin \alpha \left(x_1\right)),\\ & W_0^3(t,x_1,{\mathit{y}}_{\ast })=\frac{1}{2(2\delta +\beta )}(1-|{\mathit{y}}_{\ast }{|}^2\left)\right(g_3(t,x_1)\cos \alpha \left(x_1\right)-g_2(t,x_1)\sin \alpha \left(x_1\right)).\end{array}$(24) ), we obtain the following problem for $(W_1^2,W_1^3)$: (35) $\begin{array}{rl}& -\delta {\mathrm{\Delta }}_{{\mathit{y}}_{\ast }}{W}_1^2-\beta (\frac{{\mathrm{\partial }}^2{W}_1^2}{\mathrm{\partial }{y}_2^2}+\frac{{\mathrm{\partial }}^2{W}_1^3}{\mathrm{\partial }{y}_2\mathrm{\partial }{y}_3})=y_2{H}_5+y_6{H}_6\text{in}\mathrm{\Omega },\\ & -\delta {\mathrm{\Delta }}_{{\mathit{y}}_{\ast }}{W}_1^3-\beta (\frac{\mathrm{\partial }{W}_1^3}{\mathrm{\partial }{y}_3^2}+\frac{\mathrm{\partial }{W}_1^2}{\mathrm{\partial }{y}_2\mathrm{\partial }{y}_3})=y_2{H}_7+y_3{H}_8\text{in}\mathrm{\Omega },\\ & W_1^2=W_1^3=0\text{on}\mathrm{\Gamma },\end{array}$(35) where $H_5(t,x_1),\dots ,H_8(t,x_1)$ can be found in the appendix (see (A3(A3) $\begin{array}{rl}{H}_5(t,x_1)& =\frac{\delta \kappa \cos \alpha }{2\delta +\beta }(g_2(t,x_1)\cos \alpha +g_3(t,x_1)\sin \alpha )+\frac{\beta \kappa }{2\delta +\beta }{g}_2(t,x_1)-\frac{\beta }{2\delta }\frac{\mathrm{\partial }{g}_1(t,x_1)}{\mathrm{\partial }{x}_1},\\ H_6(t,x_1)& =-\frac{\delta \kappa \sin \alpha }{2\delta +\beta }(g_2\cos \alpha +g_3\sin \alpha )-\frac{4a}{\pi }{F}_0^{\ast }\left(t\right),\\ H_7(t,x_1)& =\frac{\kappa \delta \cos \alpha }{2\delta +\beta }(g_3\cos \alpha -g_2\sin \alpha )+\frac{4a}{\pi }{F}_0^{\ast }\left(t\right),\\ H_8(t,x_1)& =\frac{\delta \kappa \sin \alpha }{2\delta +\beta }(g_2(t,x_1)\sin \alpha -g_3(t,x_1)\cos \alpha \sin \alpha )+\frac{\beta \kappa }{2\delta +\beta }{g}_2(t,x_1)-\frac{\beta }{2\delta }\frac{\mathrm{\partial }{g}_1(t,x_1)}{\mathrm{\partial }{x}_1}.\end{array}$(A3) )).

The solution of the problem (35(35) $\begin{array}{rl}& -\delta {\mathrm{\Delta }}_{{\mathit{y}}_{\ast }}{W}_1^2-\beta (\frac{{\mathrm{\partial }}^2{W}_1^2}{\mathrm{\partial }{y}_2^2}+\frac{{\mathrm{\partial }}^2{W}_1^3}{\mathrm{\partial }{y}_2\mathrm{\partial }{y}_3})=y_2{H}_5+y_6{H}_6\text{in}\mathrm{\Omega },\\ & -\delta {\mathrm{\Delta }}_{{\mathit{y}}_{\ast }}{W}_1^3-\beta (\frac{\mathrm{\partial }{W}_1^3}{\mathrm{\partial }{y}_3^2}+\frac{\mathrm{\partial }{W}_1^2}{\mathrm{\partial }{y}_2\mathrm{\partial }{y}_3})=y_2{H}_7+y_3{H}_8\text{in}\mathrm{\Omega },\\ & W_1^2=W_1^3=0\text{on}\mathrm{\Gamma },\end{array}$(35) ) can be written as: (36) $\begin{array}{rl}& W_1^2(t,x_1,{\mathit{y}}_{\ast })=(1-|{\mathit{y}}_{\ast }{|}^2\left)\right(y_2{H}_9(t,x_1)+y_3{H}_{10}(t,x_1)),\\ & W_1^3(t,x_1,{\mathit{y}}_{\ast })=(1-|{\mathit{y}}_{\ast }{|}^2\left)\right(y_2{H}_{11}(t,x_1)+y_3{H}_{12}(t,x_1)),\end{array}$(36) where $H_9(t,x_1),\dots ,H_{12}(t,x_1)$ are given in the appendix (see (A4(A4) $\begin{array}{rl}& H_9(t,x_1)=\frac{\beta H_8-(4\delta +3\beta )H_5}{2[{\beta }^2-(4\delta +3\beta {)}^2]},H_{10}(t,x_1)=\frac{\beta H_7-(4\delta +\beta )H_6}{2[{\beta }^2-(4\delta +\beta {)}^2]},\\ & H_{11}(t,x_1)=\frac{1}{2\beta }{H}_6-\frac{4\delta +\beta }{\beta }\frac{\beta H_7-(4\delta +\beta )H_6}{2[{\beta }^2-(4\delta +\beta {)}^2]},H_{12}(t,x_1)=\frac{1}{2\beta }{H}_5-\frac{4\delta +\beta }{\beta }\frac{\beta H_8-(4\delta +\beta )H_5}{2[{\beta }^2-(4\delta +\beta {)}^2]}.\end{array}$(A4) )).

Looking at the obtained expressions for the velocity and microrotation first-order correctors (see (27(27) $V_1^1(t,x_1,{\mathit{y}}_{\ast })=\frac{1}{8\mu }(1-|{\mathit{y}}_{\ast }{|}^2\left)\right(y_2{H}_1(t,x_1)+y_3{H}_2(t,x_1)).$(27) ), (30(30) $\begin{array}{rl}& V_1^2(t,x_1,{\mathit{y}}_{\ast })=-\frac{a}{16\mu \delta }{g}_1(t,x_1)(1-|{\mathit{y}}_{\ast }{|}^2)y_3,V_1^3(t,x_1,{\mathit{y}}_{\ast })=\frac{a}{16\mu \delta }{g}_1(t,x_1\left)\right(1-\left|{\mathit{y}}_{\ast }{|}^2\right)y_2,\\ & P_2(t,x_1)=x_1{p}_1\left(t\right)+\frac{1}{2}{x}_1^2(\frac{p_2\left(t\right)-p_1\left(t\right)}{l}),\end{array}$(30) ), (33(33) $W_1^1(t,x_1,{\mathit{y}}_{\ast })=\frac{1}{8\mu }(1-|{\mathit{y}}_{\ast }{|}^2\left)\right(y_2{H}_3(t,x_1)+y_3{H}_4(t,x_1)).$(33) ), (36(36) $\begin{array}{rl}& W_1^2(t,x_1,{\mathit{y}}_{\ast })=(1-|{\mathit{y}}_{\ast }{|}^2\left)\right(y_2{H}_9(t,x_1)+y_3{H}_{10}(t,x_1)),\\ & W_1^3(t,x_1,{\mathit{y}}_{\ast })=(1-|{\mathit{y}}_{\ast }{|}^2\left)\right(y_2{H}_{11}(t,x_1)+y_3{H}_{12}(t,x_1)),\end{array}$(36) ) with (A1(A1) $\begin{array}{rl}{H}_1(t,x_1)& =\cos \alpha (-\frac{4\mu \kappa }{\pi }{F}_0^{\ast }-\kappa f_1-\frac{\mathrm{\partial }{f}_2}{\mathrm{\partial }{x}_1}+\tau f_3-\frac{a}{2\delta +\beta }{g}_3)+\sin \alpha (-\tau f_2-\frac{\mathrm{\partial }{f}_3}{\mathrm{\partial }{x}_1}+\frac{a}{2\delta +\beta }{g}_2),\\ H_2(t,x_1)& =\sin \alpha (\frac{4\mu \kappa }{\pi }{F}_0^{\ast }+\kappa f_1+\frac{\mathrm{\partial }{f}_2}{\mathrm{\partial }{x}_1}-\tau f_3-\frac{a}{2\delta +\beta }{g}_3)-\cos \alpha (\tau f_2+\frac{\mathrm{\partial }{f}_3}{\mathrm{\partial }{x}_1}+\frac{a}{2\delta +\beta }{g}_2).\end{array}$(A1) )–(A4(A4) $\begin{array}{rl}& H_9(t,x_1)=\frac{\beta H_8-(4\delta +3\beta )H_5}{2[{\beta }^2-(4\delta +3\beta {)}^2]},H_{10}(t,x_1)=\frac{\beta H_7-(4\delta +\beta )H_6}{2[{\beta }^2-(4\delta +\beta {)}^2]},\\ & H_{11}(t,x_1)=\frac{1}{2\beta }{H}_6-\frac{4\delta +\beta }{\beta }\frac{\beta H_7-(4\delta +\beta )H_6}{2[{\beta }^2-(4\delta +\beta {)}^2]},H_{12}(t,x_1)=\frac{1}{2\beta }{H}_5-\frac{4\delta +\beta }{\beta }\frac{\beta H_8-(4\delta +\beta )H_5}{2[{\beta }^2-(4\delta +\beta {)}^2]}.\end{array}$(A4) )), we can clearly see the effects of the curvature, torsion and micropolarity, as in the stationary case (see [14, Section 4.2]). In order to capture the effects of the time derivative as well, we need to continue the computation and construct the second-order correctors.

4.1.3. Second-order corrector

Plugging the expansions (17(17) $\begin{array}{rl}& V_{\epsilon }^i(t,\mathit{x})={\epsilon }^2{V}_0^i(t,x_1,\frac{x_2}{\epsilon },\frac{x_3}{\epsilon })+{\epsilon }^3{V}_1^i(t,x_1,\frac{x_2}{\epsilon },\frac{x_3}{\epsilon })+{\epsilon }^4{V}_2^i(t,x_1,\frac{x_2}{\epsilon },\frac{x_3}{\epsilon })+\cdots \\ & W_{\epsilon }^i(t,\mathit{x})={\epsilon }^2{W}_0^i(t,x_1,\frac{x_2}{\epsilon },\frac{x_3}{\epsilon })+{\epsilon }^3{W}_1^i(t,x_1,\frac{x_2}{\epsilon },\frac{x_3}{\epsilon })+{\epsilon }^4{W}_2^i(t,x_1,\frac{x_2}{\epsilon },\frac{x_3}{\epsilon })+\cdots \\ & P_{\epsilon }(t,\mathit{x})=P_0(t,x_1)+\epsilon P_1(t,x_1,\frac{x_2}{\epsilon },\frac{x_3}{\epsilon })+{\epsilon }^2{P}_2(t,x_1,\frac{x_2}{\epsilon },\frac{x_3}{\epsilon })+{\epsilon }^3{P}_3(t,x_1,\frac{x_2}{\epsilon },\frac{x_3}{\epsilon })+\cdots \end{array}$(17) ) into the Equation (14(14) $\begin{array}{rl}& -\mu ({\kappa }^2\cos \alpha ({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{x}}_{\ast })\frac{\mathrm{\partial }{V}_{\epsilon }^3}{\mathrm{\partial }{x}_2}-{\kappa }^2\sin \alpha ({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{x}}_{\ast })\frac{\mathrm{\partial }{V}_{\epsilon }^3}{\mathrm{\partial }{x}_3}){\mathbf{\text{a}}}^3\\ & +\frac{\mathrm{\partial }{P}_{\epsilon }}{\mathrm{\partial }{x}_1}(1+\kappa ({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{x}}_{\ast })+\cdots )\mathbf{\text{t}}+\frac{\mathrm{\partial }{P}_{\epsilon }}{\mathrm{\partial }{x}_2}{\mathbf{\text{a}}}^2+\frac{\mathrm{\partial }{P}_{\epsilon }}{\mathrm{\partial }{x}_3}{\mathbf{\text{a}}}^3\\ & =a(\frac{\mathrm{\partial }{W}_{\epsilon }^3}{\mathrm{\partial }{x}_2}-\frac{\mathrm{\partial }{W}_{\epsilon }^2}{\mathrm{\partial }{x}_3})\mathbf{\text{t}}+a(1+\kappa ({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{x}}_{\ast })+\cdots )(\frac{\mathrm{\partial }{W}_{\epsilon }^1}{\mathrm{\partial }{x}_3}-\frac{\mathrm{\partial }{W}_{\epsilon }^3}{\mathrm{\partial }{x}_1}){\mathbf{\text{a}}}^2+a(1+\kappa ({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{x}}_{\ast })+\cdots )\\ & (\frac{\mathrm{\partial }{W}_{\epsilon }^2}{\mathrm{\partial }{x}_1}-\frac{\mathrm{\partial }{W}_{\epsilon }^1}{\mathrm{\partial }{x}_2}){\mathbf{\text{a}}}^3\\ & +f_1\mathbf{\text{t}}+(f_2\cos \alpha +f_3\sin \alpha ){\mathbf{\text{a}}}^2+(f_3\cos \alpha -f_2\sin \alpha ){\mathbf{\text{a}}}^3.\end{array}$(14) )–(15(15) $\begin{array}{rl}& [\frac{1}{1-\kappa ({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{x}}_{\ast })}{]}^2(\frac{\mathrm{\partial }{V}_{\epsilon }^1}{\mathrm{\partial }{x}_1}-\kappa (V_{\epsilon }^2\cos \alpha -V_{\epsilon }^3\sin \alpha )+\left({\kappa }^{\prime }\right({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{x}}_{\ast })+\kappa \tau ({\mathbf{\text{e}}}_{\alpha }^{\perp }\cdot {\mathit{x}}_{\ast }\left)\right)V_{\epsilon }^1\\ & +\kappa ({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{x}}_{\ast })\left({\kappa }^{\prime }\right({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{x}}_{\ast })+\kappa \tau ({\mathbf{\text{e}}}_{\alpha }^{\perp }\cdot {\mathit{x}}_{\ast }\left)\right)V_{\epsilon }^1+{\kappa }^2({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{x}}_{\ast })\cos \alpha V_{\epsilon }^2-{\kappa }^2({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{x}}_{\ast })\sin \alpha V_{\epsilon }^3)\\ & +\frac{\mathrm{\partial }{V}_{\epsilon }^2}{\mathrm{\partial }{x}_2}+\frac{\mathrm{\partial }{V}_{\epsilon }^3}{\mathrm{\partial }{x}_3}=\frac{\mathrm{\partial }{V}_{\epsilon }^1}{\mathrm{\partial }{x}_1}-\kappa (V_{\epsilon }^2\cos \alpha -V_{\epsilon }^3\sin \alpha )+\left({\kappa }^{\prime }\right({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{x}}_{\ast })+\kappa \tau ({\mathbf{\text{e}}}_{\alpha }^{\perp }\cdot {\mathit{x}}_{\ast }\left)\right)V_{\epsilon }^1\\ & +\kappa ({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{x}}_{\ast })\left({\kappa }^{\prime }\right({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{x}}_{\ast })+\kappa \tau ({\mathbf{\text{e}}}_{\alpha }^{\perp }\cdot {\mathit{x}}_{\ast }\left)\right)V_{\epsilon }^1+{\kappa }^2({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{x}}_{\ast })\cos \alpha V_{\epsilon }^2-{\kappa }^2({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{x}}_{\ast })\sin \alpha V_{\epsilon }^3\\ & +\frac{\mathrm{\partial }{V}_{\epsilon }^2}{\mathrm{\partial }{x}_2}+\frac{\mathrm{\partial }{V}_{\epsilon }^3}{\mathrm{\partial }{x}_3}+2\kappa ({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{x}}_{\ast })(\frac{\mathrm{\partial }{V}_{\epsilon }^1}{\mathrm{\partial }{x}_1}-\kappa (V_{\epsilon }^2\cos \alpha -V_{\epsilon }^3\sin \alpha )+\left({\kappa }^{\prime }\right({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{x}}_{\ast })+\kappa \tau ({\mathbf{\text{e}}}_{\alpha }^{\perp }\cdot {\mathit{x}}_{\ast }\left)\right)V_{\epsilon }^1\\ & +{\kappa }^2({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{x}}_{\ast })\cos \alpha V_{\epsilon }^2-{\kappa }^2({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{x}}_{\ast })\sin \alpha V_{\epsilon }^3)+3{\kappa }^2({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{x}}_{\ast }{)}^2(\frac{\mathrm{\partial }{V}_{\epsilon }^1}{\mathrm{\partial }{x}_1}-\kappa (V_{\epsilon }^2\cos \alpha -V_{\epsilon }^3\sin \alpha ))\\ & +4{\kappa }^3({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{x}}_{\ast }{)}^3\frac{\mathrm{\partial }{V}_{\epsilon }^1}{\mathrm{\partial }{x}_1}+\mathcal{O}({\epsilon }^3)=0.\end{array}$(15) ) and collecting the terms of order ${\epsilon }^2$, we get the equation for the first component of the velocity second-order corrector $V_2^1$: (37) $\begin{array}{rl}\frac{\mathrm{\partial }{V}_0^1}{\mathrm{\partial }t}& -\mu (\frac{{\mathrm{\partial }}^2{V}_0^1}{\mathrm{\partial }{x}_1^2}+{\mathrm{\Delta }}_{{\mathit{y}}_{\ast }}{V}_2^1+\kappa \cos \alpha \frac{\mathrm{\partial }{V}_1^1}{\mathrm{\partial }{y}_2}-\kappa \sin \alpha \frac{\mathrm{\partial }{V}_1^1}{\mathrm{\partial }{y}_3}-{\kappa }^2{V}_0^1+\kappa ({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast })\\ & ({\mathrm{\Delta }}_{{\mathit{y}}_{\ast }}{V}_1^1-\kappa \cos \alpha \frac{\mathrm{\partial }{V}_0^1}{\mathrm{\partial }{y}_2}+\kappa \sin \alpha \frac{\mathrm{\partial }{V}_0^1}{\mathrm{\partial }{y}_3}))\\ & -\mu {\kappa }^2({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast }{)}^2{\mathrm{\Delta }}_{{\mathit{y}}_{\ast }}{V}_0^1+{\kappa }^2({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast }{)}^2\frac{\mathrm{\partial }{P}_0}{\mathrm{\partial }{x}_1}+\kappa ({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast })\frac{\mathrm{\partial }{P}_1}{\mathrm{\partial }{x}_1}+\frac{\mathrm{\partial }{P}_2}{\mathrm{\partial }{x}_1}=a(\frac{\mathrm{\partial }{W}_1^3}{\mathrm{\partial }{y}_2}-\frac{\mathrm{\partial }{W}_1^2}{\mathrm{\partial }{y}_3}).\end{array}$(37) Inserting the expressions for $V_0^1,V_1^1,P_0,P_1,P_2,W_1^2$ and $W_1^3$ given by (19(19) $\begin{array}{rl}& V_0^1(t,x_1,{\mathit{y}}_{\ast })=\frac{1}{4\mu }(1-|{\mathit{y}}_{\ast }{|}^2\left)(f_1\right(t,x_1)-\frac{\mathrm{\partial }{P}_0(x_1,t)}{\mathrm{\partial }{x}_1}),V_0^2=V_0^3=0,\\ & P_1(t,x_1,{\mathit{y}}_{\ast })=f_2(t,x_1)\left({\mathbf{\text{e}}}_{\alpha }\right(x_1)\cdot {\mathit{y}}_{\ast })+f_3(t,x_1)\left({\mathbf{\text{e}}}_{\alpha }^{\perp }\right(x_1)\cdot {\mathit{y}}_{\ast }),\end{array}$(19) ) (22(22) $P_0(t,x_1)=-\frac{8\mu }{\pi }{F}_0^{\ast }\left(t\right)x_1+{\int }_0^{x_1}{f}_1(t,\xi )d\xi +p_0\left(t\right),$(22) ), (23(23) $V_0^1(t,{\mathit{y}}_{\ast })=\frac{2}{\pi }(1-|{\mathit{y}}_{\ast }{|}^2\left)F_0^{\ast }\right(t).$(23) ), (27(27) $V_1^1(t,x_1,{\mathit{y}}_{\ast })=\frac{1}{8\mu }(1-|{\mathit{y}}_{\ast }{|}^2\left)\right(y_2{H}_1(t,x_1)+y_3{H}_2(t,x_1)).$(27) ), (30(30) $\begin{array}{rl}& V_1^2(t,x_1,{\mathit{y}}_{\ast })=-\frac{a}{16\mu \delta }{g}_1(t,x_1)(1-|{\mathit{y}}_{\ast }{|}^2)y_3,V_1^3(t,x_1,{\mathit{y}}_{\ast })=\frac{a}{16\mu \delta }{g}_1(t,x_1\left)\right(1-\left|{\mathit{y}}_{\ast }{|}^2\right)y_2,\\ & P_2(t,x_1)=x_1{p}_1\left(t\right)+\frac{1}{2}{x}_1^2(\frac{p_2\left(t\right)-p_1\left(t\right)}{l}),\end{array}$(30) ) and (36(36) $\begin{array}{rl}& W_1^2(t,x_1,{\mathit{y}}_{\ast })=(1-|{\mathit{y}}_{\ast }{|}^2\left)\right(y_2{H}_9(t,x_1)+y_3{H}_{10}(t,x_1)),\\ & W_1^3(t,x_1,{\mathit{y}}_{\ast })=(1-|{\mathit{y}}_{\ast }{|}^2\left)\right(y_2{H}_{11}(t,x_1)+y_3{H}_{12}(t,x_1)),\end{array}$(36) ) into (37(37) $\begin{array}{rl}\frac{\mathrm{\partial }{V}_0^1}{\mathrm{\partial }t}& -\mu (\frac{{\mathrm{\partial }}^2{V}_0^1}{\mathrm{\partial }{x}_1^2}+{\mathrm{\Delta }}_{{\mathit{y}}_{\ast }}{V}_2^1+\kappa \cos \alpha \frac{\mathrm{\partial }{V}_1^1}{\mathrm{\partial }{y}_2}-\kappa \sin \alpha \frac{\mathrm{\partial }{V}_1^1}{\mathrm{\partial }{y}_3}-{\kappa }^2{V}_0^1+\kappa ({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast })\\ & ({\mathrm{\Delta }}_{{\mathit{y}}_{\ast }}{V}_1^1-\kappa \cos \alpha \frac{\mathrm{\partial }{V}_0^1}{\mathrm{\partial }{y}_2}+\kappa \sin \alpha \frac{\mathrm{\partial }{V}_0^1}{\mathrm{\partial }{y}_3}))\\ & -\mu {\kappa }^2({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast }{)}^2{\mathrm{\Delta }}_{{\mathit{y}}_{\ast }}{V}_0^1+{\kappa }^2({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast }{)}^2\frac{\mathrm{\partial }{P}_0}{\mathrm{\partial }{x}_1}+\kappa ({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast })\frac{\mathrm{\partial }{P}_1}{\mathrm{\partial }{x}_1}+\frac{\mathrm{\partial }{P}_2}{\mathrm{\partial }{x}_1}=a(\frac{\mathrm{\partial }{W}_1^3}{\mathrm{\partial }{y}_2}-\frac{\mathrm{\partial }{W}_1^2}{\mathrm{\partial }{y}_3}).\end{array}$(37) ) yields (38) $\begin{array}{rl}& {\mathrm{\Delta }}_{{\mathit{y}}_{\ast }}{V}_2^1=A_1{y}_2^2+A_2{y}_2{y}_3+A_3{y}_3^2+A_4\text{in}\mathrm{\Omega },\\ & V_2^1=0\text{on}\mathrm{\Gamma },\end{array}$(38) where the functions $A_1(t,x_1),\dots ,A_4(t,x_1)$ are provided in the appendix (see (A5(A5) $\begin{array}{rl}& A_1(t,x_1)\\ & =-\frac{2}{\pi \mu }\frac{\mathrm{d}}{\mathrm{d}t}{F}_0^{\ast }+\frac{3\kappa \cos \alpha }{8\mu }{H}_1-\frac{\kappa \sin \alpha }{8\mu }{H}_2-\frac{2{\kappa }^2}{\pi }{F}_0^{\ast }+\frac{\kappa }{\mu }\cos \alpha H_1+\frac{4{\kappa }^2{\cos }^2\alpha }{\pi }{F}_0^{\ast }\\ & -\frac{{\kappa }^2}{\mu }{\cos }^2\alpha (\frac{8\mu }{\pi }{F}_0^{\ast }-f_1)+\frac{\kappa }{\mu }({\cos }^2\alpha \frac{\mathrm{\partial }{f}_2}{\mathrm{\partial }{x}_1}-\sin \alpha \cos \alpha f_2+\cos \alpha \sin \alpha \frac{\mathrm{\partial }{f}_3}{\mathrm{\partial }{x}_1}+{\cos }^2\alpha f_3)\\ & -\frac{a}{\mu }(-3H_{11}+H_{10}),\\ & A_2(t,x_1)\\ & =\frac{\kappa \cos \alpha }{4\mu }{H}_2-\frac{\kappa \sin \alpha }{4\mu }{H}_1+\frac{\kappa \cos \alpha }{\mu }{H}_2-\frac{\kappa \sin \alpha }{\mu }{H}_1-\frac{8{\kappa }^2\cos \alpha \sin \alpha }{\pi }{F}_0^{\ast }+\frac{2{\kappa }^2\sin \alpha \cos \alpha }{\mu }(\frac{8\mu }{\pi }{F}_0^{\ast }+f_1)\\ & -\frac{\kappa }{\mu }(-2\sin \alpha \cos \alpha \frac{\mathrm{\partial }{f}_2}{\mathrm{\partial }{x}_1}-({\cos }^2\alpha -{\sin }^2\alpha )f_2+({\cos }^2\alpha -{\sin }^2\alpha )\frac{\mathrm{\partial }{f}_3}{\mathrm{\partial }{x}_1}\\ & -2f_3\sin \alpha \cos \alpha )+\frac{a}{\mu }(2H_{12}-2H_9),\\ & A_3(t,x_1)\\ & =-\frac{2}{\pi \mu }\frac{\mathrm{d}}{\mathrm{d}t}{F}_0^{\ast }\left(t\right)+\frac{\kappa \cos \alpha }{8\mu }{H}_1-\frac{3\kappa \sin \alpha }{8\mu }{H}_2-\frac{2{\kappa }^2}{\pi }{F}_0^{\ast }\left(t\right)-\frac{\kappa \sin \alpha }{\mu }{H}_2+\frac{4{\kappa }^2{\sin }^2\alpha }{\pi }{F}_0^{\ast }\left(t\right)\\ & -\frac{{\kappa }^2{\sin }^2\alpha }{\mu }(\frac{8}{\pi }{F}_0^{\ast }-f_1)+\frac{\kappa }{\mu }(\frac{\mathrm{\partial }{f}_2}{\mathrm{\partial }{x}_1}{\sin }^2\alpha -\sin \alpha \cos \alpha f_2+\frac{\mathrm{\partial }{f}_3}{\mathrm{\partial }{x}_1}\sin \alpha \cos \alpha +f_3{\sin }^2\alpha )\\ & -\frac{a}{\mu }(-H_{11}+3H_{10}),\\ & A_4(t,x_1)\\ & =\frac{2}{\mu \pi }\frac{\mathrm{d}}{\mathrm{d}t}{F}_0^{\ast }\left(t\right)-\frac{\kappa \cos \alpha }{8\mu }{H}_1+\frac{\kappa \sin \alpha }{8\mu }{H}_2+\frac{2{\kappa }^2}{\pi }{F}_0^{\ast }\left(t\right)+\frac{1}{\mu }{p}_1\left(t\right)+\frac{1}{\mu }{x}_1(\frac{p_2\left(t\right)-p_1\left(t\right)}{l})-\frac{a}{\mu }(H_{11}-H_{10}).\end{array}$(A5) )).

The solution of (38(38) $\begin{array}{rl}& {\mathrm{\Delta }}_{{\mathit{y}}_{\ast }}{V}_2^1=A_1{y}_2^2+A_2{y}_2{y}_3+A_3{y}_3^2+A_4\text{in}\mathrm{\Omega },\\ & V_2^1=0\text{on}\mathrm{\Gamma },\end{array}$(38) ) is given in the form $V_2^1(t,x_1,{\mathit{y}}_{\ast })=\left(\right|{\mathit{y}}_{\ast }{|}^2-1)[B_1{y}_2^2+B_2{y}_2{y}_3+B_3{y}_3^2+B_4],$ where $B_1(t,x_1)=\frac{7A_1-A_3}{96},B_2(t,x_1)=\frac{1}{12}{A}_2,B_3(t,x_1)=\frac{7A_3-A_1}{96},B_4(t,x_1)=\frac{1}{4}{A}_4+\frac{1}{32}(A_1+A_3).$ It is important to notice that the effects of the time derivative appear in the second-order corrector as the derivative of the flux function $F_0^{\ast }\left(t\right)$ (see (A5(A5) $\begin{array}{rl}& A_1(t,x_1)\\ & =-\frac{2}{\pi \mu }\frac{\mathrm{d}}{\mathrm{d}t}{F}_0^{\ast }+\frac{3\kappa \cos \alpha }{8\mu }{H}_1-\frac{\kappa \sin \alpha }{8\mu }{H}_2-\frac{2{\kappa }^2}{\pi }{F}_0^{\ast }+\frac{\kappa }{\mu }\cos \alpha H_1+\frac{4{\kappa }^2{\cos }^2\alpha }{\pi }{F}_0^{\ast }\\ & -\frac{{\kappa }^2}{\mu }{\cos }^2\alpha (\frac{8\mu }{\pi }{F}_0^{\ast }-f_1)+\frac{\kappa }{\mu }({\cos }^2\alpha \frac{\mathrm{\partial }{f}_2}{\mathrm{\partial }{x}_1}-\sin \alpha \cos \alpha f_2+\cos \alpha \sin \alpha \frac{\mathrm{\partial }{f}_3}{\mathrm{\partial }{x}_1}+{\cos }^2\alpha f_3)\\ & -\frac{a}{\mu }(-3H_{11}+H_{10}),\\ & A_2(t,x_1)\\ & =\frac{\kappa \cos \alpha }{4\mu }{H}_2-\frac{\kappa \sin \alpha }{4\mu }{H}_1+\frac{\kappa \cos \alpha }{\mu }{H}_2-\frac{\kappa \sin \alpha }{\mu }{H}_1-\frac{8{\kappa }^2\cos \alpha \sin \alpha }{\pi }{F}_0^{\ast }+\frac{2{\kappa }^2\sin \alpha \cos \alpha }{\mu }(\frac{8\mu }{\pi }{F}_0^{\ast }+f_1)\\ & -\frac{\kappa }{\mu }(-2\sin \alpha \cos \alpha \frac{\mathrm{\partial }{f}_2}{\mathrm{\partial }{x}_1}-({\cos }^2\alpha -{\sin }^2\alpha )f_2+({\cos }^2\alpha -{\sin }^2\alpha )\frac{\mathrm{\partial }{f}_3}{\mathrm{\partial }{x}_1}\\ & -2f_3\sin \alpha \cos \alpha )+\frac{a}{\mu }(2H_{12}-2H_9),\\ & A_3(t,x_1)\\ & =-\frac{2}{\pi \mu }\frac{\mathrm{d}}{\mathrm{d}t}{F}_0^{\ast }\left(t\right)+\frac{\kappa \cos \alpha }{8\mu }{H}_1-\frac{3\kappa \sin \alpha }{8\mu }{H}_2-\frac{2{\kappa }^2}{\pi }{F}_0^{\ast }\left(t\right)-\frac{\kappa \sin \alpha }{\mu }{H}_2+\frac{4{\kappa }^2{\sin }^2\alpha }{\pi }{F}_0^{\ast }\left(t\right)\\ & -\frac{{\kappa }^2{\sin }^2\alpha }{\mu }(\frac{8}{\pi }{F}_0^{\ast }-f_1)+\frac{\kappa }{\mu }(\frac{\mathrm{\partial }{f}_2}{\mathrm{\partial }{x}_1}{\sin }^2\alpha -\sin \alpha \cos \alpha f_2+\frac{\mathrm{\partial }{f}_3}{\mathrm{\partial }{x}_1}\sin \alpha \cos \alpha +f_3{\sin }^2\alpha )\\ & -\frac{a}{\mu }(-H_{11}+3H_{10}),\\ & A_4(t,x_1)\\ & =\frac{2}{\mu \pi }\frac{\mathrm{d}}{\mathrm{d}t}{F}_0^{\ast }\left(t\right)-\frac{\kappa \cos \alpha }{8\mu }{H}_1+\frac{\kappa \sin \alpha }{8\mu }{H}_2+\frac{2{\kappa }^2}{\pi }{F}_0^{\ast }\left(t\right)+\frac{1}{\mu }{p}_1\left(t\right)+\frac{1}{\mu }{x}_1(\frac{p_2\left(t\right)-p_1\left(t\right)}{l})-\frac{a}{\mu }(H_{11}-H_{10}).\end{array}$(A5) )).

In a similar way, we now obtain the equations for the second and third component of the velocity second-order corrector ${\mathbf{\text{V}}}_2^{\ast }=(V_2^2,V_2^3)$: (39) $\begin{array}{rl}& -\mu ({\mathrm{\Delta }}_{{\mathit{y}}_{\ast }}{V}_2^2-\kappa \cos \alpha \frac{\mathrm{\partial }{V}_1^2}{\mathrm{\partial }{y}_2}+\kappa \sin \alpha \frac{\mathrm{\partial }{V}_1^2}{\mathrm{\partial }{y}_3}+(2\kappa \frac{\mathrm{\partial }{V}_0^1}{\mathrm{\partial }{x}_1}+{\kappa }^{\prime }{V}_0^1)\cos \alpha +\kappa \tau V_0^1\sin \alpha )+\frac{\mathrm{\partial }{P}_3}{\mathrm{\partial }{y}_2}\\ & =a(\frac{\mathrm{\partial }{W}_1^1}{\mathrm{\partial }{y}_3}+\kappa ({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast })\frac{\mathrm{\partial }{W}_0^1}{\mathrm{\partial }{y}_3}-\frac{\mathrm{\partial }{W}_0^3}{\mathrm{\partial }{x}_1})\text{in}\mathrm{\Omega },\\ & -\mu ({\mathrm{\Delta }}_{{\mathit{y}}_{\ast }}{V}_2^3+\kappa \sin \alpha \frac{\mathrm{\partial }{V}_1^3}{\mathrm{\partial }{y}_3}-\kappa \cos \alpha \frac{\mathrm{\partial }{V}_1^3}{\mathrm{\partial }{y}_2}+\kappa \tau V_0^1\cos \alpha -(2\kappa \frac{\mathrm{\partial }{V}_0^1}{\mathrm{\partial }{x}_1}+{\kappa }^{\prime }{V}_0^1)\sin \alpha )+\frac{\mathrm{\partial }{P}_3}{\mathrm{\partial }{y}_3}\\ & =a(\frac{\mathrm{\partial }{W}_0^2}{\mathrm{\partial }{x}_1}-\frac{\mathrm{\partial }{W}_1^1}{\mathrm{\partial }{y}_2}-\kappa ({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast })\frac{\mathrm{\partial }{W}_0^1}{\mathrm{\partial }{y}_2})\text{in}\mathrm{\Omega },\\ & \frac{\mathrm{\partial }{V}_2^2}{\mathrm{\partial }{y}_2}+\frac{\mathrm{\partial }{V}_2^3}{\mathrm{\partial }{y}_3}=-\frac{\mathrm{\partial }{V}_1^1}{\mathrm{\partial }{x}_1}+\kappa (V_1^2\cos \alpha -V_1^3\sin \alpha )-\left({\kappa }^{\prime }\right({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast })+\kappa \tau ({\mathbf{\text{e}}}_{\alpha }^{\perp }\cdot {\mathit{y}}_{\ast }\left)\right)V_0^1\ne 0\text{in}\mathrm{\Omega },\\ & V_2^2=V_2^3=0\text{on}\mathrm{\Gamma }.\end{array}$(39) The system of equations (39(39) $\begin{array}{rl}& -\mu ({\mathrm{\Delta }}_{{\mathit{y}}_{\ast }}{V}_2^2-\kappa \cos \alpha \frac{\mathrm{\partial }{V}_1^2}{\mathrm{\partial }{y}_2}+\kappa \sin \alpha \frac{\mathrm{\partial }{V}_1^2}{\mathrm{\partial }{y}_3}+(2\kappa \frac{\mathrm{\partial }{V}_0^1}{\mathrm{\partial }{x}_1}+{\kappa }^{\prime }{V}_0^1)\cos \alpha +\kappa \tau V_0^1\sin \alpha )+\frac{\mathrm{\partial }{P}_3}{\mathrm{\partial }{y}_2}\\ & =a(\frac{\mathrm{\partial }{W}_1^1}{\mathrm{\partial }{y}_3}+\kappa ({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast })\frac{\mathrm{\partial }{W}_0^1}{\mathrm{\partial }{y}_3}-\frac{\mathrm{\partial }{W}_0^3}{\mathrm{\partial }{x}_1})\text{in}\mathrm{\Omega },\\ & -\mu ({\mathrm{\Delta }}_{{\mathit{y}}_{\ast }}{V}_2^3+\kappa \sin \alpha \frac{\mathrm{\partial }{V}_1^3}{\mathrm{\partial }{y}_3}-\kappa \cos \alpha \frac{\mathrm{\partial }{V}_1^3}{\mathrm{\partial }{y}_2}+\kappa \tau V_0^1\cos \alpha -(2\kappa \frac{\mathrm{\partial }{V}_0^1}{\mathrm{\partial }{x}_1}+{\kappa }^{\prime }{V}_0^1)\sin \alpha )+\frac{\mathrm{\partial }{P}_3}{\mathrm{\partial }{y}_3}\\ & =a(\frac{\mathrm{\partial }{W}_0^2}{\mathrm{\partial }{x}_1}-\frac{\mathrm{\partial }{W}_1^1}{\mathrm{\partial }{y}_2}-\kappa ({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast })\frac{\mathrm{\partial }{W}_0^1}{\mathrm{\partial }{y}_2})\text{in}\mathrm{\Omega },\\ & \frac{\mathrm{\partial }{V}_2^2}{\mathrm{\partial }{y}_2}+\frac{\mathrm{\partial }{V}_2^3}{\mathrm{\partial }{y}_3}=-\frac{\mathrm{\partial }{V}_1^1}{\mathrm{\partial }{x}_1}+\kappa (V_1^2\cos \alpha -V_1^3\sin \alpha )-\left({\kappa }^{\prime }\right({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast })+\kappa \tau ({\mathbf{\text{e}}}_{\alpha }^{\perp }\cdot {\mathit{y}}_{\ast }\left)\right)V_0^1\ne 0\text{in}\mathrm{\Omega },\\ & V_2^2=V_2^3=0\text{on}\mathrm{\Gamma }.\end{array}$(39) ) admits a unique solution since ${\int }_B(-\frac{\mathrm{\partial }{V}_1^1}{\mathrm{\partial }{x}_1}+\kappa (V_1^2\cos \alpha -V_1^3\sin \alpha )-\left({\kappa }^{\prime }\right({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast })+\kappa \tau ({\mathbf{\text{e}}}_{\alpha }^{\perp }\cdot {\mathit{y}}_{\ast }\left)\right)V_0^1)dB=0$ (see [25]). The explicit solution is constructed below.

Inserting the expressions for $V_0^1,W_0^1,W_0^2,W_0^3,V_1^1{V}_1^2,V_1^3,W_1^1$ given by (23(23) $V_0^1(t,{\mathit{y}}_{\ast })=\frac{2}{\pi }(1-|{\mathit{y}}_{\ast }{|}^2\left)F_0^{\ast }\right(t).$(23) ), (24(24) $\begin{array}{rl}& W_0^1(t,x_1,{\mathit{y}}_{\ast })=\frac{1}{4\delta }(1-|{\mathit{y}}_{\ast }{|}^2\left)g_1\right(t,x_1),\\ & W_0^2(t,x_1,{\mathit{y}}_{\ast })=\frac{1}{2(2\delta +\beta )}(1-|{\mathit{y}}_{\ast }{|}^2\left)\right(g_2(t,x_1)\cos \alpha \left(x_1\right)+g_3(t,x_1)\sin \alpha \left(x_1\right)),\\ & W_0^3(t,x_1,{\mathit{y}}_{\ast })=\frac{1}{2(2\delta +\beta )}(1-|{\mathit{y}}_{\ast }{|}^2\left)\right(g_3(t,x_1)\cos \alpha \left(x_1\right)-g_2(t,x_1)\sin \alpha \left(x_1\right)).\end{array}$(24) ), (27(27) $V_1^1(t,x_1,{\mathit{y}}_{\ast })=\frac{1}{8\mu }(1-|{\mathit{y}}_{\ast }{|}^2\left)\right(y_2{H}_1(t,x_1)+y_3{H}_2(t,x_1)).$(27) ), (30(30) $\begin{array}{rl}& V_1^2(t,x_1,{\mathit{y}}_{\ast })=-\frac{a}{16\mu \delta }{g}_1(t,x_1)(1-|{\mathit{y}}_{\ast }{|}^2)y_3,V_1^3(t,x_1,{\mathit{y}}_{\ast })=\frac{a}{16\mu \delta }{g}_1(t,x_1\left)\right(1-\left|{\mathit{y}}_{\ast }{|}^2\right)y_2,\\ & P_2(t,x_1)=x_1{p}_1\left(t\right)+\frac{1}{2}{x}_1^2(\frac{p_2\left(t\right)-p_1\left(t\right)}{l}),\end{array}$(30) ) and (33(33) $W_1^1(t,x_1,{\mathit{y}}_{\ast })=\frac{1}{8\mu }(1-|{\mathit{y}}_{\ast }{|}^2\left)\right(y_2{H}_3(t,x_1)+y_3{H}_4(t,x_1)).$(33) ) into (39(39) $\begin{array}{rl}& -\mu ({\mathrm{\Delta }}_{{\mathit{y}}_{\ast }}{V}_2^2-\kappa \cos \alpha \frac{\mathrm{\partial }{V}_1^2}{\mathrm{\partial }{y}_2}+\kappa \sin \alpha \frac{\mathrm{\partial }{V}_1^2}{\mathrm{\partial }{y}_3}+(2\kappa \frac{\mathrm{\partial }{V}_0^1}{\mathrm{\partial }{x}_1}+{\kappa }^{\prime }{V}_0^1)\cos \alpha +\kappa \tau V_0^1\sin \alpha )+\frac{\mathrm{\partial }{P}_3}{\mathrm{\partial }{y}_2}\\ & =a(\frac{\mathrm{\partial }{W}_1^1}{\mathrm{\partial }{y}_3}+\kappa ({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast })\frac{\mathrm{\partial }{W}_0^1}{\mathrm{\partial }{y}_3}-\frac{\mathrm{\partial }{W}_0^3}{\mathrm{\partial }{x}_1})\text{in}\mathrm{\Omega },\\ & -\mu ({\mathrm{\Delta }}_{{\mathit{y}}_{\ast }}{V}_2^3+\kappa \sin \alpha \frac{\mathrm{\partial }{V}_1^3}{\mathrm{\partial }{y}_3}-\kappa \cos \alpha \frac{\mathrm{\partial }{V}_1^3}{\mathrm{\partial }{y}_2}+\kappa \tau V_0^1\cos \alpha -(2\kappa \frac{\mathrm{\partial }{V}_0^1}{\mathrm{\partial }{x}_1}+{\kappa }^{\prime }{V}_0^1)\sin \alpha )+\frac{\mathrm{\partial }{P}_3}{\mathrm{\partial }{y}_3}\\ & =a(\frac{\mathrm{\partial }{W}_0^2}{\mathrm{\partial }{x}_1}-\frac{\mathrm{\partial }{W}_1^1}{\mathrm{\partial }{y}_2}-\kappa ({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast })\frac{\mathrm{\partial }{W}_0^1}{\mathrm{\partial }{y}_2})\text{in}\mathrm{\Omega },\\ & \frac{\mathrm{\partial }{V}_2^2}{\mathrm{\partial }{y}_2}+\frac{\mathrm{\partial }{V}_2^3}{\mathrm{\partial }{y}_3}=-\frac{\mathrm{\partial }{V}_1^1}{\mathrm{\partial }{x}_1}+\kappa (V_1^2\cos \alpha -V_1^3\sin \alpha )-\left({\kappa }^{\prime }\right({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast })+\kappa \tau ({\mathbf{\text{e}}}_{\alpha }^{\perp }\cdot {\mathit{y}}_{\ast }\left)\right)V_0^1\ne 0\text{in}\mathrm{\Omega },\\ & V_2^2=V_2^3=0\text{on}\mathrm{\Gamma }.\end{array}$(39) ), we get: (40) $\begin{array}{rl}& -\mu {\mathrm{\Delta }}_{{\mathit{y}}_{\ast }}{V}_2^2+\frac{\mathrm{\partial }{P}_3}{\mathrm{\partial }{y}_2}=A_5{y}_2^2+A_6{y}_2{y}_3+A_7{y}_3^2+A_8,\\ & -\mu {\mathrm{\Delta }}_{{\mathit{y}}_{\ast }}{V}_2^3+\frac{\mathrm{\partial }{P}_3}{\mathrm{\partial }{y}_3}=A_9{y}_2^2+A_{10}{y}_2{y}_3+A_{11}{y}_3^2+A_{12},\\ & \frac{\mathrm{\partial }{V}_2^2}{\mathrm{\partial }{y}_2}+\frac{\mathrm{\partial }{V}_2^3}{\mathrm{\partial }{y}_3}=A_{13}{y}_2^3+A_{14}{y}_3^3+A_{13}{y}_2{y}_3^2+A_{14}{y}_2^2{y}_3-A_{13}{y}_2-A_{14}{y}_3,\\ & V_2^2=V_2^3=0\text{on}\mathrm{\Gamma },\end{array}$(40) where $A_5(t,x_1),\dots ,A_{14}(t,x_1)$ are given in the appendix (see (A6(A6) $\begin{array}{rl}{A}_{10}(t,x_1)& =-\frac{a{g}_1\mu \kappa \sin \alpha }{8\mu \delta }+2a{H}_4-\frac{a{g}_1\kappa \sin \alpha }{2\delta },\\ A_{11}(t,x_1)& =\frac{a\kappa \cos \alpha g_1}{16\mu \delta }-\frac{2\mu (\kappa \tau \cos \alpha -{\kappa }^{\prime }\sin \alpha )F_0^{\ast }}{\pi }\\ & -\frac{a}{2(2\delta +\beta )}(\frac{\mathrm{\partial }{g}_2}{\mathrm{\partial }{x}_1}\cos \alpha +g_2\tau \sin \alpha +\frac{\mathrm{\partial }{g}_3}{\mathrm{\partial }{x}_1}\sin \alpha -g_3\tau \sin \alpha )+a{H}_3,\\ A_{12}(t,x_1)& =-\frac{a{g}_1\mu \kappa \cos \alpha }{16\mu \delta }+\frac{2\mu (\kappa \tau \cos \alpha -{\kappa }^{\prime }\sin \alpha )F_0^{\ast }}{\pi }\\ & +\frac{a}{2(2\delta +\beta )}(\frac{\mathrm{\partial }{g}_2}{\mathrm{\partial }{x}_1}\cos \alpha +g_2\tau \sin \alpha +\frac{\mathrm{\partial }{g}_3}{\mathrm{\partial }{x}_1}\sin \alpha -g_3\tau \sin \alpha )-a{H}_3,\\ A_{13}(t,x_1)& =\frac{1}{8\mu }\frac{\mathrm{\partial }{H}_1}{\mathrm{\partial }{x}_1}+\frac{a\kappa g_1\sin \alpha }{16\mu \delta }+\frac{2{\kappa }^{\prime }\cos \alpha +2\kappa \tau \sin \alpha }{\pi }{F}_0^{\ast },\\ A_{14}(t,x_1)& =\frac{1}{8\mu }\frac{\mathrm{\partial }{H}_2}{\mathrm{\partial }{x}_1}+\frac{a\kappa g_1\cos \alpha }{16\mu \delta }+\frac{-2{\kappa }^{\prime }\sin \alpha +2\kappa \tau \cos \alpha }{\pi }{F}_0^{\ast }.\end{array}$(A6) )).

We seek the solution of (40(40) $\begin{array}{rl}& -\mu {\mathrm{\Delta }}_{{\mathit{y}}_{\ast }}{V}_2^2+\frac{\mathrm{\partial }{P}_3}{\mathrm{\partial }{y}_2}=A_5{y}_2^2+A_6{y}_2{y}_3+A_7{y}_3^2+A_8,\\ & -\mu {\mathrm{\Delta }}_{{\mathit{y}}_{\ast }}{V}_2^3+\frac{\mathrm{\partial }{P}_3}{\mathrm{\partial }{y}_3}=A_9{y}_2^2+A_{10}{y}_2{y}_3+A_{11}{y}_3^2+A_{12},\\ & \frac{\mathrm{\partial }{V}_2^2}{\mathrm{\partial }{y}_2}+\frac{\mathrm{\partial }{V}_2^3}{\mathrm{\partial }{y}_3}=A_{13}{y}_2^3+A_{14}{y}_3^3+A_{13}{y}_2{y}_3^2+A_{14}{y}_2^2{y}_3-A_{13}{y}_2-A_{14}{y}_3,\\ & V_2^2=V_2^3=0\text{on}\mathrm{\Gamma },\end{array}$(40) ) in the following form: (41) $\begin{array}{rl}& V_2^2(t,x_1,{\mathit{y}}_{\ast })=(1-|{\mathit{y}}_{\ast }{|}^2\left)\right(B_5{y}_2^2+B_6{y}_2{y}_3+B_7{y}_2^3+B_8),\\ & V_2^3(t,x_1,{\mathit{y}}_{\ast })=(1-|{\mathit{y}}_{\ast }{|}^2\left)\right(B_9{y}_2^2+B_{10}{y}_2{y}_3+B_{11}{y}_2^3+B_{12}),\\ & P_3(t,x_1,{\mathit{y}}_{\ast })=M_1{y}_2^3+M_2{y}_3^3+M_3{y}_2{y}_3^2+M_4{y}_2^2{y}_3+M_5{y}_2+M_6{y}_3.\end{array}$(41) Plugging (41(41) $\begin{array}{rl}& V_2^2(t,x_1,{\mathit{y}}_{\ast })=(1-|{\mathit{y}}_{\ast }{|}^2\left)\right(B_5{y}_2^2+B_6{y}_2{y}_3+B_7{y}_2^3+B_8),\\ & V_2^3(t,x_1,{\mathit{y}}_{\ast })=(1-|{\mathit{y}}_{\ast }{|}^2\left)\right(B_9{y}_2^2+B_{10}{y}_2{y}_3+B_{11}{y}_2^3+B_{12}),\\ & P_3(t,x_1,{\mathit{y}}_{\ast })=M_1{y}_2^3+M_2{y}_3^3+M_3{y}_2{y}_3^2+M_4{y}_2^2{y}_3+M_5{y}_2+M_6{y}_3.\end{array}$(41) ) into the system (40(40) $\begin{array}{rl}& -\mu {\mathrm{\Delta }}_{{\mathit{y}}_{\ast }}{V}_2^2+\frac{\mathrm{\partial }{P}_3}{\mathrm{\partial }{y}_2}=A_5{y}_2^2+A_6{y}_2{y}_3+A_7{y}_3^2+A_8,\\ & -\mu {\mathrm{\Delta }}_{{\mathit{y}}_{\ast }}{V}_2^3+\frac{\mathrm{\partial }{P}_3}{\mathrm{\partial }{y}_3}=A_9{y}_2^2+A_{10}{y}_2{y}_3+A_{11}{y}_3^2+A_{12},\\ & \frac{\mathrm{\partial }{V}_2^2}{\mathrm{\partial }{y}_2}+\frac{\mathrm{\partial }{V}_2^3}{\mathrm{\partial }{y}_3}=A_{13}{y}_2^3+A_{14}{y}_3^3+A_{13}{y}_2{y}_3^2+A_{14}{y}_2^2{y}_3-A_{13}{y}_2-A_{14}{y}_3,\\ & V_2^2=V_2^3=0\text{on}\mathrm{\Gamma },\end{array}$(40) ), we obtain a system of equations relating $B_5(t,x_1),\dots ,B_{12}(t,x_1)$ and $M_1(t,x_1),\dots ,M_6(t,x_1)$ with $A_5(t,x_1),\dots ,A_{14}(t,x_1)$. Solving this system, we deduce $B_5(t,x_1),\dots ,B_{12}(t,x_1)$ and $M_1(t,x_1),\dots ,M_6(t,x_1)$ (see (A7(A7) $\begin{array}{rl}{M}_1(t,x_1)& =\mu A_{13}+\frac{1}{3}{A}_5-\frac{1}{12}{A}_7+\frac{1}{24}{A}_{10},M_2(t,x_1)=\frac{144}{147}\mu A_{14}+\frac{4}{147}{A}_6-\frac{8}{147}{A}_9+\frac{8}{147}{A}_{11},\\ M_3(t,x_1)& =\mu A_{13}+\frac{1}{4}{A}_7-\frac{3}{8}{A}_{10},M_4(t,x_1)=\frac{96}{105}\mu A_{14}-\frac{8}{21}{A}_6+\frac{5}{21}{A}_9+\frac{17}{105}{A}_{11},\\ M_5(t,x_1)& =-\frac{4}{3}\mu A_{13}+\frac{1}{6}{A}_7-A_8-\frac{5}{24}{A}_{10},M_6(t,x_1)=-\frac{998}{735}\mu A_{14}-\frac{191}{882}{A}_6\\ & +\frac{79}{441}{A}_9+\frac{5491}{311640}{A}_{11}+A_{12},\end{array}$(A7) )–(A8(A8) $\begin{array}{rl}{B}_5(t,x_1)& =-\frac{21}{96\mu }{M}_1+\frac{1}{96\mu }{M}_3+\frac{7}{96\mu }{A}_5-\frac{1}{96\mu }{A}_7,B_6(t,x_1)=-\frac{1}{6\mu }{M}_4+\frac{1}{12\mu }{A}_6,\\ B_7(t,x_1)& =\frac{3}{96\mu }{M}_1-\frac{7}{96\mu }{M}_3-\frac{1}{96\mu }{A}_5+\frac{7}{96\mu }{A}_7,\\ B_8(t,x_1)& =-\frac{9}{96\mu }{M}_1-\frac{3}{96\mu }{M}_3+\frac{3}{96\mu }{A}_5+\frac{3}{96\mu }{A}_7-\frac{1}{4\mu }{M}_5+\frac{1}{4\mu }{A}_8,\\ B_9(t,x_1)& =\frac{3}{96\mu }{M}_2-\frac{7}{96\mu }{M}_4+\frac{7}{96\mu }{A}_9-\frac{1}{96\mu }{A}_{11},B_{10}(t,x_1)=-\frac{1}{6\mu }{M}_3+\frac{1}{12\mu }{A}_{10},\\ B_{11}(t,x_1)& =-\frac{21}{96\mu }{M}_2+\frac{1}{96\mu }{M}_4+\frac{7}{96\mu }{A}_{11}-\frac{1}{96\mu }{A}_9,\\ B_{12}(t,x_1)& =-\frac{9}{96\mu }{M}_2-\frac{3}{96\mu }{M}_4+\frac{3}{96\mu }{A}_9+\frac{6}{96\mu }{A}_{11}-\frac{1}{4\mu }{M}_6+\frac{1}{4\mu }{A}_{12}.\end{array}$(A8) ) in the appendix).

In this way, we have obtained the explicit expressions for the velocity second-order corrector $(V_2^2,V_2^3)$ and pressure third-order corrector $P_3$ and we now compute the microrotation second-order corrector in the following.

Substituting (17(17) $\begin{array}{rl}& V_{\epsilon }^i(t,\mathit{x})={\epsilon }^2{V}_0^i(t,x_1,\frac{x_2}{\epsilon },\frac{x_3}{\epsilon })+{\epsilon }^3{V}_1^i(t,x_1,\frac{x_2}{\epsilon },\frac{x_3}{\epsilon })+{\epsilon }^4{V}_2^i(t,x_1,\frac{x_2}{\epsilon },\frac{x_3}{\epsilon })+\cdots \\ & W_{\epsilon }^i(t,\mathit{x})={\epsilon }^2{W}_0^i(t,x_1,\frac{x_2}{\epsilon },\frac{x_3}{\epsilon })+{\epsilon }^3{W}_1^i(t,x_1,\frac{x_2}{\epsilon },\frac{x_3}{\epsilon })+{\epsilon }^4{W}_2^i(t,x_1,\frac{x_2}{\epsilon },\frac{x_3}{\epsilon })+\cdots \\ & P_{\epsilon }(t,\mathit{x})=P_0(t,x_1)+\epsilon P_1(t,x_1,\frac{x_2}{\epsilon },\frac{x_3}{\epsilon })+{\epsilon }^2{P}_2(t,x_1,\frac{x_2}{\epsilon },\frac{x_3}{\epsilon })+{\epsilon }^3{P}_3(t,x_1,\frac{x_2}{\epsilon },\frac{x_3}{\epsilon })+\cdots \end{array}$(17) ) into (16(16) $\begin{array}{rl}& -\beta (\frac{{\mathrm{\partial }}^2{W}_{\epsilon }^1}{\mathrm{\partial }{x}_1\mathrm{\partial }{x}_3}-\kappa (\frac{\mathrm{\partial }{W}_{\epsilon }^2}{\mathrm{\partial }{x}_3}\cos \alpha -\frac{\mathrm{\partial }{W}_{\epsilon }^3}{\mathrm{\partial }{x}_3}\sin \alpha )+\kappa \tau W_{\epsilon }^1\cos \alpha \\ & -({\kappa }^{\prime }{W}_{\epsilon }^1+{\kappa }^2\cos \alpha W_{\epsilon }^2-{\kappa }^2\sin \alpha W_{\epsilon }^3)\sin \alpha ){\mathbf{\text{a}}}^3\\ & -\beta (2\kappa (\frac{\mathrm{\partial }{W}_{\epsilon }^1}{\mathrm{\partial }{x}_1}-\kappa (W_{\epsilon }^2\cos \alpha -W_{\epsilon }^3\sin \alpha ))\sin \alpha \\ & +{\kappa }^{\prime }({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{x}}_{\ast })\frac{\mathrm{\partial }{W}_{\epsilon }^1}{\mathrm{\partial }{x}_3}+\kappa \tau ({\mathbf{\text{e}}}_{\alpha }^{\perp }\cdot {\mathit{x}}_{\ast })\frac{\mathrm{\partial }{W}_{\epsilon }^1}{\mathrm{\partial }{x}_3}+{\kappa }^2({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{x}}_{\ast })\cos \alpha \frac{\mathrm{\partial }{W}_{\epsilon }^2}{\mathrm{\partial }{x}_3}){\mathbf{\text{a}}}^3\\ & -\beta (-{\kappa }^2({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{x}}_{\ast })\frac{\mathrm{\partial }{W}_{\epsilon }^3}{\mathrm{\partial }{x}_3}\sin \alpha +\frac{{\mathrm{\partial }}^2{W}_{\epsilon }^2}{\mathrm{\partial }{x}_2\mathrm{\partial }{x}_3}+\frac{{\mathrm{\partial }}^2{W}_{\epsilon }^3}{\mathrm{\partial }{x}_3^2}+2\kappa ({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{x}}_{\ast })\\ & (\frac{{\mathrm{\partial }}^2{W}_{\epsilon }^1}{\mathrm{\partial }{x}_1\mathrm{\partial }{x}_3}-\kappa (\frac{\mathrm{\partial }{W}_{\epsilon }^2}{\mathrm{\partial }{x}_3}\cos \alpha -\frac{\mathrm{\partial }{W}_{\epsilon }^3}{\mathrm{\partial }{x}_3}\sin \alpha ))]{\mathbf{\text{a}}}^3\\ & +2a(1+\kappa ({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{x}}_{\ast })+\cdots )W_{\epsilon }^1\mathbf{\text{t}}+2a{W}_{\epsilon }^2{\mathbf{\text{a}}}^2+2a{W}_{\epsilon }^3{\mathbf{\text{a}}}^3\\ & =a(\frac{\mathrm{\partial }{V}_{\epsilon }^3}{\mathrm{\partial }{x}_2}-\frac{\mathrm{\partial }{V}_{\epsilon }^2}{\mathrm{\partial }{x}_3})\mathbf{\text{t}}+a((1+\kappa ({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{x}}_{\ast })+\cdots )(\frac{\mathrm{\partial }{V}_{\epsilon }^1}{\mathrm{\partial }{x}_3}-\frac{\mathrm{\partial }{V}_{\epsilon }^3}{\mathrm{\partial }{x}_1})){\mathbf{\text{a}}}^2\\ & +a((1+\kappa ({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{x}}_{\ast })+\cdots )(\frac{\mathrm{\partial }{V}_{\epsilon }^2}{\mathrm{\partial }{x}_1}-\frac{\mathrm{\partial }{V}_{\epsilon }^1}{\mathrm{\partial }{x}_2})){\mathbf{\text{a}}}^3+g_1\mathbf{\text{t}}+(g_2\cos \alpha +g_3\sin \alpha ){\mathbf{\text{a}}}^2\\ & +(g_3\cos \alpha -g_2\sin \alpha ){\mathbf{\text{a}}}^3.\end{array}$(16) ) and collecting the ${\epsilon }^2$ terms, we obtain the following problem for the first component of the microrotation second-order corrector $W_2^1$: (42) $\begin{array}{rl}\frac{\mathrm{\partial }{W}_0^1}{\mathrm{\partial }t}& -\delta (\frac{{\mathrm{\partial }}^2{W}_0^1}{\mathrm{\partial }{x}_1^2}+{\mathrm{\Delta }}_{{\mathit{y}}_{\ast }}{W}_2^1+\kappa \cos \alpha (\frac{\mathrm{\partial }{W}_1^1}{\mathrm{\partial }{y}_2}-\frac{\mathrm{\partial }{W}_0^2}{\mathrm{\partial }{x}_1})+\kappa \sin \alpha (\frac{\mathrm{\partial }{W}_0^3}{\mathrm{\partial }{x}_1}-\frac{\mathrm{\partial }{W}_1^1}{\mathrm{\partial }{y}_3}))\\ & -\delta \frac{\mathrm{\partial }}{\mathrm{\partial }{x}_1}(W_0^2\kappa \cos \alpha -W_0^3\kappa \sin \alpha -{\kappa }^{\prime }(W_0^2\cos \alpha -W_0^3\sin \alpha ))+\delta {\kappa }^2{W}_0^1\\ & -\delta \kappa ({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast })({\mathrm{\Delta }}_{{\mathit{y}}_{\ast }}{W}_1^1-\kappa \cos \alpha \frac{\mathrm{\partial }{W}_0^1}{\mathrm{\partial }{y}_2}+\kappa \sin \alpha \frac{\mathrm{\partial }{W}_0^1}{\mathrm{\partial }{y}_3})-\delta {\kappa }^2({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast }{)}^2{\mathrm{\Delta }}_{{\mathit{y}}_{\ast }}{W}_0^1\\ & -\beta (\frac{{\mathrm{\partial }}^2{W}_0^1}{\mathrm{\partial }{x}_1^2}-(\kappa \cos \alpha {)}^{\prime }{W}_0^2-\kappa \cos \alpha \frac{\mathrm{\partial }{W}_0^2}{\mathrm{\partial }{x}_1}+(\kappa \sin \alpha {)}^{\prime }{W}_0^3\\ & +\kappa \sin \alpha \frac{\mathrm{\partial }{W}_0^3}{\mathrm{\partial }{x}_1}+\frac{{\mathrm{\partial }}^2{W}_1^2}{\mathrm{\partial }{x}_1\mathrm{\partial }{y}_2}+\frac{{\mathrm{\partial }}^2{W}_1^3}{\mathrm{\partial }{x}_1\mathrm{\partial }{y}_3})\\ & -\beta (\kappa ({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast })\frac{{\mathrm{\partial }}^2{W}_0^2}{\mathrm{\partial }{x}_1\mathrm{\partial }{y}_2}+\kappa ({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast })\frac{\mathrm{\partial }{W}_0^3}{\mathrm{\partial }{x}_1\mathrm{\partial }{y}_3})+2a{W}_0^1=a(\frac{\mathrm{\partial }{V}_1^3}{\mathrm{\partial }{y}_2}-\frac{\mathrm{\partial }{V}_1^2}{\mathrm{\partial }{y}_3})\text{in}\mathrm{\Omega },\\ W_2^1& =0\text{on}\mathrm{\Gamma }.\end{array}$(42) Inserting the expressions for $W_0^1,W_0^2,W_0^3,V_1^2,V_1^3,W_1^1,W_1^2,W_1^3$ given by (24(24) $\begin{array}{rl}& W_0^1(t,x_1,{\mathit{y}}_{\ast })=\frac{1}{4\delta }(1-|{\mathit{y}}_{\ast }{|}^2\left)g_1\right(t,x_1),\\ & W_0^2(t,x_1,{\mathit{y}}_{\ast })=\frac{1}{2(2\delta +\beta )}(1-|{\mathit{y}}_{\ast }{|}^2\left)\right(g_2(t,x_1)\cos \alpha \left(x_1\right)+g_3(t,x_1)\sin \alpha \left(x_1\right)),\\ & W_0^3(t,x_1,{\mathit{y}}_{\ast })=\frac{1}{2(2\delta +\beta )}(1-|{\mathit{y}}_{\ast }{|}^2\left)\right(g_3(t,x_1)\cos \alpha \left(x_1\right)-g_2(t,x_1)\sin \alpha \left(x_1\right)).\end{array}$(24) ), (30(30) $\begin{array}{rl}& V_1^2(t,x_1,{\mathit{y}}_{\ast })=-\frac{a}{16\mu \delta }{g}_1(t,x_1)(1-|{\mathit{y}}_{\ast }{|}^2)y_3,V_1^3(t,x_1,{\mathit{y}}_{\ast })=\frac{a}{16\mu \delta }{g}_1(t,x_1\left)\right(1-\left|{\mathit{y}}_{\ast }{|}^2\right)y_2,\\ & P_2(t,x_1)=x_1{p}_1\left(t\right)+\frac{1}{2}{x}_1^2(\frac{p_2\left(t\right)-p_1\left(t\right)}{l}),\end{array}$(30) ), (33(33) $W_1^1(t,x_1,{\mathit{y}}_{\ast })=\frac{1}{8\mu }(1-|{\mathit{y}}_{\ast }{|}^2\left)\right(y_2{H}_3(t,x_1)+y_3{H}_4(t,x_1)).$(33) ) and (36(36) $\begin{array}{rl}& W_1^2(t,x_1,{\mathit{y}}_{\ast })=(1-|{\mathit{y}}_{\ast }{|}^2\left)\right(y_2{H}_9(t,x_1)+y_3{H}_{10}(t,x_1)),\\ & W_1^3(t,x_1,{\mathit{y}}_{\ast })=(1-|{\mathit{y}}_{\ast }{|}^2\left)\right(y_2{H}_{11}(t,x_1)+y_3{H}_{12}(t,x_1)),\end{array}$(36) ) into (42(42) $\begin{array}{rl}\frac{\mathrm{\partial }{W}_0^1}{\mathrm{\partial }t}& -\delta (\frac{{\mathrm{\partial }}^2{W}_0^1}{\mathrm{\partial }{x}_1^2}+{\mathrm{\Delta }}_{{\mathit{y}}_{\ast }}{W}_2^1+\kappa \cos \alpha (\frac{\mathrm{\partial }{W}_1^1}{\mathrm{\partial }{y}_2}-\frac{\mathrm{\partial }{W}_0^2}{\mathrm{\partial }{x}_1})+\kappa \sin \alpha (\frac{\mathrm{\partial }{W}_0^3}{\mathrm{\partial }{x}_1}-\frac{\mathrm{\partial }{W}_1^1}{\mathrm{\partial }{y}_3}))\\ & -\delta \frac{\mathrm{\partial }}{\mathrm{\partial }{x}_1}(W_0^2\kappa \cos \alpha -W_0^3\kappa \sin \alpha -{\kappa }^{\prime }(W_0^2\cos \alpha -W_0^3\sin \alpha ))+\delta {\kappa }^2{W}_0^1\\ & -\delta \kappa ({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast })({\mathrm{\Delta }}_{{\mathit{y}}_{\ast }}{W}_1^1-\kappa \cos \alpha \frac{\mathrm{\partial }{W}_0^1}{\mathrm{\partial }{y}_2}+\kappa \sin \alpha \frac{\mathrm{\partial }{W}_0^1}{\mathrm{\partial }{y}_3})-\delta {\kappa }^2({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast }{)}^2{\mathrm{\Delta }}_{{\mathit{y}}_{\ast }}{W}_0^1\\ & -\beta (\frac{{\mathrm{\partial }}^2{W}_0^1}{\mathrm{\partial }{x}_1^2}-(\kappa \cos \alpha {)}^{\prime }{W}_0^2-\kappa \cos \alpha \frac{\mathrm{\partial }{W}_0^2}{\mathrm{\partial }{x}_1}+(\kappa \sin \alpha {)}^{\prime }{W}_0^3\\ & +\kappa \sin \alpha \frac{\mathrm{\partial }{W}_0^3}{\mathrm{\partial }{x}_1}+\frac{{\mathrm{\partial }}^2{W}_1^2}{\mathrm{\partial }{x}_1\mathrm{\partial }{y}_2}+\frac{{\mathrm{\partial }}^2{W}_1^3}{\mathrm{\partial }{x}_1\mathrm{\partial }{y}_3})\\ & -\beta (\kappa ({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast })\frac{{\mathrm{\partial }}^2{W}_0^2}{\mathrm{\partial }{x}_1\mathrm{\partial }{y}_2}+\kappa ({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast })\frac{\mathrm{\partial }{W}_0^3}{\mathrm{\partial }{x}_1\mathrm{\partial }{y}_3})+2a{W}_0^1=a(\frac{\mathrm{\partial }{V}_1^3}{\mathrm{\partial }{y}_2}-\frac{\mathrm{\partial }{V}_1^2}{\mathrm{\partial }{y}_3})\text{in}\mathrm{\Omega },\\ W_2^1& =0\text{on}\mathrm{\Gamma }.\end{array}$(42) ) yields (43) $\begin{array}{rl}& {\mathrm{\Delta }}_{{\mathit{y}}_{\ast }}{W}_2^1=C_1{y}_2^2+C_2{y}_2{y}_3+C_3{y}_3^2+C_4\text{in}\mathrm{\Omega },\\ & W_2^1=0\text{on}\mathrm{\Gamma },\end{array}$(43) where the functions $C_1(t,x_1),\dots ,C_4(t,x_1)$ are given in the appendix (see (A9(A9) $\begin{array}{rl}{C}_4(t,x_1)=& \frac{1}{4{\delta }^2}\frac{\mathrm{\partial }{g}_1}{\mathrm{\partial }t}+\frac{1}{4\delta }\frac{{\mathrm{\partial }}^2{g}_1}{\mathrm{\partial }{x}_1^2}-\frac{\kappa \cos \alpha }{8\mu }{H}_3+\frac{\kappa \cos \alpha }{2\delta (2\delta +\beta )}(\frac{\mathrm{\partial }{g}_2}{\mathrm{\partial }{x}_1}\cos \alpha +\tau g_2\sin \alpha +\frac{\mathrm{\partial }{g}_3}{\mathrm{\partial }{x}_1}\sin \alpha -\tau g_3\sin \alpha )\\ & -\frac{\kappa \sin \alpha }{2(2\delta +\beta )}(\frac{\mathrm{\partial }{g}_3}{\mathrm{\partial }{x}_1}\cos \alpha +\tau g_3\sin \alpha -\frac{\mathrm{\partial }{g}_2}{\mathrm{\partial }{x}_1}\sin \alpha +\tau g_2\cos \alpha )+\frac{\kappa \sin \alpha }{8\mu }{H}_4\\ & -\frac{1}{2(2\delta +\beta )}\frac{\mathrm{\partial }}{\mathrm{\partial }{x}_1}((\kappa -{\kappa }^{\prime })\cos \alpha (g_2\cos \alpha +g_3\sin \alpha )-(\kappa -{\kappa }^{\prime })\sin \alpha (g_3\cos \alpha -g_2\sin \alpha ))\\ & +\frac{{\kappa }^2}{4\delta }{g}_1-\frac{\beta }{4{\delta }^2}\frac{{\mathrm{\partial }}^2{g}_1}{\mathrm{\partial }{x}_1^2}-\frac{\beta }{2\delta (2\delta +\beta )}(-(\kappa \cos \alpha {)}^{\prime }(g_2\cos \alpha +g_3\sin \alpha )-\kappa \cos \alpha (g_2\cos \alpha +g_3\sin \alpha {)}^{\prime })\\ & +(\kappa \sin \alpha {)}^{\prime }(g_3\cos \alpha -g_2\sin \alpha )+\kappa \sin \alpha (g_3\cos \alpha -g_2\sin \alpha {)}^{\prime })\\ & +\frac{\beta }{\delta }\frac{\mathrm{\partial }{H}_9}{\mathrm{\partial }{x}_1}-\frac{\beta }{\delta }\frac{\mathrm{\partial }{H}_{12}}{\mathrm{\partial }{x}_1}-\frac{a^2}{8\mu {\delta }^2}{g}_1+\frac{a}{2{\delta }^2}{g}_1.\end{array}$(A9) )).

We find the solution of the problem (43(43) $\begin{array}{rl}& {\mathrm{\Delta }}_{{\mathit{y}}_{\ast }}{W}_2^1=C_1{y}_2^2+C_2{y}_2{y}_3+C_3{y}_3^2+C_4\text{in}\mathrm{\Omega },\\ & W_2^1=0\text{on}\mathrm{\Gamma },\end{array}$(43) ) in the form (44) $W_2^1(t,x_1,{\mathit{y}}_{\ast })=\left(\right|{\mathit{y}}_{\ast }{|}^2-1\left)\right(D_1{y}_2^2+D_2{y}_2{y}_3+D_3{y}_3^2+D_4),$(44) where (45) $D_1=\frac{7C_1-C_3}{96},D_2=\frac{1}{12}{C}_2,D_3=\frac{7C_3-C_1}{96},D_4=\frac{1}{4}{C}_4+\frac{1}{32}(C_1+C_3).$(45)

Plugging expansions (17(17) $\begin{array}{rl}& V_{\epsilon }^i(t,\mathit{x})={\epsilon }^2{V}_0^i(t,x_1,\frac{x_2}{\epsilon },\frac{x_3}{\epsilon })+{\epsilon }^3{V}_1^i(t,x_1,\frac{x_2}{\epsilon },\frac{x_3}{\epsilon })+{\epsilon }^4{V}_2^i(t,x_1,\frac{x_2}{\epsilon },\frac{x_3}{\epsilon })+\cdots \\ & W_{\epsilon }^i(t,\mathit{x})={\epsilon }^2{W}_0^i(t,x_1,\frac{x_2}{\epsilon },\frac{x_3}{\epsilon })+{\epsilon }^3{W}_1^i(t,x_1,\frac{x_2}{\epsilon },\frac{x_3}{\epsilon })+{\epsilon }^4{W}_2^i(t,x_1,\frac{x_2}{\epsilon },\frac{x_3}{\epsilon })+\cdots \\ & P_{\epsilon }(t,\mathit{x})=P_0(t,x_1)+\epsilon P_1(t,x_1,\frac{x_2}{\epsilon },\frac{x_3}{\epsilon })+{\epsilon }^2{P}_2(t,x_1,\frac{x_2}{\epsilon },\frac{x_3}{\epsilon })+{\epsilon }^3{P}_3(t,x_1,\frac{x_2}{\epsilon },\frac{x_3}{\epsilon })+\cdots \end{array}$(17) ) into equation (16(16) $\begin{array}{rl}& -\beta (\frac{{\mathrm{\partial }}^2{W}_{\epsilon }^1}{\mathrm{\partial }{x}_1\mathrm{\partial }{x}_3}-\kappa (\frac{\mathrm{\partial }{W}_{\epsilon }^2}{\mathrm{\partial }{x}_3}\cos \alpha -\frac{\mathrm{\partial }{W}_{\epsilon }^3}{\mathrm{\partial }{x}_3}\sin \alpha )+\kappa \tau W_{\epsilon }^1\cos \alpha \\ & -({\kappa }^{\prime }{W}_{\epsilon }^1+{\kappa }^2\cos \alpha W_{\epsilon }^2-{\kappa }^2\sin \alpha W_{\epsilon }^3)\sin \alpha ){\mathbf{\text{a}}}^3\\ & -\beta (2\kappa (\frac{\mathrm{\partial }{W}_{\epsilon }^1}{\mathrm{\partial }{x}_1}-\kappa (W_{\epsilon }^2\cos \alpha -W_{\epsilon }^3\sin \alpha ))\sin \alpha \\ & +{\kappa }^{\prime }({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{x}}_{\ast })\frac{\mathrm{\partial }{W}_{\epsilon }^1}{\mathrm{\partial }{x}_3}+\kappa \tau ({\mathbf{\text{e}}}_{\alpha }^{\perp }\cdot {\mathit{x}}_{\ast })\frac{\mathrm{\partial }{W}_{\epsilon }^1}{\mathrm{\partial }{x}_3}+{\kappa }^2({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{x}}_{\ast })\cos \alpha \frac{\mathrm{\partial }{W}_{\epsilon }^2}{\mathrm{\partial }{x}_3}){\mathbf{\text{a}}}^3\\ & -\beta (-{\kappa }^2({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{x}}_{\ast })\frac{\mathrm{\partial }{W}_{\epsilon }^3}{\mathrm{\partial }{x}_3}\sin \alpha +\frac{{\mathrm{\partial }}^2{W}_{\epsilon }^2}{\mathrm{\partial }{x}_2\mathrm{\partial }{x}_3}+\frac{{\mathrm{\partial }}^2{W}_{\epsilon }^3}{\mathrm{\partial }{x}_3^2}+2\kappa ({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{x}}_{\ast })\\ & (\frac{{\mathrm{\partial }}^2{W}_{\epsilon }^1}{\mathrm{\partial }{x}_1\mathrm{\partial }{x}_3}-\kappa (\frac{\mathrm{\partial }{W}_{\epsilon }^2}{\mathrm{\partial }{x}_3}\cos \alpha -\frac{\mathrm{\partial }{W}_{\epsilon }^3}{\mathrm{\partial }{x}_3}\sin \alpha ))]{\mathbf{\text{a}}}^3\\ & +2a(1+\kappa ({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{x}}_{\ast })+\cdots )W_{\epsilon }^1\mathbf{\text{t}}+2a{W}_{\epsilon }^2{\mathbf{\text{a}}}^2+2a{W}_{\epsilon }^3{\mathbf{\text{a}}}^3\\ & =a(\frac{\mathrm{\partial }{V}_{\epsilon }^3}{\mathrm{\partial }{x}_2}-\frac{\mathrm{\partial }{V}_{\epsilon }^2}{\mathrm{\partial }{x}_3})\mathbf{\text{t}}+a((1+\kappa ({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{x}}_{\ast })+\cdots )(\frac{\mathrm{\partial }{V}_{\epsilon }^1}{\mathrm{\partial }{x}_3}-\frac{\mathrm{\partial }{V}_{\epsilon }^3}{\mathrm{\partial }{x}_1})){\mathbf{\text{a}}}^2\\ & +a((1+\kappa ({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{x}}_{\ast })+\cdots )(\frac{\mathrm{\partial }{V}_{\epsilon }^2}{\mathrm{\partial }{x}_1}-\frac{\mathrm{\partial }{V}_{\epsilon }^1}{\mathrm{\partial }{x}_2})){\mathbf{\text{a}}}^3+g_1\mathbf{\text{t}}+(g_2\cos \alpha +g_3\sin \alpha ){\mathbf{\text{a}}}^2\\ & +(g_3\cos \alpha -g_2\sin \alpha ){\mathbf{\text{a}}}^3.\end{array}$(16) ) and collecting the ${\epsilon }^2$ terms, we finally obtain the problem for the second and third component of the microrotation second-order corector $(W_2^2,W_2^3)$ as: $\begin{array}{rl}\frac{\mathrm{\partial }{W}_0^2}{\mathrm{\partial }t}& -\delta (\frac{{\mathrm{\partial }}^2{W}_0^2}{\mathrm{\partial }{x}_1^2}+{\mathrm{\Delta }}_{{\mathit{y}}_{\ast }}{W}_2^2-\kappa \cos \alpha \frac{\mathrm{\partial }{W}_1^2}{\mathrm{\partial }{y}_2}+\kappa \sin \alpha \frac{\mathrm{\partial }{W}_1^2}{\mathrm{\partial }{y}_3}+2\kappa ({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast })\\ & (-\kappa \cos \alpha \frac{\mathrm{\partial }{W}_0^2}{\mathrm{\partial }{y}_2}+\kappa \sin \alpha \frac{\mathrm{\partial }{W}_0^2}{\mathrm{\partial }{y}_3}))\\ & -\delta ((2\kappa \frac{\mathrm{\partial }{W}_0^1}{\mathrm{\partial }{x}_1}+{\kappa }^{\prime }{W}_0^1-{\kappa }^2{W}_0^2)\cos \alpha +\kappa \tau W_0^1\sin \alpha +{\kappa }^2\cos \alpha ({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast })\\ & \frac{\mathrm{\partial }{W}_0^2}{\mathrm{\partial }{y}_2}-{\kappa }^2\sin \alpha ({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast })\frac{\mathrm{\partial }{W}_0^2}{\mathrm{\partial }{y}_3})\\ & -\beta (\frac{{\mathrm{\partial }}^2{W}_1^1}{\mathrm{\partial }{x}_1\mathrm{\partial }{y}_2}-\kappa (\frac{\mathrm{\partial }{W}_1^2}{\mathrm{\partial }{y}_2}\cos \alpha -\frac{\mathrm{\partial }{W}_1^3}{\mathrm{\partial }{y}_2}\sin \alpha )+({\kappa }^{\prime }{W}_0^1+{\kappa }^2\cos \alpha W_0^2-{\kappa }^2\sin \alpha W_0^3)\cos \alpha )\\ & -2\beta \kappa (\frac{\mathrm{\partial }{W}_0^1}{\mathrm{\partial }{x}_1}-\kappa (W_0^2\cos \alpha -W_0^3\sin \alpha ))\cos \alpha \\ & -\beta (\kappa \tau W_0^1\sin \alpha +{\kappa }^{\prime }({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast })\frac{\mathrm{\partial }{W}_0^1}{\mathrm{\partial }{y}_2}+\kappa \tau ({\mathbf{\text{e}}}_{\alpha }^{\perp }\cdot {\mathit{y}}_{\ast })\frac{\mathrm{\partial }{W}_0^1}{\mathrm{\partial }{y}_2})\\ & -\beta {\kappa }^2({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast })(\frac{\mathrm{\partial }{W}_0^2}{\mathrm{\partial }{y}_2}\cos \alpha -\frac{\mathrm{\partial }{W}_0^3}{\mathrm{\partial }{y}_3}\sin \alpha )-\beta (\frac{{\mathrm{\partial }}^2{W}_2^2}{\mathrm{\partial }{y}_2^2}+\frac{{\mathrm{\partial }}^2{W}_2^3}{\mathrm{\partial }{y}_2\mathrm{\partial }{y}_3})\\ & -2\beta \kappa ({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast })(\frac{{\mathrm{\partial }}^2{W}_0^1}{\mathrm{\partial }{x}_1\mathrm{\partial }{y}_2}-\kappa (\frac{\mathrm{\partial }{W}_0^2}{\mathrm{\partial }{y}_2}\cos \alpha -\frac{\mathrm{\partial }{W}_0^3}{\mathrm{\partial }{y}_2}\sin \alpha ))+2a{W}_0^2\\ & =a(\frac{\mathrm{\partial }{V}_1^1}{\mathrm{\partial }{y}_3}+\kappa ({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast })\frac{\mathrm{\partial }{V}_0^1}{\mathrm{\partial }{y}_3}-\frac{\mathrm{\partial }{V}_0^3}{\mathrm{\partial }{x}_1})\text{in}\mathrm{\Omega },\\ \frac{\mathrm{\partial }{W}_0^3}{\mathrm{\partial }t}& -\delta (\frac{{\mathrm{\partial }}^2{W}_0^3}{\mathrm{\partial }{x}_1^2}+{\mathrm{\Delta }}_{{\mathit{y}}_{\ast }}{W}_2^3+\kappa \sin \alpha \frac{\mathrm{\partial }{W}_1^3}{\mathrm{\partial }{y}_3}-\kappa \cos \alpha \frac{\mathrm{\partial }{W}_1^3}{\mathrm{\partial }{y}_2}+2\kappa ({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast })\\ & (\kappa \sin \alpha \frac{\mathrm{\partial }{W}_0^3}{\mathrm{\partial }{y}_3}-\kappa \cos \alpha \frac{\mathrm{\partial }{W}_0^3}{\mathrm{\partial }{y}_2}))\end{array}$ (46) $\begin{array}{rl}& -\delta (\kappa \tau W_0^1\cos \alpha -(2\kappa \frac{\mathrm{\partial }{W}_0^1}{\mathrm{\partial }{x}_1}+{\kappa }^{\prime }{W}_0^1-{\kappa }^2{W}_0^2)\sin \alpha +{\kappa }^2\cos \alpha ({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast })\frac{\mathrm{\partial }{W}_0^3}{\mathrm{\partial }{y}_2}\\ & -{\kappa }^2\sin \alpha ({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast })\frac{\mathrm{\partial }{W}_0^3}{\mathrm{\partial }{y}_3})\\ & -\beta (\frac{{\mathrm{\partial }}^2{W}_1^1}{\mathrm{\partial }{x}_1\mathrm{\partial }{y}_3}-\kappa (\frac{\mathrm{\partial }{W}_1^2}{\mathrm{\partial }{y}_3}\cos \alpha -\frac{\mathrm{\partial }{W}_1^3}{\mathrm{\partial }{y}_3}\sin \alpha )+\kappa \tau W_0^1\cos \alpha \\ & -({\kappa }^{\prime }{W}_0^1+{\kappa }^2\cos \alpha W_0^2-{\kappa }^2\sin \alpha W_0^3))\\ & -2\beta \kappa (\frac{\mathrm{\partial }{W}_0^1}{\mathrm{\partial }{x}_1}-\kappa (W_0^2\cos \alpha -W_0^3\sin \alpha ))\sin \alpha -\beta ({\kappa }^{\prime }({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast })\frac{\mathrm{\partial }{W}_0^1}{\mathrm{\partial }{y}_3}+\kappa \tau ({\mathbf{\text{e}}}_{\alpha }^{\perp }\cdot {\mathit{y}}_{\ast })\frac{\mathrm{\partial }{W}_0^1}{\mathrm{\partial }{y}_3})\\ & -\beta {\kappa }^2({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast })(\cos \alpha \frac{\mathrm{\partial }{W}_0^2}{\mathrm{\partial }{y}_3}-\sin \alpha \frac{\mathrm{\partial }{W}_0^3}{\mathrm{\partial }{y}_3})-\beta (\frac{{\mathrm{\partial }}^2{W}_2^3}{\mathrm{\partial }{y}_3^2}+\frac{{\mathrm{\partial }}^2{W}_2^2}{\mathrm{\partial }{y}_2\mathrm{\partial }{y}_3})\\ & -2\beta \kappa ({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast })(\frac{{\mathrm{\partial }}^2{W}_0^1}{\mathrm{\partial }{x}_1\mathrm{\partial }{y}_3}-\kappa (\frac{\mathrm{\partial }{W}_0^2}{\mathrm{\partial }{y}_3}\cos \alpha -\frac{\mathrm{\partial }{W}_0^3}{\mathrm{\partial }{y}_3}\sin \alpha ))+2a{W}_0^3\\ & =a(\frac{\mathrm{\partial }{V}_0^2}{\mathrm{\partial }{x}_1}-\frac{\mathrm{\partial }{V}_1^1}{\mathrm{\partial }{y}_2}-\kappa ({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast })\frac{\mathrm{\partial }{V}_0^1}{\mathrm{\partial }{y}_2})\text{in}\mathrm{\Omega },\\ W_2^2& =W_2^3=0\text{on}\mathrm{\Gamma }.\end{array}$(46) Plugging the expressions for $V_0^1,V_0^2,V_0^3,V_1^1,W_0^1,W_0^2,W_0^3,W_1^1,W_1^2,W_1^3$ given by (19(19) $\begin{array}{rl}& V_0^1(t,x_1,{\mathit{y}}_{\ast })=\frac{1}{4\mu }(1-|{\mathit{y}}_{\ast }{|}^2\left)(f_1\right(t,x_1)-\frac{\mathrm{\partial }{P}_0(x_1,t)}{\mathrm{\partial }{x}_1}),V_0^2=V_0^3=0,\\ & P_1(t,x_1,{\mathit{y}}_{\ast })=f_2(t,x_1)\left({\mathbf{\text{e}}}_{\alpha }\right(x_1)\cdot {\mathit{y}}_{\ast })+f_3(t,x_1)\left({\mathbf{\text{e}}}_{\alpha }^{\perp }\right(x_1)\cdot {\mathit{y}}_{\ast }),\end{array}$(19) ), (23(23) $V_0^1(t,{\mathit{y}}_{\ast })=\frac{2}{\pi }(1-|{\mathit{y}}_{\ast }{|}^2\left)F_0^{\ast }\right(t).$(23) ), (24(24) $\begin{array}{rl}& W_0^1(t,x_1,{\mathit{y}}_{\ast })=\frac{1}{4\delta }(1-|{\mathit{y}}_{\ast }{|}^2\left)g_1\right(t,x_1),\\ & W_0^2(t,x_1,{\mathit{y}}_{\ast })=\frac{1}{2(2\delta +\beta )}(1-|{\mathit{y}}_{\ast }{|}^2\left)\right(g_2(t,x_1)\cos \alpha \left(x_1\right)+g_3(t,x_1)\sin \alpha \left(x_1\right)),\\ & W_0^3(t,x_1,{\mathit{y}}_{\ast })=\frac{1}{2(2\delta +\beta )}(1-|{\mathit{y}}_{\ast }{|}^2\left)\right(g_3(t,x_1)\cos \alpha \left(x_1\right)-g_2(t,x_1)\sin \alpha \left(x_1\right)).\end{array}$(24) ), (27(27) $V_1^1(t,x_1,{\mathit{y}}_{\ast })=\frac{1}{8\mu }(1-|{\mathit{y}}_{\ast }{|}^2\left)\right(y_2{H}_1(t,x_1)+y_3{H}_2(t,x_1)).$(27) ), (33(33) $W_1^1(t,x_1,{\mathit{y}}_{\ast })=\frac{1}{8\mu }(1-|{\mathit{y}}_{\ast }{|}^2\left)\right(y_2{H}_3(t,x_1)+y_3{H}_4(t,x_1)).$(33) ) and (36(36) $\begin{array}{rl}& W_1^2(t,x_1,{\mathit{y}}_{\ast })=(1-|{\mathit{y}}_{\ast }{|}^2\left)\right(y_2{H}_9(t,x_1)+y_3{H}_{10}(t,x_1)),\\ & W_1^3(t,x_1,{\mathit{y}}_{\ast })=(1-|{\mathit{y}}_{\ast }{|}^2\left)\right(y_2{H}_{11}(t,x_1)+y_3{H}_{12}(t,x_1)),\end{array}$(36) ) into (46(46) $\begin{array}{rl}& -\delta (\kappa \tau W_0^1\cos \alpha -(2\kappa \frac{\mathrm{\partial }{W}_0^1}{\mathrm{\partial }{x}_1}+{\kappa }^{\prime }{W}_0^1-{\kappa }^2{W}_0^2)\sin \alpha +{\kappa }^2\cos \alpha ({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast })\frac{\mathrm{\partial }{W}_0^3}{\mathrm{\partial }{y}_2}\\ & -{\kappa }^2\sin \alpha ({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast })\frac{\mathrm{\partial }{W}_0^3}{\mathrm{\partial }{y}_3})\\ & -\beta (\frac{{\mathrm{\partial }}^2{W}_1^1}{\mathrm{\partial }{x}_1\mathrm{\partial }{y}_3}-\kappa (\frac{\mathrm{\partial }{W}_1^2}{\mathrm{\partial }{y}_3}\cos \alpha -\frac{\mathrm{\partial }{W}_1^3}{\mathrm{\partial }{y}_3}\sin \alpha )+\kappa \tau W_0^1\cos \alpha \\ & -({\kappa }^{\prime }{W}_0^1+{\kappa }^2\cos \alpha W_0^2-{\kappa }^2\sin \alpha W_0^3))\\ & -2\beta \kappa (\frac{\mathrm{\partial }{W}_0^1}{\mathrm{\partial }{x}_1}-\kappa (W_0^2\cos \alpha -W_0^3\sin \alpha ))\sin \alpha -\beta ({\kappa }^{\prime }({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast })\frac{\mathrm{\partial }{W}_0^1}{\mathrm{\partial }{y}_3}+\kappa \tau ({\mathbf{\text{e}}}_{\alpha }^{\perp }\cdot {\mathit{y}}_{\ast })\frac{\mathrm{\partial }{W}_0^1}{\mathrm{\partial }{y}_3})\\ & -\beta {\kappa }^2({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast })(\cos \alpha \frac{\mathrm{\partial }{W}_0^2}{\mathrm{\partial }{y}_3}-\sin \alpha \frac{\mathrm{\partial }{W}_0^3}{\mathrm{\partial }{y}_3})-\beta (\frac{{\mathrm{\partial }}^2{W}_2^3}{\mathrm{\partial }{y}_3^2}+\frac{{\mathrm{\partial }}^2{W}_2^2}{\mathrm{\partial }{y}_2\mathrm{\partial }{y}_3})\\ & -2\beta \kappa ({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast })(\frac{{\mathrm{\partial }}^2{W}_0^1}{\mathrm{\partial }{x}_1\mathrm{\partial }{y}_3}-\kappa (\frac{\mathrm{\partial }{W}_0^2}{\mathrm{\partial }{y}_3}\cos \alpha -\frac{\mathrm{\partial }{W}_0^3}{\mathrm{\partial }{y}_3}\sin \alpha ))+2a{W}_0^3\\ & =a(\frac{\mathrm{\partial }{V}_0^2}{\mathrm{\partial }{x}_1}-\frac{\mathrm{\partial }{V}_1^1}{\mathrm{\partial }{y}_2}-\kappa ({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast })\frac{\mathrm{\partial }{V}_0^1}{\mathrm{\partial }{y}_2})\text{in}\mathrm{\Omega },\\ W_2^2& =W_2^3=0\text{on}\mathrm{\Gamma }.\end{array}$(46) ) yields: (47) $\begin{array}{rl}& -\delta (\frac{{\mathrm{\partial }}^2{W}_2^2}{\mathrm{\partial }{y}_2^2}+\frac{\mathrm{\partial }{W}_2^2}{\mathrm{\partial }{y}_3^2})-\beta (\frac{{\mathrm{\partial }}^2{W}_2^2}{\mathrm{\partial }{y}_2^2}+\frac{\mathrm{\partial }{W}_2^3}{\mathrm{\partial }{y}_2\mathrm{\partial }{y}_3})=C_5{y}_2^2+C_6{y}_2{y}_3+C_7{y}_3^2+C_8\text{in}\mathrm{\Omega },\\ & -\delta (\frac{{\mathrm{\partial }}^2{W}_2^3}{\mathrm{\partial }{y}_2^2}+\frac{{\mathrm{\partial }}^2{W}_2^3}{\mathrm{\partial }{y}_3^2})-\beta (\frac{{\mathrm{\partial }}^2{W}_2^2}{\mathrm{\partial }{y}_2\mathrm{\partial }{y}_3}+\frac{{\mathrm{\partial }}^2{W}_2^3}{\mathrm{\partial }{y}_3^2})=C_9{y}_2^2+C_{10}{y}_2{y}_3+C_{11}{y}_3^2+C_{12}\text{in}\mathrm{\Omega },\\ W_2^2& =W_2^3=0\text{on}\mathrm{\Gamma }.\end{array}$(47) The functions $C_5(t,x_1),\dots ,C_{12}(t,x_1)$ are given in the appendix (see (A10(A10) $\begin{array}{r}{C}_8(t,x_1)\\ & =-\frac{1}{2\delta +\beta }(\frac{\mathrm{\partial }{g}_2}{\mathrm{\partial }t}\cos \alpha +\frac{\mathrm{\partial }{g}_3}{\mathrm{\partial }t}\sin \alpha )+\frac{\delta }{2(2\delta +\beta )}(\frac{{\mathrm{\partial }}^2{g}_2}{\mathrm{\partial }{x}_1^2}\cos \alpha +\frac{\mathrm{\partial }{g}_2}{\mathrm{\partial }{x}_1}\tau \sin \alpha +({\tau }^{\prime }{g}_2+\tau \frac{\mathrm{\partial }{g}_2}{\mathrm{\partial }{x}_1})\sin \alpha \\ & -{\tau }^2{g}_2\cos \alpha +\frac{{\mathrm{\partial }}^2{g}_3}{\mathrm{\partial }{x}_1^2}\sin \alpha -\frac{\mathrm{\partial }{g}_3}{\mathrm{\partial }{x}_1}\tau \cos \alpha -({\tau }^{\prime }{g}_3+\tau \frac{\mathrm{\partial }{g}_3}{\mathrm{\partial }{x}_1})\cos \alpha -{\tau }^2{g}_3\sin \alpha )-\delta \kappa \cos \alpha H_9+\delta \kappa \sin \alpha H_{10}\\ & +\frac{1}{4}((2\kappa \frac{\mathrm{\partial }{g}_1}{\mathrm{\partial }{x}_1}+{\kappa }^{\prime }{g}_1-\frac{2\delta {\kappa }^2}{2\delta +\beta }(g_2\cos \alpha +g_3\sin \alpha ))\cos \alpha +\kappa \tau g_1\sin \alpha )+\frac{\beta }{8\mu }\frac{\mathrm{\partial }{H}_3}{\mathrm{\partial }{x}_1}-\beta \kappa \cos \alpha H_9\\ & +\beta \kappa \sin \alpha H_{11}+\beta \cos \alpha (\frac{{\kappa }^{\prime }}{4\delta }{g}_1+{\kappa }^2\cos \alpha \frac{1}{2(2\delta +\beta )}(g_2\cos \alpha +g_3\sin \alpha )\\ & -{\kappa }^2\sin \alpha \frac{1}{2(2\delta +\beta )}(g_2\cos \alpha +g_3\sin \alpha )\\ & +2\kappa (\frac{1}{4\alpha }\frac{\mathrm{\partial }{g}_1}{\mathrm{\partial }{x}_1}-\kappa (\cos \alpha \frac{1}{2(2\delta +\beta )}(g_2\cos \alpha +g_3\sin \alpha )-\sin \alpha \frac{1}{2(2\delta +\beta )}(g_3\cos \alpha -g_2\sin \alpha ))))\\ & +\beta \kappa \tau \sin \alpha \frac{g_1}{4\delta }\\ & +\frac{a}{8\mu }{H}_2-\frac{a}{2\delta +\beta }(g_2\cos \alpha +g_3\sin \alpha ),\end{array}$(A10) )–(A11(A11) $\begin{array}{rl}& C_{12}(t,x_1)\\ & =-\frac{2}{2(2\delta +\beta )}(\frac{\mathrm{\partial }{g}_3}{\mathrm{\partial }t}\cos \alpha -\frac{\mathrm{\partial }{g}_2}{\mathrm{\partial }t}\sin \alpha )+\frac{\delta }{2(2\delta +\beta )}(\frac{{\mathrm{\partial }}^2{g}_3}{\mathrm{\partial }{x}_1^2}\cos \alpha +\tau \frac{\mathrm{\partial }{g}_3}{\mathrm{\partial }{x}_1}\sin \alpha +({\tau }^{\prime }{g}_3+\tau \frac{\mathrm{\partial }{g}_3}{\mathrm{\partial }{x}_1})\sin \alpha \\ & -{\tau }^2{g}_3\cos \alpha \\ & -\frac{{\mathrm{\partial }}^2{g}_2}{\mathrm{\partial }{x}_1^2}\sin \alpha +\tau \frac{\mathrm{\partial }{g}_2}{\mathrm{\partial }{x}_1}\cos \alpha +({\tau }^{\prime }{g}_2+\tau \frac{\mathrm{\partial }{g}_2}{\mathrm{\partial }{x}_1})\cos \alpha +{\tau }^2{g}_2\sin \alpha )+\delta \kappa \sin \alpha H_{12}-\delta \kappa \cos \alpha H_{11}\\ & +(\cos \alpha \frac{\kappa \tau }{4}{g}_1-(\frac{\kappa }{2}\frac{\mathrm{\partial }{g}_1}{\mathrm{\partial }{x}_1}+\frac{{\kappa }^{\prime }}{4}{g}_1-\frac{{\kappa }^2}{2(2\delta +\beta )}(g_2\cos \alpha +g_3\sin \alpha ))\sin \alpha )+\frac{\beta }{8\mu }\frac{\mathrm{\partial }{H}_4}{\mathrm{\partial }{x}_1}-\beta \kappa \cos \alpha H_{10}\\ & +\beta \kappa \sin \alpha H_{12}+\beta \kappa \tau \cos \alpha \frac{1}{4\delta }{g}_1-\beta \kappa ({\kappa }^{\prime }\frac{1}{4\delta }{g}_1+{\kappa }^2\cos \alpha \frac{1}{2(2\delta +\beta )}(g_2\cos \alpha +g_3\sin \alpha )\\ & -{\kappa }^2\sin \alpha \frac{1}{2(2\delta +\beta )}(g_3\cos \alpha -g_2\sin \alpha ))+2\beta \kappa (\frac{1}{4\delta }\frac{\mathrm{\partial }{g}_1}{\mathrm{\partial }{x}_1}-\kappa (\cos \alpha \frac{1}{2(2\delta +\beta )}(g_2\cos \alpha +g_3\sin \alpha )\\ & -\frac{1}{2(2\delta +\beta )}\sin \alpha (g_3\cos \alpha -g_2\sin \alpha )))\sin \alpha -\frac{a}{8\mu }{H}_1-\frac{a}{2\delta +\beta }(g_3\cos \alpha -g_2\sin \alpha ).\end{array}$(A11) )).

The solution of the problem (47(47) $\begin{array}{rl}& -\delta (\frac{{\mathrm{\partial }}^2{W}_2^2}{\mathrm{\partial }{y}_2^2}+\frac{\mathrm{\partial }{W}_2^2}{\mathrm{\partial }{y}_3^2})-\beta (\frac{{\mathrm{\partial }}^2{W}_2^2}{\mathrm{\partial }{y}_2^2}+\frac{\mathrm{\partial }{W}_2^3}{\mathrm{\partial }{y}_2\mathrm{\partial }{y}_3})=C_5{y}_2^2+C_6{y}_2{y}_3+C_7{y}_3^2+C_8\text{in}\mathrm{\Omega },\\ & -\delta (\frac{{\mathrm{\partial }}^2{W}_2^3}{\mathrm{\partial }{y}_2^2}+\frac{{\mathrm{\partial }}^2{W}_2^3}{\mathrm{\partial }{y}_3^2})-\beta (\frac{{\mathrm{\partial }}^2{W}_2^2}{\mathrm{\partial }{y}_2\mathrm{\partial }{y}_3}+\frac{{\mathrm{\partial }}^2{W}_2^3}{\mathrm{\partial }{y}_3^2})=C_9{y}_2^2+C_{10}{y}_2{y}_3+C_{11}{y}_3^2+C_{12}\text{in}\mathrm{\Omega },\\ W_2^2& =W_2^3=0\text{on}\mathrm{\Gamma }.\end{array}$(47) ) takes the following form (48) $\begin{array}{rl}{W}_2^2(t,x_1,{\mathit{y}}_{\ast })& =\left(\right|{\mathit{y}}_{\ast }{|}^2-1\left)\right(D_5{y}_2^2+D_6{y}_2{y}_3+D_7{y}_3^2+D_8),\\ W_2^3(t,x_1,{\mathit{y}}_{\ast })& =\left(\right|{\mathit{y}}_{\ast }{|}^2-1\left)\right(D_9{y}_2^2+D_{10}{y}_2{y}_3+D_{11}{y}_3^2+D_{12}),\end{array}$(48) where $D_5(t,x_1),\dots ,D_{12}(t,x_1)$ can be found in the appendix (see (A12(A12) $\begin{array}{rl}{D}_5(t,x_1)& =\frac{(2\delta -\frac{12{\beta }^2}{12\delta +6\beta })D_7+C_5-\frac{3\beta C_{10}}{12\delta +6\beta }}{-14\delta -12\beta +\frac{12{\beta }^2}{12\delta +6\beta }},D_6(t,x_1)=\frac{-4\beta (D_9+D_{11})-C_6}{12\delta +6\beta },\\ D_7(t,x_1)& =\frac{(C_7-\frac{3\beta C_{10}}{12\delta +6\beta })(-14\delta -12\beta +\frac{12{\beta }^2}{12\delta +6\beta })-(-2\delta -2\beta +\frac{12{\beta }^2}{12\delta +6\beta })(C_5-\frac{3\beta C_{10}}{12\delta +6\beta })}{(-2\delta -2\beta +\frac{12{\beta }^2}{12\delta +6\beta })(2\delta -\frac{12{\beta }^2}{12\delta +6\beta })+(-14\delta -2\beta +\frac{12{\beta }^2}{12\delta +6\beta }{)}^2},\\ D_8(t,x_1)& =\frac{2\delta (D_5+D_7)+2\beta D_5+\beta D_{10}-C_8}{4\alpha +2\beta },\\ D_9(t,x_1)& =\frac{C_9-\frac{3\beta C_6}{12\delta +6\beta }+(2\delta +2\beta -\frac{12{\beta }^2}{12\delta +6\beta })D_{11}}{-14\delta -2\beta +\frac{12{\beta }^2}{12\delta +6\beta }},D_{10}(t,x_1)=\frac{-4\beta (D_5+D_7)-C_{10}}{12\delta +6\beta },\\ D_{11}(t,x_1)& =\frac{(C_{11}-\frac{3\beta C_6}{12\delta +6\beta })(-14\delta -2\beta +\frac{12{\beta }^2}{12\delta +6\beta })+(2\delta -\frac{12{\beta }^2}{12\delta +6\beta })(C_9-\frac{3\beta C_6}{12\delta +6\beta })}{(-2\delta +\frac{12{\beta }^2}{12\delta +6\beta })(2\delta +2\beta -\frac{12{\beta }^2}{12\delta +6\beta })+(-14\delta -12\beta +\frac{12{\beta }^2}{12\delta +6\beta })(-14\delta -2\beta +\frac{12{\beta }^2}{12\delta +6\beta })},\\ D_{12}(t,x_1)& =\frac{2\delta (D_9+D_{11})+2\beta D_{11}+\beta D_6-C_{12}}{4\delta +2\beta }.\end{array}$(A12) )).

To conclude this subsection, we notice that the expressions for $(W_2^1,W_2^2,W_2^3)$ given by (44(44) $W_2^1(t,x_1,{\mathit{y}}_{\ast })=\left(\right|{\mathit{y}}_{\ast }{|}^2-1\left)\right(D_1{y}_2^2+D_2{y}_2{y}_3+D_3{y}_3^2+D_4),$(44) )–(45(45) $D_1=\frac{7C_1-C_3}{96},D_2=\frac{1}{12}{C}_2,D_3=\frac{7C_3-C_1}{96},D_4=\frac{1}{4}{C}_4+\frac{1}{32}(C_1+C_3).$(45) ) and (48) contain the effects of the time derivative of the external force function $\mathbf{\text{g}}$.

4.2. Boundary layers

The regular part of our expansion (49) $\begin{array}{rl}{\mathbf{\text{U}}}_{\epsilon ,reg}^{\left[2\right]}(t,\mathit{x})& ={\epsilon }^2{V}_0(t,\frac{{\mathit{x}}_{\ast }}{\epsilon })+{\epsilon }^3{V}_1(t,x_1,\frac{{\mathit{x}}_{\ast }}{\epsilon })+{\epsilon }^4{V}_2(t,x_1,\frac{{\mathit{x}}_{\ast }}{\epsilon }),\\ V_j& =V_j^1{\mathbf{\text{a}}}^1+V_j^2{\mathbf{\text{a}}}^2+V_j^3{\mathbf{\text{a}}}^3,j=0,1,2,\\ {\mathbf{\text{W}}}_{\epsilon ,reg}^{\left[2\right]}(t,\mathit{x})& ={\epsilon }^2{W}_0(t,x_1\frac{{\mathit{x}}_{\ast }}{\epsilon })+{\epsilon }^3{W}_1(t,x_1,\frac{{\mathit{x}}_{\ast }}{\epsilon })+{\epsilon }^4{W}_2(t,x_1,\frac{{\mathit{x}}_{\ast }}{\epsilon }),\\ W_j& =W_j^1{\mathbf{\text{a}}}^1+W_j^2{\mathbf{\text{a}}}^2+W_j^3{\mathbf{\text{a}}}^3,j=0,1,2,\\ P_{\epsilon ,reg}^{\left[3\right]}(t,\mathit{x})& =P_0(t,x_1)+\epsilon P_1(t,x_1,\frac{{\mathit{x}}_{\ast }}{\epsilon })+{\epsilon }^2{P}_2(t,x_1,\frac{{\mathit{x}}_{\ast }}{\epsilon })+{\epsilon }^3{P}_3(t,x_1,\frac{{\mathit{x}}_{\ast }}{\epsilon })\end{array}$(49) has been computed to satisfy the governing equations and the lateral boundary conditions, while the boundary conditions at the ends of the pipe have been neglected in the process. To fix that, we introduce the boundary layer correctors at $x_1=0$: (50) $\begin{array}{rl}{\mathbf{\text{U}}}_{\epsilon ,bl,0}^{\left[2\right]}(t,\mathit{x})& ={\epsilon }^2{\mathcal{V}}_0(t,\frac{x_1}{\epsilon },\frac{{\mathit{x}}_{\ast }}{\epsilon })+{\epsilon }^3{\mathcal{V}}_1(t,\frac{x_1}{\epsilon },\frac{{\mathit{x}}_{\ast }}{\epsilon })\\ & +{\epsilon }^4{\mathcal{V}}_2(t,\frac{x_1}{\epsilon },\frac{{\mathit{x}}_{\ast }}{\epsilon }),{\mathcal{V}}_j={\mathcal{V}}_j^1{\mathbf{\text{a}}}^1+{\mathcal{V}}_j^2{\mathbf{\text{a}}}^2+{\mathcal{V}}_j^3{\mathbf{\text{a}}}^3,j=0,1,2,\\ {\mathbf{\text{W}}}_{\epsilon ,bl,0}^{\left[2\right]}(t,\mathit{x})& ={\epsilon }^2{\mathcal{W}}_0(t,\frac{x_1}{\epsilon },\frac{{\mathit{x}}_{\ast }}{\epsilon })+{\epsilon }^3{\mathcal{W}}_1(t,\frac{x_1}{\epsilon },\frac{{\mathit{x}}_{\ast }}{\epsilon })\\ & +{\epsilon }^4{\mathcal{W}}_2(t,\frac{x_1}{\epsilon },\frac{{\mathit{x}}_{\ast }}{\epsilon }),{\mathcal{W}}_j={\mathcal{W}}_j^1{\mathbf{\text{a}}}^1+{\mathcal{W}}_j^2{\mathbf{\text{a}}}^2+{\mathcal{W}}_j^3{\mathbf{\text{a}}}^3,j=0,1,2,\\ P_{\epsilon ,bl,0}^{\left[2\right]}(t,\mathit{x})& =\epsilon {\mathcal{P}}_0(t,\frac{x_1}{\epsilon },\frac{{\mathit{x}}_{\ast }}{\epsilon })+{\epsilon }^2{\mathcal{P}}_1(t,\frac{x_1}{\epsilon },\frac{{\mathit{x}}_{\ast }}{\epsilon })+{\epsilon }^3{\mathcal{P}}_2(t,\frac{x_1}{\epsilon },\frac{{\mathit{x}}_{\ast }}{\epsilon }),\end{array}$(50) and the boundary layer correctors on the opposite side $x_1=l$: (51) $\begin{array}{rl}{\mathbf{\text{U}}}_{\epsilon ,bl,l}^{\left[2\right]}(t,\mathit{x})& ={\epsilon }^2{\mathcal{Y}}_0(t,\frac{x_1-l}{\epsilon },\frac{{\mathit{x}}_{\ast }}{\epsilon })+{\epsilon }^3{\mathcal{Y}}_1(t,\frac{x_1-l}{\epsilon },\frac{{\mathit{x}}_{\ast }}{\epsilon })\\ & +{\epsilon }^4{\mathcal{Y}}_2(t,\frac{x_1-l}{\epsilon },\frac{{\mathit{x}}_{\ast }}{\epsilon }),{\mathcal{Y}}_j={\mathcal{Y}}_j{\mathbf{\text{a}}}^1+{\mathcal{Y}}_j{\mathbf{\text{a}}}^2+{\mathcal{Y}}_j{\mathbf{\text{a}}}^3,j=0,1,2,\\ {\mathbf{\text{W}}}_{\epsilon ,bl,l}^{\left[2\right]}(t,\mathit{x})& ={\epsilon }^2{\mathcal{Z}}_0(t,\frac{x_1-l}{\epsilon },\frac{{\mathit{x}}_{\ast }}{\epsilon })+{\epsilon }^3{\mathcal{Z}}_1(t,\frac{x_1-l}{\epsilon },\frac{{\mathit{x}}_{\ast }}{\epsilon })\\ & +{\epsilon }^4{\mathcal{Z}}_2(t,\frac{x_1-l}{\epsilon },\frac{{\mathit{x}}_{\ast }}{\epsilon }),{\mathcal{Z}}_j={\mathcal{Z}}_j^1{\mathbf{\text{a}}}^1+{\mathcal{Z}}_j^2{\mathbf{\text{a}}}^2+{\mathcal{Z}}_j^3{\mathbf{\text{a}}}^3,j=0,1,2,\\ P_{\epsilon ,bl,l}^{\left[2\right]}(t,\mathit{x})& =\epsilon {\mathcal{Q}}_0(t,\frac{x_1-l}{\epsilon },\frac{{\mathit{x}}_{\ast }}{\epsilon })+{\epsilon }^2{\mathcal{Q}}_1(t,\frac{x_1-l}{\epsilon },\frac{{\mathit{x}}_{\ast }}{\epsilon })+{\epsilon }^3{\mathcal{Q}}_2(t,\frac{x_1-l}{\epsilon },\frac{{\mathit{x}}_{\ast }}{\epsilon }).\end{array}$(51) The boundary layer corrector at $x_1=0$ given by (50(50) $\begin{array}{rl}{\mathbf{\text{U}}}_{\epsilon ,bl,0}^{\left[2\right]}(t,\mathit{x})& ={\epsilon }^2{\mathcal{V}}_0(t,\frac{x_1}{\epsilon },\frac{{\mathit{x}}_{\ast }}{\epsilon })+{\epsilon }^3{\mathcal{V}}_1(t,\frac{x_1}{\epsilon },\frac{{\mathit{x}}_{\ast }}{\epsilon })\\ & +{\epsilon }^4{\mathcal{V}}_2(t,\frac{x_1}{\epsilon },\frac{{\mathit{x}}_{\ast }}{\epsilon }),{\mathcal{V}}_j={\mathcal{V}}_j^1{\mathbf{\text{a}}}^1+{\mathcal{V}}_j^2{\mathbf{\text{a}}}^2+{\mathcal{V}}_j^3{\mathbf{\text{a}}}^3,j=0,1,2,\\ {\mathbf{\text{W}}}_{\epsilon ,bl,0}^{\left[2\right]}(t,\mathit{x})& ={\epsilon }^2{\mathcal{W}}_0(t,\frac{x_1}{\epsilon },\frac{{\mathit{x}}_{\ast }}{\epsilon })+{\epsilon }^3{\mathcal{W}}_1(t,\frac{x_1}{\epsilon },\frac{{\mathit{x}}_{\ast }}{\epsilon })\\ & +{\epsilon }^4{\mathcal{W}}_2(t,\frac{x_1}{\epsilon },\frac{{\mathit{x}}_{\ast }}{\epsilon }),{\mathcal{W}}_j={\mathcal{W}}_j^1{\mathbf{\text{a}}}^1+{\mathcal{W}}_j^2{\mathbf{\text{a}}}^2+{\mathcal{W}}_j^3{\mathbf{\text{a}}}^3,j=0,1,2,\\ P_{\epsilon ,bl,0}^{\left[2\right]}(t,\mathit{x})& =\epsilon {\mathcal{P}}_0(t,\frac{x_1}{\epsilon },\frac{{\mathit{x}}_{\ast }}{\epsilon })+{\epsilon }^2{\mathcal{P}}_1(t,\frac{x_1}{\epsilon },\frac{{\mathit{x}}_{\ast }}{\epsilon })+{\epsilon }^3{\mathcal{P}}_2(t,\frac{x_1}{\epsilon },\frac{{\mathit{x}}_{\ast }}{\epsilon }),\end{array}$(50) ) are defined on the semi-infinite cylinder ${\mathcal{G}}_0=⟨0,\mathrm{\infty }⟩\times B$, whereas the boundary layer correctors at $x_1=l$ given by (51(51) $\begin{array}{rl}{\mathbf{\text{U}}}_{\epsilon ,bl,l}^{\left[2\right]}(t,\mathit{x})& ={\epsilon }^2{\mathcal{Y}}_0(t,\frac{x_1-l}{\epsilon },\frac{{\mathit{x}}_{\ast }}{\epsilon })+{\epsilon }^3{\mathcal{Y}}_1(t,\frac{x_1-l}{\epsilon },\frac{{\mathit{x}}_{\ast }}{\epsilon })\\ & +{\epsilon }^4{\mathcal{Y}}_2(t,\frac{x_1-l}{\epsilon },\frac{{\mathit{x}}_{\ast }}{\epsilon }),{\mathcal{Y}}_j={\mathcal{Y}}_j{\mathbf{\text{a}}}^1+{\mathcal{Y}}_j{\mathbf{\text{a}}}^2+{\mathcal{Y}}_j{\mathbf{\text{a}}}^3,j=0,1,2,\\ {\mathbf{\text{W}}}_{\epsilon ,bl,l}^{\left[2\right]}(t,\mathit{x})& ={\epsilon }^2{\mathcal{Z}}_0(t,\frac{x_1-l}{\epsilon },\frac{{\mathit{x}}_{\ast }}{\epsilon })+{\epsilon }^3{\mathcal{Z}}_1(t,\frac{x_1-l}{\epsilon },\frac{{\mathit{x}}_{\ast }}{\epsilon })\\ & +{\epsilon }^4{\mathcal{Z}}_2(t,\frac{x_1-l}{\epsilon },\frac{{\mathit{x}}_{\ast }}{\epsilon }),{\mathcal{Z}}_j={\mathcal{Z}}_j^1{\mathbf{\text{a}}}^1+{\mathcal{Z}}_j^2{\mathbf{\text{a}}}^2+{\mathcal{Z}}_j^3{\mathbf{\text{a}}}^3,j=0,1,2,\\ P_{\epsilon ,bl,l}^{\left[2\right]}(t,\mathit{x})& =\epsilon {\mathcal{Q}}_0(t,\frac{x_1-l}{\epsilon },\frac{{\mathit{x}}_{\ast }}{\epsilon })+{\epsilon }^2{\mathcal{Q}}_1(t,\frac{x_1-l}{\epsilon },\frac{{\mathit{x}}_{\ast }}{\epsilon })+{\epsilon }^3{\mathcal{Q}}_2(t,\frac{x_1-l}{\epsilon },\frac{{\mathit{x}}_{\ast }}{\epsilon }).\end{array}$(51) ) are defined on ${\mathcal{G}}_l=⟨-\mathrm{\infty },0⟩\times B$.

It should be emphasized that, due to (11(11) ${\mathbf{\text{h}}}_0^{\epsilon }(t,\cdot ),{\mathbf{\text{h}}}_l^{\epsilon }(t,\cdot ),\mathbf{\text{F}}(t,\cdot ),\mathbf{\text{G}}(t,\cdot ),\mathrm{\forall }t\in [0,T^{\ast }],T^{\ast }>0.$(11) ), the homogeneous initial conditions (6(6) $\begin{array}{rl}& {\mathbf{\text{u}}}_{\epsilon }=0\text{on}{\mathrm{\Gamma }}_{\epsilon }^{\alpha },{\mathbf{\text{u}}}_{\epsilon }={\epsilon }^2{\mathbf{\text{h}}}_i^{\epsilon }\text{on}{\mathrm{\Sigma }}_{\epsilon }^i,i=0,l,\\ & {\mathbf{\text{w}}}_{\epsilon }=0\text{on}\mathrm{\partial }{\mathrm{\Omega }}_{\epsilon }^{\alpha },\\ & {\mathbf{\text{u}}}_{\epsilon }(0,\tilde{\mathit{x}})=0,{\mathbf{\text{w}}}_{\epsilon }(0,\tilde{\mathit{x}})=0,\end{array}$(6) )₃ are automatically satisfied and for this reason the boundary layer in time does not appear.

4.2.1. Boundary layer for the velocity and pressure

Zero-order approximation: Substituting the boundary layer correctors (50(50) $\begin{array}{rl}{\mathbf{\text{U}}}_{\epsilon ,bl,0}^{\left[2\right]}(t,\mathit{x})& ={\epsilon }^2{\mathcal{V}}_0(t,\frac{x_1}{\epsilon },\frac{{\mathit{x}}_{\ast }}{\epsilon })+{\epsilon }^3{\mathcal{V}}_1(t,\frac{x_1}{\epsilon },\frac{{\mathit{x}}_{\ast }}{\epsilon })\\ & +{\epsilon }^4{\mathcal{V}}_2(t,\frac{x_1}{\epsilon },\frac{{\mathit{x}}_{\ast }}{\epsilon }),{\mathcal{V}}_j={\mathcal{V}}_j^1{\mathbf{\text{a}}}^1+{\mathcal{V}}_j^2{\mathbf{\text{a}}}^2+{\mathcal{V}}_j^3{\mathbf{\text{a}}}^3,j=0,1,2,\\ {\mathbf{\text{W}}}_{\epsilon ,bl,0}^{\left[2\right]}(t,\mathit{x})& ={\epsilon }^2{\mathcal{W}}_0(t,\frac{x_1}{\epsilon },\frac{{\mathit{x}}_{\ast }}{\epsilon })+{\epsilon }^3{\mathcal{W}}_1(t,\frac{x_1}{\epsilon },\frac{{\mathit{x}}_{\ast }}{\epsilon })\\ & +{\epsilon }^4{\mathcal{W}}_2(t,\frac{x_1}{\epsilon },\frac{{\mathit{x}}_{\ast }}{\epsilon }),{\mathcal{W}}_j={\mathcal{W}}_j^1{\mathbf{\text{a}}}^1+{\mathcal{W}}_j^2{\mathbf{\text{a}}}^2+{\mathcal{W}}_j^3{\mathbf{\text{a}}}^3,j=0,1,2,\\ P_{\epsilon ,bl,0}^{\left[2\right]}(t,\mathit{x})& =\epsilon {\mathcal{P}}_0(t,\frac{x_1}{\epsilon },\frac{{\mathit{x}}_{\ast }}{\epsilon })+{\epsilon }^2{\mathcal{P}}_1(t,\frac{x_1}{\epsilon },\frac{{\mathit{x}}_{\ast }}{\epsilon })+{\epsilon }^3{\mathcal{P}}_2(t,\frac{x_1}{\epsilon },\frac{{\mathit{x}}_{\ast }}{\epsilon }),\end{array}$(50) ) into the Equations (14(14) $\begin{array}{rl}& -\mu ({\kappa }^2\cos \alpha ({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{x}}_{\ast })\frac{\mathrm{\partial }{V}_{\epsilon }^3}{\mathrm{\partial }{x}_2}-{\kappa }^2\sin \alpha ({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{x}}_{\ast })\frac{\mathrm{\partial }{V}_{\epsilon }^3}{\mathrm{\partial }{x}_3}){\mathbf{\text{a}}}^3\\ & +\frac{\mathrm{\partial }{P}_{\epsilon }}{\mathrm{\partial }{x}_1}(1+\kappa ({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{x}}_{\ast })+\cdots )\mathbf{\text{t}}+\frac{\mathrm{\partial }{P}_{\epsilon }}{\mathrm{\partial }{x}_2}{\mathbf{\text{a}}}^2+\frac{\mathrm{\partial }{P}_{\epsilon }}{\mathrm{\partial }{x}_3}{\mathbf{\text{a}}}^3\\ & =a(\frac{\mathrm{\partial }{W}_{\epsilon }^3}{\mathrm{\partial }{x}_2}-\frac{\mathrm{\partial }{W}_{\epsilon }^2}{\mathrm{\partial }{x}_3})\mathbf{\text{t}}+a(1+\kappa ({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{x}}_{\ast })+\cdots )(\frac{\mathrm{\partial }{W}_{\epsilon }^1}{\mathrm{\partial }{x}_3}-\frac{\mathrm{\partial }{W}_{\epsilon }^3}{\mathrm{\partial }{x}_1}){\mathbf{\text{a}}}^2+a(1+\kappa ({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{x}}_{\ast })+\cdots )\\ & (\frac{\mathrm{\partial }{W}_{\epsilon }^2}{\mathrm{\partial }{x}_1}-\frac{\mathrm{\partial }{W}_{\epsilon }^1}{\mathrm{\partial }{x}_2}){\mathbf{\text{a}}}^3\\ & +f_1\mathbf{\text{t}}+(f_2\cos \alpha +f_3\sin \alpha ){\mathbf{\text{a}}}^2+(f_3\cos \alpha -f_2\sin \alpha ){\mathbf{\text{a}}}^3.\end{array}$(14) )–(15(15) $\begin{array}{rl}& [\frac{1}{1-\kappa ({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{x}}_{\ast })}{]}^2(\frac{\mathrm{\partial }{V}_{\epsilon }^1}{\mathrm{\partial }{x}_1}-\kappa (V_{\epsilon }^2\cos \alpha -V_{\epsilon }^3\sin \alpha )+\left({\kappa }^{\prime }\right({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{x}}_{\ast })+\kappa \tau ({\mathbf{\text{e}}}_{\alpha }^{\perp }\cdot {\mathit{x}}_{\ast }\left)\right)V_{\epsilon }^1\\ & +\kappa ({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{x}}_{\ast })\left({\kappa }^{\prime }\right({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{x}}_{\ast })+\kappa \tau ({\mathbf{\text{e}}}_{\alpha }^{\perp }\cdot {\mathit{x}}_{\ast }\left)\right)V_{\epsilon }^1+{\kappa }^2({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{x}}_{\ast })\cos \alpha V_{\epsilon }^2-{\kappa }^2({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{x}}_{\ast })\sin \alpha V_{\epsilon }^3)\\ & +\frac{\mathrm{\partial }{V}_{\epsilon }^2}{\mathrm{\partial }{x}_2}+\frac{\mathrm{\partial }{V}_{\epsilon }^3}{\mathrm{\partial }{x}_3}=\frac{\mathrm{\partial }{V}_{\epsilon }^1}{\mathrm{\partial }{x}_1}-\kappa (V_{\epsilon }^2\cos \alpha -V_{\epsilon }^3\sin \alpha )+\left({\kappa }^{\prime }\right({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{x}}_{\ast })+\kappa \tau ({\mathbf{\text{e}}}_{\alpha }^{\perp }\cdot {\mathit{x}}_{\ast }\left)\right)V_{\epsilon }^1\\ & +\kappa ({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{x}}_{\ast })\left({\kappa }^{\prime }\right({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{x}}_{\ast })+\kappa \tau ({\mathbf{\text{e}}}_{\alpha }^{\perp }\cdot {\mathit{x}}_{\ast }\left)\right)V_{\epsilon }^1+{\kappa }^2({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{x}}_{\ast })\cos \alpha V_{\epsilon }^2-{\kappa }^2({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{x}}_{\ast })\sin \alpha V_{\epsilon }^3\\ & +\frac{\mathrm{\partial }{V}_{\epsilon }^2}{\mathrm{\partial }{x}_2}+\frac{\mathrm{\partial }{V}_{\epsilon }^3}{\mathrm{\partial }{x}_3}+2\kappa ({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{x}}_{\ast })(\frac{\mathrm{\partial }{V}_{\epsilon }^1}{\mathrm{\partial }{x}_1}-\kappa (V_{\epsilon }^2\cos \alpha -V_{\epsilon }^3\sin \alpha )+\left({\kappa }^{\prime }\right({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{x}}_{\ast })+\kappa \tau ({\mathbf{\text{e}}}_{\alpha }^{\perp }\cdot {\mathit{x}}_{\ast }\left)\right)V_{\epsilon }^1\\ & +{\kappa }^2({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{x}}_{\ast })\cos \alpha V_{\epsilon }^2-{\kappa }^2({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{x}}_{\ast })\sin \alpha V_{\epsilon }^3)+3{\kappa }^2({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{x}}_{\ast }{)}^2(\frac{\mathrm{\partial }{V}_{\epsilon }^1}{\mathrm{\partial }{x}_1}-\kappa (V_{\epsilon }^2\cos \alpha -V_{\epsilon }^3\sin \alpha ))\\ & +4{\kappa }^3({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{x}}_{\ast }{)}^3\frac{\mathrm{\partial }{V}_{\epsilon }^1}{\mathrm{\partial }{x}_1}+\mathcal{O}({\epsilon }^3)=0.\end{array}$(15) ) and collecting the ${\epsilon }^0$ terms, we obtain the problem for the velocity and pressure zero-order boundary layer approximation at $x_1=0$: (52) $\begin{array}{rl}& -\mu \mathrm{\Delta }{\mathcal{V}}_0+\mathrm{\nabla }{\mathcal{P}}_0=0\text{in}{\mathcal{G}}_0,\\ & \text{div}{\mathcal{V}}_0=0\text{in}{\mathcal{G}}_0,{\mathcal{V}}_0=0\text{on}\omega =⟨0,\mathrm{\infty }⟩\times \mathrm{\partial }B,\\ & {\mathcal{V}}_0(t,0,\cdot )=(h_0^t-V_0^1(t,0,\cdot ),h_0^n\cos \alpha (0)+h_0^b\sin \alpha (0),h_0^b\cos \alpha (0)-h_0^n\sin \alpha (0\left)\right).\end{array}$(52) System (52(52) $\begin{array}{rl}& -\mu \mathrm{\Delta }{\mathcal{V}}_0+\mathrm{\nabla }{\mathcal{P}}_0=0\text{in}{\mathcal{G}}_0,\\ & \text{div}{\mathcal{V}}_0=0\text{in}{\mathcal{G}}_0,{\mathcal{V}}_0=0\text{on}\omega =⟨0,\mathrm{\infty }⟩\times \mathrm{\partial }B,\\ & {\mathcal{V}}_0(t,0,\cdot )=(h_0^t-V_0^1(t,0,\cdot ),h_0^n\cos \alpha (0)+h_0^b\sin \alpha (0),h_0^b\cos \alpha (0)-h_0^n\sin \alpha (0\left)\right).\end{array}$(52) ) admits a unique (up to an additive constant in the pressure) solution ${\mathcal{V}}_0(t,\cdot )\in {\stackrel{\circ }{W}}^{1,2}\left({\mathcal{G}}_0\right),{\mathcal{P}}_0(t,\cdot )\in L_{loc}^2\left({\mathcal{G}}_0\right)$ since the necessary compatibility conditions holds ${\int }_B(h_0^t-V_0^1(t,0,\cdot ))=F_0^{\ast }\left(t\right)-F_0^{\ast }\left(t\right)=0.$ It is also well known that these functions in some sense exponentially decay to zero as $y_1\to \mathrm{\infty }$ (see e.g. [25]).

The problem for the velocity and pressure zero-order boundary layer approximations at $x_1=l$ given by (51(51) $\begin{array}{rl}{\mathbf{\text{U}}}_{\epsilon ,bl,l}^{\left[2\right]}(t,\mathit{x})& ={\epsilon }^2{\mathcal{Y}}_0(t,\frac{x_1-l}{\epsilon },\frac{{\mathit{x}}_{\ast }}{\epsilon })+{\epsilon }^3{\mathcal{Y}}_1(t,\frac{x_1-l}{\epsilon },\frac{{\mathit{x}}_{\ast }}{\epsilon })\\ & +{\epsilon }^4{\mathcal{Y}}_2(t,\frac{x_1-l}{\epsilon },\frac{{\mathit{x}}_{\ast }}{\epsilon }),{\mathcal{Y}}_j={\mathcal{Y}}_j{\mathbf{\text{a}}}^1+{\mathcal{Y}}_j{\mathbf{\text{a}}}^2+{\mathcal{Y}}_j{\mathbf{\text{a}}}^3,j=0,1,2,\\ {\mathbf{\text{W}}}_{\epsilon ,bl,l}^{\left[2\right]}(t,\mathit{x})& ={\epsilon }^2{\mathcal{Z}}_0(t,\frac{x_1-l}{\epsilon },\frac{{\mathit{x}}_{\ast }}{\epsilon })+{\epsilon }^3{\mathcal{Z}}_1(t,\frac{x_1-l}{\epsilon },\frac{{\mathit{x}}_{\ast }}{\epsilon })\\ & +{\epsilon }^4{\mathcal{Z}}_2(t,\frac{x_1-l}{\epsilon },\frac{{\mathit{x}}_{\ast }}{\epsilon }),{\mathcal{Z}}_j={\mathcal{Z}}_j^1{\mathbf{\text{a}}}^1+{\mathcal{Z}}_j^2{\mathbf{\text{a}}}^2+{\mathcal{Z}}_j^3{\mathbf{\text{a}}}^3,j=0,1,2,\\ P_{\epsilon ,bl,l}^{\left[2\right]}(t,\mathit{x})& =\epsilon {\mathcal{Q}}_0(t,\frac{x_1-l}{\epsilon },\frac{{\mathit{x}}_{\ast }}{\epsilon })+{\epsilon }^2{\mathcal{Q}}_1(t,\frac{x_1-l}{\epsilon },\frac{{\mathit{x}}_{\ast }}{\epsilon })+{\epsilon }^3{\mathcal{Q}}_2(t,\frac{x_1-l}{\epsilon },\frac{{\mathit{x}}_{\ast }}{\epsilon }).\end{array}$(51) ) is analogous as for $({\mathcal{V}}_0,{\mathcal{P}}_0)$ : (53) $\begin{array}{rl}& -\mu \mathrm{\Delta }{\mathcal{Y}}_0+\mathrm{\nabla }{\mathcal{Q}}_0=0\text{in}{\mathcal{G}}_l,\\ & \text{div}{\mathcal{Y}}_0=0\text{in}{\mathcal{G}}_l,{\mathcal{Y}}_0=0\text{on}\sigma =⟨-\mathrm{\infty },0⟩\times \mathrm{\partial }B,\\ & {\mathcal{Y}}_0(t,0,\cdot )=(h_l^t-V_0^1(t,l,\cdot ),h_l^n\cos \alpha (l)+h_l^b\sin \alpha (l),h_l^b\cos \alpha (l)-h_l^n\sin \alpha (l\left)\right).\end{array}$(53) The well-posedness and the exponential decay of the solution $({\mathcal{Y}}_0,{\mathcal{Q}}_0)$ follow analogously as for $({\mathcal{V}}_0,{\mathcal{P}}_0)$.

First-order corrector: The first-order boundary layer correctors at $x_1=0$ for the velocity and pressure $({\mathcal{V}}_1,{\mathcal{P}}_1)$ are given as the solution of the following problem: (54) $\begin{array}{rl}& -\mu \mathrm{\Delta }{\mathcal{V}}_1+\mathrm{\nabla }{\mathcal{P}}_1=\mathrm{\Xi }(t,0,{\mathcal{V}}_0,{\mathcal{W}}_0,{\mathcal{P}}_0)\text{in}{\mathcal{G}}_0,\\ & \text{div}{\mathcal{V}}_1=\kappa \left(0\right)\cos \alpha \left(0\right){\mathcal{V}}_0^2-\kappa \left(0\right)\sin \alpha \left(0\right){\mathcal{V}}_0^3\\ & -(2\kappa \left(0\right)\cos \alpha \left(0\right)y_2\frac{\mathrm{\partial }{\mathcal{V}}_0^1}{\mathrm{\partial }{y}_1}-2\kappa \left(0\right)\sin \alpha \left(0\right)y_3\frac{\mathrm{\partial }{\mathcal{V}}_0^1}{\mathrm{\partial }{y}_1}),{\mathcal{V}}_1=0\text{on}\omega =⟨0,\mathrm{\infty }⟩\times \mathrm{\partial }B,\\ & {\mathcal{V}}_1(t,0,\cdot )=-\left(V_1^1\right(t,0,\cdot ),V_1^2(t,0,\cdot ),V_1^3(t,0,\cdot \left)\right)-\left(\kappa \right(0\left)\right({\mathbf{\text{e}}}_{\alpha }\left(0\right)\cdot {\mathit{y}}_{\ast }\left)V_0^1\right(t,0,\cdot ),0,0)\\ & -\left(\kappa \right(0\left)\right({\mathbf{\text{e}}}_{\alpha }\left(0\right)\cdot {\mathit{y}}_{\ast }\left){\mathcal{V}}_0^1\right(t,0,\cdot ),0,0),\end{array}$(54) where $\mathrm{\Xi }(t,x_1,\mathbf{\text{J}},\mathbf{\text{K}},j)$ is an exponentially decreasing function provided in the appendix (see (A13(A13) $\begin{array}{rl}\mathrm{\Xi }(t,x_1,\mathbf{\text{J}},\mathbf{\text{K}},j)& =\mu (\kappa \cos \alpha (\frac{\mathrm{\partial }{J}^1}{\mathrm{\partial }{y}_2}-\frac{\mathrm{\partial }{J}^2}{\mathrm{\partial }{y}_1})+\kappa \sin \alpha (\frac{\mathrm{\partial }{J}^3}{\mathrm{\partial }{y}_1}-\frac{\mathrm{\partial }{V}^1}{\mathrm{\partial }{y}_3})-\kappa (\frac{\mathrm{\partial }{J}^2}{\mathrm{\partial }{y}_1}\cos \alpha -\frac{\mathrm{\partial }{J}^3}{\mathrm{\partial }{y}_1}\sin \alpha )+{\kappa }^{\prime }\frac{\mathrm{\partial }{J}^2}{\mathrm{\partial }{y}_1}\cos \alpha \\ & -{\kappa }^{\prime }\frac{\mathrm{\partial }{J}^3}{\mathrm{\partial }{y}_1}\sin \alpha +\kappa ({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast })\mathrm{\Delta }{J}^1,-\kappa \cos \alpha \frac{\mathrm{\partial }{J}^2}{\mathrm{\partial }{y}_2}+\kappa \sin \alpha \frac{\mathrm{\partial }{J}^2}{\mathrm{\partial }{y}_3}+2\kappa \cos \alpha \frac{\mathrm{\partial }{J}^1}{\mathrm{\partial }{y}_1},\kappa \sin \alpha \frac{\mathrm{\partial }{J}^3}{\mathrm{\partial }{y}_3}\\ & -\kappa \cos \alpha \frac{\mathrm{\partial }{J}^3}{\mathrm{\partial }{y}_2}-2\kappa \sin \alpha \frac{\mathrm{\partial }{J}^1}{\mathrm{\partial }{y}_1})-(\kappa ({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast })\frac{\mathrm{\partial }j}{\mathrm{\partial }{y}_1},0,0)\\ & +a(\frac{\mathrm{\partial }{K}^3}{\mathrm{\partial }{y}_2}-\frac{\mathrm{\partial }{K}^2}{\mathrm{\partial }{y}_3},\frac{\mathrm{\partial }{K}^1}{\mathrm{\partial }{y}_3}-\frac{\mathrm{\partial }{K}^3}{\mathrm{\partial }{y}_1},\frac{\mathrm{\partial }{K}^2}{\mathrm{\partial }{y}_1}-\frac{\mathrm{\partial }{K}^1}{\mathrm{\partial }{y}_2}),\end{array}$(A13) )).

To ensure the well-posedness of the problem (54(54) $\begin{array}{rl}& -\mu \mathrm{\Delta }{\mathcal{V}}_1+\mathrm{\nabla }{\mathcal{P}}_1=\mathrm{\Xi }(t,0,{\mathcal{V}}_0,{\mathcal{W}}_0,{\mathcal{P}}_0)\text{in}{\mathcal{G}}_0,\\ & \text{div}{\mathcal{V}}_1=\kappa \left(0\right)\cos \alpha \left(0\right){\mathcal{V}}_0^2-\kappa \left(0\right)\sin \alpha \left(0\right){\mathcal{V}}_0^3\\ & -(2\kappa \left(0\right)\cos \alpha \left(0\right)y_2\frac{\mathrm{\partial }{\mathcal{V}}_0^1}{\mathrm{\partial }{y}_1}-2\kappa \left(0\right)\sin \alpha \left(0\right)y_3\frac{\mathrm{\partial }{\mathcal{V}}_0^1}{\mathrm{\partial }{y}_1}),{\mathcal{V}}_1=0\text{on}\omega =⟨0,\mathrm{\infty }⟩\times \mathrm{\partial }B,\\ & {\mathcal{V}}_1(t,0,\cdot )=-\left(V_1^1\right(t,0,\cdot ),V_1^2(t,0,\cdot ),V_1^3(t,0,\cdot \left)\right)-\left(\kappa \right(0\left)\right({\mathbf{\text{e}}}_{\alpha }\left(0\right)\cdot {\mathit{y}}_{\ast }\left)V_0^1\right(t,0,\cdot ),0,0)\\ & -\left(\kappa \right(0\left)\right({\mathbf{\text{e}}}_{\alpha }\left(0\right)\cdot {\mathit{y}}_{\ast }\left){\mathcal{V}}_0^1\right(t,0,\cdot ),0,0),\end{array}$(54) ), we need to verify the compatibility condition (55) ${\int }_{{\mathcal{G}}_0}\text{div}{\mathcal{V}}_1=-{\int }_B{\mathcal{V}}_1^1(t,0,\cdot ).$(55) Since $\text{div}\left(y_2{\mathcal{V}}_0\right)={\mathcal{V}}_0^2,\text{div}\left(y_3{\mathcal{V}}_0\right)={\mathcal{V}}_0^3,$ we obtain $\begin{array}{rl}{\int }_{{\mathcal{G}}_0}{\mathcal{V}}_0^2& =-{\int }_B{y}_2{\mathcal{V}}_0^1(t,0,\cdot )={\int }_B{y}_2(V_0^1-h_0^t)=-{\int }_B{y}_2{h}_0^t,\\ {\int }_{{\mathcal{G}}_0}{\mathcal{V}}_0^3& =-{\int }_B{y}_3{\mathcal{V}}_0^1(t,0,\cdot )={\int }_B{y}_3(V_0^1-h_0^t)=-{\int }_B{y}_3{h}_0^t\end{array}$ yielding $\begin{array}{rl}{\int }_{{\mathcal{G}}_0}\text{div}{\mathcal{V}}_1& =\kappa \left(0\right)\cos \alpha \left(0\right){\int }_{{\mathcal{G}}_0}{\mathcal{V}}_0^2-\kappa \left(0\right)\sin \alpha \left(0\right){\int }_{{\mathcal{G}}_0}{\mathcal{V}}_0^3-2\kappa \left(0\right)\cos \alpha \left(0\right)\\ & {\int }_{{\mathcal{G}}_0}{y}_2\frac{\mathrm{\partial }{\mathcal{V}}_0^1}{\mathrm{\partial }{y}_1}+2\kappa \left(0\right)\sin \alpha \left(0\right){\int }_{{\mathcal{G}}_0}{y}_3\frac{\mathrm{\partial }{\mathcal{V}}_0^1}{\mathrm{\partial }{y}_1}\\ & =-\kappa \left(0\right)\cos \alpha \left(0\right){\int }_B{y}_2{h}_0^t+\kappa \left(0\right)\sin \alpha \left(0\right){\int }_B{y}_3{h}_0^t-2\kappa \left(0\right)\cos \alpha \left(0\right)\\ & {\int }_{{\mathcal{G}}_0}{y}_2\frac{\mathrm{\partial }{\mathcal{V}}_0^1}{\mathrm{\partial }{y}_1}+2\kappa \left(0\right)\sin \alpha \left(0\right){\int }_{{\mathcal{G}}_0}{y}_3\frac{\mathrm{\partial }{\mathcal{V}}_0^1}{\mathrm{\partial }{y}_1}.\end{array}$ On the other hand, we have $\begin{array}{rl}{\int }_B{\mathcal{V}}_1^1(t,0,\cdot )=& -{\int }_B{V}_1^1(t,0,\cdot )-\kappa \left(0\right)\cos \alpha \left(0\right){\int }_B{y}_2{V}_0^1(t,0,\cdot )+\kappa \left(0\right)\sin \alpha \left(0\right){\int }_B{y}_3{V}_0^1(t,0,\cdot )\\ & -\kappa \left(0\right)\cos \alpha \left(0\right){\int }_B{y}_2(h_0^t-V_0^1(t,0,\cdot ))+\kappa \left(0\right)\sin \alpha \left(0\right){\int }_B{y}_3(h_0^t-V_0^1(t,0,\cdot ))\\ & =-\kappa \left(0\right)\cos \alpha \left(0\right){\int }_B{y}_2{h}_0^t+\kappa \left(0\right)\sin \alpha \left(0\right){\int }_B{y}_3{h}_0^t,\end{array}$ and, in order to satisfy the compatibility condition (55(55) ${\int }_{{\mathcal{G}}_0}\text{div}{\mathcal{V}}_1=-{\int }_B{\mathcal{V}}_1^1(t,0,\cdot ).$(55) ), we need to verify the following: (56) $\begin{array}{rl}& -2\kappa \left(0\right)\cos \alpha \left(0\right){\int }_{{\mathcal{G}}_0}{y}_2\frac{\mathrm{\partial }{\mathcal{V}}_0^1}{\mathrm{\partial }{y}_1}+2\kappa \left(0\right)\sin \alpha \left(0\right){\int }_{{\mathcal{G}}_0}{y}_3\frac{\mathrm{\partial }{\mathcal{V}}_0^1}{\mathrm{\partial }{y}_1}=2\kappa \left(0\right)\cos \alpha \left(0\right)\\ & {\int }_B{y}_2{h}_0^t-2\kappa \left(0\right)\sin \alpha \left(0\right){\int }_B{y}_3{h}_0^t.\end{array}$(56) Using the fact that ${\mathcal{V}}_0^1$ exponentially decays as $y_1\to \mathrm{\infty }$, we have: $\begin{array}{rl}{\int }_{{\mathcal{G}}_0}{y}_2\frac{\mathrm{\partial }{\mathcal{V}}_0^1}{\mathrm{\partial }{y}_1}& ={\int }_B{y}_2\underset{a\to \mathrm{\infty }}{lim}{\int }_0^a\frac{\mathrm{\partial }{\mathcal{V}}_0^1}{\mathrm{\partial }{y}_1}={\int }_B{y}_2\underset{a\to \mathrm{\infty }}{lim}\left({\mathcal{V}}_0^1\right(t,a,\cdot )-{\mathcal{V}}_0(t,0,\cdot \left)\right)\\ & =-{\int }_B{y}_2(h_0^t-V_0^1(t,0,\cdot \left)\right)=-{\int }_B{y}_2{h}_0^t\end{array}$ and ${\int }_{{\mathcal{G}}_0}{y}_3\frac{\mathrm{\partial }{\mathcal{V}}_0^1}{\mathrm{\partial }{y}_1}=-{\int }_B{y}_3{h}_0^t,$ thus verifying the compatibility condition (56(56) $\begin{array}{rl}& -2\kappa \left(0\right)\cos \alpha \left(0\right){\int }_{{\mathcal{G}}_0}{y}_2\frac{\mathrm{\partial }{\mathcal{V}}_0^1}{\mathrm{\partial }{y}_1}+2\kappa \left(0\right)\sin \alpha \left(0\right){\int }_{{\mathcal{G}}_0}{y}_3\frac{\mathrm{\partial }{\mathcal{V}}_0^1}{\mathrm{\partial }{y}_1}=2\kappa \left(0\right)\cos \alpha \left(0\right)\\ & {\int }_B{y}_2{h}_0^t-2\kappa \left(0\right)\sin \alpha \left(0\right){\int }_B{y}_3{h}_0^t.\end{array}$(56) ). Consequently, the well-posedness of the problem (54(54) $\begin{array}{rl}& -\mu \mathrm{\Delta }{\mathcal{V}}_1+\mathrm{\nabla }{\mathcal{P}}_1=\mathrm{\Xi }(t,0,{\mathcal{V}}_0,{\mathcal{W}}_0,{\mathcal{P}}_0)\text{in}{\mathcal{G}}_0,\\ & \text{div}{\mathcal{V}}_1=\kappa \left(0\right)\cos \alpha \left(0\right){\mathcal{V}}_0^2-\kappa \left(0\right)\sin \alpha \left(0\right){\mathcal{V}}_0^3\\ & -(2\kappa \left(0\right)\cos \alpha \left(0\right)y_2\frac{\mathrm{\partial }{\mathcal{V}}_0^1}{\mathrm{\partial }{y}_1}-2\kappa \left(0\right)\sin \alpha \left(0\right)y_3\frac{\mathrm{\partial }{\mathcal{V}}_0^1}{\mathrm{\partial }{y}_1}),{\mathcal{V}}_1=0\text{on}\omega =⟨0,\mathrm{\infty }⟩\times \mathrm{\partial }B,\\ & {\mathcal{V}}_1(t,0,\cdot )=-\left(V_1^1\right(t,0,\cdot ),V_1^2(t,0,\cdot ),V_1^3(t,0,\cdot \left)\right)-\left(\kappa \right(0\left)\right({\mathbf{\text{e}}}_{\alpha }\left(0\right)\cdot {\mathit{y}}_{\ast }\left)V_0^1\right(t,0,\cdot ),0,0)\\ & -\left(\kappa \right(0\left)\right({\mathbf{\text{e}}}_{\alpha }\left(0\right)\cdot {\mathit{y}}_{\ast }\left){\mathcal{V}}_0^1\right(t,0,\cdot ),0,0),\end{array}$(54) ) is established. Notice that $({\mathcal{V}}_1,{\mathcal{P}}_1)$ exponentially decay to zero as $y_1\to \mathrm{\infty }$ since Ξ is an exponentially decreasing function in $y_1$ (see [25]).

The existence of the unique solution exponentially decaying to zero corresponding to the problem on the opposite side $x_1=l$ for the first-order boundary layer correctors $({\mathcal{Y}}_1,{\mathcal{Q}}_1)$ follows in a similar manner.

Second-order corrector: The second-order boundary layer correctors at $x_1=0$ for the velocity and pressure $({\mathcal{V}}_2,{\mathcal{P}}_2)$ are given by: (57) $\begin{array}{rl}& -\mu \mathrm{\Delta }{\mathcal{V}}_2+\mathrm{\nabla }{\mathcal{P}}_2=\mathrm{\Pi }(t,0,{\mathcal{V}}_0,{\mathcal{V}}_1,{\mathcal{W}}_0,{\mathcal{W}}_1,{\mathcal{P}}_0,{\mathcal{P}}_1)\text{in}{\mathcal{G}}_0,\\ & \text{div}{\mathcal{V}}_2=\kappa \left(0\right)\cos \alpha \left(0\right){\mathcal{V}}_1^2-\kappa \left(0\right)\sin \alpha \left(0\right){\mathcal{V}}_1^3-\left({\kappa }^{\prime }\right(0\left)\right({\mathbf{\text{e}}}_{\alpha }\left(0\right)\cdot {\mathit{y}}_{\ast })+\kappa (0\left)\tau \right(0\left)\right({\mathbf{\text{e}}}_{\alpha }^{\perp }\left(0\right)\cdot {\mathit{y}}_{\ast }\left)\right){\mathcal{V}}_0^1\\ & -{\kappa }^2\left(0\right)\left({\mathbf{\text{e}}}_{\alpha }\right(0)\cdot {\mathit{y}}_{\ast })\cos \alpha \left(0\right){\mathcal{V}}_0^2+{\kappa }^2\left(0\right)\left({\mathbf{\text{e}}}_{\alpha }\right(0)\cdot {\mathit{y}}_{\ast })\sin \alpha \left(0\right){\mathcal{V}}_0^3\\ & +2\kappa \left(0\right)\left({\mathbf{\text{e}}}_{\alpha }\right(0)\cdot {\mathit{y}}_{\ast })(\kappa \left(0\right)\cos \alpha \left(0\right){\mathcal{V}}_0^2-\kappa \left(0\right)\sin \alpha \left(0\right){\mathcal{V}}_0^3)\\ & -2\kappa \left(0\right)\left({\mathbf{\text{e}}}_{\alpha }\right(0)\cdot {\mathit{y}}_{\ast })\frac{\mathrm{\partial }{\mathcal{V}}_1^1}{\mathrm{\partial }{y}_1}-3{\kappa }^2\left(0\right)\left({\mathbf{\text{e}}}_{\alpha }\right(0)\cdot {\mathit{y}}_{\ast }{)}^2\frac{\mathrm{\partial }{\mathcal{V}}_0^1}{\mathrm{\partial }{y}_1}\text{in}{\mathcal{G}}_0,\\ & {\mathcal{V}}_2=0\text{in}\omega =⟨0,\mathrm{\infty }⟩\times \mathrm{\partial }B,\\ & {\mathcal{V}}_2(t,0,\cdot )=-V_2(t,0,\cdot )-\left(\kappa \right({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast }\left)V_1^1\right(t,0,\cdot ),0,0)-\left({\kappa }^2\right({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast }{)}^2{V}_0^1(t,0,\cdot ),0,0)\\ & -\left(\kappa \right({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast }\left){\mathcal{V}}_1^1\right(t,0,\cdot ),0,0)-\left({\kappa }^2\right({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast }{)}^2{\mathcal{V}}_0^1(t,0,\cdot ),0,0),\end{array}$(57) where $\mathrm{\Pi }(t,x_1,{\mathbf{\text{J}}}_0,{\mathbf{\text{J}}}_1,{\mathbf{\text{K}}}_0,{\mathbf{\text{K}}}_1,j_0,j_1)$ is an exponentially decreasing function given in the appendix (see (A14(A14) $\begin{array}{rl}\mathrm{\Pi }(t,x_1,{\mathbf{\text{J}}}_0,{\mathbf{\text{J}}}_1,{\mathbf{\text{K}}}_0,{\mathbf{\text{K}}}_1,j_0,j_1)& =-(\frac{\mathrm{\partial }{J}_0^1}{\mathrm{\partial }t},\frac{\mathrm{\partial }{J}_0^2}{\mathrm{\partial }t},\frac{\mathrm{\partial }{J}_0^3}{\mathrm{\partial }t})+\mu (\kappa \cos \alpha (\frac{\mathrm{\partial }{J}_1^1}{\mathrm{\partial }{y}_2}-\frac{\mathrm{\partial }{J}_1^2}{\mathrm{\partial }{y}_1})+\kappa \sin \alpha (\frac{\mathrm{\partial }{J}_1^3}{\mathrm{\partial }{y}_1}-\frac{\mathrm{\partial }{J}_1^1}{\mathrm{\partial }{y}_3})\\ & -(\kappa \cos \alpha \frac{\mathrm{\partial }{J}_1^2}{\mathrm{\partial }{y}_1}-\kappa \sin \alpha \frac{\mathrm{\partial }{J}_1^3}{\mathrm{\partial }{y}_1})+{\kappa }^{\prime }(\frac{\mathrm{\partial }{J}_1^2}{\mathrm{\partial }{y}_1}\cos \alpha -\frac{\mathrm{\partial }{J}_1^3}{\mathrm{\partial }{y}_1}\sin \alpha )\\ & -(J_0^2(\kappa \cos \alpha {)}^{\prime }-J_0^3(\kappa \sin \alpha {)}^{\prime }-J_0^2({\kappa }^{\prime }\cos \alpha {)}^{\prime }+J_0^3({\kappa }^{\prime }\sin \alpha {)}^{\prime })\\ & -{\kappa }^2{J}_0^1+\kappa ({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast })(\mathrm{\Delta }{J}_1^1-\kappa \cos \alpha \frac{\mathrm{\partial }{J}_0^1}{\mathrm{\partial }{y}_2}+\kappa \sin \alpha \frac{\mathrm{\partial }{J}_0^1}{\mathrm{\partial }{y}_3})+{\kappa }^2({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast }{)}^2\mathrm{\Delta }{J}_0^1,\\ & -\kappa \cos \alpha \frac{\mathrm{\partial }{J}_1^2}{\mathrm{\partial }{y}_2}+\kappa \sin \alpha \frac{\mathrm{\partial }{J}_1^2}{\mathrm{\partial }{y}_3}+2\kappa ({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast })(-\kappa \cos \alpha \frac{\mathrm{\partial }{J}_0^2}{\mathrm{\partial }{y}_2}+\kappa \sin \alpha \frac{\mathrm{\partial }{J}_0^2}{\mathrm{\partial }{y}_3})\\ & +(2\kappa \frac{\mathrm{\partial }{J}_1^1}{\mathrm{\partial }{y}_1}+{\kappa }^{\prime }{J}_0^1-{\kappa }^2{J}_0^2)\cos \alpha +\kappa \tau J_0^1\sin \alpha +{\kappa }^2\cos \alpha ({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast })\frac{\mathrm{\partial }{J}_0^2}{\mathrm{\partial }{y}_2}\\ & -{\kappa }^2\sin \alpha ({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast })\frac{\mathrm{\partial }{J}_0^2}{\mathrm{\partial }{y}_3},\kappa \sin \alpha \frac{\mathrm{\partial }{J}_1^3}{\mathrm{\partial }{y}_3}-\kappa \cos \alpha \frac{\mathrm{\partial }{J}_1^3}{\mathrm{\partial }{y}_2}+2\kappa ({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast })\kappa \sin \alpha \frac{\mathrm{\partial }{J}_0^3}{\mathrm{\partial }{y}_3}\\ & -2\kappa ({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast })\kappa \cos \alpha \frac{\mathrm{\partial }{J}_0^3}{\mathrm{\partial }{y}_2}+\kappa \tau J_0^1\cos \alpha -(2\kappa \frac{\mathrm{\partial }{J}_1^1}{\mathrm{\partial }{y}_1}+{\kappa }^{\prime }{J}_0^1-{\kappa }^2{J}_0^2)\sin \alpha \\ & +{\kappa }^2\cos \alpha ({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast })\frac{\mathrm{\partial }{J}_0^3}{\mathrm{\partial }{y}_2}-{\kappa }^2\sin \alpha ({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast })\frac{\mathrm{\partial }{J}_0^3}{\mathrm{\partial }{y}_3})\\ & -(\kappa ({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast })\frac{\mathrm{\partial }{j}_1}{\mathrm{\partial }{y}_1}+{\kappa }^2({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast }{)}^2\frac{\mathrm{\partial }{j}_0}{\mathrm{\partial }{y}_1},0,0)\\ & +a(\frac{\mathrm{\partial }{K}_1^3}{\mathrm{\partial }{y}_2}-\frac{\mathrm{\partial }{K}_1^2}{\mathrm{\partial }{y}_3},\frac{\mathrm{\partial }{K}_1^1}{\mathrm{\partial }{y}_3}-\frac{\mathrm{\partial }{K}_1^3}{\mathrm{\partial }{y}_1}+\kappa ({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast })(\frac{\mathrm{\partial }{K}_0^1}{\mathrm{\partial }{y}_3}-\frac{\mathrm{\partial }{K}_0^3}{\mathrm{\partial }{y}_1}),\\ & \frac{\mathrm{\partial }{K}_1^2}{\mathrm{\partial }{y}_1}-\frac{\mathrm{\partial }{K}_1^1}{\mathrm{\partial }{y}_1}+\kappa ({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast })(\frac{\mathrm{\partial }{K}_0^2}{\mathrm{\partial }{y}_1}-\frac{\mathrm{\partial }{K}_0^1}{\mathrm{\partial }{y}_2}),\end{array}$(A14) )).

To ensure the well-posedness of (57(57) $\begin{array}{rl}& -\mu \mathrm{\Delta }{\mathcal{V}}_2+\mathrm{\nabla }{\mathcal{P}}_2=\mathrm{\Pi }(t,0,{\mathcal{V}}_0,{\mathcal{V}}_1,{\mathcal{W}}_0,{\mathcal{W}}_1,{\mathcal{P}}_0,{\mathcal{P}}_1)\text{in}{\mathcal{G}}_0,\\ & \text{div}{\mathcal{V}}_2=\kappa \left(0\right)\cos \alpha \left(0\right){\mathcal{V}}_1^2-\kappa \left(0\right)\sin \alpha \left(0\right){\mathcal{V}}_1^3-\left({\kappa }^{\prime }\right(0\left)\right({\mathbf{\text{e}}}_{\alpha }\left(0\right)\cdot {\mathit{y}}_{\ast })+\kappa (0\left)\tau \right(0\left)\right({\mathbf{\text{e}}}_{\alpha }^{\perp }\left(0\right)\cdot {\mathit{y}}_{\ast }\left)\right){\mathcal{V}}_0^1\\ & -{\kappa }^2\left(0\right)\left({\mathbf{\text{e}}}_{\alpha }\right(0)\cdot {\mathit{y}}_{\ast })\cos \alpha \left(0\right){\mathcal{V}}_0^2+{\kappa }^2\left(0\right)\left({\mathbf{\text{e}}}_{\alpha }\right(0)\cdot {\mathit{y}}_{\ast })\sin \alpha \left(0\right){\mathcal{V}}_0^3\\ & +2\kappa \left(0\right)\left({\mathbf{\text{e}}}_{\alpha }\right(0)\cdot {\mathit{y}}_{\ast })(\kappa \left(0\right)\cos \alpha \left(0\right){\mathcal{V}}_0^2-\kappa \left(0\right)\sin \alpha \left(0\right){\mathcal{V}}_0^3)\\ & -2\kappa \left(0\right)\left({\mathbf{\text{e}}}_{\alpha }\right(0)\cdot {\mathit{y}}_{\ast })\frac{\mathrm{\partial }{\mathcal{V}}_1^1}{\mathrm{\partial }{y}_1}-3{\kappa }^2\left(0\right)\left({\mathbf{\text{e}}}_{\alpha }\right(0)\cdot {\mathit{y}}_{\ast }{)}^2\frac{\mathrm{\partial }{\mathcal{V}}_0^1}{\mathrm{\partial }{y}_1}\text{in}{\mathcal{G}}_0,\\ & {\mathcal{V}}_2=0\text{in}\omega =⟨0,\mathrm{\infty }⟩\times \mathrm{\partial }B,\\ & {\mathcal{V}}_2(t,0,\cdot )=-V_2(t,0,\cdot )-\left(\kappa \right({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast }\left)V_1^1\right(t,0,\cdot ),0,0)-\left({\kappa }^2\right({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast }{)}^2{V}_0^1(t,0,\cdot ),0,0)\\ & -\left(\kappa \right({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast }\left){\mathcal{V}}_1^1\right(t,0,\cdot ),0,0)-\left({\kappa }^2\right({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast }{)}^2{\mathcal{V}}_0^1(t,0,\cdot ),0,0),\end{array}$(57) ), we check the compatibility condition (58) ${\int }_{{\mathcal{G}}_0}\text{div}{\mathcal{V}}_2=-{\int }_B{\mathcal{V}}_2^1(t,0,\cdot ).$(58) Using the relations $\begin{array}{rl}{\mathcal{V}}_1^2& =\text{div}\left(y_2{\mathcal{V}}_1\right)-\kappa \left(0\right)\cos \alpha \left(0\right)y_2{\mathcal{V}}_0^2+\kappa \left(0\right)\sin \alpha \left(0\right)y_2{\mathcal{V}}_0^3\\ & +(2\kappa \left(0\right)\cos \alpha \left(0\right)y_2^2\frac{\mathrm{\partial }{\mathcal{V}}_0^1}{\mathrm{\partial }{y}_1}-2\kappa \left(0\right)\sin \alpha \left(0\right)y_2{y}_3\frac{\mathrm{\partial }{\mathcal{V}}_0^1}{\mathrm{\partial }{y}_1}),\\ {\mathcal{V}}_1^3& =\text{div}\left(y_3{\mathcal{V}}_1\right)-\kappa \left(0\right)\cos \alpha \left(0\right)y_3{\mathcal{V}}_0^2+\kappa \left(0\right)\sin \alpha \left(0\right)y_3{\mathcal{V}}_0^3\\ & +(2\kappa \left(0\right)\cos \alpha \left(0\right)y_2{y}_3\frac{\mathrm{\partial }{\mathcal{V}}_0^1}{\mathrm{\partial }{y}_1}-2\kappa \left(0\right)\sin \alpha \left(0\right)y_3^2\frac{\mathrm{\partial }{\mathcal{V}}_0^1}{\mathrm{\partial }{y}_1}),\end{array}$ and integrating (57(57) $\begin{array}{rl}& -\mu \mathrm{\Delta }{\mathcal{V}}_2+\mathrm{\nabla }{\mathcal{P}}_2=\mathrm{\Pi }(t,0,{\mathcal{V}}_0,{\mathcal{V}}_1,{\mathcal{W}}_0,{\mathcal{W}}_1,{\mathcal{P}}_0,{\mathcal{P}}_1)\text{in}{\mathcal{G}}_0,\\ & \text{div}{\mathcal{V}}_2=\kappa \left(0\right)\cos \alpha \left(0\right){\mathcal{V}}_1^2-\kappa \left(0\right)\sin \alpha \left(0\right){\mathcal{V}}_1^3-\left({\kappa }^{\prime }\right(0\left)\right({\mathbf{\text{e}}}_{\alpha }\left(0\right)\cdot {\mathit{y}}_{\ast })+\kappa (0\left)\tau \right(0\left)\right({\mathbf{\text{e}}}_{\alpha }^{\perp }\left(0\right)\cdot {\mathit{y}}_{\ast }\left)\right){\mathcal{V}}_0^1\\ & -{\kappa }^2\left(0\right)\left({\mathbf{\text{e}}}_{\alpha }\right(0)\cdot {\mathit{y}}_{\ast })\cos \alpha \left(0\right){\mathcal{V}}_0^2+{\kappa }^2\left(0\right)\left({\mathbf{\text{e}}}_{\alpha }\right(0)\cdot {\mathit{y}}_{\ast })\sin \alpha \left(0\right){\mathcal{V}}_0^3\\ & +2\kappa \left(0\right)\left({\mathbf{\text{e}}}_{\alpha }\right(0)\cdot {\mathit{y}}_{\ast })(\kappa \left(0\right)\cos \alpha \left(0\right){\mathcal{V}}_0^2-\kappa \left(0\right)\sin \alpha \left(0\right){\mathcal{V}}_0^3)\\ & -2\kappa \left(0\right)\left({\mathbf{\text{e}}}_{\alpha }\right(0)\cdot {\mathit{y}}_{\ast })\frac{\mathrm{\partial }{\mathcal{V}}_1^1}{\mathrm{\partial }{y}_1}-3{\kappa }^2\left(0\right)\left({\mathbf{\text{e}}}_{\alpha }\right(0)\cdot {\mathit{y}}_{\ast }{)}^2\frac{\mathrm{\partial }{\mathcal{V}}_0^1}{\mathrm{\partial }{y}_1}\text{in}{\mathcal{G}}_0,\\ & {\mathcal{V}}_2=0\text{in}\omega =⟨0,\mathrm{\infty }⟩\times \mathrm{\partial }B,\\ & {\mathcal{V}}_2(t,0,\cdot )=-V_2(t,0,\cdot )-\left(\kappa \right({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast }\left)V_1^1\right(t,0,\cdot ),0,0)-\left({\kappa }^2\right({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast }{)}^2{V}_0^1(t,0,\cdot ),0,0)\\ & -\left(\kappa \right({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast }\left){\mathcal{V}}_1^1\right(t,0,\cdot ),0,0)-\left({\kappa }^2\right({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast }{)}^2{\mathcal{V}}_0^1(t,0,\cdot ),0,0),\end{array}$(57) )₂ over ${\mathcal{G}}_0$, the first two terms can be written as: $\begin{array}{rl}& \kappa \left(0\right)\cos \alpha \left(0\right){\int }_{{\mathcal{G}}_0}{\mathcal{V}}_1^2-\kappa \left(0\right)\sin \alpha \left(0\right){\int }_{{\mathcal{G}}_0}{\mathcal{V}}_1^3=-{\int }_B(\kappa \left(0\right)\cos \alpha \left(0\right)y_2-\kappa \left(0\right)\sin \alpha \left(0\right)y_3){\mathcal{V}}_1^1(t,0,\cdot )\\ & +2{\kappa }^2\left(0\right){\cos }^2\alpha \left(0\right){\int }_{{\mathcal{G}}_0}{y}_2^2\frac{\mathrm{\partial }{\mathcal{V}}_0^1}{\mathrm{\partial }{y}_1}-4\kappa \left(0\right)\sin \alpha \cos \alpha \left(0\right){\int }_{{\mathcal{G}}_0}{y}_2{y}_3\frac{\mathrm{\partial }{\mathcal{V}}_0^1}{\mathrm{\partial }{y}_1}+2{\kappa }^2\left(0\right){\sin }^2\left(0\right)y_3^2\frac{\mathrm{\partial }{\mathcal{V}}_0^1}{\mathrm{\partial }{y}_1}\\ & ={\int }_B(\kappa \left(0\right)\cos \alpha \left(0\right)y_2-\kappa \left(0\right)\sin \alpha \left(0\right)y_3)(V_1^1(t,0,\cdot )+\kappa \left(0\right)\left({\mathbf{\text{e}}}_{\alpha }\right(0)\cdot {\mathit{y}}_{\ast })h_0^t)\\ & +{\int }_B(2{\kappa }^2\left(0\right){\cos }^2\alpha \left(0\right)y_2^2-4\kappa \left(0\right)\sin \alpha \left(0\right)\cos \alpha \left(0\right)y_2{y}_3+2{\kappa }^2\left(0\right){\sin }^2\left(0\right)y_3^2)(V_0^1(t,0,\cdot )-h_0^t).\end{array}$ while other terms in the divergence equations can be written in the following way: $\begin{array}{rl}& -{\int }_{{\mathcal{G}}_0}(({\kappa }^{\prime }\left(0\right)\cos \alpha \left(0\right)+\kappa \left(0\right)\tau \left(0\right)\sin \alpha \left(0\right))y_2+(-{\kappa }^{\prime }\left(0\right)\sin \alpha \left(0\right)+\kappa \left(0\right)\tau \left(0\right)\cos \alpha \left(0\right))y_3){\mathcal{V}}_0^1\\ & =-{\int }_B((({\kappa }^{\prime }\left(0\right)\cos \alpha \left(0\right)+\kappa \left(0\right)\tau \left(0\right)\sin \alpha \left(0\right))y_2+(-{\kappa }^{\prime }\left(0\right)\sin \alpha \left(0\right)+\kappa \left(0\right)\tau \left(0\right)\cos \alpha \left(0\right))y_3)\\ & {\int }_0^{\mathrm{\infty }}{\mathcal{V}}_0^1),\\ & {\int }_{{\mathcal{G}}_0}({\kappa }^2\left(0\right){\cos }^2\alpha \left(0\right)y_2{\mathcal{V}}_0^2-{\kappa }^2\left(0\right)\sin \alpha \cos \alpha \left(0\right)(y_3{\mathcal{V}}_0^2+y_2{\mathcal{V}}_0^3)+{\kappa }^2\left(0\right){\sin }^2\alpha \left(0\right)y_3{\mathcal{V}}_0^3)\\ & ={\int }_B(\frac{{\kappa }^2\left(0\right){\cos }^2\alpha \left(0\right)}{2}{y}_2^2-{\kappa }^2\left(0\right)\sin \alpha \left(0\right)\cos \alpha \left(0\right)y_2{y}_3+\frac{{\kappa }^2\left(0\right){\sin }^2\alpha \left(0\right)}{2}{y}_3^2)(V_0^1(t,0,\cdot )-h_0^t).\end{array}$ Using the fact that ${\mathcal{V}}_0^1,{\mathcal{V}}_1^1$ exponentially decay to zero as $y_1\to \mathrm{\infty }$, we get: $\begin{array}{rl}& -{\int }_{{\mathcal{G}}_0}2\kappa \left(0\right)(\cos \alpha \left(0\right)y_2-\sin \alpha \left(0\right)y_3)\frac{\mathrm{\partial }{\mathcal{V}}_1^1}{\mathrm{\partial }{y}_1}-{\int }_{{\mathcal{G}}_0}3{\kappa }^2\left(0\right)({\cos }^2\alpha \left(0\right)y_2^2-2\sin \alpha \left(0\right)\cos \alpha \left(0\right)y_2{y}_3\\ & +{\sin }^2\left(0\right)y_3^2)\frac{\mathrm{\partial }{\mathcal{V}}_0^1}{\mathrm{\partial }{y}_1}\\ & ={\int }_B(-2\kappa \left(0\right)\cos \alpha \left(0\right)y_2+2\kappa \left(0\right)\sin \alpha \left(0\right)y_3)(V_1^1(t,0,\cdot )+\kappa \left(0\right)\left({\mathbf{\text{e}}}_{\alpha }\right(0)\cdot {\mathit{y}}_{\ast })h_0^t)\\ & +{\int }_B(-3{\kappa }^2\left(0\right){\cos }^2\alpha \left(0\right)y_2+6{\kappa }^2\sin \alpha \left(0\right)\cos \alpha \left(0\right)y_2{y}_3-3{\kappa }^2\left(0\right){\sin }^2\left(0\right)y_3^2)\\ & (V_0^1(t,0,\cdot )-h_0^t).\end{array}$ On the other hand, we have $\begin{array}{rl}& -{\int }_B{\mathcal{V}}_2^1(t,0,\cdot )={\int }_B{V}_2^1(t,0,\cdot )={\int }_B\left(\right|{\mathit{y}}_{\ast }{|}^2-1\left)(B_1\right(t,0)y_2^2+B_2(t,0)y_2{y}_3\\ & +B_3(t,0)y_3^2+{\tilde{B}}_4(t,0)+\frac{1}{4\mu }{p}_1\left(t\right)).\end{array}$ To ensure that the compatibility condition (58(58) ${\int }_{{\mathcal{G}}_0}\text{div}{\mathcal{V}}_2=-{\int }_B{\mathcal{V}}_2^1(t,0,\cdot ).$(58) ), we choose $p_1\left(t\right)=\frac{8\mu }{\pi }(-{\int }_{{\mathcal{G}}_0}\text{div}{\mathcal{V}}_2+{\int }_B\left(\right|{\mathit{y}}_{\ast }{|}^2-1\left)(B_1\right(t,0)y_2^2+B_2(t,0)y_2{y}_3+B_3(t,0)y_3^2+{\tilde{B}}_4(t,0))),$ where ${\tilde{B}}_4(t,x_1)=\frac{1}{4}(\frac{2}{\mu \pi }\frac{\mathrm{d}}{\mathrm{d}t}{F}_0^{\ast }-\frac{\kappa \cos \alpha }{8\mu }{H}_1+\frac{\kappa \sin \alpha }{8\mu }{H}_2+\frac{2{\kappa }^2}{\pi }{F}_0^{\ast }-\frac{a}{\mu }(H_{11}-H_{10}\left)\right)+\frac{1}{32}(A_1+A_3)$.

The existence of a unique solution exponentially decaying to zero corresponding to the problem on the opposite side for the second-order boundary layer correctors $({\mathcal{Y}}_2,{\mathcal{Q}}_2)$ follows analogously, choosing the corresponding $p_2\left(t\right)$ such that the compatibility condition holds.

Remark 4.1

Simplified model

Let us consider a simplified model by assuming the following: $\kappa ,\alpha$ are constants, $\mathbf{\text{f}},\mathbf{\text{g}}$ vanish in the neighbourhood of $x_1=0$ and $x_1=l$, ${\int }_0^l\mathbf{\text{g}}=0$ and there hold the following relations: ${\int }_B{y}_2^2{h}_0^t={\int }_B{y}_2^2{h}_l^t,{\int }_B{y}_2{y}_3{h}_0^t={\int }_B{y}_2{y}_3{h}_l^t,{\int }_B{y}_3^2{h}_0^t={\int }_B{y}_3^2{h}_l^t.$ We also take $P_2(t,x_1)=x_1{p}_{bl}\left(t\right)+p_{div}(t,x_1)$, where $p_{bl}\left(t\right)$ will be obtained from the compatibility conditions for the second-order boundary layer correctors and $p_{div}$ will be chosen such that the ${\epsilon }^4$ term in the divergence equation vanishes, thus improving our final estimate (see Section 4.3 and Section 5).

The following compatibility conditions needs to be fulfilled: (59) $\begin{array}{rl}& {\int }_{{\mathcal{G}}_0}\text{div}{\mathcal{V}}_2=-{\int }_B{\mathcal{V}}_2^1(t,0,\cdot ),\\ & {\int }_{{\mathcal{G}}_l}\text{div}{\mathcal{Y}}_2={\int }_B{\mathcal{Y}}_2^1(t,0,\cdot ).\end{array}$(59) One can easily verify that the Equations (59(59) $\begin{array}{rl}& {\int }_{{\mathcal{G}}_0}\text{div}{\mathcal{V}}_2=-{\int }_B{\mathcal{V}}_2^1(t,0,\cdot ),\\ & {\int }_{{\mathcal{G}}_l}\text{div}{\mathcal{Y}}_2={\int }_B{\mathcal{Y}}_2^1(t,0,\cdot ).\end{array}$(59) )₁ and (59(59) $\begin{array}{rl}& {\int }_{{\mathcal{G}}_0}\text{div}{\mathcal{V}}_2=-{\int }_B{\mathcal{V}}_2^1(t,0,\cdot ),\\ & {\int }_{{\mathcal{G}}_l}\text{div}{\mathcal{Y}}_2={\int }_B{\mathcal{Y}}_2^1(t,0,\cdot ).\end{array}$(59) )₂ are equivalent due to the assumptions of the simplified model, so choosing $p_{bl}\left(t\right)=\frac{8\mu }{\pi }(-{\int }_{{\mathcal{G}}_0}\text{div}{\mathcal{V}}_2+{\int }_B\left(\right|{\mathit{y}}_{\ast }{|}^2-1\left)(B_1\right(t,0)y_2^2+B_2(t,0)y_2{y}_3+B_3(t,0)y_3^2+{\tilde{B}}_4(t,0))),$ where ${\tilde{B}}_4(t,x_1)=\frac{1}{4}(\frac{2}{\mu \pi }\frac{d}{dt}{F}_0^{\ast }-\frac{\kappa \cos \alpha }{8\mu }{H}_1+\frac{\kappa \sin \alpha }{8\mu }{H}_2+\frac{2{\kappa }^2}{\pi }{F}_0^{\ast }-\frac{a}{\mu }(H_{11}-H_{10}\left)\right)+\frac{1}{32}(A_1+A_3)$, both the compatibility conditions (59(59) $\begin{array}{rl}& {\int }_{{\mathcal{G}}_0}\text{div}{\mathcal{V}}_2=-{\int }_B{\mathcal{V}}_2^1(t,0,\cdot ),\\ & {\int }_{{\mathcal{G}}_l}\text{div}{\mathcal{Y}}_2={\int }_B{\mathcal{Y}}_2^1(t,0,\cdot ).\end{array}$(59) ) are satisfied.

We will address these considerations in Section 4.3 as they are essential in order to improve the divergence estimate.

4.2.2. Boundary layer for the microrotation

As for the velocity and pressure, we need to correct the microrotation approximation to satisfy the conditions on the end of the pipe ${\mathrm{\Sigma }}_{\epsilon }^i$, i=0,l.

Plugging the boundary layer correctors given by (50(50) $\begin{array}{rl}{\mathbf{\text{U}}}_{\epsilon ,bl,0}^{\left[2\right]}(t,\mathit{x})& ={\epsilon }^2{\mathcal{V}}_0(t,\frac{x_1}{\epsilon },\frac{{\mathit{x}}_{\ast }}{\epsilon })+{\epsilon }^3{\mathcal{V}}_1(t,\frac{x_1}{\epsilon },\frac{{\mathit{x}}_{\ast }}{\epsilon })\\ & +{\epsilon }^4{\mathcal{V}}_2(t,\frac{x_1}{\epsilon },\frac{{\mathit{x}}_{\ast }}{\epsilon }),{\mathcal{V}}_j={\mathcal{V}}_j^1{\mathbf{\text{a}}}^1+{\mathcal{V}}_j^2{\mathbf{\text{a}}}^2+{\mathcal{V}}_j^3{\mathbf{\text{a}}}^3,j=0,1,2,\\ {\mathbf{\text{W}}}_{\epsilon ,bl,0}^{\left[2\right]}(t,\mathit{x})& ={\epsilon }^2{\mathcal{W}}_0(t,\frac{x_1}{\epsilon },\frac{{\mathit{x}}_{\ast }}{\epsilon })+{\epsilon }^3{\mathcal{W}}_1(t,\frac{x_1}{\epsilon },\frac{{\mathit{x}}_{\ast }}{\epsilon })\\ & +{\epsilon }^4{\mathcal{W}}_2(t,\frac{x_1}{\epsilon },\frac{{\mathit{x}}_{\ast }}{\epsilon }),{\mathcal{W}}_j={\mathcal{W}}_j^1{\mathbf{\text{a}}}^1+{\mathcal{W}}_j^2{\mathbf{\text{a}}}^2+{\mathcal{W}}_j^3{\mathbf{\text{a}}}^3,j=0,1,2,\\ P_{\epsilon ,bl,0}^{\left[2\right]}(t,\mathit{x})& =\epsilon {\mathcal{P}}_0(t,\frac{x_1}{\epsilon },\frac{{\mathit{x}}_{\ast }}{\epsilon })+{\epsilon }^2{\mathcal{P}}_1(t,\frac{x_1}{\epsilon },\frac{{\mathit{x}}_{\ast }}{\epsilon })+{\epsilon }^3{\mathcal{P}}_2(t,\frac{x_1}{\epsilon },\frac{{\mathit{x}}_{\ast }}{\epsilon }),\end{array}$(50) ) into the angular momentum equation (16(16) $\begin{array}{rl}& -\beta (\frac{{\mathrm{\partial }}^2{W}_{\epsilon }^1}{\mathrm{\partial }{x}_1\mathrm{\partial }{x}_3}-\kappa (\frac{\mathrm{\partial }{W}_{\epsilon }^2}{\mathrm{\partial }{x}_3}\cos \alpha -\frac{\mathrm{\partial }{W}_{\epsilon }^3}{\mathrm{\partial }{x}_3}\sin \alpha )+\kappa \tau W_{\epsilon }^1\cos \alpha \\ & -({\kappa }^{\prime }{W}_{\epsilon }^1+{\kappa }^2\cos \alpha W_{\epsilon }^2-{\kappa }^2\sin \alpha W_{\epsilon }^3)\sin \alpha ){\mathbf{\text{a}}}^3\\ & -\beta (2\kappa (\frac{\mathrm{\partial }{W}_{\epsilon }^1}{\mathrm{\partial }{x}_1}-\kappa (W_{\epsilon }^2\cos \alpha -W_{\epsilon }^3\sin \alpha ))\sin \alpha \\ & +{\kappa }^{\prime }({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{x}}_{\ast })\frac{\mathrm{\partial }{W}_{\epsilon }^1}{\mathrm{\partial }{x}_3}+\kappa \tau ({\mathbf{\text{e}}}_{\alpha }^{\perp }\cdot {\mathit{x}}_{\ast })\frac{\mathrm{\partial }{W}_{\epsilon }^1}{\mathrm{\partial }{x}_3}+{\kappa }^2({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{x}}_{\ast })\cos \alpha \frac{\mathrm{\partial }{W}_{\epsilon }^2}{\mathrm{\partial }{x}_3}){\mathbf{\text{a}}}^3\\ & -\beta (-{\kappa }^2({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{x}}_{\ast })\frac{\mathrm{\partial }{W}_{\epsilon }^3}{\mathrm{\partial }{x}_3}\sin \alpha +\frac{{\mathrm{\partial }}^2{W}_{\epsilon }^2}{\mathrm{\partial }{x}_2\mathrm{\partial }{x}_3}+\frac{{\mathrm{\partial }}^2{W}_{\epsilon }^3}{\mathrm{\partial }{x}_3^2}+2\kappa ({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{x}}_{\ast })\\ & (\frac{{\mathrm{\partial }}^2{W}_{\epsilon }^1}{\mathrm{\partial }{x}_1\mathrm{\partial }{x}_3}-\kappa (\frac{\mathrm{\partial }{W}_{\epsilon }^2}{\mathrm{\partial }{x}_3}\cos \alpha -\frac{\mathrm{\partial }{W}_{\epsilon }^3}{\mathrm{\partial }{x}_3}\sin \alpha ))]{\mathbf{\text{a}}}^3\\ & +2a(1+\kappa ({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{x}}_{\ast })+\cdots )W_{\epsilon }^1\mathbf{\text{t}}+2a{W}_{\epsilon }^2{\mathbf{\text{a}}}^2+2a{W}_{\epsilon }^3{\mathbf{\text{a}}}^3\\ & =a(\frac{\mathrm{\partial }{V}_{\epsilon }^3}{\mathrm{\partial }{x}_2}-\frac{\mathrm{\partial }{V}_{\epsilon }^2}{\mathrm{\partial }{x}_3})\mathbf{\text{t}}+a((1+\kappa ({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{x}}_{\ast })+\cdots )(\frac{\mathrm{\partial }{V}_{\epsilon }^1}{\mathrm{\partial }{x}_3}-\frac{\mathrm{\partial }{V}_{\epsilon }^3}{\mathrm{\partial }{x}_1})){\mathbf{\text{a}}}^2\\ & +a((1+\kappa ({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{x}}_{\ast })+\cdots )(\frac{\mathrm{\partial }{V}_{\epsilon }^2}{\mathrm{\partial }{x}_1}-\frac{\mathrm{\partial }{V}_{\epsilon }^1}{\mathrm{\partial }{x}_2})){\mathbf{\text{a}}}^3+g_1\mathbf{\text{t}}+(g_2\cos \alpha +g_3\sin \alpha ){\mathbf{\text{a}}}^2\\ & +(g_3\cos \alpha -g_2\sin \alpha ){\mathbf{\text{a}}}^3.\end{array}$(16) ) and collecting the ${\epsilon }^0$ terms, we obtain the problem for the microrotation zero-order boundary layer approximation at $x_1=0$: (60) $\begin{array}{rl}& -\delta \mathrm{\Delta }{\mathcal{W}}_0-\beta \mathrm{\nabla }\text{div}{\mathcal{W}}_0=0\text{in}{\mathcal{G}}_0,\\ & {\mathcal{W}}_0=0\text{on}\omega =⟨0,\mathrm{\infty }⟩\times \mathrm{\partial }B,\\ & {\mathcal{W}}_0(t,0,\cdot )=-W_0(t,0,\cdot ).\end{array}$(60) The existence of the solution ${\mathcal{W}}_0(t,\cdot )\in {\stackrel{\circ }{W}}^{1,2}\left({\mathcal{G}}_0\right)$ exponentially decaying to zero as $y_1\to \mathrm{\infty }$ can be easily proven (see [25]).

The first-order boundary layer microrotation corrector ${\mathcal{W}}_1$ is the solution of the following system: (61) $\begin{array}{rl}& -\delta {\mathcal{W}}_1-\beta \mathrm{\nabla }\text{div}{\mathcal{W}}_1=\mathrm{\Theta }(t,0,{\mathcal{V}}_0,{\mathcal{W}}_0)\text{in}{\mathcal{G}}_0,\\ & {\mathcal{W}}_1=0\text{on}\omega =⟨0,\mathrm{\infty }⟩\times \mathrm{\partial }B,\\ & {\mathcal{W}}_1(t,0,\cdot )=-W_1(t,0,\cdot )-\left(\kappa \right({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast }\left)W_0^1\right(t,0,\cdot ),0,0)-\left(\kappa \right({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast }\left){\mathcal{W}}_0^1\right(t,0,\cdot ),0,0),\end{array}$(61) where $\mathrm{\Theta }(t,x_1,\mathbf{\text{J}},\mathbf{\text{K}})$ is an exponentially decreasing function given in the appendix (see (A15(A15) $\begin{array}{rl}\mathrm{\Theta }(t,x_1,\mathbf{\text{J}},\mathbf{\text{K}})& =\delta (\kappa \cos \alpha (\frac{\mathrm{\partial }{K}^1}{\mathrm{\partial }{y}_2}-\frac{\mathrm{\partial }{K}^2}{\mathrm{\partial }{y}_1})+\kappa \sin \alpha (\frac{\mathrm{\partial }{K}^3}{\mathrm{\partial }{y}_1}-\frac{\mathrm{\partial }{K}^1}{\mathrm{\partial }{y}_3})-\kappa (\frac{\mathrm{\partial }{K}^2}{\mathrm{\partial }{y}_1}\cos \alpha -\frac{\mathrm{\partial }{K}^3}{\mathrm{\partial }{y}_1}\sin \alpha )\\ & +{\kappa }^{\prime }(\frac{\mathrm{\partial }{K}^2}{\mathrm{\partial }{y}_1}\cos \alpha -\frac{\mathrm{\partial }{K}^3}{\mathrm{\partial }{y}_1}\sin \alpha )+\kappa ({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast })\mathrm{\Delta }{K}^1,-\kappa \cos \alpha \frac{\mathrm{\partial }{K}^2}{\mathrm{\partial }{y}_2}+\kappa \sin \alpha \frac{\mathrm{\partial }{K}^2}{\mathrm{\partial }{y}_3}+2\kappa \cos \alpha \frac{\mathrm{\partial }{K}^1}{\mathrm{\partial }{y}_1},\\ & \kappa \sin \alpha \frac{\mathrm{\partial }{K}^3}{\mathrm{\partial }{y}_3}-\kappa \cos \alpha \frac{\mathrm{\partial }{K}^3}{\mathrm{\partial }{y}_2}-2\kappa \sin \alpha \frac{\mathrm{\partial }{K}^1}{\mathrm{\partial }{y}_1})+\beta (-\kappa \cos \alpha \frac{\mathrm{\partial }{K}^2}{\mathrm{\partial }{y}_1}+\kappa \sin \alpha \frac{\mathrm{\partial }{K}^3}{\mathrm{\partial }{y}_1},\\ & -\kappa (\frac{\mathrm{\partial }{K}^2}{\mathrm{\partial }{y}_2}\cos \alpha -\frac{\mathrm{\partial }{K}^3}{\mathrm{\partial }{y}_2}\sin \alpha )+2\kappa \cos \alpha \frac{\mathrm{\partial }{K}^1}{\mathrm{\partial }{y}_1},-\kappa (\frac{\mathrm{\partial }{K}^2}{\mathrm{\partial }{y}_3}\cos \alpha -\frac{\mathrm{\partial }{K}^3}{\mathrm{\partial }{y}_3}\sin \alpha )-2\kappa \sin \alpha \frac{\mathrm{\partial }{K}^1}{\mathrm{\partial }{y}_1})\\ & +a(\frac{\mathrm{\partial }{J}^3}{\mathrm{\partial }{y}_2}-\frac{\mathrm{\partial }{J}^2}{\mathrm{\partial }{y}_3},\frac{\mathrm{\partial }{J}^1}{\mathrm{\partial }{y}_3}-\frac{\mathrm{\partial }{J}^3}{\mathrm{\partial }{y}_1},\frac{\mathrm{\partial }{J}^2}{\mathrm{\partial }{y}_1}-\frac{\mathrm{\partial }{J}^1}{\mathrm{\partial }{y}_2}),\end{array}$(A15) )). The existence of the unique exponentially decreasing solution ${\mathcal{W}}_1(t,\cdot )\in {\stackrel{\circ }{W}}^{1,2}\left({\mathcal{G}}_0\right)$ is established in the same manner as for ${\mathcal{W}}_0$.

Finally, the system for the second-order boundary layer microrotation corrector ${\mathcal{W}}_2$ is given with (62) $\begin{array}{rl}& -\delta {\mathcal{W}}_2-\beta \mathrm{\nabla }\text{div}{\mathcal{W}}_2=\mathrm{\Lambda }(t,0,{\mathcal{V}}_0,{\mathcal{V}}_1,{\mathcal{W}}_0,{\mathcal{W}}_1)\text{in}{\mathcal{G}}_0,\\ & {\mathcal{W}}_2=0\text{on}\omega =⟨0,\mathrm{\infty }⟩\times \mathrm{\partial }B,\\ & {\mathcal{W}}_2(t,0,\cdot )=-W_2(t,0,\cdot )-\left(\kappa \right({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast }\left)W_1^1\right(t,0,\cdot ),0,0)-\left({\kappa }^2\right({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast }{)}^2{W}_0^1(t,0,\cdot ),0,0)\\ & -\left(\kappa \right({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast }\left){\mathcal{W}}_1^1\right(t,0,\cdot ),0,0)-\left({\kappa }^2\right({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast }{)}^2{\mathcal{W}}_0^1(t,0,\cdot ),0,0),\end{array}$(62) where $\mathrm{\Theta }(t,x_1,{\mathbf{\text{J}}}_0,{\mathbf{\text{J}}}_1,{\mathbf{\text{K}}}_0,{\mathbf{\text{K}}}_1)$ is an exponentially decreasing function given in the appendix (see (A16(A16) $\begin{array}{rl}& +{\kappa }^2({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast })(\cos \alpha \frac{\mathrm{\partial }{K}_0^2}{\mathrm{\partial }{y}_3}-\sin \alpha \frac{\mathrm{\partial }{K}_0^2}{\mathrm{\partial }{y}_3})+2\kappa ({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast })\frac{{\mathrm{\partial }}^2{K}_1^1}{\mathrm{\partial }{y}_1\mathrm{\partial }{y}_3}\\ & -2{\kappa }^2({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast })(\frac{\mathrm{\partial }{K}_0^2}{\mathrm{\partial }{y}_3}\cos \alpha -\frac{\mathrm{\partial }{K}_0^3}{\mathrm{\partial }{y}_3}\sin \alpha ))+2a(K_0^1,K_0^2,K_0^3)\\ & +a(\frac{\mathrm{\partial }{J}_1^3}{\mathrm{\partial }{y}_2}-\frac{\mathrm{\partial }{J}_1^2}{\mathrm{\partial }{y}_3},\frac{\mathrm{\partial }{J}_1^1}{\mathrm{\partial }{y}_3}-\frac{\mathrm{\partial }{J}_1^3}{\mathrm{\partial }{y}_1}+\kappa ({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast })(\frac{\mathrm{\partial }{J}_0^1}{\mathrm{\partial }{y}_3}-\frac{\mathrm{\partial }{J}_0^3}{\mathrm{\partial }{y}_1}),\frac{\mathrm{\partial }{J}_1^2}{\mathrm{\partial }{y}_1}-\frac{\mathrm{\partial }{J}_1^1}{\mathrm{\partial }{y}_2}\\ & +\kappa ({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast })(\frac{\mathrm{\partial }{J}_0^2}{\mathrm{\partial }{y}_1}-\frac{\mathrm{\partial }{J}_0^1}{\mathrm{\partial }{y}_2})),\end{array}$(A16) )). The existence of a unique exponentially decreasing solution ${\mathcal{W}}_2(t,\cdot )\in {\stackrel{\circ }{W}}^{1,2}\left({\mathcal{G}}_0\right)$ is established as for ${\mathcal{W}}_0$ and ${\mathcal{W}}_1$.

The construction of the boundary layer correctors ${\mathcal{Z}}_j,j=0,1,2$ on the opposite side $x_1=l$ can be done in the same way and the exponential decay easily follows.

4.3. Divergence correction

Collecting the regular part of the approximation given by (49(49) $\begin{array}{rl}{\mathbf{\text{U}}}_{\epsilon ,reg}^{\left[2\right]}(t,\mathit{x})& ={\epsilon }^2{V}_0(t,\frac{{\mathit{x}}_{\ast }}{\epsilon })+{\epsilon }^3{V}_1(t,x_1,\frac{{\mathit{x}}_{\ast }}{\epsilon })+{\epsilon }^4{V}_2(t,x_1,\frac{{\mathit{x}}_{\ast }}{\epsilon }),\\ V_j& =V_j^1{\mathbf{\text{a}}}^1+V_j^2{\mathbf{\text{a}}}^2+V_j^3{\mathbf{\text{a}}}^3,j=0,1,2,\\ {\mathbf{\text{W}}}_{\epsilon ,reg}^{\left[2\right]}(t,\mathit{x})& ={\epsilon }^2{W}_0(t,x_1\frac{{\mathit{x}}_{\ast }}{\epsilon })+{\epsilon }^3{W}_1(t,x_1,\frac{{\mathit{x}}_{\ast }}{\epsilon })+{\epsilon }^4{W}_2(t,x_1,\frac{{\mathit{x}}_{\ast }}{\epsilon }),\\ W_j& =W_j^1{\mathbf{\text{a}}}^1+W_j^2{\mathbf{\text{a}}}^2+W_j^3{\mathbf{\text{a}}}^3,j=0,1,2,\\ P_{\epsilon ,reg}^{\left[3\right]}(t,\mathit{x})& =P_0(t,x_1)+\epsilon P_1(t,x_1,\frac{{\mathit{x}}_{\ast }}{\epsilon })+{\epsilon }^2{P}_2(t,x_1,\frac{{\mathit{x}}_{\ast }}{\epsilon })+{\epsilon }^3{P}_3(t,x_1,\frac{{\mathit{x}}_{\ast }}{\epsilon })\end{array}$(49) ) and the boundary layer correctors at $x_1=0$ and $x_1=l$ given by (50(50) $\begin{array}{rl}{\mathbf{\text{U}}}_{\epsilon ,bl,0}^{\left[2\right]}(t,\mathit{x})& ={\epsilon }^2{\mathcal{V}}_0(t,\frac{x_1}{\epsilon },\frac{{\mathit{x}}_{\ast }}{\epsilon })+{\epsilon }^3{\mathcal{V}}_1(t,\frac{x_1}{\epsilon },\frac{{\mathit{x}}_{\ast }}{\epsilon })\\ & +{\epsilon }^4{\mathcal{V}}_2(t,\frac{x_1}{\epsilon },\frac{{\mathit{x}}_{\ast }}{\epsilon }),{\mathcal{V}}_j={\mathcal{V}}_j^1{\mathbf{\text{a}}}^1+{\mathcal{V}}_j^2{\mathbf{\text{a}}}^2+{\mathcal{V}}_j^3{\mathbf{\text{a}}}^3,j=0,1,2,\\ {\mathbf{\text{W}}}_{\epsilon ,bl,0}^{\left[2\right]}(t,\mathit{x})& ={\epsilon }^2{\mathcal{W}}_0(t,\frac{x_1}{\epsilon },\frac{{\mathit{x}}_{\ast }}{\epsilon })+{\epsilon }^3{\mathcal{W}}_1(t,\frac{x_1}{\epsilon },\frac{{\mathit{x}}_{\ast }}{\epsilon })\\ & +{\epsilon }^4{\mathcal{W}}_2(t,\frac{x_1}{\epsilon },\frac{{\mathit{x}}_{\ast }}{\epsilon }),{\mathcal{W}}_j={\mathcal{W}}_j^1{\mathbf{\text{a}}}^1+{\mathcal{W}}_j^2{\mathbf{\text{a}}}^2+{\mathcal{W}}_j^3{\mathbf{\text{a}}}^3,j=0,1,2,\\ P_{\epsilon ,bl,0}^{\left[2\right]}(t,\mathit{x})& =\epsilon {\mathcal{P}}_0(t,\frac{x_1}{\epsilon },\frac{{\mathit{x}}_{\ast }}{\epsilon })+{\epsilon }^2{\mathcal{P}}_1(t,\frac{x_1}{\epsilon },\frac{{\mathit{x}}_{\ast }}{\epsilon })+{\epsilon }^3{\mathcal{P}}_2(t,\frac{x_1}{\epsilon },\frac{{\mathit{x}}_{\ast }}{\epsilon }),\end{array}$(50) )–(51(51) $\begin{array}{rl}{\mathbf{\text{U}}}_{\epsilon ,bl,l}^{\left[2\right]}(t,\mathit{x})& ={\epsilon }^2{\mathcal{Y}}_0(t,\frac{x_1-l}{\epsilon },\frac{{\mathit{x}}_{\ast }}{\epsilon })+{\epsilon }^3{\mathcal{Y}}_1(t,\frac{x_1-l}{\epsilon },\frac{{\mathit{x}}_{\ast }}{\epsilon })\\ & +{\epsilon }^4{\mathcal{Y}}_2(t,\frac{x_1-l}{\epsilon },\frac{{\mathit{x}}_{\ast }}{\epsilon }),{\mathcal{Y}}_j={\mathcal{Y}}_j{\mathbf{\text{a}}}^1+{\mathcal{Y}}_j{\mathbf{\text{a}}}^2+{\mathcal{Y}}_j{\mathbf{\text{a}}}^3,j=0,1,2,\\ {\mathbf{\text{W}}}_{\epsilon ,bl,l}^{\left[2\right]}(t,\mathit{x})& ={\epsilon }^2{\mathcal{Z}}_0(t,\frac{x_1-l}{\epsilon },\frac{{\mathit{x}}_{\ast }}{\epsilon })+{\epsilon }^3{\mathcal{Z}}_1(t,\frac{x_1-l}{\epsilon },\frac{{\mathit{x}}_{\ast }}{\epsilon })\\ & +{\epsilon }^4{\mathcal{Z}}_2(t,\frac{x_1-l}{\epsilon },\frac{{\mathit{x}}_{\ast }}{\epsilon }),{\mathcal{Z}}_j={\mathcal{Z}}_j^1{\mathbf{\text{a}}}^1+{\mathcal{Z}}_j^2{\mathbf{\text{a}}}^2+{\mathcal{Z}}_j^3{\mathbf{\text{a}}}^3,j=0,1,2,\\ P_{\epsilon ,bl,l}^{\left[2\right]}(t,\mathit{x})& =\epsilon {\mathcal{Q}}_0(t,\frac{x_1-l}{\epsilon },\frac{{\mathit{x}}_{\ast }}{\epsilon })+{\epsilon }^2{\mathcal{Q}}_1(t,\frac{x_1-l}{\epsilon },\frac{{\mathit{x}}_{\ast }}{\epsilon })+{\epsilon }^3{\mathcal{Q}}_2(t,\frac{x_1-l}{\epsilon },\frac{{\mathit{x}}_{\ast }}{\epsilon }).\end{array}$(51) ), we define our approximation as: (63) $\begin{array}{rl}{\mathbf{\text{U}}}_{\epsilon }^{\left[2\right]}(t,\mathit{x})& ={\mathbf{\text{U}}}_{\epsilon ,reg}^{\left[2\right]}(t,\mathit{x})+{\mathbf{\text{U}}}_{\epsilon ,bl,0}^{\left[2\right]}(t,\mathit{x})+{\mathbf{\text{U}}}_{\epsilon ,bl,l}^{\left[2\right]}(t,\mathit{x})\\ & ={\epsilon }^2(V_0(t,\frac{{\mathit{x}}_{\ast }}{\epsilon })+{\mathcal{V}}_0(t,\frac{x_1}{\epsilon },\frac{{\mathit{x}}_{\ast }}{\epsilon })+{\mathcal{Y}}_0(t,\frac{x_1-l}{\epsilon },\frac{{\mathit{x}}_{\ast }}{\epsilon }))\\ & +{\epsilon }^3(V_1(t,x_1,\frac{{\mathit{x}}_{\ast }}{\epsilon })+{\mathcal{V}}_1(t,\frac{x_1}{\epsilon },\frac{{\mathit{x}}_{\ast }}{\epsilon })+{\mathcal{Y}}_1(t,\frac{x_1-l}{\epsilon },\frac{{\mathit{x}}_{\ast }}{\epsilon }))\\ & +{\epsilon }^4(V_2(t,x_1,\frac{{\mathit{x}}_{\ast }}{\epsilon })+{\mathcal{V}}_2(t,\frac{x_1}{\epsilon },\frac{{\mathit{x}}_{\ast }}{\epsilon })+{\mathcal{Y}}_2(t,\frac{x_1-l}{\epsilon },\frac{{\mathit{x}}_{\ast }}{\epsilon })),\end{array}$(63) (64) $\begin{array}{rl}{\mathbf{\text{W}}}_{\epsilon }^{\left[2\right]}(t,\mathit{x})& ={\mathbf{\text{W}}}_{\epsilon ,reg}^{\left[2\right]}(t,\mathit{x})+{\mathbf{\text{W}}}_{\epsilon ,bl,0}^{\left[2\right]}(t,\mathit{x})+{\mathbf{\text{W}}}_{\epsilon ,bl,l}^{\left[2\right]}(t,\mathit{x})\\ & ={\epsilon }^2(W_0(t,x_1\frac{{\mathit{x}}_{\ast }}{\epsilon })+{\mathcal{W}}_0(t,\frac{x_1}{\epsilon },\frac{{\mathit{x}}_{\ast }}{\epsilon })+{\mathcal{Z}}_0(t,\frac{x_1-l}{\epsilon },\frac{{\mathit{x}}_{\ast }}{\epsilon }))\\ & +{\epsilon }^3(W_1(t,x_1,\frac{{\mathit{x}}_{\ast }}{\epsilon })+{\mathcal{W}}_1(t,\frac{x_1}{\epsilon },\frac{{\mathit{x}}_{\ast }}{\epsilon })+{\mathcal{Z}}_1(t,\frac{x_1-l}{\epsilon },\frac{{\mathit{x}}_{\ast }}{\epsilon }))\\ & +{\epsilon }^4(W_2(t,x_1,\frac{{\mathit{x}}_{\ast }}{\epsilon })+{\mathcal{W}}_2(t,\frac{x_1}{\epsilon },\frac{{\mathit{x}}_{\ast }}{\epsilon })+{\mathcal{Z}}_2(t,\frac{x_1-l}{\epsilon },\frac{{\mathit{x}}_{\ast }}{\epsilon })),\end{array}$(64) (65) $\begin{array}{rl}{P}_{\epsilon }^{\left[2\right]}(t,\mathit{x})& =P_{\epsilon ,reg}^{\left[3\right]}(t,\mathit{x})+P_{\epsilon ,bl,0}^{\left[2\right]}(t,\mathit{x})+P_{\epsilon ,bl,l}^{\left[2\right]}(t,\mathit{x})\\ & =P_0(t,x_1)+\epsilon (P_1(t,x_1,\frac{{\mathit{x}}_{\ast }}{\epsilon })+{\mathcal{P}}_0(t,\frac{x_1}{\epsilon },\frac{{\mathit{x}}_{\ast }}{\epsilon })+{\mathcal{Q}}_0(t,\frac{x_1-l}{\epsilon },\frac{{\mathit{x}}_{\ast }}{\epsilon }))\\ & +{\epsilon }^2(P_2(t,x_1,\frac{{\mathit{x}}_{\ast }}{\epsilon })+{\mathcal{P}}_1(t,\frac{x_1}{\epsilon },\frac{{\mathit{x}}_{\ast }}{\epsilon })+{\mathcal{Q}}_1(t,\frac{x_1-l}{\epsilon },\frac{{\mathit{x}}_{\ast }}{\epsilon })),\\ & +{\epsilon }^3(P_3(t,x_1,\frac{{\mathit{x}}_{\ast }}{\epsilon })+{\mathcal{P}}_2(t,\frac{x_1}{\epsilon },\frac{{\mathit{x}}_{\ast }}{\epsilon })+{\mathcal{Q}}_2(t,\frac{x_1-l}{\epsilon },\frac{{\mathit{x}}_{\ast }}{\epsilon })),\end{array}$(65) (66) ${\mathbf{\text{u}}}_{\epsilon }^{\left[2\right]}(t,\tilde{\mathit{x}})={\mathbf{\text{U}}}_{\epsilon }^{\left[2\right]}(t,\mathit{x}),{\mathbf{\text{w}}}_{\epsilon }^{\left[2\right]}(t,\tilde{\mathit{x}})={\mathbf{\text{W}}}_{\epsilon }^{\left[2\right]}(t,\mathit{x}),p_{\epsilon }^{\left[2\right]}(t,\tilde{\mathit{x}})=P_{\epsilon }^{\left[2\right]}(t,\mathit{x}),\tilde{\mathit{x}}={\mathrm{\Phi }}_{\epsilon }^{\alpha }\left(\mathit{x}\right).$(66) where (67) $\begin{array}{rl}{V}_j& =V_j^1{\mathbf{\text{a}}}^1+V_j^2{\mathbf{\text{a}}}^2+V_j^3{\mathbf{\text{a}}}^3,{\mathcal{V}}_j={\mathcal{V}}_j^1{\mathbf{\text{a}}}^1+{\mathcal{V}}_j^2{\mathbf{\text{a}}}^2+{\mathcal{V}}_j^3{\mathbf{\text{a}}}^3,{\mathcal{Y}}_j={\mathcal{Y}}_j^1{\mathbf{\text{a}}}^1+{\mathcal{Y}}_j^2{\mathbf{\text{a}}}^2+{\mathcal{Y}}_j^3{\mathbf{\text{a}}}^3,j=0,1,2,\\ W_j& =W_j^1{\mathbf{\text{a}}}^1+W_j^2{\mathbf{\text{a}}}^2+W_j^3{\mathbf{\text{a}}}^3,{\mathcal{W}}_j={\mathcal{W}}_j^1{\mathbf{\text{a}}}^1+{\mathcal{W}}_j^2{\mathbf{\text{a}}}^2+{\mathcal{W}}_j^3{\mathbf{\text{a}}}^3,{\mathcal{Z}}_j={\mathcal{Z}}_j^1{\mathbf{\text{a}}}^1+{\mathcal{Z}}_j^2{\mathbf{\text{a}}}^2+{\mathcal{Z}}_j^3{\mathbf{\text{a}}}^3,\\ & j=0,1,2.\end{array}$(67)

It is well-known that the incompresibility equation need one more corrector than the momentum and angular momentum equation (see e.g. [9]). Therefore, we need to correct the residual in the divergence equation. Plugging the expansion (63(63) $\begin{array}{rl}{\mathbf{\text{U}}}_{\epsilon }^{\left[2\right]}(t,\mathit{x})& ={\mathbf{\text{U}}}_{\epsilon ,reg}^{\left[2\right]}(t,\mathit{x})+{\mathbf{\text{U}}}_{\epsilon ,bl,0}^{\left[2\right]}(t,\mathit{x})+{\mathbf{\text{U}}}_{\epsilon ,bl,l}^{\left[2\right]}(t,\mathit{x})\\ & ={\epsilon }^2(V_0(t,\frac{{\mathit{x}}_{\ast }}{\epsilon })+{\mathcal{V}}_0(t,\frac{x_1}{\epsilon },\frac{{\mathit{x}}_{\ast }}{\epsilon })+{\mathcal{Y}}_0(t,\frac{x_1-l}{\epsilon },\frac{{\mathit{x}}_{\ast }}{\epsilon }))\\ & +{\epsilon }^3(V_1(t,x_1,\frac{{\mathit{x}}_{\ast }}{\epsilon })+{\mathcal{V}}_1(t,\frac{x_1}{\epsilon },\frac{{\mathit{x}}_{\ast }}{\epsilon })+{\mathcal{Y}}_1(t,\frac{x_1-l}{\epsilon },\frac{{\mathit{x}}_{\ast }}{\epsilon }))\\ & +{\epsilon }^4(V_2(t,x_1,\frac{{\mathit{x}}_{\ast }}{\epsilon })+{\mathcal{V}}_2(t,\frac{x_1}{\epsilon },\frac{{\mathit{x}}_{\ast }}{\epsilon })+{\mathcal{Y}}_2(t,\frac{x_1-l}{\epsilon },\frac{{\mathit{x}}_{\ast }}{\epsilon })),\end{array}$(63) ) into the divergence Equation (15(15) $\begin{array}{rl}& [\frac{1}{1-\kappa ({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{x}}_{\ast })}{]}^2(\frac{\mathrm{\partial }{V}_{\epsilon }^1}{\mathrm{\partial }{x}_1}-\kappa (V_{\epsilon }^2\cos \alpha -V_{\epsilon }^3\sin \alpha )+\left({\kappa }^{\prime }\right({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{x}}_{\ast })+\kappa \tau ({\mathbf{\text{e}}}_{\alpha }^{\perp }\cdot {\mathit{x}}_{\ast }\left)\right)V_{\epsilon }^1\\ & +\kappa ({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{x}}_{\ast })\left({\kappa }^{\prime }\right({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{x}}_{\ast })+\kappa \tau ({\mathbf{\text{e}}}_{\alpha }^{\perp }\cdot {\mathit{x}}_{\ast }\left)\right)V_{\epsilon }^1+{\kappa }^2({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{x}}_{\ast })\cos \alpha V_{\epsilon }^2-{\kappa }^2({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{x}}_{\ast })\sin \alpha V_{\epsilon }^3)\\ & +\frac{\mathrm{\partial }{V}_{\epsilon }^2}{\mathrm{\partial }{x}_2}+\frac{\mathrm{\partial }{V}_{\epsilon }^3}{\mathrm{\partial }{x}_3}=\frac{\mathrm{\partial }{V}_{\epsilon }^1}{\mathrm{\partial }{x}_1}-\kappa (V_{\epsilon }^2\cos \alpha -V_{\epsilon }^3\sin \alpha )+\left({\kappa }^{\prime }\right({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{x}}_{\ast })+\kappa \tau ({\mathbf{\text{e}}}_{\alpha }^{\perp }\cdot {\mathit{x}}_{\ast }\left)\right)V_{\epsilon }^1\\ & +\kappa ({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{x}}_{\ast })\left({\kappa }^{\prime }\right({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{x}}_{\ast })+\kappa \tau ({\mathbf{\text{e}}}_{\alpha }^{\perp }\cdot {\mathit{x}}_{\ast }\left)\right)V_{\epsilon }^1+{\kappa }^2({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{x}}_{\ast })\cos \alpha V_{\epsilon }^2-{\kappa }^2({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{x}}_{\ast })\sin \alpha V_{\epsilon }^3\\ & +\frac{\mathrm{\partial }{V}_{\epsilon }^2}{\mathrm{\partial }{x}_2}+\frac{\mathrm{\partial }{V}_{\epsilon }^3}{\mathrm{\partial }{x}_3}+2\kappa ({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{x}}_{\ast })(\frac{\mathrm{\partial }{V}_{\epsilon }^1}{\mathrm{\partial }{x}_1}-\kappa (V_{\epsilon }^2\cos \alpha -V_{\epsilon }^3\sin \alpha )+\left({\kappa }^{\prime }\right({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{x}}_{\ast })+\kappa \tau ({\mathbf{\text{e}}}_{\alpha }^{\perp }\cdot {\mathit{x}}_{\ast }\left)\right)V_{\epsilon }^1\\ & +{\kappa }^2({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{x}}_{\ast })\cos \alpha V_{\epsilon }^2-{\kappa }^2({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{x}}_{\ast })\sin \alpha V_{\epsilon }^3)+3{\kappa }^2({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{x}}_{\ast }{)}^2(\frac{\mathrm{\partial }{V}_{\epsilon }^1}{\mathrm{\partial }{x}_1}-\kappa (V_{\epsilon }^2\cos \alpha -V_{\epsilon }^3\sin \alpha ))\\ & +4{\kappa }^3({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{x}}_{\ast }{)}^3\frac{\mathrm{\partial }{V}_{\epsilon }^1}{\mathrm{\partial }{x}_1}+\mathcal{O}({\epsilon }^3)=0.\end{array}$(15) ), we obtain: $\begin{array}{rl}\text{div}{\mathbf{\text{U}}}_{\epsilon }^{\left[2\right]}& =\epsilon (\frac{\mathrm{\partial }{V}_0^2}{\mathrm{\partial }{y}_2}+\frac{\mathrm{\partial }{V}_0^3}{\mathrm{\partial }{y}_3})+\epsilon (\frac{\mathrm{\partial }{\mathcal{V}}_0^1}{\mathrm{\partial }{y}_1}+\frac{\mathrm{\partial }{\mathcal{V}}_0^2}{\mathrm{\partial }{y}_2}+\frac{\mathrm{\partial }{\mathcal{V}}_0^3}{\mathrm{\partial }{y}_3})+\epsilon (\frac{\mathrm{\partial }{\mathcal{Y}}_0^1}{\mathrm{\partial }{y}_1}+\frac{\mathrm{\partial }{\mathcal{Y}}_0^2}{\mathrm{\partial }{y}_2}+\frac{\mathrm{\partial }{\mathcal{Y}}_0^3}{\mathrm{\partial }{y}_3})\\ & +{\epsilon }^2(\frac{\mathrm{\partial }{V}_0^1}{\mathrm{\partial }{x}_1}+\frac{\mathrm{\partial }{V}_1^2}{\mathrm{\partial }{y}_2}+\frac{\mathrm{\partial }{V}_1^3}{\mathrm{\partial }{y}_3})\\ & +{\epsilon }^2(\frac{\mathrm{\partial }{\mathcal{V}}_1^1}{\mathrm{\partial }{y}_1}+\frac{\mathrm{\partial }{\mathcal{V}}_1^2}{\mathrm{\partial }{y}_2}+\frac{\mathrm{\partial }{\mathcal{V}}_1^3}{\mathrm{\partial }{y}_3}-\kappa ({\mathcal{V}}_0^2\cos \alpha -{\mathcal{V}}_0^3\sin \alpha )\\ & +2\kappa (y_2\cos \alpha \frac{\mathrm{\partial }{\mathcal{V}}_0^1}{\mathrm{\partial }{y}_1}-y_3\sin \alpha \frac{\mathrm{\partial }{\mathcal{V}}_0^1}{\mathrm{\partial }{y}_1}))\\ & +{\epsilon }^2(\frac{\mathrm{\partial }{\mathcal{Y}}_1^1}{\mathrm{\partial }{y}_1}+\frac{\mathrm{\partial }{\mathcal{Y}}_1^2}{\mathrm{\partial }{y}_2}+\frac{\mathrm{\partial }{\mathcal{Y}}_1^3}{\mathrm{\partial }{y}_3}-\kappa ({\mathcal{Y}}_0^2\cos \alpha -{\mathcal{Y}}_0^3\sin \alpha )\\ & +2\kappa (y_2\cos \alpha \frac{\mathrm{\partial }{\mathcal{Y}}_0^1}{\mathrm{\partial }{y}_1}-y_3\sin \alpha \frac{\mathrm{\partial }{\mathcal{Y}}_0^1}{\mathrm{\partial }{y}_1}))\\ & +{\epsilon }^3(\frac{\mathrm{\partial }{V}_1^1}{\mathrm{\partial }{x}_1}-\kappa (V_1^2\cos \alpha -V_1^3\sin \alpha )+\left({\kappa }^{\prime }\right({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast })+\kappa \tau ({\mathbf{\text{e}}}_{\alpha }^{\perp }\cdot {\mathit{y}}_{\ast }\left)\right)V_0^1+\frac{\mathrm{\partial }{V}_2^2}{\mathrm{\partial }{y}_2}+\frac{\mathrm{\partial }{V}_2^3}{\mathrm{\partial }{y}_3})\\ & +{\epsilon }^3(\frac{\mathrm{\partial }{\mathcal{V}}_2^1}{\mathrm{\partial }{y}_1}+\frac{\mathrm{\partial }{\mathcal{V}}_2^2}{\mathrm{\partial }{y}_2}+\frac{\mathrm{\partial }{\mathcal{V}}_2^3}{\mathrm{\partial }{y}_3}-\kappa ({\mathcal{V}}_1^2\cos \alpha -{\mathcal{V}}_1^3\sin \alpha )+\left({\kappa }^{\prime }\right({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast })+\kappa \tau ({\mathbf{\text{e}}}_{\alpha }^{\perp }\cdot {\mathit{y}}_{\ast }\left)\right){\mathcal{V}}_0^1\\ & +{\kappa }^2({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast })\cos \alpha {\mathcal{V}}_0^2\\ & -{\kappa }^2({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast })\sin \alpha {\mathcal{V}}_0^3+2\kappa ({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast })(\frac{\mathrm{\partial }{\mathcal{V}}_1^1}{\mathrm{\partial }{y}_1}-\kappa ({\mathcal{V}}_0^2\cos \alpha -{\mathcal{V}}_0^3\sin \alpha ))\\ & +3{\kappa }^2({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast }{)}^2\frac{\mathrm{\partial }{\mathcal{V}}_0^1}{\mathrm{\partial }{y}_1})\end{array}$ $\begin{array}{rl}& +{\epsilon }^3(\frac{\mathrm{\partial }{\mathcal{Y}}_2^1}{\mathrm{\partial }{y}_1}+\frac{\mathrm{\partial }{\mathcal{Y}}_2^2}{\mathrm{\partial }{y}_2}+\frac{\mathrm{\partial }{\mathcal{Y}}_2^3}{\mathrm{\partial }{y}_3}-\kappa ({\mathcal{Y}}_1^2\cos \alpha -{\mathcal{Y}}_1^3\sin \alpha )+\left({\kappa }^{\prime }\right({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast })+\kappa \tau ({\mathbf{\text{e}}}_{\alpha }^{\perp }\cdot {\mathit{y}}_{\ast }\left)\right){\mathcal{Y}}_0^1\\ & +{\kappa }^2({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast })\cos \alpha {\mathcal{Y}}_0^2\\ & -{\kappa }^2({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast })\sin \alpha {\mathcal{Y}}_0^3+2\kappa ({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast })(\frac{\mathrm{\partial }{\mathcal{Y}}_1^1}{\mathrm{\partial }{y}_1}-\kappa ({\mathcal{Y}}_0^2\cos \alpha -{\mathcal{Y}}_0^3\sin \alpha ))\\ & +3{\kappa }^2({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast }{)}^2\frac{\mathrm{\partial }{\mathcal{Y}}_0^1}{\mathrm{\partial }{y}_1})\\ & +{\epsilon }^4(\frac{\mathrm{\partial }{V}_2^1}{\mathrm{\partial }{x}_1}-\kappa (V_2^2\cos \alpha -V_2^3\sin \alpha )+\left({\kappa }^{\prime }\right({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast })+\kappa \tau ({\mathbf{\text{e}}}_{\alpha }^{\perp }\cdot {\mathit{y}}_{\ast }\left)\right)V_1^1\\ & +\kappa ({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast })\left({\kappa }^{\prime }\right({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast })\\ & +\kappa \tau ({\mathbf{\text{e}}}_{\alpha }^{\perp }\cdot {\mathit{y}}_{\ast }))V_0^1\\ & +{\kappa }^2({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast })(\cos \alpha V_1^2-\sin \alpha V_1^3)+2\kappa ({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast })(\frac{\mathrm{\partial }{V}_1^1}{\mathrm{\partial }{x}_1}-\kappa (V_1^2\cos \alpha -V_1^3\sin \alpha ))\\ & +2\kappa ({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast })\left({\kappa }^{\prime }\right({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast })+\kappa \tau ({\mathbf{\text{e}}}_{\alpha }^{\perp }\cdot {\mathit{y}}_{\ast }\left)\right)V_0^1)\\ & +{\epsilon }^4(-\kappa ({\mathcal{V}}_2^2\cos \alpha -{\mathcal{V}}_2^3\sin \alpha )+\left({\kappa }^{\prime }\right({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast })+\kappa \tau ({\mathbf{\text{e}}}_{\alpha }^{\perp }\cdot {\mathit{y}}_{\ast }\left)\right){\mathcal{V}}_1^1+\kappa ({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast })\left(\kappa \right({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast })\\ & +\kappa \tau ({\mathbf{\text{e}}}_{\alpha }^{\perp }\cdot {\mathit{y}}_{\ast })){\mathcal{V}}_0^1\\ & +{\kappa }^2({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast })\cos \alpha {\mathcal{V}}_1^2-{\kappa }^2({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast })\sin \alpha {\mathcal{V}}_1^3+2\kappa ({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast })\\ & (\frac{\mathrm{\partial }{\mathcal{V}}_2^1}{\mathrm{\partial }{y}_1}-\kappa ({\mathcal{V}}_1^2\cos \alpha -{\mathcal{V}}_1^3\sin \alpha ))\end{array}$ (68) $\begin{array}{rl}& +2\kappa ({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast })(\left({\kappa }^{\prime }\right({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast })+\kappa \tau ({\mathbf{\text{e}}}_{\alpha }^{\perp }\cdot {\mathit{y}}_{\ast }\left)\right){\mathcal{V}}_0^1+{\kappa }^2({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast })\cos \alpha {\mathcal{V}}_0^2-{\kappa }^2({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast })\sin \alpha {\mathcal{V}}_0^3)\\ & +3{\kappa }^2({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast }{)}^2(\frac{\mathrm{\partial }{\mathcal{V}}_1^1}{\mathrm{\partial }{y}_1}-\kappa ({\mathcal{V}}_0^2\cos \alpha -{\mathcal{V}}_0^3\sin \alpha ))+4{\kappa }^3({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast }{)}^3\frac{\mathrm{\partial }{\mathcal{V}}_0^1}{\mathrm{\partial }{y}_1})\\ & +{\epsilon }^4(-\kappa ({\mathcal{Y}}_2^2\cos \alpha -{\mathcal{Y}}_2^3\sin \alpha )+\left({\kappa }^{\prime }\right({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast })+\kappa \tau ({\mathbf{\text{e}}}_{\alpha }^{\perp }\cdot {\mathit{y}}_{\ast }\left)\right){\mathcal{Y}}_1^1\\ & +\kappa ({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast })\left(\kappa \right({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast })+\kappa \tau ({\mathbf{\text{e}}}_{\alpha }^{\perp }\cdot {\mathit{y}}_{\ast }\left)\right){\mathcal{Y}}_0^1\\ & +{\kappa }^2({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast })\cos \alpha {\mathcal{Y}}_1^2-{\kappa }^2({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast })\sin \alpha {\mathcal{Y}}_1^3+2\kappa ({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast })\\ & (\frac{\mathrm{\partial }{\mathcal{Y}}_2^1}{\mathrm{\partial }{y}_1}-\kappa ({\mathcal{Y}}_1^2\cos \alpha -{\mathcal{Y}}_1^3\sin \alpha ))\\ & +2\kappa ({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast })(\left({\kappa }^{\prime }\right({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast })+\kappa \tau ({\mathbf{\text{e}}}_{\alpha }^{\perp }\cdot {\mathit{y}}_{\ast }\left)\right){\mathcal{Y}}_0^1+{\kappa }^2({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast })\cos \alpha {\mathcal{Y}}_0^2-{\kappa }^2({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast })\sin \alpha {\mathcal{Y}}_0^3)\\ & +3{\kappa }^2({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast }{)}^2(\frac{\mathrm{\partial }{\mathcal{Y}}_1^1}{\mathrm{\partial }{y}_1}-\kappa ({\mathcal{Y}}_0^2\cos \alpha -{\mathcal{Y}}_0^3\sin \alpha ))+4{\kappa }^3({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast }{)}^3\frac{\mathrm{\partial }{\mathcal{Y}}_0^1}{\mathrm{\partial }{y}_1})\\ & +\mathcal{O}\left({\epsilon }^5\right)\end{array}$(68) Taking into account (18(18) $\begin{array}{rl}& -\mu ({\mathrm{\Delta }}_{{\mathit{y}}_{\ast }}{V}_0^1,{\mathrm{\Delta }}_{{\mathit{y}}_{\ast }}{V}_0^2,{\mathrm{\Delta }}_{{\mathit{y}}_{\ast }}{V}_0^3)+(\frac{\mathrm{\partial }{P}_0}{\mathrm{\partial }{x}_1},\frac{\mathrm{\partial }{P}_1}{\mathrm{\partial }{y}_2},\frac{\mathrm{\partial }{P}_1}{\mathrm{\partial }{y}_3})=(f_1,f_2\cos \alpha +f_3\sin \alpha ,f_3\cos \alpha -f_2\sin \alpha )\text{in}\mathrm{\Omega },\\ & {\text{div}}_{{\mathit{y}}_{\ast }}{\mathbf{\text{V}}}_0=0\text{in}\mathrm{\Omega },{\mathbf{\text{V}}}_0=\mathbf{\text{0}}\text{on}\mathrm{\Gamma }=⟨0,l⟩\times \mathrm{\partial }B.\end{array}$(18) )₂, (29(29) $\begin{array}{rl}& -\mu {\mathrm{\Delta }}_{{\mathit{y}}_{\ast }}{\mathbf{\text{V}}}_1^{\ast }+{\mathrm{\nabla }}_{{\mathit{y}}_{\ast }}{P}_2=-\frac{a}{2\delta }{g}_1(y_3,-y_2)\text{in}\mathrm{\Omega },\\ & {\text{div}}_{{\mathit{y}}_{\ast }}{\mathbf{\text{V}}}_1^{\ast }=0\text{in}\mathrm{\Omega },{\mathbf{\text{V}}}_1^{\ast }=\mathbf{\text{0}}\text{on}\mathrm{\Gamma }.\end{array}$(29) )₂, (39(39) $\begin{array}{rl}& -\mu ({\mathrm{\Delta }}_{{\mathit{y}}_{\ast }}{V}_2^2-\kappa \cos \alpha \frac{\mathrm{\partial }{V}_1^2}{\mathrm{\partial }{y}_2}+\kappa \sin \alpha \frac{\mathrm{\partial }{V}_1^2}{\mathrm{\partial }{y}_3}+(2\kappa \frac{\mathrm{\partial }{V}_0^1}{\mathrm{\partial }{x}_1}+{\kappa }^{\prime }{V}_0^1)\cos \alpha +\kappa \tau V_0^1\sin \alpha )+\frac{\mathrm{\partial }{P}_3}{\mathrm{\partial }{y}_2}\\ & =a(\frac{\mathrm{\partial }{W}_1^1}{\mathrm{\partial }{y}_3}+\kappa ({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast })\frac{\mathrm{\partial }{W}_0^1}{\mathrm{\partial }{y}_3}-\frac{\mathrm{\partial }{W}_0^3}{\mathrm{\partial }{x}_1})\text{in}\mathrm{\Omega },\\ & -\mu ({\mathrm{\Delta }}_{{\mathit{y}}_{\ast }}{V}_2^3+\kappa \sin \alpha \frac{\mathrm{\partial }{V}_1^3}{\mathrm{\partial }{y}_3}-\kappa \cos \alpha \frac{\mathrm{\partial }{V}_1^3}{\mathrm{\partial }{y}_2}+\kappa \tau V_0^1\cos \alpha -(2\kappa \frac{\mathrm{\partial }{V}_0^1}{\mathrm{\partial }{x}_1}+{\kappa }^{\prime }{V}_0^1)\sin \alpha )+\frac{\mathrm{\partial }{P}_3}{\mathrm{\partial }{y}_3}\\ & =a(\frac{\mathrm{\partial }{W}_0^2}{\mathrm{\partial }{x}_1}-\frac{\mathrm{\partial }{W}_1^1}{\mathrm{\partial }{y}_2}-\kappa ({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast })\frac{\mathrm{\partial }{W}_0^1}{\mathrm{\partial }{y}_2})\text{in}\mathrm{\Omega },\\ & \frac{\mathrm{\partial }{V}_2^2}{\mathrm{\partial }{y}_2}+\frac{\mathrm{\partial }{V}_2^3}{\mathrm{\partial }{y}_3}=-\frac{\mathrm{\partial }{V}_1^1}{\mathrm{\partial }{x}_1}+\kappa (V_1^2\cos \alpha -V_1^3\sin \alpha )-\left({\kappa }^{\prime }\right({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast })+\kappa \tau ({\mathbf{\text{e}}}_{\alpha }^{\perp }\cdot {\mathit{y}}_{\ast }\left)\right)V_0^1\ne 0\text{in}\mathrm{\Omega },\\ & V_2^2=V_2^3=0\text{on}\mathrm{\Gamma }.\end{array}$(39) )₃, (52(52) $\begin{array}{rl}& -\mu \mathrm{\Delta }{\mathcal{V}}_0+\mathrm{\nabla }{\mathcal{P}}_0=0\text{in}{\mathcal{G}}_0,\\ & \text{div}{\mathcal{V}}_0=0\text{in}{\mathcal{G}}_0,{\mathcal{V}}_0=0\text{on}\omega =⟨0,\mathrm{\infty }⟩\times \mathrm{\partial }B,\\ & {\mathcal{V}}_0(t,0,\cdot )=(h_0^t-V_0^1(t,0,\cdot ),h_0^n\cos \alpha (0)+h_0^b\sin \alpha (0),h_0^b\cos \alpha (0)-h_0^n\sin \alpha (0\left)\right).\end{array}$(52) )₂, (53(53) $\begin{array}{rl}& -\mu \mathrm{\Delta }{\mathcal{Y}}_0+\mathrm{\nabla }{\mathcal{Q}}_0=0\text{in}{\mathcal{G}}_l,\\ & \text{div}{\mathcal{Y}}_0=0\text{in}{\mathcal{G}}_l,{\mathcal{Y}}_0=0\text{on}\sigma =⟨-\mathrm{\infty },0⟩\times \mathrm{\partial }B,\\ & {\mathcal{Y}}_0(t,0,\cdot )=(h_l^t-V_0^1(t,l,\cdot ),h_l^n\cos \alpha (l)+h_l^b\sin \alpha (l),h_l^b\cos \alpha (l)-h_l^n\sin \alpha (l\left)\right).\end{array}$(53) )₂, (54(54) $\begin{array}{rl}& -\mu \mathrm{\Delta }{\mathcal{V}}_1+\mathrm{\nabla }{\mathcal{P}}_1=\mathrm{\Xi }(t,0,{\mathcal{V}}_0,{\mathcal{W}}_0,{\mathcal{P}}_0)\text{in}{\mathcal{G}}_0,\\ & \text{div}{\mathcal{V}}_1=\kappa \left(0\right)\cos \alpha \left(0\right){\mathcal{V}}_0^2-\kappa \left(0\right)\sin \alpha \left(0\right){\mathcal{V}}_0^3\\ & -(2\kappa \left(0\right)\cos \alpha \left(0\right)y_2\frac{\mathrm{\partial }{\mathcal{V}}_0^1}{\mathrm{\partial }{y}_1}-2\kappa \left(0\right)\sin \alpha \left(0\right)y_3\frac{\mathrm{\partial }{\mathcal{V}}_0^1}{\mathrm{\partial }{y}_1}),{\mathcal{V}}_1=0\text{on}\omega =⟨0,\mathrm{\infty }⟩\times \mathrm{\partial }B,\\ & {\mathcal{V}}_1(t,0,\cdot )=-\left(V_1^1\right(t,0,\cdot ),V_1^2(t,0,\cdot ),V_1^3(t,0,\cdot \left)\right)-\left(\kappa \right(0\left)\right({\mathbf{\text{e}}}_{\alpha }\left(0\right)\cdot {\mathit{y}}_{\ast }\left)V_0^1\right(t,0,\cdot ),0,0)\\ & -\left(\kappa \right(0\left)\right({\mathbf{\text{e}}}_{\alpha }\left(0\right)\cdot {\mathit{y}}_{\ast }\left){\mathcal{V}}_0^1\right(t,0,\cdot ),0,0),\end{array}$(54) )₂, (57(57) $\begin{array}{rl}& -\mu \mathrm{\Delta }{\mathcal{V}}_2+\mathrm{\nabla }{\mathcal{P}}_2=\mathrm{\Pi }(t,0,{\mathcal{V}}_0,{\mathcal{V}}_1,{\mathcal{W}}_0,{\mathcal{W}}_1,{\mathcal{P}}_0,{\mathcal{P}}_1)\text{in}{\mathcal{G}}_0,\\ & \text{div}{\mathcal{V}}_2=\kappa \left(0\right)\cos \alpha \left(0\right){\mathcal{V}}_1^2-\kappa \left(0\right)\sin \alpha \left(0\right){\mathcal{V}}_1^3-\left({\kappa }^{\prime }\right(0\left)\right({\mathbf{\text{e}}}_{\alpha }\left(0\right)\cdot {\mathit{y}}_{\ast })+\kappa (0\left)\tau \right(0\left)\right({\mathbf{\text{e}}}_{\alpha }^{\perp }\left(0\right)\cdot {\mathit{y}}_{\ast }\left)\right){\mathcal{V}}_0^1\\ & -{\kappa }^2\left(0\right)\left({\mathbf{\text{e}}}_{\alpha }\right(0)\cdot {\mathit{y}}_{\ast })\cos \alpha \left(0\right){\mathcal{V}}_0^2+{\kappa }^2\left(0\right)\left({\mathbf{\text{e}}}_{\alpha }\right(0)\cdot {\mathit{y}}_{\ast })\sin \alpha \left(0\right){\mathcal{V}}_0^3\\ & +2\kappa \left(0\right)\left({\mathbf{\text{e}}}_{\alpha }\right(0)\cdot {\mathit{y}}_{\ast })(\kappa \left(0\right)\cos \alpha \left(0\right){\mathcal{V}}_0^2-\kappa \left(0\right)\sin \alpha \left(0\right){\mathcal{V}}_0^3)\\ & -2\kappa \left(0\right)\left({\mathbf{\text{e}}}_{\alpha }\right(0)\cdot {\mathit{y}}_{\ast })\frac{\mathrm{\partial }{\mathcal{V}}_1^1}{\mathrm{\partial }{y}_1}-3{\kappa }^2\left(0\right)\left({\mathbf{\text{e}}}_{\alpha }\right(0)\cdot {\mathit{y}}_{\ast }{)}^2\frac{\mathrm{\partial }{\mathcal{V}}_0^1}{\mathrm{\partial }{y}_1}\text{in}{\mathcal{G}}_0,\\ & {\mathcal{V}}_2=0\text{in}\omega =⟨0,\mathrm{\infty }⟩\times \mathrm{\partial }B,\\ & {\mathcal{V}}_2(t,0,\cdot )=-V_2(t,0,\cdot )-\left(\kappa \right({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast }\left)V_1^1\right(t,0,\cdot ),0,0)-\left({\kappa }^2\right({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast }{)}^2{V}_0^1(t,0,\cdot ),0,0)\\ & -\left(\kappa \right({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast }\left){\mathcal{V}}_1^1\right(t,0,\cdot ),0,0)-\left({\kappa }^2\right({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast }{)}^2{\mathcal{V}}_0^1(t,0,\cdot ),0,0),\end{array}$(57) )₂, we get from (68(68) $\begin{array}{rl}& +2\kappa ({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast })(\left({\kappa }^{\prime }\right({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast })+\kappa \tau ({\mathbf{\text{e}}}_{\alpha }^{\perp }\cdot {\mathit{y}}_{\ast }\left)\right){\mathcal{V}}_0^1+{\kappa }^2({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast })\cos \alpha {\mathcal{V}}_0^2-{\kappa }^2({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast })\sin \alpha {\mathcal{V}}_0^3)\\ & +3{\kappa }^2({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast }{)}^2(\frac{\mathrm{\partial }{\mathcal{V}}_1^1}{\mathrm{\partial }{y}_1}-\kappa ({\mathcal{V}}_0^2\cos \alpha -{\mathcal{V}}_0^3\sin \alpha ))+4{\kappa }^3({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast }{)}^3\frac{\mathrm{\partial }{\mathcal{V}}_0^1}{\mathrm{\partial }{y}_1})\\ & +{\epsilon }^4(-\kappa ({\mathcal{Y}}_2^2\cos \alpha -{\mathcal{Y}}_2^3\sin \alpha )+\left({\kappa }^{\prime }\right({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast })+\kappa \tau ({\mathbf{\text{e}}}_{\alpha }^{\perp }\cdot {\mathit{y}}_{\ast }\left)\right){\mathcal{Y}}_1^1\\ & +\kappa ({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast })\left(\kappa \right({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast })+\kappa \tau ({\mathbf{\text{e}}}_{\alpha }^{\perp }\cdot {\mathit{y}}_{\ast }\left)\right){\mathcal{Y}}_0^1\\ & +{\kappa }^2({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast })\cos \alpha {\mathcal{Y}}_1^2-{\kappa }^2({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast })\sin \alpha {\mathcal{Y}}_1^3+2\kappa ({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast })\\ & (\frac{\mathrm{\partial }{\mathcal{Y}}_2^1}{\mathrm{\partial }{y}_1}-\kappa ({\mathcal{Y}}_1^2\cos \alpha -{\mathcal{Y}}_1^3\sin \alpha ))\\ & +2\kappa ({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast })(\left({\kappa }^{\prime }\right({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast })+\kappa \tau ({\mathbf{\text{e}}}_{\alpha }^{\perp }\cdot {\mathit{y}}_{\ast }\left)\right){\mathcal{Y}}_0^1+{\kappa }^2({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast })\cos \alpha {\mathcal{Y}}_0^2-{\kappa }^2({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast })\sin \alpha {\mathcal{Y}}_0^3)\\ & +3{\kappa }^2({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast }{)}^2(\frac{\mathrm{\partial }{\mathcal{Y}}_1^1}{\mathrm{\partial }{y}_1}-\kappa ({\mathcal{Y}}_0^2\cos \alpha -{\mathcal{Y}}_0^3\sin \alpha ))+4{\kappa }^3({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast }{)}^3\frac{\mathrm{\partial }{\mathcal{Y}}_0^1}{\mathrm{\partial }{y}_1})\\ & +\mathcal{O}\left({\epsilon }^5\right)\end{array}$(68) ) the following equation: $\begin{array}{rl}\text{div}{\mathbf{\text{U}}}_{\epsilon }^{\left[2\right]}& ={\epsilon }^2(\kappa \left(0\right)({\mathcal{V}}_0^2\cos \alpha (0)-{\mathcal{V}}_0^3\sin \alpha (0\left)\right)-\kappa \left(x_1\right)({\mathcal{V}}_0^2\cos \alpha (x_1)-{\mathcal{V}}_0^3\sin \alpha (x_1\left)\right))\\ & +{\epsilon }^2(-2\kappa \left(0\right)(y_2\cos \alpha \left(0\right)-y_3\sin \alpha \left(0\right))\frac{\mathrm{\partial }{\mathcal{V}}_0^1}{\mathrm{\partial }{y}_1}\\ & +2\kappa \left(x_1\right)(y_2\cos \alpha \left(x_1\right)-y_3\sin \alpha \left(x_1\right))\frac{\mathrm{\partial }{\mathcal{V}}_0^1}{\mathrm{\partial }{y}_1})\\ & +{\epsilon }^2(\kappa \left(l\right)({\mathcal{Y}}_0^2\cos \alpha (l)-{\mathcal{Y}}_0^3\sin \alpha (l\left)\right)-\kappa \left(x_1\right)({\mathcal{Y}}_0^2\cos \alpha (x_1)-{\mathcal{Y}}_0^3\sin \alpha (x_1\left)\right))\\ & +{\epsilon }^2(-2\kappa \left(l\right)(y_2\cos \alpha \left(l\right)-y_3\sin \alpha \left(l\right))\frac{\mathrm{\partial }{\mathcal{Y}}_0^1}{\mathrm{\partial }{y}_1}\\ & +2\kappa \left(x_1\right)(y_2\cos \alpha \left(x_1\right)-y_3\sin \alpha \left(x_1\right))\frac{\mathrm{\partial }{\mathcal{Y}}_0^1}{\mathrm{\partial }{y}_1})\\ & +{\epsilon }^3(\kappa \left(0\right)({\mathcal{V}}_1^2\cos \alpha (0)-{\mathcal{V}}_1^3\sin \alpha (0\left)\right)-\kappa \left(x_1\right)({\mathcal{V}}_1^2\cos \alpha (x_1)-{\mathcal{V}}_1^3\sin \alpha (x_1\left)\right))\\ & +{\epsilon }^3((-{\kappa }^{\prime }(0\left)\right(y_2\cos \alpha \left(0\right)-y_3\sin \alpha \left(0\right))-\kappa (0\left)\tau \right(0\left)\right(y_2\sin \alpha \left(0\right)+y_3\cos \alpha \left(0\right)\left)\right){\mathcal{V}}_0^1)\\ & +{\epsilon }^3(\left({\kappa }^{\prime }\right(x_1\left)\right(y_2\cos \alpha \left(x_1\right)-y_3\sin \alpha \left(x_1\right))+\kappa (x_1\left)\tau \right(x_1\left)\right(y_2\sin \alpha \left(x_1\right)+y_3\cos \alpha \left(x_1\right)\left)\right){\mathcal{V}}_0^1)\\ & +{\epsilon }^3(-{\kappa }^2\left(0\right)(y_2\cos \alpha (0)-y_3\sin \alpha (0\left)\right)(\cos \alpha (0){\mathcal{V}}_0^2-\sin \alpha (0\left){\mathcal{V}}_0^3\right))\end{array}$ (69) $\begin{array}{rl}& +{\epsilon }^3({\kappa }^2\left(x_1\right)(y_2\cos \alpha (x_1)-y_3\sin \alpha (x_1\left)\right)(\cos \alpha (x_1){\mathcal{V}}_0^2-\sin \alpha (x_1\left){\mathcal{V}}_0^3\right))\\ & +{\epsilon }^3(-2\kappa \left(0\right)(y_2\cos \alpha \left(0\right)-y_3\sin \alpha \left(0\right))\frac{\mathrm{\partial }{\mathcal{V}}_1^1}{\mathrm{\partial }{y}_1}\\ & +2\kappa \left(x_1\right)(y_2\cos \alpha \left(x_1\right)-y_3\sin \alpha \left(x_1\right))\frac{\mathrm{\partial }{\mathcal{V}}_1^1}{\mathrm{\partial }{y}_1})\\ & +{\epsilon }^3(-3{\kappa }^2\left(0\right)(\cos \alpha (0)-\sin \alpha (0){)}^2\frac{\mathrm{\partial }{\mathcal{V}}_0^1}{\mathrm{\partial }{y}_1}+3{\kappa }^2(x_1\left)\right(\cos \alpha \left(x_1\right)-\sin \alpha \left(x_1\right){)}^2\frac{\mathrm{\partial }{\mathcal{V}}_0^1}{\mathrm{\partial }{y}_1})\\ & +{\epsilon }^4(\frac{\mathrm{\partial }{V}_2^1}{\mathrm{\partial }{x}_1}-\kappa (V_2^2\cos \alpha -V_2^3\sin \alpha )+\left({\kappa }^{\prime }\right({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast })+\kappa \tau ({\mathbf{\text{e}}}_{\alpha }^{\perp }\cdot {\mathit{y}}_{\ast }\left)\right)V_1^1\\ & +\kappa ({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast })\left({\kappa }^{\prime }\right({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast })+\kappa \tau ({\mathbf{\text{e}}}_{\alpha }^{\perp }\cdot {\mathit{y}}_{\ast }\left)\right)V_0^1\\ & +{\kappa }^2({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast })(\cos \alpha V_1^2-\sin \alpha V_1^3)+2\kappa ({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast })(\frac{\mathrm{\partial }{V}_1^1}{\mathrm{\partial }{x}_1}-\kappa (V_1^2\cos \alpha -V_1^3\sin \alpha ))\\ & +2\kappa ({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast })\left({\kappa }^{\prime }\right({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast })+\kappa \tau ({\mathbf{\text{e}}}_{\alpha }^{\perp }\cdot {\mathit{y}}_{\ast }\left)\right)V_0^1)+{\mathit{\pi }}_{\epsilon ,res},\end{array}$(69) where $\parallel {\mathit{\pi }}_{\epsilon ,res}{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}=\mathcal{O}\left({\epsilon }^{11/2}\right)$. We write the above equation as (70) ${\mathit{\pi }}_{\epsilon }={\mathit{\pi }}_{\epsilon ,1}+{\mathit{\pi }}_{\epsilon ,2}+{\mathit{\pi }}_{\epsilon ,3}+{\mathit{\pi }}_{\epsilon ,res}$(70) where ${\mathit{\pi }}_{\epsilon ,1}$ is ${\epsilon }^2$ term (first six lines), ${\mathit{\pi }}_{\epsilon ,2}$ the ${\epsilon }^3$ term (seventh to fourteenth line) and ${\mathit{\pi }}_{\epsilon ,3}$ the ${\epsilon }^4$ term (last four lines) in equation (69(69) $\begin{array}{rl}& +{\epsilon }^3({\kappa }^2\left(x_1\right)(y_2\cos \alpha (x_1)-y_3\sin \alpha (x_1\left)\right)(\cos \alpha (x_1){\mathcal{V}}_0^2-\sin \alpha (x_1\left){\mathcal{V}}_0^3\right))\\ & +{\epsilon }^3(-2\kappa \left(0\right)(y_2\cos \alpha \left(0\right)-y_3\sin \alpha \left(0\right))\frac{\mathrm{\partial }{\mathcal{V}}_1^1}{\mathrm{\partial }{y}_1}\\ & +2\kappa \left(x_1\right)(y_2\cos \alpha \left(x_1\right)-y_3\sin \alpha \left(x_1\right))\frac{\mathrm{\partial }{\mathcal{V}}_1^1}{\mathrm{\partial }{y}_1})\\ & +{\epsilon }^3(-3{\kappa }^2\left(0\right)(\cos \alpha (0)-\sin \alpha (0){)}^2\frac{\mathrm{\partial }{\mathcal{V}}_0^1}{\mathrm{\partial }{y}_1}+3{\kappa }^2(x_1\left)\right(\cos \alpha \left(x_1\right)-\sin \alpha \left(x_1\right){)}^2\frac{\mathrm{\partial }{\mathcal{V}}_0^1}{\mathrm{\partial }{y}_1})\\ & +{\epsilon }^4(\frac{\mathrm{\partial }{V}_2^1}{\mathrm{\partial }{x}_1}-\kappa (V_2^2\cos \alpha -V_2^3\sin \alpha )+\left({\kappa }^{\prime }\right({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast })+\kappa \tau ({\mathbf{\text{e}}}_{\alpha }^{\perp }\cdot {\mathit{y}}_{\ast }\left)\right)V_1^1\\ & +\kappa ({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast })\left({\kappa }^{\prime }\right({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast })+\kappa \tau ({\mathbf{\text{e}}}_{\alpha }^{\perp }\cdot {\mathit{y}}_{\ast }\left)\right)V_0^1\\ & +{\kappa }^2({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast })(\cos \alpha V_1^2-\sin \alpha V_1^3)+2\kappa ({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast })(\frac{\mathrm{\partial }{V}_1^1}{\mathrm{\partial }{x}_1}-\kappa (V_1^2\cos \alpha -V_1^3\sin \alpha ))\\ & +2\kappa ({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast })\left({\kappa }^{\prime }\right({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast })+\kappa \tau ({\mathbf{\text{e}}}_{\alpha }^{\perp }\cdot {\mathit{y}}_{\ast }\left)\right)V_0^1)+{\mathit{\pi }}_{\epsilon ,res},\end{array}$(69) ).

We now obtain for ${\mathit{\pi }}_{\epsilon ,1}$: (71) $\begin{array}{rl}{\mathit{\pi }}_{\epsilon ,1}& ={\epsilon }^2(\kappa \left(0\right)(\cos \alpha (0)-\cos \alpha (x_1\left)\right)+\left(\kappa \right(0)-\kappa (x_1\left)\right)\cos \alpha \left(x_1\right)){\mathcal{V}}_0^2\\ & -{\epsilon }^2(\kappa \left(0\right)(\sin \alpha (0)-\sin \alpha (x_1\left)\right)+\left(\kappa \right(0)-\kappa (x_1\left)\right)\sin \alpha \left(x_1\right)){\mathcal{V}}_0^3\\ & -{\epsilon }^2(2\kappa \left(0\right)(\cos \alpha (0)-\cos \alpha (x_1\left)\right)+\left(2\kappa \right(0)-2\kappa (x_1\left)\right)\cos \alpha \left(x_1\right))y_2\frac{\mathrm{\partial }{\mathcal{V}}_0^1}{\mathrm{\partial }{y}_1}\\ & +{\epsilon }^2(2\kappa \left(0\right)(\sin \alpha (0)-\sin \alpha (x_1\left)\right)+2\left(\kappa \right(0)-\kappa (x_1\left)\right)\sin \alpha \left(x_1\right))y_3\frac{\mathrm{\partial }{\mathcal{V}}_0^1}{\mathrm{\partial }{y}_1}\\ & +{\epsilon }^2(\kappa \left(l\right)(\cos \alpha (l)-\cos \alpha (x_1\left)\right)+\left(\kappa \right(l)-\kappa (x_1\left)\right)\cos \alpha \left(x_1\right)){\mathcal{Y}}_0^2\\ & -{\epsilon }^2(\kappa \left(l\right)(\sin \alpha (l)-\sin \alpha (x_1\left)\right)+\left(\kappa \right(l)-\kappa (x_1\left)\right)\sin \alpha \left(x_1\right)){\mathcal{Y}}_0^3\\ & -{\epsilon }^2(2\kappa \left(l\right)(\cos \alpha (l)-\cos \alpha (x_1\left)\right)+\left(2\kappa \right(l)-2\kappa (x_1\left)\right)\cos \alpha \left(x_1\right))y_2\frac{\mathrm{\partial }{\mathcal{Y}}_0^1}{\mathrm{\partial }{y}_1}\\ & +{\epsilon }^2(2\kappa \left(l\right)(\sin \alpha (l)-\sin \alpha (x_1\left)\right)+2\left(\kappa \right(l)-\kappa (x_1\left)\right)\sin \alpha \left(x_1\right))y_3\frac{\mathrm{\partial }{\mathcal{Y}}_0^1}{\mathrm{\partial }{y}_1}\end{array}$(71) Using a simple change of variables and the fact that ${\mathcal{V}}_0$ is concentrated near $y_1=0$ and ${\mathcal{Y}}_0$ near $x_1=l$, we deduce the estimate (72) $\parallel {\mathit{\pi }}_{\epsilon ,1}{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}=\mathcal{O}\left({\epsilon }^{9/2}\right),$(72) and it easily follows from (69(69) $\begin{array}{rl}& +{\epsilon }^3({\kappa }^2\left(x_1\right)(y_2\cos \alpha (x_1)-y_3\sin \alpha (x_1\left)\right)(\cos \alpha (x_1){\mathcal{V}}_0^2-\sin \alpha (x_1\left){\mathcal{V}}_0^3\right))\\ & +{\epsilon }^3(-2\kappa \left(0\right)(y_2\cos \alpha \left(0\right)-y_3\sin \alpha \left(0\right))\frac{\mathrm{\partial }{\mathcal{V}}_1^1}{\mathrm{\partial }{y}_1}\\ & +2\kappa \left(x_1\right)(y_2\cos \alpha \left(x_1\right)-y_3\sin \alpha \left(x_1\right))\frac{\mathrm{\partial }{\mathcal{V}}_1^1}{\mathrm{\partial }{y}_1})\\ & +{\epsilon }^3(-3{\kappa }^2\left(0\right)(\cos \alpha (0)-\sin \alpha (0){)}^2\frac{\mathrm{\partial }{\mathcal{V}}_0^1}{\mathrm{\partial }{y}_1}+3{\kappa }^2(x_1\left)\right(\cos \alpha \left(x_1\right)-\sin \alpha \left(x_1\right){)}^2\frac{\mathrm{\partial }{\mathcal{V}}_0^1}{\mathrm{\partial }{y}_1})\\ & +{\epsilon }^4(\frac{\mathrm{\partial }{V}_2^1}{\mathrm{\partial }{x}_1}-\kappa (V_2^2\cos \alpha -V_2^3\sin \alpha )+\left({\kappa }^{\prime }\right({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast })+\kappa \tau ({\mathbf{\text{e}}}_{\alpha }^{\perp }\cdot {\mathit{y}}_{\ast }\left)\right)V_1^1\\ & +\kappa ({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast })\left({\kappa }^{\prime }\right({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast })+\kappa \tau ({\mathbf{\text{e}}}_{\alpha }^{\perp }\cdot {\mathit{y}}_{\ast }\left)\right)V_0^1\\ & +{\kappa }^2({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast })(\cos \alpha V_1^2-\sin \alpha V_1^3)+2\kappa ({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast })(\frac{\mathrm{\partial }{V}_1^1}{\mathrm{\partial }{x}_1}-\kappa (V_1^2\cos \alpha -V_1^3\sin \alpha ))\\ & +2\kappa ({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast })\left({\kappa }^{\prime }\right({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast })+\kappa \tau ({\mathbf{\text{e}}}_{\alpha }^{\perp }\cdot {\mathit{y}}_{\ast }\left)\right)V_0^1)+{\mathit{\pi }}_{\epsilon ,res},\end{array}$(69) )–(70(70) ${\mathit{\pi }}_{\epsilon }={\mathit{\pi }}_{\epsilon ,1}+{\mathit{\pi }}_{\epsilon ,2}+{\mathit{\pi }}_{\epsilon ,3}+{\mathit{\pi }}_{\epsilon ,res}$(70) ) that $\parallel {\mathit{\pi }}_{\epsilon }{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}=\mathcal{O}\left({\epsilon }^{9/2}\right)$. It is important to observe that, since ${\mathit{\pi }}_{\epsilon ,i},i=1,2,3$ do not vanish when integrated over the cross-section B, we cannot define the divergence corrector in the general case. For that reason, we look at the simplified model (see Remark 4.1) and complete the construction of the divergence correction in the following.

If we assume that κ and α are constants, it easily follows from (69(69) $\begin{array}{rl}& +{\epsilon }^3({\kappa }^2\left(x_1\right)(y_2\cos \alpha (x_1)-y_3\sin \alpha (x_1\left)\right)(\cos \alpha (x_1){\mathcal{V}}_0^2-\sin \alpha (x_1\left){\mathcal{V}}_0^3\right))\\ & +{\epsilon }^3(-2\kappa \left(0\right)(y_2\cos \alpha \left(0\right)-y_3\sin \alpha \left(0\right))\frac{\mathrm{\partial }{\mathcal{V}}_1^1}{\mathrm{\partial }{y}_1}\\ & +2\kappa \left(x_1\right)(y_2\cos \alpha \left(x_1\right)-y_3\sin \alpha \left(x_1\right))\frac{\mathrm{\partial }{\mathcal{V}}_1^1}{\mathrm{\partial }{y}_1})\\ & +{\epsilon }^3(-3{\kappa }^2\left(0\right)(\cos \alpha (0)-\sin \alpha (0){)}^2\frac{\mathrm{\partial }{\mathcal{V}}_0^1}{\mathrm{\partial }{y}_1}+3{\kappa }^2(x_1\left)\right(\cos \alpha \left(x_1\right)-\sin \alpha \left(x_1\right){)}^2\frac{\mathrm{\partial }{\mathcal{V}}_0^1}{\mathrm{\partial }{y}_1})\\ & +{\epsilon }^4(\frac{\mathrm{\partial }{V}_2^1}{\mathrm{\partial }{x}_1}-\kappa (V_2^2\cos \alpha -V_2^3\sin \alpha )+\left({\kappa }^{\prime }\right({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast })+\kappa \tau ({\mathbf{\text{e}}}_{\alpha }^{\perp }\cdot {\mathit{y}}_{\ast }\left)\right)V_1^1\\ & +\kappa ({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast })\left({\kappa }^{\prime }\right({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast })+\kappa \tau ({\mathbf{\text{e}}}_{\alpha }^{\perp }\cdot {\mathit{y}}_{\ast }\left)\right)V_0^1\\ & +{\kappa }^2({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast })(\cos \alpha V_1^2-\sin \alpha V_1^3)+2\kappa ({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast })(\frac{\mathrm{\partial }{V}_1^1}{\mathrm{\partial }{x}_1}-\kappa (V_1^2\cos \alpha -V_1^3\sin \alpha ))\\ & +2\kappa ({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast })\left({\kappa }^{\prime }\right({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast })+\kappa \tau ({\mathbf{\text{e}}}_{\alpha }^{\perp }\cdot {\mathit{y}}_{\ast }\left)\right)V_0^1)+{\mathit{\pi }}_{\epsilon ,res},\end{array}$(69) ) that ${\mathit{\pi }}_{\epsilon ,1},{\mathit{\pi }}_{\epsilon ,2}=0$, so we have (73) ${\mathit{\pi }}_{\epsilon }={\mathit{\pi }}_{\epsilon ,3}+{\mathit{\pi }}_{\epsilon ,res}.$(73) As in the general case, ${\int }_B{\mathit{\pi }}_{\epsilon ,3}\ne 0$, so we obtain $\parallel {\mathit{\pi }}_{\epsilon }{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}=\mathcal{O}\left({\epsilon }^5\right)$.

If we additionally assume that $\mathbf{\text{f}},\mathbf{\text{g}}$ vanish in the neighbourhood of $x_1=0$ and $x_1=l$ and ${\int }_0^l\mathbf{\text{g}}=0$, the following relations hold (74) ${\int }_B{y}_2^2{h}_0^t={\int }_B{y}_2^2{h}_l^t,{\int }_B{y}_2{y}_3{h}_0^t={\int }_B{y}_2{y}_3{h}_l^t,{\int }_B{y}_3^2{h}_0^t={\int }_B{y}_3^2{h}_l^t$(74) and take $P_2=x_1{p}_{bl}\left(t\right)+p_{div}(t,x_1)$, we can choose $p_{div}$ such that the ${\int }_B{\mathit{\pi }}_{\epsilon ,3}=0$ (note that we have obtained $p_{bl}$ from the compatibility conditions for the second-order velocity and pressure boundary layer correctors).

The ${\epsilon }^4$ divergence term that needs to be corrected reads: (75) $\begin{array}{rl}{\mathit{\pi }}_{\epsilon ,3}& =\frac{\mathrm{\partial }{V}_2^1}{\mathrm{\partial }{x}_1}-\kappa (V_2^2\cos \alpha -V_2^3\sin \alpha )+{\kappa }^2({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast })(\cos \alpha V_1^2-\sin \alpha V_1^3)\\ & +2\kappa ({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast })(\frac{\mathrm{\partial }{V}_1^1}{\mathrm{\partial }{x}_1}-\kappa (V_1^2\cos \alpha -V_1^3\sin \alpha )),\end{array}$(75) while (76) $\begin{array}{rl}{p}_{div}(x_1,t)& =-\frac{\mu }{{\int }_B\mathrm{d}B}{\int }_0^{x_1}({\int }_0^{\zeta }{\int }_B\left(\right|{\mathit{y}}_{\ast }{|}^2-1)(\frac{\mathrm{\partial }{B}_1}{\mathrm{\partial }{x}_1}{y}_2^2+\frac{\mathrm{\partial }{B}_2}{\mathrm{\partial }{x}_1}{y}_2{y}_3+\frac{\mathrm{\partial }{B}_3}{\mathrm{\partial }{x}_1}{y}_3^2+\frac{1}{4}\frac{\mathrm{\partial }{\tilde{A}}_4}{\mathrm{\partial }{x}_1}\\ & +\frac{1}{32}(\frac{\mathrm{\partial }{A}_1}{\mathrm{\partial }{x}_1}+\frac{\mathrm{\partial }{A}_3}{\mathrm{\partial }{x}_1}))\mathrm{d}\xi )\mathrm{d}\zeta \\ & +\frac{\mu }{{\int }_B}{\int }_0^{x_1}({\int }_0^{\zeta }\kappa (\cos \alpha {\int }_B{V}_2^2-\sin \alpha {\int }_B{V}_2^3)\mathrm{d}\xi )\mathrm{d}\zeta \\ & -\frac{\mu }{{\int }_B}{\int }_0^{x_1}({\int }_0^{\zeta }{\int }_B{\kappa }^2({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast })(\cos \alpha V_1^2-\sin \alpha V_1^3)\mathrm{d}\xi )\mathrm{d}\zeta \\ & -\frac{\mu }{{\int }_B}{\int }_0^{x_1}({\int }_0^{\zeta }{\int }_{B}2\kappa ({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast })(\frac{1}{8\mu }(1-|{\mathit{y}}_{\ast }{|}^2\left)\right(y_2\frac{\mathrm{\partial }{H}_1}{\mathrm{\partial }{x}_1}+y_3\frac{\mathrm{\partial }{H}_2}{\mathrm{\partial }{x}_1})\\ & -\kappa (V_1^2\cos \alpha -V_1^3\sin \alpha ))\mathrm{d}\xi )\mathrm{d}\zeta ,\end{array}$(76) with $A_4=\left(2/\mu \pi \right)\left(\mathrm{d}/\mathrm{d}t\right)F_0^{\ast }-\left(\kappa \cos \alpha /8\mu \right)H_1+\left(\kappa \sin \alpha /8\mu \right)H_2-\left(a/\mu \right)(H_{11}-H_{10})$. Observe that $\mathrm{\partial }{p}_{div}(x_1,t)/\mathrm{\partial }{x}_1$ vanishes at $x_1=0$ and $x_1=l$ due to our assumptions and does not appear in the compatibility conditions for the velocity and pressure second-order boundary layer correctors at $x_1=0$ and $x_1=l$.

Finally, we can now define our divergence corrector as (77) ${\mathrm{\Psi }}_{\epsilon }(t,\mathit{x})={\epsilon }^5\sum _{i=2}^3{\mathrm{\Psi }}_i(x_1,\frac{{\mathit{x}}_{\ast }}{\epsilon }){\mathbf{\text{a}}}^i,$(77) where ${\mathrm{\Psi }}_{\epsilon }$ is the solution of the problem (78) $\begin{array}{rl}& {\text{div}}_{{\mathit{y}}_{\ast }}{\mathrm{\Psi }}_{\epsilon }={\mathit{\pi }}_{\mathit{\epsilon }\mathbf{,}\mathbf{3}},\\ & {\mathrm{\Psi }}_{\epsilon }=0\text{on}⟨0,l⟩\times \mathrm{\partial }B.\end{array}$(78) In this case, we define ${\widetilde{\mathbf{\text{u}}}}_{\epsilon }^{\left[2\right]}(t,\stackrel{\mathbf{~}}{\mathit{x}})={\widetilde{\mathbf{\text{U}}}}_{\epsilon }^{\left[2\right]}(t,\mathit{x})$, $\stackrel{\mathbf{~}}{\mathit{x}}={\mathrm{\Phi }}_{\epsilon }^{\alpha }\left(\mathit{x}\right)$, where ${\widetilde{\mathbf{\text{U}}}}_{\epsilon }^{\left[2\right]}={\mathbf{\text{U}}}_{\epsilon }^{\left[2\right]}-{\mathrm{\Psi }}_{\epsilon }$.

In this way, the divergence estimate is improved, namely: (79) $\text{div}{\widetilde{\mathbf{\text{u}}}}_{\epsilon }^{\left[2\right]}={\tilde{\mathit{\pi }}}_{\epsilon },\parallel {\tilde{\mathit{\pi }}}_{\epsilon }{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}=\mathcal{O}\left({\epsilon }^{11/2}\right).$(79)

5. Error analysis

5.1. A priori estimates

To derive the a priori estimates, we first provide two technical results, namely the Poincaré's inequality and the estimates for the divergence equation.

The following result is a direct consequence of [9, Lemma 7].

Lemma 5.1

Poincaré's inequality

Let $T\in ⟨0,\mathrm{\infty }⟩$ and $t\in [0,T]$. There then exists a constant $C>0,$ independent of ε, such that (80) $\parallel {\mathit{\varphi }}_{\epsilon }(t,\cdot ){\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}\le C\epsilon \parallel \mathrm{\nabla }{\mathit{\varphi }}_{\epsilon }(t,\cdot ){\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)},$(80) for any ${\mathit{\varphi }}_{\epsilon }(t,\cdot )\in W^{1,2}\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)$ such that ${\mathit{\varphi }}_{\epsilon }=0$ on ${\mathrm{\Gamma }}_{\epsilon }^{\alpha }$.

We will also need to consider the following result on the divergence equation, which can be straightforwardly deduced from [9, Lemma 9].

Lemma 5.2

Divergence equation

Let $T\in ⟨0,\mathrm{\infty }⟩$ and $t\in [0,T]$. Futhermore, let ${\displaystyle \frac{{\mathrm{\partial }}^{j}K}{\mathrm{\partial }{t}^j}}\in L^2(0,T;L^2({\mathrm{\Omega }}_{\epsilon }^{\alpha }\left)\right),{\displaystyle \frac{{\mathrm{\partial }}^j{\mathbf{\text{g}}}_0}{\mathrm{\partial }{t}^j}}\in L^2(0,T;H^{1/2}({\mathrm{\Sigma }}_{\epsilon }^0\left)\right),{\displaystyle \frac{{\mathrm{\partial }}^j{\mathbf{\text{g}}}_l}{\mathrm{\partial }{t}^j}}\in L^2(0,T;H^{1/2}({\mathrm{\Sigma }}_{\epsilon }^l\left)\right)$ for j=0,1,2. There then exists ${\mathit{\varphi }}_{\epsilon }\in L^2(0,T;W^{1,2}({\mathrm{\Omega }}_{\epsilon }^{\alpha }\left)\right)$ such that ${\displaystyle \frac{{\mathrm{\partial }}^j{\mathit{\varphi }}_{\epsilon }}{\mathrm{\partial }{t}^j}}\in L^2(0,T;W^{1,2}({\mathrm{\Omega }}_{\epsilon }^{\alpha }\left)\right),j=1,2$ satisfying $\begin{array}{rl}& \text{div}{\mathit{\varphi }}_{\epsilon }=K\text{in}{\mathrm{\Omega }}_{\epsilon }^{\alpha },\\ & {\mathit{\varphi }}_{\epsilon }=0\text{on}{\mathrm{\Gamma }}_{\epsilon }^{\alpha },\\ & \mathit{\varphi }={\mathbf{\text{g}}}_0\text{on}{\mathrm{\Sigma }}_{\epsilon }^0,\mathit{\varphi }={\mathbf{\text{g}}}_l\text{on}{\mathrm{\Sigma }}_{\epsilon }^l,\end{array}$ where ${\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}K=-{\int }_{{\mathrm{\Sigma }}_{\epsilon }^0}{\mathbf{\text{g}}}_0\cdot \mathbf{\text{t}}\left(0\right)+{\int }_{{\mathrm{\Sigma }}_{\epsilon }^l}{\mathbf{\text{g}}}_l^{\epsilon }\cdot \mathbf{\text{t}}\left(l\right).$ Moreover, if ${\mathbf{\text{g}}}_i^t=({\mathbf{\text{g}}}_i\cdot \mathbf{\text{t}})\mathbf{\text{t}},{\mathbf{\text{g}}}_i^{nb}=(\mathbf{\text{I}}-\mathbf{\text{t}}\otimes \mathbf{\text{t}}){\mathbf{\text{g}}}_i,i=0,l,$ then ${\mathit{\varphi }}_{\epsilon }$ satisfies the following estimate (81) $\begin{array}{rl}& \parallel \mathrm{\nabla }{\mathit{\varphi }}_{\epsilon }{\parallel }_{L^2(0,T;L^2({\mathrm{\Omega }}_{\epsilon }^{\alpha }\left)\right)}\\ & \le C(\frac{1}{\epsilon }\parallel K{\parallel }_{L^2(0,T;L^2({\mathrm{\Omega }}_{\epsilon }^{\alpha }\left)\right)}+\frac{1}{\sqrt{\epsilon }}(\parallel {\mathbf{\text{g}}}_0^t{\parallel }_{L^2(0,T;H^{1/2}({\mathrm{\Sigma }}_{\epsilon }^0\left)\right)}+\parallel {\mathbf{\text{g}}}_l^t{\parallel }_{L^2(0,T;H^{1/2}({\mathrm{\Sigma }}_{\epsilon }^l\left)\right)}))\\ & +C(\parallel {\mathbf{\text{g}}}_0^{nb}{\parallel }_{L^2(0,T;H^{1/2}({\mathrm{\Sigma }}_{\epsilon }^0\left)\right)}+\parallel {\mathbf{\text{g}}}_l^{nb}{\parallel }_{L^2(0,T;H^{1/2}({\mathrm{\Sigma }}_{\epsilon }^l\left)\right)}),\\ & ||\mathrm{\nabla }\frac{\mathrm{\partial }{\mathit{\varphi }}_{\mathit{\epsilon }}}{\mathrm{\partial }t}|{|}_{L^2(0,T;L^2({\mathrm{\Omega }}_{\epsilon }^{\alpha }\left)\right)}\\ & \le C(\frac{1}{\epsilon }||\frac{\mathrm{\partial }K}{\mathrm{\partial }t}|{|}_{L^2(0,T;L^2({\mathrm{\Omega }}_{\epsilon }^{\alpha }\left)\right)}+\frac{1}{\sqrt{\epsilon }}(||\frac{\mathrm{\partial }{\mathbf{\text{g}}}_0^t}{\mathrm{\partial }t}|{|}_{L^2(0,T;H^{1/2}({\mathrm{\Sigma }}_{\epsilon }^0\left)\right)}+||\frac{\mathrm{\partial }{\mathbf{\text{g}}}_l^t}{\mathrm{\partial }t}|{|}_{L^2(0,T;H^{1/2}({\mathrm{\Sigma }}_{\epsilon }^l\left)\right)})\\ & +C(||\frac{\mathrm{\partial }{\mathbf{\text{g}}}_0^{nb}}{\mathrm{\partial }t}|{|}_{L^2(0,T;H^{1/2}({\mathrm{\Sigma }}_{\epsilon }^0\left)\right)}+||\frac{\mathrm{\partial }{\mathbf{\text{g}}}_l^{nb}}{\mathrm{\partial }t}|{|}_{L^2(0,T;H^{1/2}({\mathrm{\Sigma }}_{\epsilon }^l\left)\right)}),\\ & ||\mathrm{\nabla }\frac{{\mathrm{\partial }}^2{\mathit{\varphi }}_{\mathit{\epsilon }}}{\mathrm{\partial }{t}^2}|{|}_{L^2(0,T;L^2({\mathrm{\Omega }}_{\epsilon }^{\alpha }\left)\right)}\\ & \le C(\frac{1}{\epsilon }||\frac{{\mathrm{\partial }}^{2}K}{\mathrm{\partial }{t}^2}|{|}_{L^2(0,T;L^2({\mathrm{\Omega }}_{\epsilon }^{\alpha }\left)\right)}+\frac{1}{\sqrt{\epsilon }}(||\frac{{\mathrm{\partial }}^2{\mathbf{\text{h}}}_0^t}{\mathrm{\partial }{t}^2}|{|}_{L^2(0,T;H^{1/2}({\mathrm{\Sigma }}_{\epsilon }^0\left)\right)}+||\frac{{\mathrm{\partial }}^2{\mathbf{\text{h}}}_l^t}{\mathrm{\partial }{t}^2}|{|}_{L^2(0,T;H^{1/2}({\mathrm{\Sigma }}_{\epsilon }^l\left)\right)})\\ & +C(||\frac{{\mathrm{\partial }}^2{\mathbf{\text{h}}}_0^{nb}}{\mathrm{\partial }{t}^2}|{|}_{L^2(0,T;H^{1/2}({\mathrm{\Sigma }}_{\epsilon }^0\left)\right)}+||\frac{{\mathrm{\partial }}^2{\mathbf{\text{h}}}_l^{nb}}{\mathrm{\partial }{t}^2}|{|}_{L^2(0,T;H^{1/2}({\mathrm{\Sigma }}_{\epsilon }^l\left)\right)}),\end{array}$(81) where the constant C>0 is independent of ε.

Remark 5.3

It can be easily shown that we need higher time regularity conditions for the given data to ensure that ${\displaystyle \frac{{\mathrm{\partial }}^{j}K}{\mathrm{\partial }{t}^j}}\in L^2(0,T;L^2({\mathrm{\Omega }}_{\epsilon }^{\alpha }\left)\right),{\displaystyle \frac{{\mathrm{\partial }}^j{\mathbf{\text{g}}}_0}{\mathrm{\partial }{t}^j}}\in L^2(0,T;H^{1/2}({\mathrm{\Sigma }}_{\epsilon }^0\left)\right),{\displaystyle \frac{{\mathrm{\partial }}^j{\mathbf{\text{g}}}_l}{\mathrm{\partial }{t}^j}}\in L^2(0,T;H^{1/2}({\mathrm{\Sigma }}_{\epsilon }^l\left)\right),j=0,1,2,$ namely: $\frac{{\mathrm{\partial }}^j\mathbf{\text{F}}}{\mathrm{\partial }{t}^j},\frac{{\mathrm{\partial }}^j\mathbf{\text{G}}}{\mathrm{\partial }{t}^j},\frac{\mathrm{\partial }{\mathbf{\text{h}}}_0^{\epsilon }}{\mathrm{\partial }{t}^j},\frac{\mathrm{\partial }{\mathbf{\text{h}}}_l^{\epsilon }}{\mathrm{\partial }{t}^j}\in L^2(0,T;L^2({\mathrm{\Omega }}_{\epsilon }^{\alpha }\left)\right),j=0,1,2,3.$

The next step is to derive the a priori estimates for the velocity, pressure and microrotation.

Proposition 5.4

Apriori estimates

Let $T\in ⟨0,\mathrm{\infty }⟩$ and let $({\mathbf{u}}_{\epsilon },p_{\epsilon },{\mathbf{w}}_{\epsilon })=({\mathbf{v}}_{\epsilon }+{\mathbf{h}}_{\epsilon },p_{\epsilon },{\mathbf{w}}_{\epsilon })$ be the solution of the problem (5(5) $\begin{array}{rl}& \frac{\mathrm{\partial }{\mathbf{\text{u}}}_{\epsilon }}{\mathrm{\partial }t}-\mu \mathrm{\Delta }{\mathbf{\text{u}}}_{\epsilon }+\mathrm{\nabla }{p}_{\epsilon }=a\text{rot}{\mathbf{\text{w}}}_{\epsilon }+\mathbf{\text{F}},\\ & \text{div}{\mathbf{\text{u}}}_{\epsilon }=0,\text{in}{\mathrm{\Omega }}_{\epsilon }^{\alpha },\\ & \frac{\mathrm{\partial }{\mathbf{\text{w}}}_{\epsilon }}{\mathrm{\partial }t}-\delta \mathrm{\Delta }{\mathbf{\text{w}}}_{\epsilon }-\beta \text{div}{\mathbf{\text{w}}}_{\epsilon }+2a{\mathbf{\text{w}}}_{\epsilon }=a\text{rot}{\mathbf{\text{u}}}_{\epsilon }+\mathbf{\text{G}}.\end{array}$(5) )–(11(11) ${\mathbf{\text{h}}}_0^{\epsilon }(t,\cdot ),{\mathbf{\text{h}}}_l^{\epsilon }(t,\cdot ),\mathbf{\text{F}}(t,\cdot ),\mathbf{\text{G}}(t,\cdot ),\mathrm{\forall }t\in [0,T^{\ast }],T^{\ast }>0.$(11) ). Then there exists a constant C>0, independent of ε, such that (82) $\begin{array}{rl}& \underset{t\in [0,T]}{sup}\parallel {\mathbf{v}}_{\epsilon }{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}^2+{\int }_0^T\parallel \mathrm{\nabla }{\mathbf{v}}_{\epsilon }{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}^2\le C{\epsilon }^4,\\ & \underset{t\in [0,T]}{sup}||\frac{\mathrm{\partial }{\mathbf{v}}_{\epsilon }}{\mathrm{\partial }t}|{|}_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}^2+{\int }_0^T||\mathrm{\nabla }\frac{\mathrm{\partial }{\mathbf{v}}_{\epsilon }}{\mathrm{\partial }t}|{|}_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}^2\le C{\epsilon }^4,\\ & \underset{t\in [0,T]}{sup}\parallel {\mathbf{w}}_{\epsilon }{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}^2+{\int }_0^T\parallel \mathrm{\nabla }{\mathbf{w}}_{\epsilon }{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}^2\le C{\epsilon }^4,\\ & \underset{t\in [0,T]}{sup}||\frac{\mathrm{\partial }{\mathbf{w}}_{\epsilon }}{\mathrm{\partial }t}|{|}_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}^2+{\int }_0^T||\mathrm{\nabla }\frac{\mathrm{\partial }{\mathbf{w}}_{\epsilon }}{\mathrm{\partial }t}|{|}_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}^2\le C{\epsilon }^4,\\ & \underset{t\in [0,T]}{sup}\parallel p_{\epsilon }{\parallel }_{L^2(0,T;L^2({\mathrm{\Omega }}_{\epsilon }^{\alpha }\left)\right)}^2\le C{\epsilon }^2.\end{array}$(82)

Proof.

Using ${\mathbf{\text{w}}}_{\epsilon }$ as a test function in the integral identity (12)₂ yields (83) $\begin{array}{rl}& \frac{1}{2}\frac{\mathrm{d}}{\mathrm{d}t}{\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}|{\mathbf{\text{w}}}_{\epsilon }{|}^2+\alpha {\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}|\mathrm{\nabla }{\mathbf{\text{w}}}_{\epsilon }{|}^2+\beta {\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}(\text{div}{\mathbf{\text{w}}}_{\epsilon }{)}^2+2a{\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}|{\mathbf{\text{w}}}_{\epsilon }{|}^2\\ & =a{\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}\text{rot}{\mathbf{\text{v}}}_{\epsilon }{\mathbf{\text{w}}}_{\epsilon }+a{\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}\text{rot}{\mathbf{\text{h}}}_{\epsilon }{\mathbf{\text{w}}}_{\epsilon }+{\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}\mathbf{\text{G}}{\mathbf{\text{w}}}_{\epsilon },\end{array}$(83) Using inequality (80(80) $\parallel {\mathit{\varphi }}_{\epsilon }(t,\cdot ){\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}\le C\epsilon \parallel \mathrm{\nabla }{\mathit{\varphi }}_{\epsilon }(t,\cdot ){\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)},$(80) ), we estimate the terms on the right–hand side of (83(83) $\begin{array}{rl}& \frac{1}{2}\frac{\mathrm{d}}{\mathrm{d}t}{\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}|{\mathbf{\text{w}}}_{\epsilon }{|}^2+\alpha {\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}|\mathrm{\nabla }{\mathbf{\text{w}}}_{\epsilon }{|}^2+\beta {\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}(\text{div}{\mathbf{\text{w}}}_{\epsilon }{)}^2+2a{\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}|{\mathbf{\text{w}}}_{\epsilon }{|}^2\\ & =a{\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}\text{rot}{\mathbf{\text{v}}}_{\epsilon }{\mathbf{\text{w}}}_{\epsilon }+a{\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}\text{rot}{\mathbf{\text{h}}}_{\epsilon }{\mathbf{\text{w}}}_{\epsilon }+{\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}\mathbf{\text{G}}{\mathbf{\text{w}}}_{\epsilon },\end{array}$(83) ) to obtain: (84) $\begin{array}{rl}& |{\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}\text{rot}{\mathbf{\text{v}}}_{\epsilon }{\mathbf{\text{w }}}_{\epsilon }|\le \parallel \text{rot}{\mathbf{\text{v}}}_{\epsilon }{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}\parallel {\mathbf{\text{w}}}_{\epsilon }{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}\le C\epsilon \parallel \mathrm{\nabla }{\mathbf{\text{v}}}_{\epsilon }{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}\parallel \mathrm{\nabla }{\mathbf{\text{w}}}_{\epsilon }{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)},\\ & |{\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}\text{rot}{\mathbf{\text{h}}}_{\epsilon }{\mathbf{\text{w}}}_{\epsilon }|\le \parallel \text{rot}{\mathbf{\text{h}}}_{\epsilon }{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}\parallel {\mathbf{\text{w}}}_{\epsilon }{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}\le C\epsilon \parallel \mathrm{\nabla }{\mathbf{\text{h}}}_{\epsilon }{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}\parallel \mathrm{\nabla }{\mathbf{\text{w}}}_{\epsilon }{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)},\\ & |{\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}\mathbf{\text{G}}{\mathbf{\text{w}}}_{\epsilon }|\le \parallel \mathbf{\text{G}}{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}\parallel {\mathbf{\text{w}}}_{\epsilon }{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}\le C{\epsilon }^2\parallel \mathrm{\nabla }{\mathbf{\text{w}}}_{\epsilon }{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}.\end{array}$(84) Using estimates (84(84) $\begin{array}{rl}& |{\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}\text{rot}{\mathbf{\text{v}}}_{\epsilon }{\mathbf{\text{w }}}_{\epsilon }|\le \parallel \text{rot}{\mathbf{\text{v}}}_{\epsilon }{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}\parallel {\mathbf{\text{w}}}_{\epsilon }{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}\le C\epsilon \parallel \mathrm{\nabla }{\mathbf{\text{v}}}_{\epsilon }{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}\parallel \mathrm{\nabla }{\mathbf{\text{w}}}_{\epsilon }{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)},\\ & |{\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}\text{rot}{\mathbf{\text{h}}}_{\epsilon }{\mathbf{\text{w}}}_{\epsilon }|\le \parallel \text{rot}{\mathbf{\text{h}}}_{\epsilon }{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}\parallel {\mathbf{\text{w}}}_{\epsilon }{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}\le C\epsilon \parallel \mathrm{\nabla }{\mathbf{\text{h}}}_{\epsilon }{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}\parallel \mathrm{\nabla }{\mathbf{\text{w}}}_{\epsilon }{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)},\\ & |{\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}\mathbf{\text{G}}{\mathbf{\text{w}}}_{\epsilon }|\le \parallel \mathbf{\text{G}}{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}\parallel {\mathbf{\text{w}}}_{\epsilon }{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}\le C{\epsilon }^2\parallel \mathrm{\nabla }{\mathbf{\text{w}}}_{\epsilon }{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}.\end{array}$(84) ), Young's inequality, integrating over t in equation (83(83) $\begin{array}{rl}& \frac{1}{2}\frac{\mathrm{d}}{\mathrm{d}t}{\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}|{\mathbf{\text{w}}}_{\epsilon }{|}^2+\alpha {\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}|\mathrm{\nabla }{\mathbf{\text{w}}}_{\epsilon }{|}^2+\beta {\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}(\text{div}{\mathbf{\text{w}}}_{\epsilon }{)}^2+2a{\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}|{\mathbf{\text{w}}}_{\epsilon }{|}^2\\ & =a{\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}\text{rot}{\mathbf{\text{v}}}_{\epsilon }{\mathbf{\text{w}}}_{\epsilon }+a{\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}\text{rot}{\mathbf{\text{h}}}_{\epsilon }{\mathbf{\text{w}}}_{\epsilon }+{\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}\mathbf{\text{G}}{\mathbf{\text{w}}}_{\epsilon },\end{array}$(83) ) and applying estimate (81(81) $\begin{array}{rl}& \parallel \mathrm{\nabla }{\mathit{\varphi }}_{\epsilon }{\parallel }_{L^2(0,T;L^2({\mathrm{\Omega }}_{\epsilon }^{\alpha }\left)\right)}\\ & \le C(\frac{1}{\epsilon }\parallel K{\parallel }_{L^2(0,T;L^2({\mathrm{\Omega }}_{\epsilon }^{\alpha }\left)\right)}+\frac{1}{\sqrt{\epsilon }}(\parallel {\mathbf{\text{g}}}_0^t{\parallel }_{L^2(0,T;H^{1/2}({\mathrm{\Sigma }}_{\epsilon }^0\left)\right)}+\parallel {\mathbf{\text{g}}}_l^t{\parallel }_{L^2(0,T;H^{1/2}({\mathrm{\Sigma }}_{\epsilon }^l\left)\right)}))\\ & +C(\parallel {\mathbf{\text{g}}}_0^{nb}{\parallel }_{L^2(0,T;H^{1/2}({\mathrm{\Sigma }}_{\epsilon }^0\left)\right)}+\parallel {\mathbf{\text{g}}}_l^{nb}{\parallel }_{L^2(0,T;H^{1/2}({\mathrm{\Sigma }}_{\epsilon }^l\left)\right)}),\\ & ||\mathrm{\nabla }\frac{\mathrm{\partial }{\mathit{\varphi }}_{\mathit{\epsilon }}}{\mathrm{\partial }t}|{|}_{L^2(0,T;L^2({\mathrm{\Omega }}_{\epsilon }^{\alpha }\left)\right)}\\ & \le C(\frac{1}{\epsilon }||\frac{\mathrm{\partial }K}{\mathrm{\partial }t}|{|}_{L^2(0,T;L^2({\mathrm{\Omega }}_{\epsilon }^{\alpha }\left)\right)}+\frac{1}{\sqrt{\epsilon }}(||\frac{\mathrm{\partial }{\mathbf{\text{g}}}_0^t}{\mathrm{\partial }t}|{|}_{L^2(0,T;H^{1/2}({\mathrm{\Sigma }}_{\epsilon }^0\left)\right)}+||\frac{\mathrm{\partial }{\mathbf{\text{g}}}_l^t}{\mathrm{\partial }t}|{|}_{L^2(0,T;H^{1/2}({\mathrm{\Sigma }}_{\epsilon }^l\left)\right)})\\ & +C(||\frac{\mathrm{\partial }{\mathbf{\text{g}}}_0^{nb}}{\mathrm{\partial }t}|{|}_{L^2(0,T;H^{1/2}({\mathrm{\Sigma }}_{\epsilon }^0\left)\right)}+||\frac{\mathrm{\partial }{\mathbf{\text{g}}}_l^{nb}}{\mathrm{\partial }t}|{|}_{L^2(0,T;H^{1/2}({\mathrm{\Sigma }}_{\epsilon }^l\left)\right)}),\\ & ||\mathrm{\nabla }\frac{{\mathrm{\partial }}^2{\mathit{\varphi }}_{\mathit{\epsilon }}}{\mathrm{\partial }{t}^2}|{|}_{L^2(0,T;L^2({\mathrm{\Omega }}_{\epsilon }^{\alpha }\left)\right)}\\ & \le C(\frac{1}{\epsilon }||\frac{{\mathrm{\partial }}^{2}K}{\mathrm{\partial }{t}^2}|{|}_{L^2(0,T;L^2({\mathrm{\Omega }}_{\epsilon }^{\alpha }\left)\right)}+\frac{1}{\sqrt{\epsilon }}(||\frac{{\mathrm{\partial }}^2{\mathbf{\text{h}}}_0^t}{\mathrm{\partial }{t}^2}|{|}_{L^2(0,T;H^{1/2}({\mathrm{\Sigma }}_{\epsilon }^0\left)\right)}+||\frac{{\mathrm{\partial }}^2{\mathbf{\text{h}}}_l^t}{\mathrm{\partial }{t}^2}|{|}_{L^2(0,T;H^{1/2}({\mathrm{\Sigma }}_{\epsilon }^l\left)\right)})\\ & +C(||\frac{{\mathrm{\partial }}^2{\mathbf{\text{h}}}_0^{nb}}{\mathrm{\partial }{t}^2}|{|}_{L^2(0,T;H^{1/2}({\mathrm{\Sigma }}_{\epsilon }^0\left)\right)}+||\frac{{\mathrm{\partial }}^2{\mathbf{\text{h}}}_l^{nb}}{\mathrm{\partial }{t}^2}|{|}_{L^2(0,T;H^{1/2}({\mathrm{\Sigma }}_{\epsilon }^l\left)\right)}),\end{array}$(81) )₁ we have: (85) $\underset{t\in [0,T]}{sup}\parallel {\mathbf{\text{w}}}_{\epsilon }{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}^2+{\int }_0^T\parallel \mathrm{\nabla }{\mathbf{\text{w}}}_{\epsilon }{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}^2\le C{\epsilon }^2{\int }_0^T\parallel \mathrm{\nabla }{\mathbf{\text{v}}}_{\epsilon }{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}^2+C{\epsilon }^4.$(85) Differentiating the integral identity (12)₂ and taking ${\displaystyle \frac{\mathrm{\partial }{\mathbf{\text{w}}}_{\epsilon }}{\mathrm{\partial }t}}$ as a test function, we obtain: (86) $\begin{array}{rl}& \frac{1}{2}\frac{\mathrm{d}}{\mathrm{d}t}{\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}|\frac{\mathrm{\partial }{\mathbf{\text{w}}}_{\epsilon }}{\mathrm{\partial }t}{|}^2+\alpha {\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}|\mathrm{\nabla }\frac{\mathrm{\partial }{\mathbf{\text{w}}}_{\epsilon }}{\mathrm{\partial }t}{|}^2+\beta {\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}(\text{div}\frac{\mathrm{\partial }\mathbf{\text{w}}}{\mathrm{\partial }t}{)}^2+2a{\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}|\frac{\mathrm{\partial }{\mathbf{\text{w}}}_{\epsilon }}{\mathrm{\partial }t}{|}^2\\ & =a{\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}\text{rot}\frac{\mathrm{\partial }{\mathbf{\text{v}}}_{\epsilon }}{\mathrm{\partial }t}\frac{\mathrm{\partial }{\mathbf{\text{w}}}_{\epsilon }}{\mathrm{\partial }t}+a{\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}\text{rot}\frac{\mathrm{\partial }{\mathbf{\text{h}}}_{\epsilon }}{\mathrm{\partial }t}\frac{\mathrm{\partial }{\mathbf{\text{w}}}_{\epsilon }}{\mathrm{\partial }t}+{\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}\frac{\mathrm{\partial }\mathbf{\text{G}}}{\mathrm{\partial }t}\frac{\mathrm{\partial }{\mathbf{\text{w}}}_{\epsilon }}{\mathrm{\partial }t}.\end{array}$(86) Again, estimating the terms on the right–hand side as above, using Young's inequality, integrating over t in (86(86) $\begin{array}{rl}& \frac{1}{2}\frac{\mathrm{d}}{\mathrm{d}t}{\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}|\frac{\mathrm{\partial }{\mathbf{\text{w}}}_{\epsilon }}{\mathrm{\partial }t}{|}^2+\alpha {\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}|\mathrm{\nabla }\frac{\mathrm{\partial }{\mathbf{\text{w}}}_{\epsilon }}{\mathrm{\partial }t}{|}^2+\beta {\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}(\text{div}\frac{\mathrm{\partial }\mathbf{\text{w}}}{\mathrm{\partial }t}{)}^2+2a{\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}|\frac{\mathrm{\partial }{\mathbf{\text{w}}}_{\epsilon }}{\mathrm{\partial }t}{|}^2\\ & =a{\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}\text{rot}\frac{\mathrm{\partial }{\mathbf{\text{v}}}_{\epsilon }}{\mathrm{\partial }t}\frac{\mathrm{\partial }{\mathbf{\text{w}}}_{\epsilon }}{\mathrm{\partial }t}+a{\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}\text{rot}\frac{\mathrm{\partial }{\mathbf{\text{h}}}_{\epsilon }}{\mathrm{\partial }t}\frac{\mathrm{\partial }{\mathbf{\text{w}}}_{\epsilon }}{\mathrm{\partial }t}+{\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}\frac{\mathrm{\partial }\mathbf{\text{G}}}{\mathrm{\partial }t}\frac{\mathrm{\partial }{\mathbf{\text{w}}}_{\epsilon }}{\mathrm{\partial }t}.\end{array}$(86) ) and using (81(81) $\begin{array}{rl}& \parallel \mathrm{\nabla }{\mathit{\varphi }}_{\epsilon }{\parallel }_{L^2(0,T;L^2({\mathrm{\Omega }}_{\epsilon }^{\alpha }\left)\right)}\\ & \le C(\frac{1}{\epsilon }\parallel K{\parallel }_{L^2(0,T;L^2({\mathrm{\Omega }}_{\epsilon }^{\alpha }\left)\right)}+\frac{1}{\sqrt{\epsilon }}(\parallel {\mathbf{\text{g}}}_0^t{\parallel }_{L^2(0,T;H^{1/2}({\mathrm{\Sigma }}_{\epsilon }^0\left)\right)}+\parallel {\mathbf{\text{g}}}_l^t{\parallel }_{L^2(0,T;H^{1/2}({\mathrm{\Sigma }}_{\epsilon }^l\left)\right)}))\\ & +C(\parallel {\mathbf{\text{g}}}_0^{nb}{\parallel }_{L^2(0,T;H^{1/2}({\mathrm{\Sigma }}_{\epsilon }^0\left)\right)}+\parallel {\mathbf{\text{g}}}_l^{nb}{\parallel }_{L^2(0,T;H^{1/2}({\mathrm{\Sigma }}_{\epsilon }^l\left)\right)}),\\ & ||\mathrm{\nabla }\frac{\mathrm{\partial }{\mathit{\varphi }}_{\mathit{\epsilon }}}{\mathrm{\partial }t}|{|}_{L^2(0,T;L^2({\mathrm{\Omega }}_{\epsilon }^{\alpha }\left)\right)}\\ & \le C(\frac{1}{\epsilon }||\frac{\mathrm{\partial }K}{\mathrm{\partial }t}|{|}_{L^2(0,T;L^2({\mathrm{\Omega }}_{\epsilon }^{\alpha }\left)\right)}+\frac{1}{\sqrt{\epsilon }}(||\frac{\mathrm{\partial }{\mathbf{\text{g}}}_0^t}{\mathrm{\partial }t}|{|}_{L^2(0,T;H^{1/2}({\mathrm{\Sigma }}_{\epsilon }^0\left)\right)}+||\frac{\mathrm{\partial }{\mathbf{\text{g}}}_l^t}{\mathrm{\partial }t}|{|}_{L^2(0,T;H^{1/2}({\mathrm{\Sigma }}_{\epsilon }^l\left)\right)})\\ & +C(||\frac{\mathrm{\partial }{\mathbf{\text{g}}}_0^{nb}}{\mathrm{\partial }t}|{|}_{L^2(0,T;H^{1/2}({\mathrm{\Sigma }}_{\epsilon }^0\left)\right)}+||\frac{\mathrm{\partial }{\mathbf{\text{g}}}_l^{nb}}{\mathrm{\partial }t}|{|}_{L^2(0,T;H^{1/2}({\mathrm{\Sigma }}_{\epsilon }^l\left)\right)}),\\ & ||\mathrm{\nabla }\frac{{\mathrm{\partial }}^2{\mathit{\varphi }}_{\mathit{\epsilon }}}{\mathrm{\partial }{t}^2}|{|}_{L^2(0,T;L^2({\mathrm{\Omega }}_{\epsilon }^{\alpha }\left)\right)}\\ & \le C(\frac{1}{\epsilon }||\frac{{\mathrm{\partial }}^{2}K}{\mathrm{\partial }{t}^2}|{|}_{L^2(0,T;L^2({\mathrm{\Omega }}_{\epsilon }^{\alpha }\left)\right)}+\frac{1}{\sqrt{\epsilon }}(||\frac{{\mathrm{\partial }}^2{\mathbf{\text{h}}}_0^t}{\mathrm{\partial }{t}^2}|{|}_{L^2(0,T;H^{1/2}({\mathrm{\Sigma }}_{\epsilon }^0\left)\right)}+||\frac{{\mathrm{\partial }}^2{\mathbf{\text{h}}}_l^t}{\mathrm{\partial }{t}^2}|{|}_{L^2(0,T;H^{1/2}({\mathrm{\Sigma }}_{\epsilon }^l\left)\right)})\\ & +C(||\frac{{\mathrm{\partial }}^2{\mathbf{\text{h}}}_0^{nb}}{\mathrm{\partial }{t}^2}|{|}_{L^2(0,T;H^{1/2}({\mathrm{\Sigma }}_{\epsilon }^0\left)\right)}+||\frac{{\mathrm{\partial }}^2{\mathbf{\text{h}}}_l^{nb}}{\mathrm{\partial }{t}^2}|{|}_{L^2(0,T;H^{1/2}({\mathrm{\Sigma }}_{\epsilon }^l\left)\right)}),\end{array}$(81) )₂, we obtain : (87) $\underset{t\in [0,T]}{sup}||\frac{\mathrm{\partial }{\mathbf{\text{w}}}_{\epsilon }}{\mathrm{\partial }t}|{|}_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}^2+{\int }_0^T||\mathrm{\nabla }\frac{\mathrm{\partial }{\mathbf{\text{w}}}_{\epsilon }}{\mathrm{\partial }t}|{|}_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}^2\le C{\epsilon }^2{\int }_0^T||\mathrm{\nabla }\frac{\mathrm{\partial }{\mathbf{\text{v}}}_{\epsilon }}{\mathrm{\partial }t}|{|}_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}^2+C{\epsilon }^4.$(87)

We now multiply the integral identity (12)₁ with ${\mathbf{\text{v}}}_{\epsilon }$ and integrate over ${\mathrm{\Omega }}_{\epsilon }^{\alpha }$ to obtain (88) $\frac{1}{2}\frac{\mathrm{d}}{\mathrm{d}t}{\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}|{\mathbf{\text{v}}}_{\epsilon }{|}^2+\mu {\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}|\mathrm{\nabla }{\mathbf{\text{v}}}_{\epsilon }{|}^2=a{\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}\text{rot}{\mathbf{\text{w}}}_{\epsilon }{\mathbf{\text{v}}}_{\epsilon }+{\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}\mathbf{\text{F}}{\mathbf{\text{v}}}_{\epsilon }-{\int }_{{\mathrm{\Omega }}_{\epsilon }}\frac{\mathrm{\partial }{\mathbf{\text{h}}}_{\epsilon }}{\mathrm{\partial }t}{\mathbf{\text{v}}}_{\epsilon }-{\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}\mathrm{\nabla }{\mathbf{\text{h}}}_{\epsilon }\mathrm{\nabla }{\mathbf{\text{v}}}_{\epsilon }.$(88) We carefully estimate the terms on the right–hand side of (88(88) $\frac{1}{2}\frac{\mathrm{d}}{\mathrm{d}t}{\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}|{\mathbf{\text{v}}}_{\epsilon }{|}^2+\mu {\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}|\mathrm{\nabla }{\mathbf{\text{v}}}_{\epsilon }{|}^2=a{\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}\text{rot}{\mathbf{\text{w}}}_{\epsilon }{\mathbf{\text{v}}}_{\epsilon }+{\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}\mathbf{\text{F}}{\mathbf{\text{v}}}_{\epsilon }-{\int }_{{\mathrm{\Omega }}_{\epsilon }}\frac{\mathrm{\partial }{\mathbf{\text{h}}}_{\epsilon }}{\mathrm{\partial }t}{\mathbf{\text{v}}}_{\epsilon }-{\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}\mathrm{\nabla }{\mathbf{\text{h}}}_{\epsilon }\mathrm{\nabla }{\mathbf{\text{v}}}_{\epsilon }.$(88) ) as follows: (89) $\begin{array}{rl}& |{\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}\text{rot}{\mathbf{\text{w}}}_{\epsilon }{\mathbf{\text{v}}}_{\epsilon }|\le C\epsilon \parallel \mathrm{\nabla }{\mathbf{\text{v}}}_{\epsilon }{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}\parallel \mathrm{\nabla }{\mathbf{\text{w}}}_{\epsilon }{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)},\\ & |{\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}\mathbf{\text{F}}{\mathbf{\text{v}}}_{\epsilon }|\le \parallel \mathbf{\text{F}}{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}\parallel {\mathbf{\text{v}}}_{\epsilon }{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}\le C{\epsilon }^2\parallel \mathrm{\nabla }{\mathbf{\text{v}}}_{\epsilon }{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)},\\ & |{\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}\frac{\mathrm{\partial }{\mathbf{\text{h}}}_{\epsilon }}{\mathrm{\partial }t}{\mathbf{\text{v}}}_{\epsilon }|\le ||\frac{\mathrm{\partial }{\mathbf{\text{h}}}_{\epsilon }}{\mathrm{\partial }t}|{|}_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}\parallel {\mathbf{\text{v}}}_{\epsilon }{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}\le C{\epsilon }^2||\mathrm{\nabla }\frac{\mathrm{\partial }{\mathbf{\text{h}}}_{\epsilon }}{\mathrm{\partial }t}|{|}_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}\parallel \mathrm{\nabla }{\mathbf{\text{v}}}_{\epsilon }{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)},\\ & |{\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}\mathrm{\nabla }{\mathbf{\text{h}}}_{\epsilon }\mathrm{\nabla }{\mathbf{\text{v}}}_{\epsilon }|\le \parallel \mathrm{\nabla }{\mathbf{\text{h}}}_{\epsilon }{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}\parallel \mathrm{\nabla }{\mathbf{\text{v}}}_{\epsilon }{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}.\end{array}$(89) Applying (89(89) $\begin{array}{rl}& |{\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}\text{rot}{\mathbf{\text{w}}}_{\epsilon }{\mathbf{\text{v}}}_{\epsilon }|\le C\epsilon \parallel \mathrm{\nabla }{\mathbf{\text{v}}}_{\epsilon }{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}\parallel \mathrm{\nabla }{\mathbf{\text{w}}}_{\epsilon }{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)},\\ & |{\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}\mathbf{\text{F}}{\mathbf{\text{v}}}_{\epsilon }|\le \parallel \mathbf{\text{F}}{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}\parallel {\mathbf{\text{v}}}_{\epsilon }{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}\le C{\epsilon }^2\parallel \mathrm{\nabla }{\mathbf{\text{v}}}_{\epsilon }{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)},\\ & |{\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}\frac{\mathrm{\partial }{\mathbf{\text{h}}}_{\epsilon }}{\mathrm{\partial }t}{\mathbf{\text{v}}}_{\epsilon }|\le ||\frac{\mathrm{\partial }{\mathbf{\text{h}}}_{\epsilon }}{\mathrm{\partial }t}|{|}_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}\parallel {\mathbf{\text{v}}}_{\epsilon }{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}\le C{\epsilon }^2||\mathrm{\nabla }\frac{\mathrm{\partial }{\mathbf{\text{h}}}_{\epsilon }}{\mathrm{\partial }t}|{|}_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}\parallel \mathrm{\nabla }{\mathbf{\text{v}}}_{\epsilon }{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)},\\ & |{\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}\mathrm{\nabla }{\mathbf{\text{h}}}_{\epsilon }\mathrm{\nabla }{\mathbf{\text{v}}}_{\epsilon }|\le \parallel \mathrm{\nabla }{\mathbf{\text{h}}}_{\epsilon }{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}\parallel \mathrm{\nabla }{\mathbf{\text{v}}}_{\epsilon }{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}.\end{array}$(89) ) and Young's inequality on the right–hand side of (88(88) $\frac{1}{2}\frac{\mathrm{d}}{\mathrm{d}t}{\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}|{\mathbf{\text{v}}}_{\epsilon }{|}^2+\mu {\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}|\mathrm{\nabla }{\mathbf{\text{v}}}_{\epsilon }{|}^2=a{\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}\text{rot}{\mathbf{\text{w}}}_{\epsilon }{\mathbf{\text{v}}}_{\epsilon }+{\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}\mathbf{\text{F}}{\mathbf{\text{v}}}_{\epsilon }-{\int }_{{\mathrm{\Omega }}_{\epsilon }}\frac{\mathrm{\partial }{\mathbf{\text{h}}}_{\epsilon }}{\mathrm{\partial }t}{\mathbf{\text{v}}}_{\epsilon }-{\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}\mathrm{\nabla }{\mathbf{\text{h}}}_{\epsilon }\mathrm{\nabla }{\mathbf{\text{v}}}_{\epsilon }.$(88) ), integrating over t and using estimates (81(81) $\begin{array}{rl}& \parallel \mathrm{\nabla }{\mathit{\varphi }}_{\epsilon }{\parallel }_{L^2(0,T;L^2({\mathrm{\Omega }}_{\epsilon }^{\alpha }\left)\right)}\\ & \le C(\frac{1}{\epsilon }\parallel K{\parallel }_{L^2(0,T;L^2({\mathrm{\Omega }}_{\epsilon }^{\alpha }\left)\right)}+\frac{1}{\sqrt{\epsilon }}(\parallel {\mathbf{\text{g}}}_0^t{\parallel }_{L^2(0,T;H^{1/2}({\mathrm{\Sigma }}_{\epsilon }^0\left)\right)}+\parallel {\mathbf{\text{g}}}_l^t{\parallel }_{L^2(0,T;H^{1/2}({\mathrm{\Sigma }}_{\epsilon }^l\left)\right)}))\\ & +C(\parallel {\mathbf{\text{g}}}_0^{nb}{\parallel }_{L^2(0,T;H^{1/2}({\mathrm{\Sigma }}_{\epsilon }^0\left)\right)}+\parallel {\mathbf{\text{g}}}_l^{nb}{\parallel }_{L^2(0,T;H^{1/2}({\mathrm{\Sigma }}_{\epsilon }^l\left)\right)}),\\ & ||\mathrm{\nabla }\frac{\mathrm{\partial }{\mathit{\varphi }}_{\mathit{\epsilon }}}{\mathrm{\partial }t}|{|}_{L^2(0,T;L^2({\mathrm{\Omega }}_{\epsilon }^{\alpha }\left)\right)}\\ & \le C(\frac{1}{\epsilon }||\frac{\mathrm{\partial }K}{\mathrm{\partial }t}|{|}_{L^2(0,T;L^2({\mathrm{\Omega }}_{\epsilon }^{\alpha }\left)\right)}+\frac{1}{\sqrt{\epsilon }}(||\frac{\mathrm{\partial }{\mathbf{\text{g}}}_0^t}{\mathrm{\partial }t}|{|}_{L^2(0,T;H^{1/2}({\mathrm{\Sigma }}_{\epsilon }^0\left)\right)}+||\frac{\mathrm{\partial }{\mathbf{\text{g}}}_l^t}{\mathrm{\partial }t}|{|}_{L^2(0,T;H^{1/2}({\mathrm{\Sigma }}_{\epsilon }^l\left)\right)})\\ & +C(||\frac{\mathrm{\partial }{\mathbf{\text{g}}}_0^{nb}}{\mathrm{\partial }t}|{|}_{L^2(0,T;H^{1/2}({\mathrm{\Sigma }}_{\epsilon }^0\left)\right)}+||\frac{\mathrm{\partial }{\mathbf{\text{g}}}_l^{nb}}{\mathrm{\partial }t}|{|}_{L^2(0,T;H^{1/2}({\mathrm{\Sigma }}_{\epsilon }^l\left)\right)}),\\ & ||\mathrm{\nabla }\frac{{\mathrm{\partial }}^2{\mathit{\varphi }}_{\mathit{\epsilon }}}{\mathrm{\partial }{t}^2}|{|}_{L^2(0,T;L^2({\mathrm{\Omega }}_{\epsilon }^{\alpha }\left)\right)}\\ & \le C(\frac{1}{\epsilon }||\frac{{\mathrm{\partial }}^{2}K}{\mathrm{\partial }{t}^2}|{|}_{L^2(0,T;L^2({\mathrm{\Omega }}_{\epsilon }^{\alpha }\left)\right)}+\frac{1}{\sqrt{\epsilon }}(||\frac{{\mathrm{\partial }}^2{\mathbf{\text{h}}}_0^t}{\mathrm{\partial }{t}^2}|{|}_{L^2(0,T;H^{1/2}({\mathrm{\Sigma }}_{\epsilon }^0\left)\right)}+||\frac{{\mathrm{\partial }}^2{\mathbf{\text{h}}}_l^t}{\mathrm{\partial }{t}^2}|{|}_{L^2(0,T;H^{1/2}({\mathrm{\Sigma }}_{\epsilon }^l\left)\right)})\\ & +C(||\frac{{\mathrm{\partial }}^2{\mathbf{\text{h}}}_0^{nb}}{\mathrm{\partial }{t}^2}|{|}_{L^2(0,T;H^{1/2}({\mathrm{\Sigma }}_{\epsilon }^0\left)\right)}+||\frac{{\mathrm{\partial }}^2{\mathbf{\text{h}}}_l^{nb}}{\mathrm{\partial }{t}^2}|{|}_{L^2(0,T;H^{1/2}({\mathrm{\Sigma }}_{\epsilon }^l\left)\right)}),\end{array}$(81) )₁–(81(81) $\begin{array}{rl}& \parallel \mathrm{\nabla }{\mathit{\varphi }}_{\epsilon }{\parallel }_{L^2(0,T;L^2({\mathrm{\Omega }}_{\epsilon }^{\alpha }\left)\right)}\\ & \le C(\frac{1}{\epsilon }\parallel K{\parallel }_{L^2(0,T;L^2({\mathrm{\Omega }}_{\epsilon }^{\alpha }\left)\right)}+\frac{1}{\sqrt{\epsilon }}(\parallel {\mathbf{\text{g}}}_0^t{\parallel }_{L^2(0,T;H^{1/2}({\mathrm{\Sigma }}_{\epsilon }^0\left)\right)}+\parallel {\mathbf{\text{g}}}_l^t{\parallel }_{L^2(0,T;H^{1/2}({\mathrm{\Sigma }}_{\epsilon }^l\left)\right)}))\\ & +C(\parallel {\mathbf{\text{g}}}_0^{nb}{\parallel }_{L^2(0,T;H^{1/2}({\mathrm{\Sigma }}_{\epsilon }^0\left)\right)}+\parallel {\mathbf{\text{g}}}_l^{nb}{\parallel }_{L^2(0,T;H^{1/2}({\mathrm{\Sigma }}_{\epsilon }^l\left)\right)}),\\ & ||\mathrm{\nabla }\frac{\mathrm{\partial }{\mathit{\varphi }}_{\mathit{\epsilon }}}{\mathrm{\partial }t}|{|}_{L^2(0,T;L^2({\mathrm{\Omega }}_{\epsilon }^{\alpha }\left)\right)}\\ & \le C(\frac{1}{\epsilon }||\frac{\mathrm{\partial }K}{\mathrm{\partial }t}|{|}_{L^2(0,T;L^2({\mathrm{\Omega }}_{\epsilon }^{\alpha }\left)\right)}+\frac{1}{\sqrt{\epsilon }}(||\frac{\mathrm{\partial }{\mathbf{\text{g}}}_0^t}{\mathrm{\partial }t}|{|}_{L^2(0,T;H^{1/2}({\mathrm{\Sigma }}_{\epsilon }^0\left)\right)}+||\frac{\mathrm{\partial }{\mathbf{\text{g}}}_l^t}{\mathrm{\partial }t}|{|}_{L^2(0,T;H^{1/2}({\mathrm{\Sigma }}_{\epsilon }^l\left)\right)})\\ & +C(||\frac{\mathrm{\partial }{\mathbf{\text{g}}}_0^{nb}}{\mathrm{\partial }t}|{|}_{L^2(0,T;H^{1/2}({\mathrm{\Sigma }}_{\epsilon }^0\left)\right)}+||\frac{\mathrm{\partial }{\mathbf{\text{g}}}_l^{nb}}{\mathrm{\partial }t}|{|}_{L^2(0,T;H^{1/2}({\mathrm{\Sigma }}_{\epsilon }^l\left)\right)}),\\ & ||\mathrm{\nabla }\frac{{\mathrm{\partial }}^2{\mathit{\varphi }}_{\mathit{\epsilon }}}{\mathrm{\partial }{t}^2}|{|}_{L^2(0,T;L^2({\mathrm{\Omega }}_{\epsilon }^{\alpha }\left)\right)}\\ & \le C(\frac{1}{\epsilon }||\frac{{\mathrm{\partial }}^{2}K}{\mathrm{\partial }{t}^2}|{|}_{L^2(0,T;L^2({\mathrm{\Omega }}_{\epsilon }^{\alpha }\left)\right)}+\frac{1}{\sqrt{\epsilon }}(||\frac{{\mathrm{\partial }}^2{\mathbf{\text{h}}}_0^t}{\mathrm{\partial }{t}^2}|{|}_{L^2(0,T;H^{1/2}({\mathrm{\Sigma }}_{\epsilon }^0\left)\right)}+||\frac{{\mathrm{\partial }}^2{\mathbf{\text{h}}}_l^t}{\mathrm{\partial }{t}^2}|{|}_{L^2(0,T;H^{1/2}({\mathrm{\Sigma }}_{\epsilon }^l\left)\right)})\\ & +C(||\frac{{\mathrm{\partial }}^2{\mathbf{\text{h}}}_0^{nb}}{\mathrm{\partial }{t}^2}|{|}_{L^2(0,T;H^{1/2}({\mathrm{\Sigma }}_{\epsilon }^0\left)\right)}+||\frac{{\mathrm{\partial }}^2{\mathbf{\text{h}}}_l^{nb}}{\mathrm{\partial }{t}^2}|{|}_{L^2(0,T;H^{1/2}({\mathrm{\Sigma }}_{\epsilon }^l\left)\right)}),\end{array}$(81) )₂, we have (90) $\underset{t\in [0,T]}{sup}\parallel {\mathbf{\text{v}}}_{\epsilon }{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}^2+{\int }_0^T\parallel \mathrm{\nabla }{\mathbf{\text{v}}}_{\epsilon }{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}^2\le C{\epsilon }^2{\int }_0^T\parallel \mathrm{\nabla }{\mathbf{\text{w}}}_{\epsilon }{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}^2+C{\epsilon }^4.$(90) Finally, combining the estimates (85(85) $\underset{t\in [0,T]}{sup}\parallel {\mathbf{\text{w}}}_{\epsilon }{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}^2+{\int }_0^T\parallel \mathrm{\nabla }{\mathbf{\text{w}}}_{\epsilon }{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}^2\le C{\epsilon }^2{\int }_0^T\parallel \mathrm{\nabla }{\mathbf{\text{v}}}_{\epsilon }{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}^2+C{\epsilon }^4.$(85) ) and (90(90) $\underset{t\in [0,T]}{sup}\parallel {\mathbf{\text{v}}}_{\epsilon }{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}^2+{\int }_0^T\parallel \mathrm{\nabla }{\mathbf{\text{v}}}_{\epsilon }{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}^2\le C{\epsilon }^2{\int }_0^T\parallel \mathrm{\nabla }{\mathbf{\text{w}}}_{\epsilon }{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}^2+C{\epsilon }^4.$(90) ), for sufficiently small ε, we obtain: (91) $\underset{t\in [0,T]}{sup}\parallel {\mathbf{\text{v}}}_{\epsilon }{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}^2+{\int }_0^T\parallel \mathrm{\nabla }{\mathbf{\text{v}}}_{\epsilon }{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}^2\le C{\epsilon }^4.$(91) and (92) $\underset{t\in [0,T]}{sup}\parallel {\mathbf{\text{w}}}_{\epsilon }{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}^2+{\int }_0^T\parallel \mathrm{\nabla }{\mathbf{\text{w}}}_{\epsilon }{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}^2\le C{\epsilon }^4$(92) proving (82(82) $\begin{array}{rl}& \underset{t\in [0,T]}{sup}\parallel {\mathbf{v}}_{\epsilon }{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}^2+{\int }_0^T\parallel \mathrm{\nabla }{\mathbf{v}}_{\epsilon }{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}^2\le C{\epsilon }^4,\\ & \underset{t\in [0,T]}{sup}||\frac{\mathrm{\partial }{\mathbf{v}}_{\epsilon }}{\mathrm{\partial }t}|{|}_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}^2+{\int }_0^T||\mathrm{\nabla }\frac{\mathrm{\partial }{\mathbf{v}}_{\epsilon }}{\mathrm{\partial }t}|{|}_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}^2\le C{\epsilon }^4,\\ & \underset{t\in [0,T]}{sup}\parallel {\mathbf{w}}_{\epsilon }{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}^2+{\int }_0^T\parallel \mathrm{\nabla }{\mathbf{w}}_{\epsilon }{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}^2\le C{\epsilon }^4,\\ & \underset{t\in [0,T]}{sup}||\frac{\mathrm{\partial }{\mathbf{w}}_{\epsilon }}{\mathrm{\partial }t}|{|}_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}^2+{\int }_0^T||\mathrm{\nabla }\frac{\mathrm{\partial }{\mathbf{w}}_{\epsilon }}{\mathrm{\partial }t}|{|}_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}^2\le C{\epsilon }^4,\\ & \underset{t\in [0,T]}{sup}\parallel p_{\epsilon }{\parallel }_{L^2(0,T;L^2({\mathrm{\Omega }}_{\epsilon }^{\alpha }\left)\right)}^2\le C{\epsilon }^2.\end{array}$(82) )₁ and (82(82) $\begin{array}{rl}& \underset{t\in [0,T]}{sup}\parallel {\mathbf{v}}_{\epsilon }{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}^2+{\int }_0^T\parallel \mathrm{\nabla }{\mathbf{v}}_{\epsilon }{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}^2\le C{\epsilon }^4,\\ & \underset{t\in [0,T]}{sup}||\frac{\mathrm{\partial }{\mathbf{v}}_{\epsilon }}{\mathrm{\partial }t}|{|}_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}^2+{\int }_0^T||\mathrm{\nabla }\frac{\mathrm{\partial }{\mathbf{v}}_{\epsilon }}{\mathrm{\partial }t}|{|}_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}^2\le C{\epsilon }^4,\\ & \underset{t\in [0,T]}{sup}\parallel {\mathbf{w}}_{\epsilon }{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}^2+{\int }_0^T\parallel \mathrm{\nabla }{\mathbf{w}}_{\epsilon }{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}^2\le C{\epsilon }^4,\\ & \underset{t\in [0,T]}{sup}||\frac{\mathrm{\partial }{\mathbf{w}}_{\epsilon }}{\mathrm{\partial }t}|{|}_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}^2+{\int }_0^T||\mathrm{\nabla }\frac{\mathrm{\partial }{\mathbf{w}}_{\epsilon }}{\mathrm{\partial }t}|{|}_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}^2\le C{\epsilon }^4,\\ & \underset{t\in [0,T]}{sup}\parallel p_{\epsilon }{\parallel }_{L^2(0,T;L^2({\mathrm{\Omega }}_{\epsilon }^{\alpha }\left)\right)}^2\le C{\epsilon }^2.\end{array}$(82) )₃.

Differentiating the integral identity (12)₁ and using ${\displaystyle \frac{\mathrm{\partial }{\mathbf{\text{v}}}_{\epsilon }}{\mathrm{\partial }t}}$ as a test function, we get (93) $\begin{array}{rl}& \frac{1}{2}\frac{\mathrm{d}}{\mathrm{d}t}{\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}|\frac{\mathrm{\partial }{\mathbf{\text{v}}}_{\epsilon }}{\mathrm{\partial }t}{|}^2+\mu {\int }_0^t|\mathrm{\nabla }\frac{\mathrm{\partial }{\mathbf{\text{v}}}_{\epsilon }}{\mathrm{\partial }t}{|}^2=a{\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}\text{rot}\frac{\mathrm{\partial }{\mathbf{\text{w}}}_{\epsilon }}{\mathrm{\partial }t}\frac{\mathrm{\partial }{\mathbf{\text{v}}}_{\epsilon }}{\mathrm{\partial }t}+{\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}\frac{\mathrm{\partial }\mathbf{\text{F}}}{\mathrm{\partial }t}\frac{\mathrm{\partial }{\mathbf{\text{v}}}_{\epsilon }}{\mathrm{\partial }t}\\ & -{\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}\frac{{\mathrm{\partial }}^2{\mathbf{\text{h}}}_{\epsilon }}{\mathrm{\partial }{t}^2}\frac{\mathrm{\partial }{\mathbf{\text{v}}}_{\epsilon }}{\mathrm{\partial }t}-{\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}\mathrm{\nabla }\frac{\mathrm{\partial }{\mathbf{\text{h}}}_{\epsilon }}{\mathrm{\partial }t}\mathrm{\nabla }\frac{\mathrm{\partial }{\mathbf{\text{v}}}_{\epsilon }}{\mathrm{\partial }t}.\end{array}$(93) In much the same way as for (88(88) $\frac{1}{2}\frac{\mathrm{d}}{\mathrm{d}t}{\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}|{\mathbf{\text{v}}}_{\epsilon }{|}^2+\mu {\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}|\mathrm{\nabla }{\mathbf{\text{v}}}_{\epsilon }{|}^2=a{\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}\text{rot}{\mathbf{\text{w}}}_{\epsilon }{\mathbf{\text{v}}}_{\epsilon }+{\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}\mathbf{\text{F}}{\mathbf{\text{v}}}_{\epsilon }-{\int }_{{\mathrm{\Omega }}_{\epsilon }}\frac{\mathrm{\partial }{\mathbf{\text{h}}}_{\epsilon }}{\mathrm{\partial }t}{\mathbf{\text{v}}}_{\epsilon }-{\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}\mathrm{\nabla }{\mathbf{\text{h}}}_{\epsilon }\mathrm{\nabla }{\mathbf{\text{v}}}_{\epsilon }.$(88) ), estimating the terms on right–hand side using Young's inequality in equation (93(93) $\begin{array}{rl}& \frac{1}{2}\frac{\mathrm{d}}{\mathrm{d}t}{\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}|\frac{\mathrm{\partial }{\mathbf{\text{v}}}_{\epsilon }}{\mathrm{\partial }t}{|}^2+\mu {\int }_0^t|\mathrm{\nabla }\frac{\mathrm{\partial }{\mathbf{\text{v}}}_{\epsilon }}{\mathrm{\partial }t}{|}^2=a{\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}\text{rot}\frac{\mathrm{\partial }{\mathbf{\text{w}}}_{\epsilon }}{\mathrm{\partial }t}\frac{\mathrm{\partial }{\mathbf{\text{v}}}_{\epsilon }}{\mathrm{\partial }t}+{\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}\frac{\mathrm{\partial }\mathbf{\text{F}}}{\mathrm{\partial }t}\frac{\mathrm{\partial }{\mathbf{\text{v}}}_{\epsilon }}{\mathrm{\partial }t}\\ & -{\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}\frac{{\mathrm{\partial }}^2{\mathbf{\text{h}}}_{\epsilon }}{\mathrm{\partial }{t}^2}\frac{\mathrm{\partial }{\mathbf{\text{v}}}_{\epsilon }}{\mathrm{\partial }t}-{\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}\mathrm{\nabla }\frac{\mathrm{\partial }{\mathbf{\text{h}}}_{\epsilon }}{\mathrm{\partial }t}\mathrm{\nabla }\frac{\mathrm{\partial }{\mathbf{\text{v}}}_{\epsilon }}{\mathrm{\partial }t}.\end{array}$(93) ), integrating over t and using (81(81) $\begin{array}{rl}& \parallel \mathrm{\nabla }{\mathit{\varphi }}_{\epsilon }{\parallel }_{L^2(0,T;L^2({\mathrm{\Omega }}_{\epsilon }^{\alpha }\left)\right)}\\ & \le C(\frac{1}{\epsilon }\parallel K{\parallel }_{L^2(0,T;L^2({\mathrm{\Omega }}_{\epsilon }^{\alpha }\left)\right)}+\frac{1}{\sqrt{\epsilon }}(\parallel {\mathbf{\text{g}}}_0^t{\parallel }_{L^2(0,T;H^{1/2}({\mathrm{\Sigma }}_{\epsilon }^0\left)\right)}+\parallel {\mathbf{\text{g}}}_l^t{\parallel }_{L^2(0,T;H^{1/2}({\mathrm{\Sigma }}_{\epsilon }^l\left)\right)}))\\ & +C(\parallel {\mathbf{\text{g}}}_0^{nb}{\parallel }_{L^2(0,T;H^{1/2}({\mathrm{\Sigma }}_{\epsilon }^0\left)\right)}+\parallel {\mathbf{\text{g}}}_l^{nb}{\parallel }_{L^2(0,T;H^{1/2}({\mathrm{\Sigma }}_{\epsilon }^l\left)\right)}),\\ & ||\mathrm{\nabla }\frac{\mathrm{\partial }{\mathit{\varphi }}_{\mathit{\epsilon }}}{\mathrm{\partial }t}|{|}_{L^2(0,T;L^2({\mathrm{\Omega }}_{\epsilon }^{\alpha }\left)\right)}\\ & \le C(\frac{1}{\epsilon }||\frac{\mathrm{\partial }K}{\mathrm{\partial }t}|{|}_{L^2(0,T;L^2({\mathrm{\Omega }}_{\epsilon }^{\alpha }\left)\right)}+\frac{1}{\sqrt{\epsilon }}(||\frac{\mathrm{\partial }{\mathbf{\text{g}}}_0^t}{\mathrm{\partial }t}|{|}_{L^2(0,T;H^{1/2}({\mathrm{\Sigma }}_{\epsilon }^0\left)\right)}+||\frac{\mathrm{\partial }{\mathbf{\text{g}}}_l^t}{\mathrm{\partial }t}|{|}_{L^2(0,T;H^{1/2}({\mathrm{\Sigma }}_{\epsilon }^l\left)\right)})\\ & +C(||\frac{\mathrm{\partial }{\mathbf{\text{g}}}_0^{nb}}{\mathrm{\partial }t}|{|}_{L^2(0,T;H^{1/2}({\mathrm{\Sigma }}_{\epsilon }^0\left)\right)}+||\frac{\mathrm{\partial }{\mathbf{\text{g}}}_l^{nb}}{\mathrm{\partial }t}|{|}_{L^2(0,T;H^{1/2}({\mathrm{\Sigma }}_{\epsilon }^l\left)\right)}),\\ & ||\mathrm{\nabla }\frac{{\mathrm{\partial }}^2{\mathit{\varphi }}_{\mathit{\epsilon }}}{\mathrm{\partial }{t}^2}|{|}_{L^2(0,T;L^2({\mathrm{\Omega }}_{\epsilon }^{\alpha }\left)\right)}\\ & \le C(\frac{1}{\epsilon }||\frac{{\mathrm{\partial }}^{2}K}{\mathrm{\partial }{t}^2}|{|}_{L^2(0,T;L^2({\mathrm{\Omega }}_{\epsilon }^{\alpha }\left)\right)}+\frac{1}{\sqrt{\epsilon }}(||\frac{{\mathrm{\partial }}^2{\mathbf{\text{h}}}_0^t}{\mathrm{\partial }{t}^2}|{|}_{L^2(0,T;H^{1/2}({\mathrm{\Sigma }}_{\epsilon }^0\left)\right)}+||\frac{{\mathrm{\partial }}^2{\mathbf{\text{h}}}_l^t}{\mathrm{\partial }{t}^2}|{|}_{L^2(0,T;H^{1/2}({\mathrm{\Sigma }}_{\epsilon }^l\left)\right)})\\ & +C(||\frac{{\mathrm{\partial }}^2{\mathbf{\text{h}}}_0^{nb}}{\mathrm{\partial }{t}^2}|{|}_{L^2(0,T;H^{1/2}({\mathrm{\Sigma }}_{\epsilon }^0\left)\right)}+||\frac{{\mathrm{\partial }}^2{\mathbf{\text{h}}}_l^{nb}}{\mathrm{\partial }{t}^2}|{|}_{L^2(0,T;H^{1/2}({\mathrm{\Sigma }}_{\epsilon }^l\left)\right)}),\end{array}$(81) )₂–(81(81) $\begin{array}{rl}& \parallel \mathrm{\nabla }{\mathit{\varphi }}_{\epsilon }{\parallel }_{L^2(0,T;L^2({\mathrm{\Omega }}_{\epsilon }^{\alpha }\left)\right)}\\ & \le C(\frac{1}{\epsilon }\parallel K{\parallel }_{L^2(0,T;L^2({\mathrm{\Omega }}_{\epsilon }^{\alpha }\left)\right)}+\frac{1}{\sqrt{\epsilon }}(\parallel {\mathbf{\text{g}}}_0^t{\parallel }_{L^2(0,T;H^{1/2}({\mathrm{\Sigma }}_{\epsilon }^0\left)\right)}+\parallel {\mathbf{\text{g}}}_l^t{\parallel }_{L^2(0,T;H^{1/2}({\mathrm{\Sigma }}_{\epsilon }^l\left)\right)}))\\ & +C(\parallel {\mathbf{\text{g}}}_0^{nb}{\parallel }_{L^2(0,T;H^{1/2}({\mathrm{\Sigma }}_{\epsilon }^0\left)\right)}+\parallel {\mathbf{\text{g}}}_l^{nb}{\parallel }_{L^2(0,T;H^{1/2}({\mathrm{\Sigma }}_{\epsilon }^l\left)\right)}),\\ & ||\mathrm{\nabla }\frac{\mathrm{\partial }{\mathit{\varphi }}_{\mathit{\epsilon }}}{\mathrm{\partial }t}|{|}_{L^2(0,T;L^2({\mathrm{\Omega }}_{\epsilon }^{\alpha }\left)\right)}\\ & \le C(\frac{1}{\epsilon }||\frac{\mathrm{\partial }K}{\mathrm{\partial }t}|{|}_{L^2(0,T;L^2({\mathrm{\Omega }}_{\epsilon }^{\alpha }\left)\right)}+\frac{1}{\sqrt{\epsilon }}(||\frac{\mathrm{\partial }{\mathbf{\text{g}}}_0^t}{\mathrm{\partial }t}|{|}_{L^2(0,T;H^{1/2}({\mathrm{\Sigma }}_{\epsilon }^0\left)\right)}+||\frac{\mathrm{\partial }{\mathbf{\text{g}}}_l^t}{\mathrm{\partial }t}|{|}_{L^2(0,T;H^{1/2}({\mathrm{\Sigma }}_{\epsilon }^l\left)\right)})\\ & +C(||\frac{\mathrm{\partial }{\mathbf{\text{g}}}_0^{nb}}{\mathrm{\partial }t}|{|}_{L^2(0,T;H^{1/2}({\mathrm{\Sigma }}_{\epsilon }^0\left)\right)}+||\frac{\mathrm{\partial }{\mathbf{\text{g}}}_l^{nb}}{\mathrm{\partial }t}|{|}_{L^2(0,T;H^{1/2}({\mathrm{\Sigma }}_{\epsilon }^l\left)\right)}),\\ & ||\mathrm{\nabla }\frac{{\mathrm{\partial }}^2{\mathit{\varphi }}_{\mathit{\epsilon }}}{\mathrm{\partial }{t}^2}|{|}_{L^2(0,T;L^2({\mathrm{\Omega }}_{\epsilon }^{\alpha }\left)\right)}\\ & \le C(\frac{1}{\epsilon }||\frac{{\mathrm{\partial }}^{2}K}{\mathrm{\partial }{t}^2}|{|}_{L^2(0,T;L^2({\mathrm{\Omega }}_{\epsilon }^{\alpha }\left)\right)}+\frac{1}{\sqrt{\epsilon }}(||\frac{{\mathrm{\partial }}^2{\mathbf{\text{h}}}_0^t}{\mathrm{\partial }{t}^2}|{|}_{L^2(0,T;H^{1/2}({\mathrm{\Sigma }}_{\epsilon }^0\left)\right)}+||\frac{{\mathrm{\partial }}^2{\mathbf{\text{h}}}_l^t}{\mathrm{\partial }{t}^2}|{|}_{L^2(0,T;H^{1/2}({\mathrm{\Sigma }}_{\epsilon }^l\left)\right)})\\ & +C(||\frac{{\mathrm{\partial }}^2{\mathbf{\text{h}}}_0^{nb}}{\mathrm{\partial }{t}^2}|{|}_{L^2(0,T;H^{1/2}({\mathrm{\Sigma }}_{\epsilon }^0\left)\right)}+||\frac{{\mathrm{\partial }}^2{\mathbf{\text{h}}}_l^{nb}}{\mathrm{\partial }{t}^2}|{|}_{L^2(0,T;H^{1/2}({\mathrm{\Sigma }}_{\epsilon }^l\left)\right)}),\end{array}$(81) )₃, we arrive at: (94) $||\frac{\mathrm{\partial }{\mathbf{\text{v}}}_{\epsilon }}{\mathrm{\partial }t}|{|}_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}^2+{\int }_0^t||\mathrm{\nabla }\frac{\mathrm{\partial }{\mathbf{\text{v}}}_{\epsilon }}{\mathrm{\partial }t}|{|}_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}^2\le C{\epsilon }^2{\int }_0^t||\mathrm{\nabla }\frac{\mathrm{\partial }{\mathbf{\text{w}}}_{\epsilon }}{\mathrm{\partial }t}|{|}_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}^2+C{\epsilon }^4.$(94) Combining the estimates (87(87) $\underset{t\in [0,T]}{sup}||\frac{\mathrm{\partial }{\mathbf{\text{w}}}_{\epsilon }}{\mathrm{\partial }t}|{|}_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}^2+{\int }_0^T||\mathrm{\nabla }\frac{\mathrm{\partial }{\mathbf{\text{w}}}_{\epsilon }}{\mathrm{\partial }t}|{|}_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}^2\le C{\epsilon }^2{\int }_0^T||\mathrm{\nabla }\frac{\mathrm{\partial }{\mathbf{\text{v}}}_{\epsilon }}{\mathrm{\partial }t}|{|}_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}^2+C{\epsilon }^4.$(87) ) and (94(94) $||\frac{\mathrm{\partial }{\mathbf{\text{v}}}_{\epsilon }}{\mathrm{\partial }t}|{|}_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}^2+{\int }_0^t||\mathrm{\nabla }\frac{\mathrm{\partial }{\mathbf{\text{v}}}_{\epsilon }}{\mathrm{\partial }t}|{|}_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}^2\le C{\epsilon }^2{\int }_0^t||\mathrm{\nabla }\frac{\mathrm{\partial }{\mathbf{\text{w}}}_{\epsilon }}{\mathrm{\partial }t}|{|}_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}^2+C{\epsilon }^4.$(94) ), for sufficiently small ε we have: (95) $\underset{t\in [0,T]}{sup}||\frac{\mathrm{\partial }{\mathbf{\text{v}}}_{\epsilon }}{\mathrm{\partial }t}|{|}_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}^2+{\int }_0^T||\mathrm{\nabla }\frac{\mathrm{\partial }{\mathbf{\text{v}}}_{\epsilon }}{\mathrm{\partial }t}|{|}_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}^2\le C{\epsilon }^4,$(95) and (96) $\underset{t\in [0,T]}{sup}||\frac{\mathrm{\partial }{\mathbf{\text{w}}}_{\epsilon }}{\mathrm{\partial }t}|{|}_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}^2+{\int }_0^T||\mathrm{\nabla }\frac{\mathrm{\partial }{\mathbf{\text{w}}}_{\epsilon }}{\mathrm{\partial }t}|{|}_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}^2\le C{\epsilon }^4$(96) leading to (82(82) $\begin{array}{rl}& \underset{t\in [0,T]}{sup}\parallel {\mathbf{v}}_{\epsilon }{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}^2+{\int }_0^T\parallel \mathrm{\nabla }{\mathbf{v}}_{\epsilon }{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}^2\le C{\epsilon }^4,\\ & \underset{t\in [0,T]}{sup}||\frac{\mathrm{\partial }{\mathbf{v}}_{\epsilon }}{\mathrm{\partial }t}|{|}_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}^2+{\int }_0^T||\mathrm{\nabla }\frac{\mathrm{\partial }{\mathbf{v}}_{\epsilon }}{\mathrm{\partial }t}|{|}_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}^2\le C{\epsilon }^4,\\ & \underset{t\in [0,T]}{sup}\parallel {\mathbf{w}}_{\epsilon }{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}^2+{\int }_0^T\parallel \mathrm{\nabla }{\mathbf{w}}_{\epsilon }{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}^2\le C{\epsilon }^4,\\ & \underset{t\in [0,T]}{sup}||\frac{\mathrm{\partial }{\mathbf{w}}_{\epsilon }}{\mathrm{\partial }t}|{|}_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}^2+{\int }_0^T||\mathrm{\nabla }\frac{\mathrm{\partial }{\mathbf{w}}_{\epsilon }}{\mathrm{\partial }t}|{|}_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}^2\le C{\epsilon }^4,\\ & \underset{t\in [0,T]}{sup}\parallel p_{\epsilon }{\parallel }_{L^2(0,T;L^2({\mathrm{\Omega }}_{\epsilon }^{\alpha }\left)\right)}^2\le C{\epsilon }^2.\end{array}$(82) )₂ and (82(82) $\begin{array}{rl}& \underset{t\in [0,T]}{sup}\parallel {\mathbf{v}}_{\epsilon }{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}^2+{\int }_0^T\parallel \mathrm{\nabla }{\mathbf{v}}_{\epsilon }{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}^2\le C{\epsilon }^4,\\ & \underset{t\in [0,T]}{sup}||\frac{\mathrm{\partial }{\mathbf{v}}_{\epsilon }}{\mathrm{\partial }t}|{|}_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}^2+{\int }_0^T||\mathrm{\nabla }\frac{\mathrm{\partial }{\mathbf{v}}_{\epsilon }}{\mathrm{\partial }t}|{|}_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}^2\le C{\epsilon }^4,\\ & \underset{t\in [0,T]}{sup}\parallel {\mathbf{w}}_{\epsilon }{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}^2+{\int }_0^T\parallel \mathrm{\nabla }{\mathbf{w}}_{\epsilon }{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}^2\le C{\epsilon }^4,\\ & \underset{t\in [0,T]}{sup}||\frac{\mathrm{\partial }{\mathbf{w}}_{\epsilon }}{\mathrm{\partial }t}|{|}_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}^2+{\int }_0^T||\mathrm{\nabla }\frac{\mathrm{\partial }{\mathbf{w}}_{\epsilon }}{\mathrm{\partial }t}|{|}_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}^2\le C{\epsilon }^4,\\ & \underset{t\in [0,T]}{sup}\parallel p_{\epsilon }{\parallel }_{L^2(0,T;L^2({\mathrm{\Omega }}_{\epsilon }^{\alpha }\left)\right)}^2\le C{\epsilon }^2.\end{array}$(82) )₄.

We now need to estimate the pressure. Let ${\mathbf{\text{d}}}_{\epsilon }$ be the solution of the problem: (97) $\begin{array}{rl}& \text{div}{\mathbf{\text{d}}}_{\epsilon }=p_{\epsilon },\\ & {\mathbf{\text{d}}}_{\epsilon }=0\text{on}\mathrm{\partial }{\mathrm{\Omega }}_{\epsilon }^{\alpha }.\end{array}$(97) Supposing ${\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}{p}_{\epsilon }=0$, for all t, it follows from Lemma 5.2 that (97(97) $\begin{array}{rl}& \text{div}{\mathbf{\text{d}}}_{\epsilon }=p_{\epsilon },\\ & {\mathbf{\text{d}}}_{\epsilon }=0\text{on}\mathrm{\partial }{\mathrm{\Omega }}_{\epsilon }^{\alpha }.\end{array}$(97) ) has at least one solution satisfying (98) $\parallel \mathrm{\nabla }{\mathbf{\text{d}}}_{\epsilon }{\parallel }_{L^2(0,T;L^2({\mathrm{\Omega }}_{\epsilon }^{\alpha }\left)\right)}\le \frac{C}{\epsilon }\parallel p_{\epsilon }{\parallel }_{L^2(0,T;L^2({\mathrm{\Omega }}_{\epsilon }^{\alpha }\left)\right)}.$(98) Multiplying the momentum equation (5(5) $\begin{array}{rl}& \frac{\mathrm{\partial }{\mathbf{\text{u}}}_{\epsilon }}{\mathrm{\partial }t}-\mu \mathrm{\Delta }{\mathbf{\text{u}}}_{\epsilon }+\mathrm{\nabla }{p}_{\epsilon }=a\text{rot}{\mathbf{\text{w}}}_{\epsilon }+\mathbf{\text{F}},\\ & \text{div}{\mathbf{\text{u}}}_{\epsilon }=0,\text{in}{\mathrm{\Omega }}_{\epsilon }^{\alpha },\\ & \frac{\mathrm{\partial }{\mathbf{\text{w}}}_{\epsilon }}{\mathrm{\partial }t}-\delta \mathrm{\Delta }{\mathbf{\text{w}}}_{\epsilon }-\beta \text{div}{\mathbf{\text{w}}}_{\epsilon }+2a{\mathbf{\text{w}}}_{\epsilon }=a\text{rot}{\mathbf{\text{u}}}_{\epsilon }+\mathbf{\text{G}}.\end{array}$(5) )₁ with ${\mathbf{\text{d}}}_{\epsilon }$ and integrating over ${\mathrm{\Omega }}_{\epsilon }^{\alpha }$ yields (99) $\begin{array}{rl}\parallel p_{\epsilon }{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}^2& \le {\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}\frac{\mathrm{\partial }{\mathbf{\text{v}}}_{\epsilon }}{\mathrm{\partial }t}{\mathbf{\text{d}}}_{\epsilon }+{\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}\frac{\mathrm{\partial }{\mathbf{\text{h}}}_{\epsilon }}{\mathrm{\partial }t}{\mathbf{\text{d}}}_{\epsilon }+\mu {\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}\mathrm{\nabla }{\mathbf{\text{v}}}_{\epsilon }\mathrm{\nabla }{\mathbf{\text{d}}}_{\epsilon }+\mu {\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}\mathrm{\nabla }{\mathbf{\text{h}}}_{\epsilon }\mathrm{\nabla }{\mathbf{\text{d}}}_{\epsilon }\\ & -a{\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}\text{rot}{\mathbf{\text{w}}}_{\epsilon }{\mathbf{\text{d}}}_{\epsilon }-{\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}{\mathbf{\text{f}}}_{\epsilon }{\mathbf{\text{d}}}_{\epsilon }.\end{array}$(99) We estimate the terms on the right–hand side of (99(99) $\begin{array}{rl}\parallel p_{\epsilon }{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}^2& \le {\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}\frac{\mathrm{\partial }{\mathbf{\text{v}}}_{\epsilon }}{\mathrm{\partial }t}{\mathbf{\text{d}}}_{\epsilon }+{\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}\frac{\mathrm{\partial }{\mathbf{\text{h}}}_{\epsilon }}{\mathrm{\partial }t}{\mathbf{\text{d}}}_{\epsilon }+\mu {\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}\mathrm{\nabla }{\mathbf{\text{v}}}_{\epsilon }\mathrm{\nabla }{\mathbf{\text{d}}}_{\epsilon }+\mu {\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}\mathrm{\nabla }{\mathbf{\text{h}}}_{\epsilon }\mathrm{\nabla }{\mathbf{\text{d}}}_{\epsilon }\\ & -a{\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}\text{rot}{\mathbf{\text{w}}}_{\epsilon }{\mathbf{\text{d}}}_{\epsilon }-{\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}{\mathbf{\text{f}}}_{\epsilon }{\mathbf{\text{d}}}_{\epsilon }.\end{array}$(99) ) using Poincaré's inequality (80(80) $\parallel {\mathit{\varphi }}_{\epsilon }(t,\cdot ){\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}\le C\epsilon \parallel \mathrm{\nabla }{\mathit{\varphi }}_{\epsilon }(t,\cdot ){\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)},$(80) ) to obtain (100) $\begin{array}{rl}& |{\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}\frac{\mathrm{\partial }{\mathbf{\text{v}}}_{\epsilon }}{\mathrm{\partial }t}{\mathbf{\text{d}}}_{\epsilon }|\le ||\frac{\mathrm{\partial }{\mathbf{\text{v}}}_{\epsilon }}{\mathrm{\partial }t}|{|}_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}\parallel {\mathbf{\text{d}}}_{\epsilon }{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}\le C{\epsilon }^2||\mathrm{\nabla }\frac{\mathrm{\partial }{\mathbf{\text{v}}}_{\epsilon }}{\mathrm{\partial }t}|{|}_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}\parallel \mathrm{\nabla }{\mathbf{\text{d}}}_{\epsilon }{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)},\\ & |{\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}\frac{\mathrm{\partial }{\mathbf{\text{h}}}_{\epsilon }}{\mathrm{\partial }t}{\mathbf{\text{d}}}_{\epsilon }|\le ||\frac{\mathrm{\partial }{\mathbf{\text{h}}}_{\epsilon }}{\mathrm{\partial }t}|{|}_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}\parallel {\mathbf{\text{d}}}_{\epsilon }{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}\le C{\epsilon }^2||\mathrm{\nabla }\frac{\mathrm{\partial }{\mathbf{\text{h}}}_{\epsilon }}{\mathrm{\partial }t}|{|}_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}\parallel \mathrm{\nabla }{\mathbf{\text{d}}}_{\epsilon }{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)},\\ & |{\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}\mathrm{\nabla }{\mathbf{\text{v}}}_{\epsilon }\mathrm{\nabla }{\mathbf{\text{d}}}_{\epsilon }|\le \parallel \mathrm{\nabla }{\mathbf{\text{v}}}_{\epsilon }{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}\parallel \mathrm{\nabla }{\mathbf{\text{d}}}_{\epsilon }{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)},\\ & |{\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}\mathrm{\nabla }{\mathbf{\text{h}}}_{\epsilon }\mathrm{\nabla }{\mathbf{\text{d}}}_{\epsilon }|\le \parallel \mathrm{\nabla }{\mathbf{\text{h}}}_{\epsilon }{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}\parallel \mathrm{\nabla }{\mathbf{\text{d}}}_{\epsilon }{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)},\\ & |{\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}\text{rot}{\mathbf{\text{w}}}_{\epsilon }{\mathbf{\text{d}}}_{\epsilon }|\le C\epsilon \parallel \mathrm{\nabla }{\mathbf{\text{w}}}_{\epsilon }{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}\parallel \mathrm{\nabla }{\mathbf{\text{d}}}_{\epsilon }{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)},\\ & |{\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}{\mathbf{\text{f}}}_{\epsilon }{\mathbf{\text{d}}}_{\epsilon }|\le C\parallel {\mathbf{\text{f}}}_{\epsilon }{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}\parallel {\mathbf{\text{d}}}_{\epsilon }{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}\le C{\epsilon }^2\parallel \mathrm{\nabla }{\mathbf{\text{d}}}_{\epsilon }{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}.\end{array}$(100)

Using the estimates (100(100) $\begin{array}{rl}& |{\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}\frac{\mathrm{\partial }{\mathbf{\text{v}}}_{\epsilon }}{\mathrm{\partial }t}{\mathbf{\text{d}}}_{\epsilon }|\le ||\frac{\mathrm{\partial }{\mathbf{\text{v}}}_{\epsilon }}{\mathrm{\partial }t}|{|}_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}\parallel {\mathbf{\text{d}}}_{\epsilon }{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}\le C{\epsilon }^2||\mathrm{\nabla }\frac{\mathrm{\partial }{\mathbf{\text{v}}}_{\epsilon }}{\mathrm{\partial }t}|{|}_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}\parallel \mathrm{\nabla }{\mathbf{\text{d}}}_{\epsilon }{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)},\\ & |{\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}\frac{\mathrm{\partial }{\mathbf{\text{h}}}_{\epsilon }}{\mathrm{\partial }t}{\mathbf{\text{d}}}_{\epsilon }|\le ||\frac{\mathrm{\partial }{\mathbf{\text{h}}}_{\epsilon }}{\mathrm{\partial }t}|{|}_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}\parallel {\mathbf{\text{d}}}_{\epsilon }{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}\le C{\epsilon }^2||\mathrm{\nabla }\frac{\mathrm{\partial }{\mathbf{\text{h}}}_{\epsilon }}{\mathrm{\partial }t}|{|}_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}\parallel \mathrm{\nabla }{\mathbf{\text{d}}}_{\epsilon }{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)},\\ & |{\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}\mathrm{\nabla }{\mathbf{\text{v}}}_{\epsilon }\mathrm{\nabla }{\mathbf{\text{d}}}_{\epsilon }|\le \parallel \mathrm{\nabla }{\mathbf{\text{v}}}_{\epsilon }{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}\parallel \mathrm{\nabla }{\mathbf{\text{d}}}_{\epsilon }{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)},\\ & |{\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}\mathrm{\nabla }{\mathbf{\text{h}}}_{\epsilon }\mathrm{\nabla }{\mathbf{\text{d}}}_{\epsilon }|\le \parallel \mathrm{\nabla }{\mathbf{\text{h}}}_{\epsilon }{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}\parallel \mathrm{\nabla }{\mathbf{\text{d}}}_{\epsilon }{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)},\\ & |{\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}\text{rot}{\mathbf{\text{w}}}_{\epsilon }{\mathbf{\text{d}}}_{\epsilon }|\le C\epsilon \parallel \mathrm{\nabla }{\mathbf{\text{w}}}_{\epsilon }{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}\parallel \mathrm{\nabla }{\mathbf{\text{d}}}_{\epsilon }{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)},\\ & |{\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}{\mathbf{\text{f}}}_{\epsilon }{\mathbf{\text{d}}}_{\epsilon }|\le C\parallel {\mathbf{\text{f}}}_{\epsilon }{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}\parallel {\mathbf{\text{d}}}_{\epsilon }{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}\le C{\epsilon }^2\parallel \mathrm{\nabla }{\mathbf{\text{d}}}_{\epsilon }{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}.\end{array}$(100) ) and Young's inequality on the right–hand side of equation (99(99) $\begin{array}{rl}\parallel p_{\epsilon }{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}^2& \le {\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}\frac{\mathrm{\partial }{\mathbf{\text{v}}}_{\epsilon }}{\mathrm{\partial }t}{\mathbf{\text{d}}}_{\epsilon }+{\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}\frac{\mathrm{\partial }{\mathbf{\text{h}}}_{\epsilon }}{\mathrm{\partial }t}{\mathbf{\text{d}}}_{\epsilon }+\mu {\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}\mathrm{\nabla }{\mathbf{\text{v}}}_{\epsilon }\mathrm{\nabla }{\mathbf{\text{d}}}_{\epsilon }+\mu {\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}\mathrm{\nabla }{\mathbf{\text{h}}}_{\epsilon }\mathrm{\nabla }{\mathbf{\text{d}}}_{\epsilon }\\ & -a{\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}\text{rot}{\mathbf{\text{w}}}_{\epsilon }{\mathbf{\text{d}}}_{\epsilon }-{\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}{\mathbf{\text{f}}}_{\epsilon }{\mathbf{\text{d}}}_{\epsilon }.\end{array}$(99) ), integrating over t and using (81(81) $\begin{array}{rl}& \parallel \mathrm{\nabla }{\mathit{\varphi }}_{\epsilon }{\parallel }_{L^2(0,T;L^2({\mathrm{\Omega }}_{\epsilon }^{\alpha }\left)\right)}\\ & \le C(\frac{1}{\epsilon }\parallel K{\parallel }_{L^2(0,T;L^2({\mathrm{\Omega }}_{\epsilon }^{\alpha }\left)\right)}+\frac{1}{\sqrt{\epsilon }}(\parallel {\mathbf{\text{g}}}_0^t{\parallel }_{L^2(0,T;H^{1/2}({\mathrm{\Sigma }}_{\epsilon }^0\left)\right)}+\parallel {\mathbf{\text{g}}}_l^t{\parallel }_{L^2(0,T;H^{1/2}({\mathrm{\Sigma }}_{\epsilon }^l\left)\right)}))\\ & +C(\parallel {\mathbf{\text{g}}}_0^{nb}{\parallel }_{L^2(0,T;H^{1/2}({\mathrm{\Sigma }}_{\epsilon }^0\left)\right)}+\parallel {\mathbf{\text{g}}}_l^{nb}{\parallel }_{L^2(0,T;H^{1/2}({\mathrm{\Sigma }}_{\epsilon }^l\left)\right)}),\\ & ||\mathrm{\nabla }\frac{\mathrm{\partial }{\mathit{\varphi }}_{\mathit{\epsilon }}}{\mathrm{\partial }t}|{|}_{L^2(0,T;L^2({\mathrm{\Omega }}_{\epsilon }^{\alpha }\left)\right)}\\ & \le C(\frac{1}{\epsilon }||\frac{\mathrm{\partial }K}{\mathrm{\partial }t}|{|}_{L^2(0,T;L^2({\mathrm{\Omega }}_{\epsilon }^{\alpha }\left)\right)}+\frac{1}{\sqrt{\epsilon }}(||\frac{\mathrm{\partial }{\mathbf{\text{g}}}_0^t}{\mathrm{\partial }t}|{|}_{L^2(0,T;H^{1/2}({\mathrm{\Sigma }}_{\epsilon }^0\left)\right)}+||\frac{\mathrm{\partial }{\mathbf{\text{g}}}_l^t}{\mathrm{\partial }t}|{|}_{L^2(0,T;H^{1/2}({\mathrm{\Sigma }}_{\epsilon }^l\left)\right)})\\ & +C(||\frac{\mathrm{\partial }{\mathbf{\text{g}}}_0^{nb}}{\mathrm{\partial }t}|{|}_{L^2(0,T;H^{1/2}({\mathrm{\Sigma }}_{\epsilon }^0\left)\right)}+||\frac{\mathrm{\partial }{\mathbf{\text{g}}}_l^{nb}}{\mathrm{\partial }t}|{|}_{L^2(0,T;H^{1/2}({\mathrm{\Sigma }}_{\epsilon }^l\left)\right)}),\\ & ||\mathrm{\nabla }\frac{{\mathrm{\partial }}^2{\mathit{\varphi }}_{\mathit{\epsilon }}}{\mathrm{\partial }{t}^2}|{|}_{L^2(0,T;L^2({\mathrm{\Omega }}_{\epsilon }^{\alpha }\left)\right)}\\ & \le C(\frac{1}{\epsilon }||\frac{{\mathrm{\partial }}^{2}K}{\mathrm{\partial }{t}^2}|{|}_{L^2(0,T;L^2({\mathrm{\Omega }}_{\epsilon }^{\alpha }\left)\right)}+\frac{1}{\sqrt{\epsilon }}(||\frac{{\mathrm{\partial }}^2{\mathbf{\text{h}}}_0^t}{\mathrm{\partial }{t}^2}|{|}_{L^2(0,T;H^{1/2}({\mathrm{\Sigma }}_{\epsilon }^0\left)\right)}+||\frac{{\mathrm{\partial }}^2{\mathbf{\text{h}}}_l^t}{\mathrm{\partial }{t}^2}|{|}_{L^2(0,T;H^{1/2}({\mathrm{\Sigma }}_{\epsilon }^l\left)\right)})\\ & +C(||\frac{{\mathrm{\partial }}^2{\mathbf{\text{h}}}_0^{nb}}{\mathrm{\partial }{t}^2}|{|}_{L^2(0,T;H^{1/2}({\mathrm{\Sigma }}_{\epsilon }^0\left)\right)}+||\frac{{\mathrm{\partial }}^2{\mathbf{\text{h}}}_l^{nb}}{\mathrm{\partial }{t}^2}|{|}_{L^2(0,T;H^{1/2}({\mathrm{\Sigma }}_{\epsilon }^l\left)\right)}),\end{array}$(81) )₁–(81(81) $\begin{array}{rl}& \parallel \mathrm{\nabla }{\mathit{\varphi }}_{\epsilon }{\parallel }_{L^2(0,T;L^2({\mathrm{\Omega }}_{\epsilon }^{\alpha }\left)\right)}\\ & \le C(\frac{1}{\epsilon }\parallel K{\parallel }_{L^2(0,T;L^2({\mathrm{\Omega }}_{\epsilon }^{\alpha }\left)\right)}+\frac{1}{\sqrt{\epsilon }}(\parallel {\mathbf{\text{g}}}_0^t{\parallel }_{L^2(0,T;H^{1/2}({\mathrm{\Sigma }}_{\epsilon }^0\left)\right)}+\parallel {\mathbf{\text{g}}}_l^t{\parallel }_{L^2(0,T;H^{1/2}({\mathrm{\Sigma }}_{\epsilon }^l\left)\right)}))\\ & +C(\parallel {\mathbf{\text{g}}}_0^{nb}{\parallel }_{L^2(0,T;H^{1/2}({\mathrm{\Sigma }}_{\epsilon }^0\left)\right)}+\parallel {\mathbf{\text{g}}}_l^{nb}{\parallel }_{L^2(0,T;H^{1/2}({\mathrm{\Sigma }}_{\epsilon }^l\left)\right)}),\\ & ||\mathrm{\nabla }\frac{\mathrm{\partial }{\mathit{\varphi }}_{\mathit{\epsilon }}}{\mathrm{\partial }t}|{|}_{L^2(0,T;L^2({\mathrm{\Omega }}_{\epsilon }^{\alpha }\left)\right)}\\ & \le C(\frac{1}{\epsilon }||\frac{\mathrm{\partial }K}{\mathrm{\partial }t}|{|}_{L^2(0,T;L^2({\mathrm{\Omega }}_{\epsilon }^{\alpha }\left)\right)}+\frac{1}{\sqrt{\epsilon }}(||\frac{\mathrm{\partial }{\mathbf{\text{g}}}_0^t}{\mathrm{\partial }t}|{|}_{L^2(0,T;H^{1/2}({\mathrm{\Sigma }}_{\epsilon }^0\left)\right)}+||\frac{\mathrm{\partial }{\mathbf{\text{g}}}_l^t}{\mathrm{\partial }t}|{|}_{L^2(0,T;H^{1/2}({\mathrm{\Sigma }}_{\epsilon }^l\left)\right)})\\ & +C(||\frac{\mathrm{\partial }{\mathbf{\text{g}}}_0^{nb}}{\mathrm{\partial }t}|{|}_{L^2(0,T;H^{1/2}({\mathrm{\Sigma }}_{\epsilon }^0\left)\right)}+||\frac{\mathrm{\partial }{\mathbf{\text{g}}}_l^{nb}}{\mathrm{\partial }t}|{|}_{L^2(0,T;H^{1/2}({\mathrm{\Sigma }}_{\epsilon }^l\left)\right)}),\\ & ||\mathrm{\nabla }\frac{{\mathrm{\partial }}^2{\mathit{\varphi }}_{\mathit{\epsilon }}}{\mathrm{\partial }{t}^2}|{|}_{L^2(0,T;L^2({\mathrm{\Omega }}_{\epsilon }^{\alpha }\left)\right)}\\ & \le C(\frac{1}{\epsilon }||\frac{{\mathrm{\partial }}^{2}K}{\mathrm{\partial }{t}^2}|{|}_{L^2(0,T;L^2({\mathrm{\Omega }}_{\epsilon }^{\alpha }\left)\right)}+\frac{1}{\sqrt{\epsilon }}(||\frac{{\mathrm{\partial }}^2{\mathbf{\text{h}}}_0^t}{\mathrm{\partial }{t}^2}|{|}_{L^2(0,T;H^{1/2}({\mathrm{\Sigma }}_{\epsilon }^0\left)\right)}+||\frac{{\mathrm{\partial }}^2{\mathbf{\text{h}}}_l^t}{\mathrm{\partial }{t}^2}|{|}_{L^2(0,T;H^{1/2}({\mathrm{\Sigma }}_{\epsilon }^l\left)\right)})\\ & +C(||\frac{{\mathrm{\partial }}^2{\mathbf{\text{h}}}_0^{nb}}{\mathrm{\partial }{t}^2}|{|}_{L^2(0,T;H^{1/2}({\mathrm{\Sigma }}_{\epsilon }^0\left)\right)}+||\frac{{\mathrm{\partial }}^2{\mathbf{\text{h}}}_l^{nb}}{\mathrm{\partial }{t}^2}|{|}_{L^2(0,T;H^{1/2}({\mathrm{\Sigma }}_{\epsilon }^l\left)\right)}),\end{array}$(81) )₂, (91(91) $\underset{t\in [0,T]}{sup}\parallel {\mathbf{\text{v}}}_{\epsilon }{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}^2+{\int }_0^T\parallel \mathrm{\nabla }{\mathbf{\text{v}}}_{\epsilon }{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}^2\le C{\epsilon }^4.$(91) )–(92(92) $\underset{t\in [0,T]}{sup}\parallel {\mathbf{\text{w}}}_{\epsilon }{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}^2+{\int }_0^T\parallel \mathrm{\nabla }{\mathbf{\text{w}}}_{\epsilon }{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}^2\le C{\epsilon }^4$(92) ), (95(95) $\underset{t\in [0,T]}{sup}||\frac{\mathrm{\partial }{\mathbf{\text{v}}}_{\epsilon }}{\mathrm{\partial }t}|{|}_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}^2+{\int }_0^T||\mathrm{\nabla }\frac{\mathrm{\partial }{\mathbf{\text{v}}}_{\epsilon }}{\mathrm{\partial }t}|{|}_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}^2\le C{\epsilon }^4,$(95) ) and (98(98) $\parallel \mathrm{\nabla }{\mathbf{\text{d}}}_{\epsilon }{\parallel }_{L^2(0,T;L^2({\mathrm{\Omega }}_{\epsilon }^{\alpha }\left)\right)}\le \frac{C}{\epsilon }\parallel p_{\epsilon }{\parallel }_{L^2(0,T;L^2({\mathrm{\Omega }}_{\epsilon }^{\alpha }\left)\right)}.$(98) ), we deduce (101) $\parallel p_{\epsilon }{\parallel }_{L^2(0,T;L^2({\mathrm{\Omega }}_{\epsilon }^{\alpha }\left)\right)}\le C\epsilon$(101) completing the proof.

5.2. Error estimate

In the following, we provide the main result of this section.

Theorem 5.5

Error estimate

Let $T\in ⟨0,\mathrm{\infty }⟩$ and let $({\mathbf{u}}_{\epsilon }^{\left[2\right]},{\mathbf{w}}_{\epsilon }^{\left[2\right]},p_{\epsilon }^{\left[2\right]})$ be the asymptotic solution defined by (63(63) $\begin{array}{rl}{\mathbf{\text{U}}}_{\epsilon }^{\left[2\right]}(t,\mathit{x})& ={\mathbf{\text{U}}}_{\epsilon ,reg}^{\left[2\right]}(t,\mathit{x})+{\mathbf{\text{U}}}_{\epsilon ,bl,0}^{\left[2\right]}(t,\mathit{x})+{\mathbf{\text{U}}}_{\epsilon ,bl,l}^{\left[2\right]}(t,\mathit{x})\\ & ={\epsilon }^2(V_0(t,\frac{{\mathit{x}}_{\ast }}{\epsilon })+{\mathcal{V}}_0(t,\frac{x_1}{\epsilon },\frac{{\mathit{x}}_{\ast }}{\epsilon })+{\mathcal{Y}}_0(t,\frac{x_1-l}{\epsilon },\frac{{\mathit{x}}_{\ast }}{\epsilon }))\\ & +{\epsilon }^3(V_1(t,x_1,\frac{{\mathit{x}}_{\ast }}{\epsilon })+{\mathcal{V}}_1(t,\frac{x_1}{\epsilon },\frac{{\mathit{x}}_{\ast }}{\epsilon })+{\mathcal{Y}}_1(t,\frac{x_1-l}{\epsilon },\frac{{\mathit{x}}_{\ast }}{\epsilon }))\\ & +{\epsilon }^4(V_2(t,x_1,\frac{{\mathit{x}}_{\ast }}{\epsilon })+{\mathcal{V}}_2(t,\frac{x_1}{\epsilon },\frac{{\mathit{x}}_{\ast }}{\epsilon })+{\mathcal{Y}}_2(t,\frac{x_1-l}{\epsilon },\frac{{\mathit{x}}_{\ast }}{\epsilon })),\end{array}$(63) )–(67(67) $\begin{array}{rl}{V}_j& =V_j^1{\mathbf{\text{a}}}^1+V_j^2{\mathbf{\text{a}}}^2+V_j^3{\mathbf{\text{a}}}^3,{\mathcal{V}}_j={\mathcal{V}}_j^1{\mathbf{\text{a}}}^1+{\mathcal{V}}_j^2{\mathbf{\text{a}}}^2+{\mathcal{V}}_j^3{\mathbf{\text{a}}}^3,{\mathcal{Y}}_j={\mathcal{Y}}_j^1{\mathbf{\text{a}}}^1+{\mathcal{Y}}_j^2{\mathbf{\text{a}}}^2+{\mathcal{Y}}_j^3{\mathbf{\text{a}}}^3,j=0,1,2,\\ W_j& =W_j^1{\mathbf{\text{a}}}^1+W_j^2{\mathbf{\text{a}}}^2+W_j^3{\mathbf{\text{a}}}^3,{\mathcal{W}}_j={\mathcal{W}}_j^1{\mathbf{\text{a}}}^1+{\mathcal{W}}_j^2{\mathbf{\text{a}}}^2+{\mathcal{W}}_j^3{\mathbf{\text{a}}}^3,{\mathcal{Z}}_j={\mathcal{Z}}_j^1{\mathbf{\text{a}}}^1+{\mathcal{Z}}_j^2{\mathbf{\text{a}}}^2+{\mathcal{Z}}_j^3{\mathbf{\text{a}}}^3,\\ & j=0,1,2.\end{array}$(67) ) and $({\mathbf{u}}_{\epsilon },{\mathbf{w}}_{\epsilon },p_{\epsilon })$ the solution of the governing problem (5(5) $\begin{array}{rl}& \frac{\mathrm{\partial }{\mathbf{\text{u}}}_{\epsilon }}{\mathrm{\partial }t}-\mu \mathrm{\Delta }{\mathbf{\text{u}}}_{\epsilon }+\mathrm{\nabla }{p}_{\epsilon }=a\text{rot}{\mathbf{\text{w}}}_{\epsilon }+\mathbf{\text{F}},\\ & \text{div}{\mathbf{\text{u}}}_{\epsilon }=0,\text{in}{\mathrm{\Omega }}_{\epsilon }^{\alpha },\\ & \frac{\mathrm{\partial }{\mathbf{\text{w}}}_{\epsilon }}{\mathrm{\partial }t}-\delta \mathrm{\Delta }{\mathbf{\text{w}}}_{\epsilon }-\beta \text{div}{\mathbf{\text{w}}}_{\epsilon }+2a{\mathbf{\text{w}}}_{\epsilon }=a\text{rot}{\mathbf{\text{u}}}_{\epsilon }+\mathbf{\text{G}}.\end{array}$(5) )–(11(11) ${\mathbf{\text{h}}}_0^{\epsilon }(t,\cdot ),{\mathbf{\text{h}}}_l^{\epsilon }(t,\cdot ),\mathbf{\text{F}}(t,\cdot ),\mathbf{\text{G}}(t,\cdot ),\mathrm{\forall }t\in [0,T^{\ast }],T^{\ast }>0.$(11) ). Then the following estimates hold: (102) $\begin{array}{rl}& \underset{t\in [0,T]}{sup}\parallel {\mathbf{u}}_{\epsilon }(t,\cdot )-{\mathbf{u}}_{\epsilon }^{\left[2\right]}(t,\cdot ){\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}+\parallel \mathrm{\nabla }({\mathbf{u}}_{\epsilon }-{\mathbf{u}}_{\epsilon }^{\left[2\right]})|{|}_{L^2(0,T;L^2({\mathrm{\Omega }}_{\epsilon }^{\alpha }\left)\right)}\le C{\epsilon }^{7/2},\\ & \underset{t\in [0,T]}{sup}||\frac{\mathrm{\partial }{\mathbf{u}}_{\epsilon }(t,\cdot )}{\mathrm{\partial }t}-\frac{\mathrm{\partial }{\mathbf{u}}_{\epsilon }^{\left[2\right]}(t,\cdot )}{\mathrm{\partial }t}|{|}_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}+||\mathrm{\nabla }(\frac{\mathrm{\partial }{\mathbf{u}}_{\epsilon }}{\mathrm{\partial }t}-\frac{\mathrm{\partial }{\mathbf{u}}_{\epsilon }^{\left[2\right]}}{\mathrm{\partial }t})|{|}_{L^2(0,T;L^2({\mathrm{\Omega }}_{\epsilon }^{\alpha }\left)\right)}\le C{\epsilon }^{7/2},\\ & \underset{t\in [0,T]}{sup}\parallel {\mathbf{w}}_{\epsilon }(t,\cdot )-{\mathbf{w}}_{\epsilon }^{\left[2\right]}(t,\cdot ){\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}+\parallel \mathrm{\nabla }({\mathbf{w}}_{\epsilon }-{\mathbf{w}}_{\epsilon }^{\left[2\right]})|{|}_{L^2(0,T;L^2({\mathrm{\Omega }}_{\epsilon }^{\alpha }\left)\right)}\le C{\epsilon }^{9/2},\\ & \underset{t\in [0,T]}{sup}||\frac{\mathrm{\partial }{\mathbf{w}}_{\epsilon }(t,\cdot )}{\mathrm{\partial }t}-\frac{\mathrm{\partial }{\mathbf{w}}_{\epsilon }^{\left[2\right]}(t,\cdot )}{\mathrm{\partial }t}|{|}_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}+||\mathrm{\nabla }(\frac{\mathrm{\partial }{\mathbf{w}}_{\epsilon }}{\mathrm{\partial }t}-\frac{\mathrm{\partial }{\mathbf{w}}_{\epsilon }^{\left[2\right]}}{\mathrm{\partial }t})|{|}_{L^2(0,T;L^2({\mathrm{\Omega }}_{\epsilon }^{\alpha }\left)\right)}\le C{\epsilon }^{9/2},\\ & \parallel p_{\epsilon }-p_{\epsilon }^{\left[2\right]}{\parallel }_{L^2(0,T;L^2({\mathrm{\Omega }}_{\epsilon }^{\alpha }\left)\right)}\le C{\epsilon }^{5/2},\end{array}$(102) where the constant C>0 is independent of ε.

Proof.

The asymptotic approximation ${\mathbf{\text{w}}}_{\epsilon }^{\left[2\right]}$ for the microrotation satisfies the following equation: (103) $\begin{array}{rl}& \frac{\mathrm{\partial }{\mathbf{\text{w}}}_{\epsilon }^{\left[2\right]}}{\mathrm{\partial }t}-\delta \mathrm{\Delta }{\mathbf{\text{w}}}_{\epsilon }^{\left[2\right]}-\beta \mathrm{\nabla }\text{div}{\mathbf{\text{w}}}_{\epsilon }^{\left[2\right]}+2a{\mathbf{\text{w}}}_{\epsilon }^{\left[2\right]}=a\text{rot}{\mathbf{\text{u}}}_{\epsilon }^{\left[1\right]}+\mathbf{\text{G}}+{\mathit{\xi }}_{\epsilon }\text{in}{\mathrm{\Omega }}_{\epsilon }^{\alpha },\\ & {\mathbf{\text{w}}}_{\epsilon }^{\left[2\right]}=\mathbf{\text{0}}\text{on}{\mathrm{\Gamma }}_{\epsilon }^{\alpha },\\ & {\mathbf{\text{w}}}_{\epsilon }^{\left[2\right]}={\mathit{\eta }}_0^{\epsilon }\text{on}{\mathrm{\Sigma }}_{\epsilon }^0,{\mathbf{\text{w}}}_{\epsilon }^{\left[2\right]}={\mathit{\eta }}_l^{\epsilon }\text{on}{\mathrm{\Sigma }}_{\epsilon }^l,\\ & {\mathbf{\text{w}}}_{\epsilon }^{\left[2\right]}(0,\cdot )=\mathbf{\text{0}}.\end{array}$(103) where $\parallel {\mathit{\xi }}_{\epsilon }{\parallel }_{L^2(0,T;L^2({\mathrm{\Omega }}_{\epsilon }^{\alpha }\left)\right)}=\mathcal{O}\left({\epsilon }^4\right)$ and $\parallel {\mathit{\eta }}_i^{\epsilon }{\parallel }_{L^2(0,T;H^{1/2}({\mathrm{\Sigma }}_{\epsilon }^i\left)\right)},=\mathcal{O}\left({\epsilon }^{11/2}\right)$, i=0,l.

Denoting ${\mathbf{\text{s}}}_{\epsilon }={\mathbf{\text{w}}}_{\epsilon }-{\mathbf{\text{w}}}_{\epsilon }^{\left[2\right]}$ and subtracting $(5{)}_3,(6{)}_2,(6{)}_{3,2}$ and (103(103) $\begin{array}{rl}& \frac{\mathrm{\partial }{\mathbf{\text{w}}}_{\epsilon }^{\left[2\right]}}{\mathrm{\partial }t}-\delta \mathrm{\Delta }{\mathbf{\text{w}}}_{\epsilon }^{\left[2\right]}-\beta \mathrm{\nabla }\text{div}{\mathbf{\text{w}}}_{\epsilon }^{\left[2\right]}+2a{\mathbf{\text{w}}}_{\epsilon }^{\left[2\right]}=a\text{rot}{\mathbf{\text{u}}}_{\epsilon }^{\left[1\right]}+\mathbf{\text{G}}+{\mathit{\xi }}_{\epsilon }\text{in}{\mathrm{\Omega }}_{\epsilon }^{\alpha },\\ & {\mathbf{\text{w}}}_{\epsilon }^{\left[2\right]}=\mathbf{\text{0}}\text{on}{\mathrm{\Gamma }}_{\epsilon }^{\alpha },\\ & {\mathbf{\text{w}}}_{\epsilon }^{\left[2\right]}={\mathit{\eta }}_0^{\epsilon }\text{on}{\mathrm{\Sigma }}_{\epsilon }^0,{\mathbf{\text{w}}}_{\epsilon }^{\left[2\right]}={\mathit{\eta }}_l^{\epsilon }\text{on}{\mathrm{\Sigma }}_{\epsilon }^l,\\ & {\mathbf{\text{w}}}_{\epsilon }^{\left[2\right]}(0,\cdot )=\mathbf{\text{0}}.\end{array}$(103) ), we obtain (104) $\begin{array}{rl}& \frac{\mathrm{\partial }{\mathbf{\text{s}}}_{\epsilon }}{\mathrm{\partial }t}-\alpha \mathrm{\Delta }{\mathbf{\text{s}}}_{\epsilon }-\beta \mathrm{\nabla }\text{div}{\mathbf{\text{s}}}_{\epsilon }+2a{\mathbf{\text{s}}}_{\epsilon }=a\text{rot}({\mathbf{\text{u}}}_{\epsilon }-{\mathbf{\text{u}}}_{\epsilon }^{\left[1\right]})-{\mathit{\xi }}_{\epsilon },\\ & {\mathbf{\text{s}}}_{\epsilon }=0\text{on}{\mathrm{\Gamma }}_{\epsilon }^{\alpha },\\ & {\mathbf{\text{s}}}_{\epsilon }=-{\mathit{\eta }}_0\text{on}{\mathrm{\Sigma }}_{\epsilon }^0,{\mathbf{\text{s}}}_{\epsilon }=-{\mathit{\eta }}_l\text{on}{\mathrm{\Sigma }}_{\epsilon }^l.\end{array}$(104) It follows from Lemma 5.2 that we can construct a function ${\mathbf{\text{D}}}_{\epsilon }$ with the same trace as ${\mathbf{\text{s}}}_{\epsilon }$ on $\mathrm{\partial }{\mathrm{\Omega }}_{\epsilon }^{\alpha }$ such that (105) $\parallel \mathrm{\nabla }{\mathbf{\text{D}}}_{\epsilon }{\parallel }_{L^2(0,T;L^2({\mathrm{\Omega }}_{\epsilon }^{\alpha }\left)\right)}+||\mathrm{\nabla }\frac{\mathrm{\partial }{\mathbf{\text{D}}}_{\epsilon }}{\mathrm{\partial }t}|{|}_{L^2(0,T;L^2({\mathrm{\Omega }}_{\epsilon }^{\alpha }\left)\right)}+||\mathrm{\nabla }\frac{{\mathrm{\partial }}^2{\mathbf{\text{D}}}_{\epsilon }}{\mathrm{\partial }{t}^2}|{|}_{L^2(0,T;L^2({\mathrm{\Omega }}_{\epsilon }^{\alpha }\left)\right)}\le C{\epsilon }^5.$(105) Multiplying the equation (104(104) $\begin{array}{rl}& \frac{\mathrm{\partial }{\mathbf{\text{s}}}_{\epsilon }}{\mathrm{\partial }t}-\alpha \mathrm{\Delta }{\mathbf{\text{s}}}_{\epsilon }-\beta \mathrm{\nabla }\text{div}{\mathbf{\text{s}}}_{\epsilon }+2a{\mathbf{\text{s}}}_{\epsilon }=a\text{rot}({\mathbf{\text{u}}}_{\epsilon }-{\mathbf{\text{u}}}_{\epsilon }^{\left[1\right]})-{\mathit{\xi }}_{\epsilon },\\ & {\mathbf{\text{s}}}_{\epsilon }=0\text{on}{\mathrm{\Gamma }}_{\epsilon }^{\alpha },\\ & {\mathbf{\text{s}}}_{\epsilon }=-{\mathit{\eta }}_0\text{on}{\mathrm{\Sigma }}_{\epsilon }^0,{\mathbf{\text{s}}}_{\epsilon }=-{\mathit{\eta }}_l\text{on}{\mathrm{\Sigma }}_{\epsilon }^l.\end{array}$(104) ) by ${\mathbf{\text{s}}}^{\ast }={\mathbf{\text{s}}}_{\epsilon }-{\mathbf{\text{D}}}_{\epsilon }$ and integrating over ${\mathrm{\Omega }}_{\epsilon }^{\alpha }$ yield (106) $\begin{array}{rl}& \frac{1}{2}\frac{\mathrm{d}}{\mathrm{d}t}{\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}|{\mathbf{\text{s}}}_{\epsilon }^{\ast }{|}^2+\alpha {\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}|\mathrm{\nabla }{\mathbf{\text{s}}}_{\epsilon }^{\ast }{|}^2+\beta {\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}(\text{div}{\mathbf{\text{s}}}_{\epsilon }^{\ast }{)}^2+2a{\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}|{\mathbf{\text{s}}}_{\epsilon }^{\ast }{|}^2\\ & =a{\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}\text{rot}({\mathbf{\text{u}}}_{\epsilon }-{\mathbf{\text{u}}}_{\epsilon }^{\left[1\right]}){\mathbf{\text{s}}}_{\epsilon }^{\ast }-{\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}\frac{\mathrm{\partial }{\mathbf{\text{D}}}_{\epsilon }}{\mathrm{\partial }t}{\mathbf{\text{s}}}_{\epsilon }^{\ast }-\delta {\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}\mathrm{\nabla }{\mathbf{\text{s}}}_{\epsilon }^{\ast }\mathrm{\nabla }{\mathbf{\text{D}}}_{\epsilon }\\ & -\beta {\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}\text{div}{\mathbf{\text{D}}}_{\epsilon }\text{div}{\mathbf{\text{s}}}_{\epsilon }^{\ast }-2a{\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}{\mathbf{\text{s}}}_{\epsilon }^{\ast }{\mathbf{\text{D}}}_{\epsilon }-{\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}{\mathit{\xi }}_{\epsilon }{\mathbf{\text{s}}}_{\epsilon }^{\ast }\end{array}$(106) We now estimate the terms on the right–hand side of (106(106) $\begin{array}{rl}& \frac{1}{2}\frac{\mathrm{d}}{\mathrm{d}t}{\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}|{\mathbf{\text{s}}}_{\epsilon }^{\ast }{|}^2+\alpha {\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}|\mathrm{\nabla }{\mathbf{\text{s}}}_{\epsilon }^{\ast }{|}^2+\beta {\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}(\text{div}{\mathbf{\text{s}}}_{\epsilon }^{\ast }{)}^2+2a{\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}|{\mathbf{\text{s}}}_{\epsilon }^{\ast }{|}^2\\ & =a{\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}\text{rot}({\mathbf{\text{u}}}_{\epsilon }-{\mathbf{\text{u}}}_{\epsilon }^{\left[1\right]}){\mathbf{\text{s}}}_{\epsilon }^{\ast }-{\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}\frac{\mathrm{\partial }{\mathbf{\text{D}}}_{\epsilon }}{\mathrm{\partial }t}{\mathbf{\text{s}}}_{\epsilon }^{\ast }-\delta {\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}\mathrm{\nabla }{\mathbf{\text{s}}}_{\epsilon }^{\ast }\mathrm{\nabla }{\mathbf{\text{D}}}_{\epsilon }\\ & -\beta {\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}\text{div}{\mathbf{\text{D}}}_{\epsilon }\text{div}{\mathbf{\text{s}}}_{\epsilon }^{\ast }-2a{\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}{\mathbf{\text{s}}}_{\epsilon }^{\ast }{\mathbf{\text{D}}}_{\epsilon }-{\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}{\mathit{\xi }}_{\epsilon }{\mathbf{\text{s}}}_{\epsilon }^{\ast }\end{array}$(106) ) using Poincare's inequality (80(80) $\parallel {\mathit{\varphi }}_{\epsilon }(t,\cdot ){\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}\le C\epsilon \parallel \mathrm{\nabla }{\mathit{\varphi }}_{\epsilon }(t,\cdot ){\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)},$(80) ) in the following way: (107) $\begin{array}{rl}& |{\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}\text{rot}({\mathbf{\text{u}}}_{\epsilon }-{\mathbf{\text{u}}}_{\epsilon }){\mathbf{\text{s}}}_{\epsilon }^{\ast }|\le C\parallel \mathrm{\nabla }({\mathbf{\text{u}}}_{\epsilon }-{\mathbf{\text{u}}}_{\epsilon }^{\left[1\right]}){\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}\parallel {\mathbf{\text{s}}}_{\epsilon }^{\ast }{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}\\ & \le C(\parallel \mathrm{\nabla }({\mathbf{\text{u}}}_{\epsilon }-{\mathbf{\text{u}}}_{\epsilon }^{\left[2\right]}){\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}+\parallel \mathrm{\nabla }({\mathbf{\text{u}}}_{\epsilon }^{\left[2\right]}-{\mathbf{\text{u}}}_{\epsilon }^{\left[1\right]}){\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)})\parallel {\mathbf{\text{s}}}_{\epsilon }^{\ast }{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}\\ & \le C\epsilon \parallel \mathrm{\nabla }({\mathbf{\text{u}}}_{\epsilon }-{\mathbf{\text{u}}}_{\epsilon }^{\left[2\right]}){\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}\parallel \mathrm{\nabla }{\mathbf{\text{s}}}_{\epsilon }^{\ast }{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }\right)}+C{\epsilon }^5\parallel \mathrm{\nabla }{\mathbf{\text{s}}}_{\epsilon }^{\ast }{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)},\\ & |{\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}\frac{\mathrm{\partial }{\mathbf{\text{D}}}_{\epsilon }}{\mathrm{\partial }t}{\mathbf{\text{s}}}_{\epsilon }^{\ast }|\le ||\frac{\mathrm{\partial }{\mathbf{\text{D}}}_{\epsilon }}{\mathrm{\partial }t}|{|}_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}\parallel {\mathbf{\text{s}}}_{\epsilon }^{\ast }{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}\le {\epsilon }^2||\mathrm{\nabla }\frac{\mathrm{\partial }{\mathbf{\text{D}}}_{\epsilon }}{\mathrm{\partial }t}|{|}_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}\parallel \mathrm{\nabla }{\mathbf{\text{s}}}_{\epsilon }^{\ast }{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)},\\ & |{\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}\mathrm{\nabla }{\mathbf{\text{s}}}_{\epsilon }^{\ast }\mathrm{\nabla }{\mathbf{\text{D}}}_{\epsilon }|\le \parallel \mathrm{\nabla }{\mathbf{\text{s}}}_{\epsilon }^{\ast }{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}\parallel \mathrm{\nabla }{\mathbf{\text{D}}}_{\epsilon }{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)},\\ & |{\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}\text{div}{\mathbf{\text{D}}}_{\epsilon }\text{div}{\mathbf{\text{s}}}_{\epsilon }^{\ast }|\le \parallel \mathrm{\nabla }{\mathbf{\text{D}}}_{\epsilon }{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}\parallel \mathrm{\nabla }{\mathbf{\text{s}}}_{\epsilon }^{\ast }{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)},\\ & |{\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}{\mathbf{\text{s}}}_{\epsilon }^{\ast }{\mathbf{\text{D}}}_{\epsilon }|\le \parallel {\mathbf{\text{s}}}_{\epsilon }^{\ast }{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}\parallel {\mathbf{\text{D}}}_{\epsilon }{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}\le C{\epsilon }^2\parallel \mathrm{\nabla }{\mathbf{\text{s}}}_{\epsilon }^{\ast }{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}\parallel \mathrm{\nabla }{\mathbf{\text{D}}}_{\epsilon }^{\ast }{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)},\\ & |{\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}{\mathit{\xi }}_{\epsilon }{\mathbf{\text{s}}}_{\epsilon }^{\ast }|\le \parallel {\mathit{\xi }}_{\epsilon }{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}\parallel {\mathbf{\text{s}}}_{\epsilon }{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}\le C\epsilon \parallel {\mathit{\xi }}_{\epsilon }{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}\parallel \mathrm{\nabla }{\mathbf{\text{s}}}_{\epsilon }^{\ast }{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }\right)}.\end{array}$(107) Applying the estimates (107(107) $\begin{array}{rl}& |{\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}\text{rot}({\mathbf{\text{u}}}_{\epsilon }-{\mathbf{\text{u}}}_{\epsilon }){\mathbf{\text{s}}}_{\epsilon }^{\ast }|\le C\parallel \mathrm{\nabla }({\mathbf{\text{u}}}_{\epsilon }-{\mathbf{\text{u}}}_{\epsilon }^{\left[1\right]}){\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}\parallel {\mathbf{\text{s}}}_{\epsilon }^{\ast }{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}\\ & \le C(\parallel \mathrm{\nabla }({\mathbf{\text{u}}}_{\epsilon }-{\mathbf{\text{u}}}_{\epsilon }^{\left[2\right]}){\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}+\parallel \mathrm{\nabla }({\mathbf{\text{u}}}_{\epsilon }^{\left[2\right]}-{\mathbf{\text{u}}}_{\epsilon }^{\left[1\right]}){\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)})\parallel {\mathbf{\text{s}}}_{\epsilon }^{\ast }{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}\\ & \le C\epsilon \parallel \mathrm{\nabla }({\mathbf{\text{u}}}_{\epsilon }-{\mathbf{\text{u}}}_{\epsilon }^{\left[2\right]}){\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}\parallel \mathrm{\nabla }{\mathbf{\text{s}}}_{\epsilon }^{\ast }{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }\right)}+C{\epsilon }^5\parallel \mathrm{\nabla }{\mathbf{\text{s}}}_{\epsilon }^{\ast }{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)},\\ & |{\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}\frac{\mathrm{\partial }{\mathbf{\text{D}}}_{\epsilon }}{\mathrm{\partial }t}{\mathbf{\text{s}}}_{\epsilon }^{\ast }|\le ||\frac{\mathrm{\partial }{\mathbf{\text{D}}}_{\epsilon }}{\mathrm{\partial }t}|{|}_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}\parallel {\mathbf{\text{s}}}_{\epsilon }^{\ast }{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}\le {\epsilon }^2||\mathrm{\nabla }\frac{\mathrm{\partial }{\mathbf{\text{D}}}_{\epsilon }}{\mathrm{\partial }t}|{|}_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}\parallel \mathrm{\nabla }{\mathbf{\text{s}}}_{\epsilon }^{\ast }{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)},\\ & |{\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}\mathrm{\nabla }{\mathbf{\text{s}}}_{\epsilon }^{\ast }\mathrm{\nabla }{\mathbf{\text{D}}}_{\epsilon }|\le \parallel \mathrm{\nabla }{\mathbf{\text{s}}}_{\epsilon }^{\ast }{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}\parallel \mathrm{\nabla }{\mathbf{\text{D}}}_{\epsilon }{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)},\\ & |{\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}\text{div}{\mathbf{\text{D}}}_{\epsilon }\text{div}{\mathbf{\text{s}}}_{\epsilon }^{\ast }|\le \parallel \mathrm{\nabla }{\mathbf{\text{D}}}_{\epsilon }{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}\parallel \mathrm{\nabla }{\mathbf{\text{s}}}_{\epsilon }^{\ast }{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)},\\ & |{\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}{\mathbf{\text{s}}}_{\epsilon }^{\ast }{\mathbf{\text{D}}}_{\epsilon }|\le \parallel {\mathbf{\text{s}}}_{\epsilon }^{\ast }{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}\parallel {\mathbf{\text{D}}}_{\epsilon }{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}\le C{\epsilon }^2\parallel \mathrm{\nabla }{\mathbf{\text{s}}}_{\epsilon }^{\ast }{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}\parallel \mathrm{\nabla }{\mathbf{\text{D}}}_{\epsilon }^{\ast }{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)},\\ & |{\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}{\mathit{\xi }}_{\epsilon }{\mathbf{\text{s}}}_{\epsilon }^{\ast }|\le \parallel {\mathit{\xi }}_{\epsilon }{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}\parallel {\mathbf{\text{s}}}_{\epsilon }{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}\le C\epsilon \parallel {\mathit{\xi }}_{\epsilon }{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}\parallel \mathrm{\nabla }{\mathbf{\text{s}}}_{\epsilon }^{\ast }{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }\right)}.\end{array}$(107) ) and Young's inequality on the right hand–side of equation (106(106) $\begin{array}{rl}& \frac{1}{2}\frac{\mathrm{d}}{\mathrm{d}t}{\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}|{\mathbf{\text{s}}}_{\epsilon }^{\ast }{|}^2+\alpha {\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}|\mathrm{\nabla }{\mathbf{\text{s}}}_{\epsilon }^{\ast }{|}^2+\beta {\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}(\text{div}{\mathbf{\text{s}}}_{\epsilon }^{\ast }{)}^2+2a{\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}|{\mathbf{\text{s}}}_{\epsilon }^{\ast }{|}^2\\ & =a{\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}\text{rot}({\mathbf{\text{u}}}_{\epsilon }-{\mathbf{\text{u}}}_{\epsilon }^{\left[1\right]}){\mathbf{\text{s}}}_{\epsilon }^{\ast }-{\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}\frac{\mathrm{\partial }{\mathbf{\text{D}}}_{\epsilon }}{\mathrm{\partial }t}{\mathbf{\text{s}}}_{\epsilon }^{\ast }-\delta {\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}\mathrm{\nabla }{\mathbf{\text{s}}}_{\epsilon }^{\ast }\mathrm{\nabla }{\mathbf{\text{D}}}_{\epsilon }\\ & -\beta {\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}\text{div}{\mathbf{\text{D}}}_{\epsilon }\text{div}{\mathbf{\text{s}}}_{\epsilon }^{\ast }-2a{\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}{\mathbf{\text{s}}}_{\epsilon }^{\ast }{\mathbf{\text{D}}}_{\epsilon }-{\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}{\mathit{\xi }}_{\epsilon }{\mathbf{\text{s}}}_{\epsilon }^{\ast }\end{array}$(106) ), integrating over t and using (105(105) $\parallel \mathrm{\nabla }{\mathbf{\text{D}}}_{\epsilon }{\parallel }_{L^2(0,T;L^2({\mathrm{\Omega }}_{\epsilon }^{\alpha }\left)\right)}+||\mathrm{\nabla }\frac{\mathrm{\partial }{\mathbf{\text{D}}}_{\epsilon }}{\mathrm{\partial }t}|{|}_{L^2(0,T;L^2({\mathrm{\Omega }}_{\epsilon }^{\alpha }\left)\right)}+||\mathrm{\nabla }\frac{{\mathrm{\partial }}^2{\mathbf{\text{D}}}_{\epsilon }}{\mathrm{\partial }{t}^2}|{|}_{L^2(0,T;L^2({\mathrm{\Omega }}_{\epsilon }^{\alpha }\left)\right)}\le C{\epsilon }^5.$(105) ), we get (108) $\underset{t\in [0,T]}{sup}\parallel {\mathbf{\text{s}}}_{\epsilon }^{\ast }{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}^2+{\int }_0^T\parallel \mathrm{\nabla }{\mathbf{\text{s}}}_{\epsilon }^{\ast }{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}^2\le C{\epsilon }^2{\int }_0^t\parallel \mathrm{\nabla }({\mathbf{\text{u}}}_{\epsilon }-{\mathbf{\text{u}}}_{\epsilon }^{\left[2\right]}){\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}^2+C{\epsilon }^{10}.$(108) Differentiating identity (104(104) $\begin{array}{rl}& \frac{\mathrm{\partial }{\mathbf{\text{s}}}_{\epsilon }}{\mathrm{\partial }t}-\alpha \mathrm{\Delta }{\mathbf{\text{s}}}_{\epsilon }-\beta \mathrm{\nabla }\text{div}{\mathbf{\text{s}}}_{\epsilon }+2a{\mathbf{\text{s}}}_{\epsilon }=a\text{rot}({\mathbf{\text{u}}}_{\epsilon }-{\mathbf{\text{u}}}_{\epsilon }^{\left[1\right]})-{\mathit{\xi }}_{\epsilon },\\ & {\mathbf{\text{s}}}_{\epsilon }=0\text{on}{\mathrm{\Gamma }}_{\epsilon }^{\alpha },\\ & {\mathbf{\text{s}}}_{\epsilon }=-{\mathit{\eta }}_0\text{on}{\mathrm{\Sigma }}_{\epsilon }^0,{\mathbf{\text{s}}}_{\epsilon }=-{\mathit{\eta }}_l\text{on}{\mathrm{\Sigma }}_{\epsilon }^l.\end{array}$(104) )₁ with respect to t and using ${\displaystyle \frac{\mathrm{\partial }{\mathbf{\text{s}}}_{\epsilon }^{\ast }}{\mathrm{\partial }t}}={\displaystyle \frac{\mathrm{\partial }{\mathbf{\text{s}}}_{\epsilon }}{\mathrm{\partial }t}}-{\displaystyle \frac{\mathrm{\partial }{\mathbf{\text{D}}}_{\epsilon }}{\mathrm{\partial }t}}$ as a test function leads to: (109) $\begin{array}{rl}& \frac{1}{2}\frac{\mathrm{d}}{\mathrm{d}t}{\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}|\frac{\mathrm{\partial }{\mathbf{\text{s}}}_{\epsilon }^{\ast }}{\mathrm{\partial }t}{|}^2+\delta {\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}|\mathrm{\nabla }\frac{\mathrm{\partial }{\mathbf{\text{s}}}_{\epsilon }^{\ast }}{\mathrm{\partial }t}{|}^2+\beta {\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}(\text{div}\frac{\mathrm{\partial }{\mathbf{\text{s}}}_{\epsilon }^{\ast }}{\mathrm{\partial }t}{)}^2+2a{\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}|\frac{\mathrm{\partial }{\mathbf{\text{s}}}_{\epsilon }^{\ast }}{\mathrm{\partial }t}{|}^2\\ & =a{\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}\text{rot}(\frac{\mathrm{\partial }{\mathbf{\text{u}}}_{\epsilon }}{\mathrm{\partial }t}-\frac{\mathrm{\partial }{\mathbf{\text{u}}}_{\epsilon }^{\left[1\right]}}{\mathrm{\partial }t})\frac{\mathrm{\partial }{\mathbf{\text{s}}}_{\epsilon }^{\ast }}{\mathrm{\partial }t}-{\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}\frac{{\mathrm{\partial }}^2{\mathbf{\text{D}}}_{\epsilon }}{\mathrm{\partial }{t}^2}\frac{\mathrm{\partial }{\mathbf{\text{s}}}_{\epsilon }^{\ast }}{\mathrm{\partial }t}-\alpha {\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}\mathrm{\nabla }\frac{\mathrm{\partial }{\mathbf{\text{s}}}_{\epsilon }^{\ast }}{\mathrm{\partial }t}\mathrm{\nabla }\frac{\mathrm{\partial }{\mathbf{\text{D}}}_{\epsilon }}{\mathrm{\partial }t}\\ & -\beta {\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}\text{div}\frac{\mathrm{\partial }{\mathbf{\text{D}}}_{\epsilon }}{\mathrm{\partial }t}\text{div}\frac{\mathrm{\partial }{\mathbf{\text{s}}}_{\epsilon }^{\ast }}{\mathrm{\partial }t}-2a{\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}\frac{\mathrm{\partial }{\mathbf{\text{s}}}_{\epsilon }^{\ast }}{\mathrm{\partial }t}\frac{\mathrm{\partial }{\mathbf{\text{D}}}_{\epsilon }}{\mathrm{\partial }t}-{\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}\frac{\mathrm{\partial }{\mathit{\xi }}_{\epsilon }}{\mathrm{\partial }t}\frac{\mathrm{\partial }{\mathbf{\text{s}}}_{\epsilon }^{\ast }}{\mathrm{\partial }t}.\end{array}$(109) Similarly as above, estimating the terms on the right–hand side of (109(109) $\begin{array}{rl}& \frac{1}{2}\frac{\mathrm{d}}{\mathrm{d}t}{\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}|\frac{\mathrm{\partial }{\mathbf{\text{s}}}_{\epsilon }^{\ast }}{\mathrm{\partial }t}{|}^2+\delta {\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}|\mathrm{\nabla }\frac{\mathrm{\partial }{\mathbf{\text{s}}}_{\epsilon }^{\ast }}{\mathrm{\partial }t}{|}^2+\beta {\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}(\text{div}\frac{\mathrm{\partial }{\mathbf{\text{s}}}_{\epsilon }^{\ast }}{\mathrm{\partial }t}{)}^2+2a{\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}|\frac{\mathrm{\partial }{\mathbf{\text{s}}}_{\epsilon }^{\ast }}{\mathrm{\partial }t}{|}^2\\ & =a{\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}\text{rot}(\frac{\mathrm{\partial }{\mathbf{\text{u}}}_{\epsilon }}{\mathrm{\partial }t}-\frac{\mathrm{\partial }{\mathbf{\text{u}}}_{\epsilon }^{\left[1\right]}}{\mathrm{\partial }t})\frac{\mathrm{\partial }{\mathbf{\text{s}}}_{\epsilon }^{\ast }}{\mathrm{\partial }t}-{\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}\frac{{\mathrm{\partial }}^2{\mathbf{\text{D}}}_{\epsilon }}{\mathrm{\partial }{t}^2}\frac{\mathrm{\partial }{\mathbf{\text{s}}}_{\epsilon }^{\ast }}{\mathrm{\partial }t}-\alpha {\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}\mathrm{\nabla }\frac{\mathrm{\partial }{\mathbf{\text{s}}}_{\epsilon }^{\ast }}{\mathrm{\partial }t}\mathrm{\nabla }\frac{\mathrm{\partial }{\mathbf{\text{D}}}_{\epsilon }}{\mathrm{\partial }t}\\ & -\beta {\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}\text{div}\frac{\mathrm{\partial }{\mathbf{\text{D}}}_{\epsilon }}{\mathrm{\partial }t}\text{div}\frac{\mathrm{\partial }{\mathbf{\text{s}}}_{\epsilon }^{\ast }}{\mathrm{\partial }t}-2a{\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}\frac{\mathrm{\partial }{\mathbf{\text{s}}}_{\epsilon }^{\ast }}{\mathrm{\partial }t}\frac{\mathrm{\partial }{\mathbf{\text{D}}}_{\epsilon }}{\mathrm{\partial }t}-{\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}\frac{\mathrm{\partial }{\mathit{\xi }}_{\epsilon }}{\mathrm{\partial }t}\frac{\mathrm{\partial }{\mathbf{\text{s}}}_{\epsilon }^{\ast }}{\mathrm{\partial }t}.\end{array}$(109) ), applying Young's inequality and integrating over t we obtain: (110) $\underset{t\in [0,T]}{sup}||\frac{\mathrm{\partial }{\mathbf{\text{s}}}_{\epsilon }^{\ast }}{\mathrm{\partial }t}|{|}_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}^2+{\int }_0^T||\mathrm{\nabla }\frac{\mathrm{\partial }{\mathbf{\text{s}}}_{\epsilon }^{\ast }}{\mathrm{\partial }t}|{|}_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}^2\le C{\epsilon }^2{\int }_0^T||\mathrm{\nabla }(\frac{\mathrm{\partial }{\mathbf{\text{u}}}_{\epsilon }}{\mathrm{\partial }t}-\frac{\mathrm{\partial }{\mathbf{\text{u}}}_{\epsilon }^{\left[2\right]}}{\mathrm{\partial }t})|{|}_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}^2+C{\epsilon }^{10}.$(110) The problem satisfied by the asymptotic approximation ${\mathbf{\text{u}}}_{\epsilon }^{\left[2\right]}$ reads: (111) $\begin{array}{rl}& \frac{\mathrm{\partial }{\mathbf{\text{u}}}_{\epsilon }^{\left[2\right]}}{\mathrm{\partial }t}-\mu \mathrm{\Delta }{\mathbf{\text{u}}}_{\epsilon }^{\left[2\right]}+\mathrm{\nabla }{p}_{\epsilon }^{\left[2\right]}=a\text{rot}{\mathbf{\text{w}}}_{\epsilon }^{\left[1\right]}+\mathbf{\text{F}}+{\mathbf{\text{E}}}_{\epsilon }\text{in}{\mathrm{\Omega }}_{\epsilon }^{\alpha },\\ & \text{div}{\mathbf{\text{u}}}_{\epsilon }^{\left[2\right]}={\mathit{\pi }}_{\epsilon }\text{in}{\mathrm{\Omega }}_{\epsilon }^{\alpha },\\ & {\mathbf{\text{u}}}_{\epsilon }^{\left[2\right]}=\mathbf{\text{0}}\text{on}{\mathrm{\Gamma }}_{\epsilon }^{\alpha },\\ & {\mathbf{\text{u}}}_{\epsilon }^{\left[2\right]}={\epsilon }^2{\mathbf{\text{h}}}_0+{\mathbf{\text{r}}}_0\text{on}{\mathrm{\Sigma }}_{\epsilon }^0,{\mathbf{\text{u}}}_{\epsilon }^{\left[2\right]}={\epsilon }^2{\mathbf{\text{h}}}_l+{\mathbf{\text{r}}}_l\text{on}{\mathrm{\Sigma }}_{\epsilon }^l,\\ & {\mathbf{\text{u}}}_{\epsilon }^{\left[2\right]}(0,\cdot )=\mathbf{\text{0}},\end{array}$(111) where $\parallel {\mathbf{\text{E}}}_{\epsilon }{\parallel }_{L^2(0,T;L^2({\mathrm{\Omega }}_{\epsilon }^{\alpha }\left)\right)}=\mathcal{O}\left({\epsilon }^4\right),\parallel {\mathit{\pi }}_{\epsilon }{\parallel }_{L^2(0,T;L^2({\mathrm{\Omega }}_{\epsilon }^{\alpha }\left)\right)}=\mathcal{O}\left({\epsilon }^{9/2}\right),\parallel {\mathbf{\text{r}}}_i^{\epsilon }{\parallel }_{L^2(0,T;H^{1/2}({\mathrm{\Sigma }}_{\epsilon }^i\left)\right)}=\mathcal{O}\left({\epsilon }^{11/2}\right),i=0,l$.

Denoting ${\mathbf{\text{R}}}_{\epsilon }={\mathbf{\text{u}}}_{\epsilon }-{\mathbf{\text{u}}}_{\epsilon }^{\left[2\right]}$, $r_{\epsilon }=p_{\epsilon }-p_{\epsilon }^{\left[2\right]}$ and subtracting $(5{)}_1,(5{)}_2,(6{)}_1,(6{)}_{3,1}$ and (111(111) $\begin{array}{rl}& \frac{\mathrm{\partial }{\mathbf{\text{u}}}_{\epsilon }^{\left[2\right]}}{\mathrm{\partial }t}-\mu \mathrm{\Delta }{\mathbf{\text{u}}}_{\epsilon }^{\left[2\right]}+\mathrm{\nabla }{p}_{\epsilon }^{\left[2\right]}=a\text{rot}{\mathbf{\text{w}}}_{\epsilon }^{\left[1\right]}+\mathbf{\text{F}}+{\mathbf{\text{E}}}_{\epsilon }\text{in}{\mathrm{\Omega }}_{\epsilon }^{\alpha },\\ & \text{div}{\mathbf{\text{u}}}_{\epsilon }^{\left[2\right]}={\mathit{\pi }}_{\epsilon }\text{in}{\mathrm{\Omega }}_{\epsilon }^{\alpha },\\ & {\mathbf{\text{u}}}_{\epsilon }^{\left[2\right]}=\mathbf{\text{0}}\text{on}{\mathrm{\Gamma }}_{\epsilon }^{\alpha },\\ & {\mathbf{\text{u}}}_{\epsilon }^{\left[2\right]}={\epsilon }^2{\mathbf{\text{h}}}_0+{\mathbf{\text{r}}}_0\text{on}{\mathrm{\Sigma }}_{\epsilon }^0,{\mathbf{\text{u}}}_{\epsilon }^{\left[2\right]}={\epsilon }^2{\mathbf{\text{h}}}_l+{\mathbf{\text{r}}}_l\text{on}{\mathrm{\Sigma }}_{\epsilon }^l,\\ & {\mathbf{\text{u}}}_{\epsilon }^{\left[2\right]}(0,\cdot )=\mathbf{\text{0}},\end{array}$(111) ), we obtain: (112) $\begin{array}{rl}& \frac{\mathrm{\partial }{\mathbf{\text{R}}}_{\epsilon }}{\mathrm{\partial }t}-\mu \mathrm{\Delta }{\mathbf{\text{R}}}_{\epsilon }+\mathrm{\nabla }{r}_{\epsilon }=a\text{rot}({\mathbf{\text{w}}}_{\epsilon }-{\mathbf{\text{w}}}_{\epsilon }^{\left[1\right]})-{\mathbf{\text{E}}}_{\epsilon }\text{in}{\mathrm{\Omega }}_{\epsilon }^{\alpha },\\ & \text{div}{\mathbf{\text{R}}}_{\epsilon }=-{\mathit{\pi }}_{\epsilon }\text{in}{\mathrm{\Omega }}_{\epsilon }^{\alpha },\\ & {\mathbf{\text{R}}}_{\epsilon }=\mathbf{\text{0}}\text{on}{\mathrm{\Gamma }}_{\epsilon }^{\alpha },\\ & {\mathbf{\text{R}}}_{\epsilon }=-{\mathbf{\text{r}}}_0\text{on}{\mathrm{\Sigma }}_{\epsilon }^0{\mathbf{\text{R}}}_{\epsilon }=-{\mathbf{\text{r}}}_l\text{on}{\mathrm{\Sigma }}_{\epsilon }^l,\\ & {\mathbf{\text{R}}}_{\epsilon }(0,\cdot )=\mathbf{\text{0}}.\end{array}$(112) It is important to emphasize that the divergence estimate $\parallel {\mathit{\pi }}_{\epsilon }{\parallel }_{L^2(0,T;L^2({\mathrm{\Omega }}_{\epsilon }^{\alpha }\left)\right)}=\mathcal{O}\left({\epsilon }^{9/2}\right)$ cannot be improved in the general case, due to its structure (see (68(68) $\begin{array}{rl}& +2\kappa ({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast })(\left({\kappa }^{\prime }\right({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast })+\kappa \tau ({\mathbf{\text{e}}}_{\alpha }^{\perp }\cdot {\mathit{y}}_{\ast }\left)\right){\mathcal{V}}_0^1+{\kappa }^2({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast })\cos \alpha {\mathcal{V}}_0^2-{\kappa }^2({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast })\sin \alpha {\mathcal{V}}_0^3)\\ & +3{\kappa }^2({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast }{)}^2(\frac{\mathrm{\partial }{\mathcal{V}}_1^1}{\mathrm{\partial }{y}_1}-\kappa ({\mathcal{V}}_0^2\cos \alpha -{\mathcal{V}}_0^3\sin \alpha ))+4{\kappa }^3({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast }{)}^3\frac{\mathrm{\partial }{\mathcal{V}}_0^1}{\mathrm{\partial }{y}_1})\\ & +{\epsilon }^4(-\kappa ({\mathcal{Y}}_2^2\cos \alpha -{\mathcal{Y}}_2^3\sin \alpha )+\left({\kappa }^{\prime }\right({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast })+\kappa \tau ({\mathbf{\text{e}}}_{\alpha }^{\perp }\cdot {\mathit{y}}_{\ast }\left)\right){\mathcal{Y}}_1^1\\ & +\kappa ({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast })\left(\kappa \right({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast })+\kappa \tau ({\mathbf{\text{e}}}_{\alpha }^{\perp }\cdot {\mathit{y}}_{\ast }\left)\right){\mathcal{Y}}_0^1\\ & +{\kappa }^2({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast })\cos \alpha {\mathcal{Y}}_1^2-{\kappa }^2({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast })\sin \alpha {\mathcal{Y}}_1^3+2\kappa ({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast })\\ & (\frac{\mathrm{\partial }{\mathcal{Y}}_2^1}{\mathrm{\partial }{y}_1}-\kappa ({\mathcal{Y}}_1^2\cos \alpha -{\mathcal{Y}}_1^3\sin \alpha ))\\ & +2\kappa ({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast })(\left({\kappa }^{\prime }\right({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast })+\kappa \tau ({\mathbf{\text{e}}}_{\alpha }^{\perp }\cdot {\mathit{y}}_{\ast }\left)\right){\mathcal{Y}}_0^1+{\kappa }^2({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast })\cos \alpha {\mathcal{Y}}_0^2-{\kappa }^2({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast })\sin \alpha {\mathcal{Y}}_0^3)\\ & +3{\kappa }^2({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast }{)}^2(\frac{\mathrm{\partial }{\mathcal{Y}}_1^1}{\mathrm{\partial }{y}_1}-\kappa ({\mathcal{Y}}_0^2\cos \alpha -{\mathcal{Y}}_0^3\sin \alpha ))+4{\kappa }^3({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast }{)}^3\frac{\mathrm{\partial }{\mathcal{Y}}_0^1}{\mathrm{\partial }{y}_1})\\ & +\mathcal{O}\left({\epsilon }^5\right)\end{array}$(68) )–(72(72) $\parallel {\mathit{\pi }}_{\epsilon ,1}{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}=\mathcal{O}\left({\epsilon }^{9/2}\right),$(72) )). However, under certain assumptions on $\kappa ,\alpha$ and ${\mathbf{\text{h}}}_0^{\epsilon },{\mathbf{\text{h}}}_l^{\epsilon },\mathbf{\text{F}}$ and $\mathbf{\text{G}}$, this estimate can, in fact, be improved providing better overall estimates. We refer the reader to (73(73) ${\mathit{\pi }}_{\epsilon }={\mathit{\pi }}_{\epsilon ,3}+{\mathit{\pi }}_{\epsilon ,res}.$(73) )–(79(79) $\text{div}{\widetilde{\mathbf{\text{u}}}}_{\epsilon }^{\left[2\right]}={\tilde{\mathit{\pi }}}_{\epsilon },\parallel {\tilde{\mathit{\pi }}}_{\epsilon }{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}=\mathcal{O}\left({\epsilon }^{11/2}\right).$(79) ) in Section 4.3 and Remark 5.6 at the end of this Section.

Let us define ${\mathit{\zeta }}_{\epsilon }$ as the solution of the problem $\begin{array}{rl}& \text{div}{\mathit{\zeta }}_{\epsilon }=-{\mathit{\pi }}_{\epsilon }\text{in}{\mathrm{\Omega }}_{\epsilon }^{\alpha },\\ & {\mathit{\zeta }}_{\epsilon }=0\text{on}{\mathrm{\Gamma }}_{\epsilon }^{\alpha },\\ & {\mathit{\zeta }}_{\epsilon }=-{\mathbf{\text{r}}}_0\text{on}{\mathrm{\Sigma }}_{\epsilon }^0{\mathbf{\text{R}}}_{\epsilon }=-{\mathbf{\text{r}}}_l\text{on}{\mathrm{\Sigma }}_{\epsilon }^l\end{array}$ satisfying the following estimates (see Lemma 5.2): (113) $\parallel \mathrm{\nabla }{\mathit{\zeta }}_{\epsilon }{\parallel }_{L^2(0,T;L^2({\mathrm{\Omega }}_{\epsilon }^{\alpha }\left)\right)}+||\mathrm{\nabla }\frac{\mathrm{\partial }{\mathit{\zeta }}_{\epsilon }}{\mathrm{\partial }t}|{|}_{L^2(0,T;L^2({\mathrm{\Omega }}_{\epsilon }^{\alpha }\left)\right)}+||\mathrm{\nabla }\frac{{\mathrm{\partial }}^2{\mathit{\zeta }}_{\epsilon }}{\mathrm{\partial }{t}^2}|{|}_{L^2(0,T;L^2({\mathrm{\Omega }}_{\epsilon }^{\alpha }\left)\right)}\le C{\epsilon }^{7/2}.$(113) Multiplying the equation (112(112) $\begin{array}{rl}& \frac{\mathrm{\partial }{\mathbf{\text{R}}}_{\epsilon }}{\mathrm{\partial }t}-\mu \mathrm{\Delta }{\mathbf{\text{R}}}_{\epsilon }+\mathrm{\nabla }{r}_{\epsilon }=a\text{rot}({\mathbf{\text{w}}}_{\epsilon }-{\mathbf{\text{w}}}_{\epsilon }^{\left[1\right]})-{\mathbf{\text{E}}}_{\epsilon }\text{in}{\mathrm{\Omega }}_{\epsilon }^{\alpha },\\ & \text{div}{\mathbf{\text{R}}}_{\epsilon }=-{\mathit{\pi }}_{\epsilon }\text{in}{\mathrm{\Omega }}_{\epsilon }^{\alpha },\\ & {\mathbf{\text{R}}}_{\epsilon }=\mathbf{\text{0}}\text{on}{\mathrm{\Gamma }}_{\epsilon }^{\alpha },\\ & {\mathbf{\text{R}}}_{\epsilon }=-{\mathbf{\text{r}}}_0\text{on}{\mathrm{\Sigma }}_{\epsilon }^0{\mathbf{\text{R}}}_{\epsilon }=-{\mathbf{\text{r}}}_l\text{on}{\mathrm{\Sigma }}_{\epsilon }^l,\\ & {\mathbf{\text{R}}}_{\epsilon }(0,\cdot )=\mathbf{\text{0}}.\end{array}$(112) )₁ with function ${\mathbf{\text{R}}}_{\epsilon }^{\ast }={\mathbf{\text{R}}}_{\epsilon }-{\mathit{\zeta }}_{\epsilon }$ and integrating over ${\mathrm{\Omega }}_{\epsilon }^{\alpha }$, we obtain: (114) $\begin{array}{rl}& \frac{1}{2}\frac{\mathrm{d}}{\mathrm{d}t}\parallel {\mathbf{\text{R}}}_{\epsilon }^{\ast }{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}^2+\mu \parallel \mathrm{\nabla }{\mathbf{\text{R}}}_{\epsilon }^{\ast }{\parallel }_{L^2({\mathrm{\Omega }}_{\epsilon }^{\alpha }}^2\le -{\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}\frac{\mathrm{\partial }{\mathit{\zeta }}_{\epsilon }}{\mathrm{\partial }t}{\mathbf{\text{R}}}_{\epsilon }^{\ast }+\mu {\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}\mathrm{\nabla }{\mathit{\zeta }}_{\epsilon }\mathrm{\nabla }{\mathbf{\text{R}}}_{\epsilon }^{\ast }\\ & +a{\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}\text{rot}({\mathbf{\text{w}}}_{\epsilon }-{\mathbf{\text{w}}}_{\epsilon }^{\left[1\right]}){\mathbf{\text{R}}}_{\epsilon }^{\ast }-{\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}{\mathbf{\text{E}}}_{\epsilon }{\mathbf{\text{R}}}_{\epsilon }^{\ast }.\end{array}$(114) Estimating the terms on the right–hand side of Equation (114(114) $\begin{array}{rl}& \frac{1}{2}\frac{\mathrm{d}}{\mathrm{d}t}\parallel {\mathbf{\text{R}}}_{\epsilon }^{\ast }{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}^2+\mu \parallel \mathrm{\nabla }{\mathbf{\text{R}}}_{\epsilon }^{\ast }{\parallel }_{L^2({\mathrm{\Omega }}_{\epsilon }^{\alpha }}^2\le -{\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}\frac{\mathrm{\partial }{\mathit{\zeta }}_{\epsilon }}{\mathrm{\partial }t}{\mathbf{\text{R}}}_{\epsilon }^{\ast }+\mu {\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}\mathrm{\nabla }{\mathit{\zeta }}_{\epsilon }\mathrm{\nabla }{\mathbf{\text{R}}}_{\epsilon }^{\ast }\\ & +a{\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}\text{rot}({\mathbf{\text{w}}}_{\epsilon }-{\mathbf{\text{w}}}_{\epsilon }^{\left[1\right]}){\mathbf{\text{R}}}_{\epsilon }^{\ast }-{\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}{\mathbf{\text{E}}}_{\epsilon }{\mathbf{\text{R}}}_{\epsilon }^{\ast }.\end{array}$(114) ) using Poincaré's inequality (80(80) $\parallel {\mathit{\varphi }}_{\epsilon }(t,\cdot ){\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}\le C\epsilon \parallel \mathrm{\nabla }{\mathit{\varphi }}_{\epsilon }(t,\cdot ){\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)},$(80) ) yields (115) $\begin{array}{rl}& |{\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}\frac{\mathrm{\partial }{\mathit{\zeta }}_{\epsilon }}{\mathrm{\partial }t}{\mathbf{\text{R}}}_{\epsilon }^{\ast }|\le ||\frac{\mathrm{\partial }{\mathit{\zeta }}_{\epsilon }}{\mathrm{\partial }t}|{|}_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}\parallel {\mathbf{\text{R}}}_{\epsilon }^{\ast }{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}\le C{\epsilon }^2||\mathrm{\nabla }\frac{\mathrm{\partial }{\mathit{\zeta }}_{\epsilon }}{\mathrm{\partial }t}|{|}_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}\parallel \mathrm{\nabla }{\mathbf{\text{R}}}_{\epsilon }^{\ast }{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)},\\ & |{\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}\mathrm{\nabla }{\mathit{\zeta }}_{\epsilon }\mathrm{\nabla }{\mathbf{\text{R}}}_{\epsilon }^{\ast }|\le \parallel \mathrm{\nabla }{\mathit{\zeta }}_{\epsilon }{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}\parallel \mathrm{\nabla }{\mathbf{\text{R}}}_{\epsilon }^{\ast }{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)},\\ & |{\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha })}\text{rot}({\mathbf{\text{w}}}_{\epsilon }-{\mathbf{\text{w}}}_{\epsilon }^{\left[1\right]}){\mathbf{\text{R}}}_{\epsilon }^{\ast }|\le C\parallel \mathrm{\nabla }({\mathbf{\text{w}}}_{\epsilon }-{\mathbf{\text{w}}}_{\epsilon }^{\left[1\right]}){\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}\parallel {\mathbf{\text{R}}}_{\epsilon }^{\ast }{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}\\ & \le C(\parallel \mathrm{\nabla }({\mathbf{\text{w}}}_{\epsilon }-{\mathbf{\text{w}}}_{\epsilon }^{\left[2\right]}){\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}+\parallel \mathrm{\nabla }({\mathbf{\text{w}}}_{\epsilon }^{\left[2\right]}-{\mathbf{\text{w}}}_{\epsilon }^{\left[1\right]}){\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)})\parallel {\mathbf{\text{R}}}_{\epsilon }^{\ast }{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}\\ & \le C\epsilon \parallel \mathrm{\nabla }{\mathbf{\text{s}}}_{\epsilon }^{\ast }{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}\parallel \mathrm{\nabla }{\mathbf{\text{R}}}_{\epsilon }^{\ast }{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}+C{\epsilon }^5\parallel \mathrm{\nabla }{\mathbf{\text{R}}}_{\epsilon }^{\ast }{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)},\\ & |{\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}{\mathbf{\text{E}}}_{\epsilon }{\mathbf{\text{R}}}_{\epsilon }^{\ast }|\le \parallel {\mathbf{\text{E}}}_{\epsilon }{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}\parallel {\mathbf{\text{R}}}_{\epsilon }^{\ast }{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}\le C\epsilon \parallel {\mathbf{\text{E}}}_{\epsilon }{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}\parallel \mathrm{\nabla }{\mathbf{\text{R}}}_{\epsilon }^{\ast }{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}.\end{array}$(115) Applying the estimates (115(115) $\begin{array}{rl}& |{\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}\frac{\mathrm{\partial }{\mathit{\zeta }}_{\epsilon }}{\mathrm{\partial }t}{\mathbf{\text{R}}}_{\epsilon }^{\ast }|\le ||\frac{\mathrm{\partial }{\mathit{\zeta }}_{\epsilon }}{\mathrm{\partial }t}|{|}_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}\parallel {\mathbf{\text{R}}}_{\epsilon }^{\ast }{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}\le C{\epsilon }^2||\mathrm{\nabla }\frac{\mathrm{\partial }{\mathit{\zeta }}_{\epsilon }}{\mathrm{\partial }t}|{|}_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}\parallel \mathrm{\nabla }{\mathbf{\text{R}}}_{\epsilon }^{\ast }{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)},\\ & |{\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}\mathrm{\nabla }{\mathit{\zeta }}_{\epsilon }\mathrm{\nabla }{\mathbf{\text{R}}}_{\epsilon }^{\ast }|\le \parallel \mathrm{\nabla }{\mathit{\zeta }}_{\epsilon }{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}\parallel \mathrm{\nabla }{\mathbf{\text{R}}}_{\epsilon }^{\ast }{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)},\\ & |{\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha })}\text{rot}({\mathbf{\text{w}}}_{\epsilon }-{\mathbf{\text{w}}}_{\epsilon }^{\left[1\right]}){\mathbf{\text{R}}}_{\epsilon }^{\ast }|\le C\parallel \mathrm{\nabla }({\mathbf{\text{w}}}_{\epsilon }-{\mathbf{\text{w}}}_{\epsilon }^{\left[1\right]}){\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}\parallel {\mathbf{\text{R}}}_{\epsilon }^{\ast }{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}\\ & \le C(\parallel \mathrm{\nabla }({\mathbf{\text{w}}}_{\epsilon }-{\mathbf{\text{w}}}_{\epsilon }^{\left[2\right]}){\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}+\parallel \mathrm{\nabla }({\mathbf{\text{w}}}_{\epsilon }^{\left[2\right]}-{\mathbf{\text{w}}}_{\epsilon }^{\left[1\right]}){\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)})\parallel {\mathbf{\text{R}}}_{\epsilon }^{\ast }{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}\\ & \le C\epsilon \parallel \mathrm{\nabla }{\mathbf{\text{s}}}_{\epsilon }^{\ast }{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}\parallel \mathrm{\nabla }{\mathbf{\text{R}}}_{\epsilon }^{\ast }{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}+C{\epsilon }^5\parallel \mathrm{\nabla }{\mathbf{\text{R}}}_{\epsilon }^{\ast }{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)},\\ & |{\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}{\mathbf{\text{E}}}_{\epsilon }{\mathbf{\text{R}}}_{\epsilon }^{\ast }|\le \parallel {\mathbf{\text{E}}}_{\epsilon }{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}\parallel {\mathbf{\text{R}}}_{\epsilon }^{\ast }{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}\le C\epsilon \parallel {\mathbf{\text{E}}}_{\epsilon }{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}\parallel \mathrm{\nabla }{\mathbf{\text{R}}}_{\epsilon }^{\ast }{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}.\end{array}$(115) ) and Young's inequality on the right–hand side of (114(114) $\begin{array}{rl}& \frac{1}{2}\frac{\mathrm{d}}{\mathrm{d}t}\parallel {\mathbf{\text{R}}}_{\epsilon }^{\ast }{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}^2+\mu \parallel \mathrm{\nabla }{\mathbf{\text{R}}}_{\epsilon }^{\ast }{\parallel }_{L^2({\mathrm{\Omega }}_{\epsilon }^{\alpha }}^2\le -{\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}\frac{\mathrm{\partial }{\mathit{\zeta }}_{\epsilon }}{\mathrm{\partial }t}{\mathbf{\text{R}}}_{\epsilon }^{\ast }+\mu {\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}\mathrm{\nabla }{\mathit{\zeta }}_{\epsilon }\mathrm{\nabla }{\mathbf{\text{R}}}_{\epsilon }^{\ast }\\ & +a{\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}\text{rot}({\mathbf{\text{w}}}_{\epsilon }-{\mathbf{\text{w}}}_{\epsilon }^{\left[1\right]}){\mathbf{\text{R}}}_{\epsilon }^{\ast }-{\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}{\mathbf{\text{E}}}_{\epsilon }{\mathbf{\text{R}}}_{\epsilon }^{\ast }.\end{array}$(114) ), integrating over t and using (108(108) $\underset{t\in [0,T]}{sup}\parallel {\mathbf{\text{s}}}_{\epsilon }^{\ast }{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}^2+{\int }_0^T\parallel \mathrm{\nabla }{\mathbf{\text{s}}}_{\epsilon }^{\ast }{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}^2\le C{\epsilon }^2{\int }_0^t\parallel \mathrm{\nabla }({\mathbf{\text{u}}}_{\epsilon }-{\mathbf{\text{u}}}_{\epsilon }^{\left[2\right]}){\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}^2+C{\epsilon }^{10}.$(108) ), (113(113) $\parallel \mathrm{\nabla }{\mathit{\zeta }}_{\epsilon }{\parallel }_{L^2(0,T;L^2({\mathrm{\Omega }}_{\epsilon }^{\alpha }\left)\right)}+||\mathrm{\nabla }\frac{\mathrm{\partial }{\mathit{\zeta }}_{\epsilon }}{\mathrm{\partial }t}|{|}_{L^2(0,T;L^2({\mathrm{\Omega }}_{\epsilon }^{\alpha }\left)\right)}+||\mathrm{\nabla }\frac{{\mathrm{\partial }}^2{\mathit{\zeta }}_{\epsilon }}{\mathrm{\partial }{t}^2}|{|}_{L^2(0,T;L^2({\mathrm{\Omega }}_{\epsilon }^{\alpha }\left)\right)}\le C{\epsilon }^{7/2}.$(113) ), for sufficiently small ε we get: (116) $\underset{t\in [0,T]}{sup}\parallel {\mathbf{\text{R}}}_{\epsilon }^{\ast }{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}^2+{\int }_0^T\parallel \mathrm{\nabla }{\mathbf{\text{R}}}_{\epsilon }^{\ast }{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}^2\le C{\epsilon }^7,$(116) and from (108(108) $\underset{t\in [0,T]}{sup}\parallel {\mathbf{\text{s}}}_{\epsilon }^{\ast }{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}^2+{\int }_0^T\parallel \mathrm{\nabla }{\mathbf{\text{s}}}_{\epsilon }^{\ast }{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}^2\le C{\epsilon }^2{\int }_0^t\parallel \mathrm{\nabla }({\mathbf{\text{u}}}_{\epsilon }-{\mathbf{\text{u}}}_{\epsilon }^{\left[2\right]}){\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}^2+C{\epsilon }^{10}.$(108) ) we now easily get (117) $\underset{t\in [0,T]}{sup}\parallel {\mathbf{\text{s}}}_{\epsilon }^{\ast }{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}^2+{\int }_0^T\parallel \mathrm{\nabla }{\mathbf{\text{s}}}_{\epsilon }^{\ast }{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}^2\le C{\epsilon }^9,$(117) implying (102(102) $\begin{array}{rl}& \underset{t\in [0,T]}{sup}\parallel {\mathbf{u}}_{\epsilon }(t,\cdot )-{\mathbf{u}}_{\epsilon }^{\left[2\right]}(t,\cdot ){\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}+\parallel \mathrm{\nabla }({\mathbf{u}}_{\epsilon }-{\mathbf{u}}_{\epsilon }^{\left[2\right]})|{|}_{L^2(0,T;L^2({\mathrm{\Omega }}_{\epsilon }^{\alpha }\left)\right)}\le C{\epsilon }^{7/2},\\ & \underset{t\in [0,T]}{sup}||\frac{\mathrm{\partial }{\mathbf{u}}_{\epsilon }(t,\cdot )}{\mathrm{\partial }t}-\frac{\mathrm{\partial }{\mathbf{u}}_{\epsilon }^{\left[2\right]}(t,\cdot )}{\mathrm{\partial }t}|{|}_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}+||\mathrm{\nabla }(\frac{\mathrm{\partial }{\mathbf{u}}_{\epsilon }}{\mathrm{\partial }t}-\frac{\mathrm{\partial }{\mathbf{u}}_{\epsilon }^{\left[2\right]}}{\mathrm{\partial }t})|{|}_{L^2(0,T;L^2({\mathrm{\Omega }}_{\epsilon }^{\alpha }\left)\right)}\le C{\epsilon }^{7/2},\\ & \underset{t\in [0,T]}{sup}\parallel {\mathbf{w}}_{\epsilon }(t,\cdot )-{\mathbf{w}}_{\epsilon }^{\left[2\right]}(t,\cdot ){\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}+\parallel \mathrm{\nabla }({\mathbf{w}}_{\epsilon }-{\mathbf{w}}_{\epsilon }^{\left[2\right]})|{|}_{L^2(0,T;L^2({\mathrm{\Omega }}_{\epsilon }^{\alpha }\left)\right)}\le C{\epsilon }^{9/2},\\ & \underset{t\in [0,T]}{sup}||\frac{\mathrm{\partial }{\mathbf{w}}_{\epsilon }(t,\cdot )}{\mathrm{\partial }t}-\frac{\mathrm{\partial }{\mathbf{w}}_{\epsilon }^{\left[2\right]}(t,\cdot )}{\mathrm{\partial }t}|{|}_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}+||\mathrm{\nabla }(\frac{\mathrm{\partial }{\mathbf{w}}_{\epsilon }}{\mathrm{\partial }t}-\frac{\mathrm{\partial }{\mathbf{w}}_{\epsilon }^{\left[2\right]}}{\mathrm{\partial }t})|{|}_{L^2(0,T;L^2({\mathrm{\Omega }}_{\epsilon }^{\alpha }\left)\right)}\le C{\epsilon }^{9/2},\\ & \parallel p_{\epsilon }-p_{\epsilon }^{\left[2\right]}{\parallel }_{L^2(0,T;L^2({\mathrm{\Omega }}_{\epsilon }^{\alpha }\left)\right)}\le C{\epsilon }^{5/2},\end{array}$(102) )₁ and (102(102) $\begin{array}{rl}& \underset{t\in [0,T]}{sup}\parallel {\mathbf{u}}_{\epsilon }(t,\cdot )-{\mathbf{u}}_{\epsilon }^{\left[2\right]}(t,\cdot ){\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}+\parallel \mathrm{\nabla }({\mathbf{u}}_{\epsilon }-{\mathbf{u}}_{\epsilon }^{\left[2\right]})|{|}_{L^2(0,T;L^2({\mathrm{\Omega }}_{\epsilon }^{\alpha }\left)\right)}\le C{\epsilon }^{7/2},\\ & \underset{t\in [0,T]}{sup}||\frac{\mathrm{\partial }{\mathbf{u}}_{\epsilon }(t,\cdot )}{\mathrm{\partial }t}-\frac{\mathrm{\partial }{\mathbf{u}}_{\epsilon }^{\left[2\right]}(t,\cdot )}{\mathrm{\partial }t}|{|}_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}+||\mathrm{\nabla }(\frac{\mathrm{\partial }{\mathbf{u}}_{\epsilon }}{\mathrm{\partial }t}-\frac{\mathrm{\partial }{\mathbf{u}}_{\epsilon }^{\left[2\right]}}{\mathrm{\partial }t})|{|}_{L^2(0,T;L^2({\mathrm{\Omega }}_{\epsilon }^{\alpha }\left)\right)}\le C{\epsilon }^{7/2},\\ & \underset{t\in [0,T]}{sup}\parallel {\mathbf{w}}_{\epsilon }(t,\cdot )-{\mathbf{w}}_{\epsilon }^{\left[2\right]}(t,\cdot ){\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}+\parallel \mathrm{\nabla }({\mathbf{w}}_{\epsilon }-{\mathbf{w}}_{\epsilon }^{\left[2\right]})|{|}_{L^2(0,T;L^2({\mathrm{\Omega }}_{\epsilon }^{\alpha }\left)\right)}\le C{\epsilon }^{9/2},\\ & \underset{t\in [0,T]}{sup}||\frac{\mathrm{\partial }{\mathbf{w}}_{\epsilon }(t,\cdot )}{\mathrm{\partial }t}-\frac{\mathrm{\partial }{\mathbf{w}}_{\epsilon }^{\left[2\right]}(t,\cdot )}{\mathrm{\partial }t}|{|}_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}+||\mathrm{\nabla }(\frac{\mathrm{\partial }{\mathbf{w}}_{\epsilon }}{\mathrm{\partial }t}-\frac{\mathrm{\partial }{\mathbf{w}}_{\epsilon }^{\left[2\right]}}{\mathrm{\partial }t})|{|}_{L^2(0,T;L^2({\mathrm{\Omega }}_{\epsilon }^{\alpha }\left)\right)}\le C{\epsilon }^{9/2},\\ & \parallel p_{\epsilon }-p_{\epsilon }^{\left[2\right]}{\parallel }_{L^2(0,T;L^2({\mathrm{\Omega }}_{\epsilon }^{\alpha }\left)\right)}\le C{\epsilon }^{5/2},\end{array}$(102) )₃.

Differentiating equation (112(112) $\begin{array}{rl}& \frac{\mathrm{\partial }{\mathbf{\text{R}}}_{\epsilon }}{\mathrm{\partial }t}-\mu \mathrm{\Delta }{\mathbf{\text{R}}}_{\epsilon }+\mathrm{\nabla }{r}_{\epsilon }=a\text{rot}({\mathbf{\text{w}}}_{\epsilon }-{\mathbf{\text{w}}}_{\epsilon }^{\left[1\right]})-{\mathbf{\text{E}}}_{\epsilon }\text{in}{\mathrm{\Omega }}_{\epsilon }^{\alpha },\\ & \text{div}{\mathbf{\text{R}}}_{\epsilon }=-{\mathit{\pi }}_{\epsilon }\text{in}{\mathrm{\Omega }}_{\epsilon }^{\alpha },\\ & {\mathbf{\text{R}}}_{\epsilon }=\mathbf{\text{0}}\text{on}{\mathrm{\Gamma }}_{\epsilon }^{\alpha },\\ & {\mathbf{\text{R}}}_{\epsilon }=-{\mathbf{\text{r}}}_0\text{on}{\mathrm{\Sigma }}_{\epsilon }^0{\mathbf{\text{R}}}_{\epsilon }=-{\mathbf{\text{r}}}_l\text{on}{\mathrm{\Sigma }}_{\epsilon }^l,\\ & {\mathbf{\text{R}}}_{\epsilon }(0,\cdot )=\mathbf{\text{0}}.\end{array}$(112) )₁ with respect to t, multiplying by ${\displaystyle \frac{\mathrm{\partial }{\mathbf{\text{R}}}_{\epsilon }^{\ast }}{\mathrm{\partial }t}}={\displaystyle \frac{\mathrm{\partial }{\mathbf{\text{R}}}_{\epsilon }}{\mathrm{\partial }t}}-{\displaystyle \frac{\mathrm{\partial }{\mathit{\zeta }}_{\epsilon }}{\mathrm{\partial }t}}$ and integrating over ${\mathrm{\Omega }}_{\epsilon }^{\alpha }$, we get: (118) $\begin{array}{rl}\frac{1}{2}\frac{\mathrm{d}}{\mathrm{d}t}{\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}|\frac{\mathrm{\partial }{\mathbf{\text{R}}}_{\epsilon }^{\ast }}{\mathrm{\partial }t}{|}_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}^2+\mu {\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}|\mathrm{\nabla }\frac{\mathrm{\partial }{\mathbf{\text{R}}}_{\epsilon }^{\ast }}{\mathrm{\partial }t}{|}_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}^2=& a{\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}\text{rot}(\frac{\mathrm{\partial }{\mathbf{\text{w}}}_{\epsilon }}{\mathrm{\partial }t}-\frac{\mathrm{\partial }{\mathbf{\text{w}}}_{\epsilon }^{\left[1\right]}}{\mathrm{\partial }t})\frac{\mathrm{\partial }{\mathbf{\text{R}}}_{\epsilon }^{\ast }}{\mathrm{\partial }t}-{\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}\frac{\mathrm{\partial }{\mathbf{\text{E}}}_{\epsilon }}{\mathrm{\partial }t}\frac{\mathrm{\partial }{\mathbf{\text{R}}}_{\epsilon }^{\ast }}{\mathrm{\partial }t}\\ & -{\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}\frac{{\mathrm{\partial }}^2{\mathit{\zeta }}_{\epsilon }}{\mathrm{\partial }{t}^2}\frac{\mathrm{\partial }{\mathbf{\text{R}}}_{\epsilon }^{\ast }}{\mathrm{\partial }t}-\alpha {\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}\mathrm{\nabla }\frac{\mathrm{\partial }{\mathit{\zeta }}_{\epsilon }}{\mathrm{\partial }t}\mathrm{\nabla }\frac{\mathrm{\partial }{\mathbf{\text{R}}}_{\epsilon }^{\ast }}{\mathrm{\partial }t}.\end{array}$(118) In much the same way, using Poincaré's and Young's inequality to estimate the terms on the right–hand side of (118(118) $\begin{array}{rl}\frac{1}{2}\frac{\mathrm{d}}{\mathrm{d}t}{\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}|\frac{\mathrm{\partial }{\mathbf{\text{R}}}_{\epsilon }^{\ast }}{\mathrm{\partial }t}{|}_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}^2+\mu {\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}|\mathrm{\nabla }\frac{\mathrm{\partial }{\mathbf{\text{R}}}_{\epsilon }^{\ast }}{\mathrm{\partial }t}{|}_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}^2=& a{\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}\text{rot}(\frac{\mathrm{\partial }{\mathbf{\text{w}}}_{\epsilon }}{\mathrm{\partial }t}-\frac{\mathrm{\partial }{\mathbf{\text{w}}}_{\epsilon }^{\left[1\right]}}{\mathrm{\partial }t})\frac{\mathrm{\partial }{\mathbf{\text{R}}}_{\epsilon }^{\ast }}{\mathrm{\partial }t}-{\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}\frac{\mathrm{\partial }{\mathbf{\text{E}}}_{\epsilon }}{\mathrm{\partial }t}\frac{\mathrm{\partial }{\mathbf{\text{R}}}_{\epsilon }^{\ast }}{\mathrm{\partial }t}\\ & -{\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}\frac{{\mathrm{\partial }}^2{\mathit{\zeta }}_{\epsilon }}{\mathrm{\partial }{t}^2}\frac{\mathrm{\partial }{\mathbf{\text{R}}}_{\epsilon }^{\ast }}{\mathrm{\partial }t}-\alpha {\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}\mathrm{\nabla }\frac{\mathrm{\partial }{\mathit{\zeta }}_{\epsilon }}{\mathrm{\partial }t}\mathrm{\nabla }\frac{\mathrm{\partial }{\mathbf{\text{R}}}_{\epsilon }^{\ast }}{\mathrm{\partial }t}.\end{array}$(118) ), integrating with respect to t and using (110(110) $\underset{t\in [0,T]}{sup}||\frac{\mathrm{\partial }{\mathbf{\text{s}}}_{\epsilon }^{\ast }}{\mathrm{\partial }t}|{|}_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}^2+{\int }_0^T||\mathrm{\nabla }\frac{\mathrm{\partial }{\mathbf{\text{s}}}_{\epsilon }^{\ast }}{\mathrm{\partial }t}|{|}_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}^2\le C{\epsilon }^2{\int }_0^T||\mathrm{\nabla }(\frac{\mathrm{\partial }{\mathbf{\text{u}}}_{\epsilon }}{\mathrm{\partial }t}-\frac{\mathrm{\partial }{\mathbf{\text{u}}}_{\epsilon }^{\left[2\right]}}{\mathrm{\partial }t})|{|}_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}^2+C{\epsilon }^{10}.$(110) ), (113(113) $\parallel \mathrm{\nabla }{\mathit{\zeta }}_{\epsilon }{\parallel }_{L^2(0,T;L^2({\mathrm{\Omega }}_{\epsilon }^{\alpha }\left)\right)}+||\mathrm{\nabla }\frac{\mathrm{\partial }{\mathit{\zeta }}_{\epsilon }}{\mathrm{\partial }t}|{|}_{L^2(0,T;L^2({\mathrm{\Omega }}_{\epsilon }^{\alpha }\left)\right)}+||\mathrm{\nabla }\frac{{\mathrm{\partial }}^2{\mathit{\zeta }}_{\epsilon }}{\mathrm{\partial }{t}^2}|{|}_{L^2(0,T;L^2({\mathrm{\Omega }}_{\epsilon }^{\alpha }\left)\right)}\le C{\epsilon }^{7/2}.$(113) ), for sufficiently small ε we obtain: (119) $\underset{t\in [0,T]}{sup}||\frac{\mathrm{\partial }{\mathbf{\text{R}}}_{\epsilon }^{\ast }}{\mathrm{\partial }t}|{|}_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}^2+{\int }_0^T||\mathrm{\nabla }\frac{\mathrm{\partial }{\mathbf{\text{R}}}_{\epsilon }^{\ast }}{\mathrm{\partial }t}|{|}_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}^2\le C{\epsilon }^7.$(119) From (110(110) $\underset{t\in [0,T]}{sup}||\frac{\mathrm{\partial }{\mathbf{\text{s}}}_{\epsilon }^{\ast }}{\mathrm{\partial }t}|{|}_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}^2+{\int }_0^T||\mathrm{\nabla }\frac{\mathrm{\partial }{\mathbf{\text{s}}}_{\epsilon }^{\ast }}{\mathrm{\partial }t}|{|}_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}^2\le C{\epsilon }^2{\int }_0^T||\mathrm{\nabla }(\frac{\mathrm{\partial }{\mathbf{\text{u}}}_{\epsilon }}{\mathrm{\partial }t}-\frac{\mathrm{\partial }{\mathbf{\text{u}}}_{\epsilon }^{\left[2\right]}}{\mathrm{\partial }t})|{|}_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}^2+C{\epsilon }^{10}.$(110) ), it follows (120) $\underset{t\in [0,T]}{sup}||\frac{\mathrm{\partial }{\mathbf{\text{s}}}_{\epsilon }^{\ast }}{\mathrm{\partial }t}|{|}_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}^2+{\int }_0^T||\mathrm{\nabla }\frac{\mathrm{\partial }{\mathbf{\text{s}}}_{\epsilon }^{\ast }}{\mathrm{\partial }t}|{|}_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}^2\le C{\epsilon }^9,$(120) thus obtaining the estimates (102(102) $\begin{array}{rl}& \underset{t\in [0,T]}{sup}\parallel {\mathbf{u}}_{\epsilon }(t,\cdot )-{\mathbf{u}}_{\epsilon }^{\left[2\right]}(t,\cdot ){\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}+\parallel \mathrm{\nabla }({\mathbf{u}}_{\epsilon }-{\mathbf{u}}_{\epsilon }^{\left[2\right]})|{|}_{L^2(0,T;L^2({\mathrm{\Omega }}_{\epsilon }^{\alpha }\left)\right)}\le C{\epsilon }^{7/2},\\ & \underset{t\in [0,T]}{sup}||\frac{\mathrm{\partial }{\mathbf{u}}_{\epsilon }(t,\cdot )}{\mathrm{\partial }t}-\frac{\mathrm{\partial }{\mathbf{u}}_{\epsilon }^{\left[2\right]}(t,\cdot )}{\mathrm{\partial }t}|{|}_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}+||\mathrm{\nabla }(\frac{\mathrm{\partial }{\mathbf{u}}_{\epsilon }}{\mathrm{\partial }t}-\frac{\mathrm{\partial }{\mathbf{u}}_{\epsilon }^{\left[2\right]}}{\mathrm{\partial }t})|{|}_{L^2(0,T;L^2({\mathrm{\Omega }}_{\epsilon }^{\alpha }\left)\right)}\le C{\epsilon }^{7/2},\\ & \underset{t\in [0,T]}{sup}\parallel {\mathbf{w}}_{\epsilon }(t,\cdot )-{\mathbf{w}}_{\epsilon }^{\left[2\right]}(t,\cdot ){\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}+\parallel \mathrm{\nabla }({\mathbf{w}}_{\epsilon }-{\mathbf{w}}_{\epsilon }^{\left[2\right]})|{|}_{L^2(0,T;L^2({\mathrm{\Omega }}_{\epsilon }^{\alpha }\left)\right)}\le C{\epsilon }^{9/2},\\ & \underset{t\in [0,T]}{sup}||\frac{\mathrm{\partial }{\mathbf{w}}_{\epsilon }(t,\cdot )}{\mathrm{\partial }t}-\frac{\mathrm{\partial }{\mathbf{w}}_{\epsilon }^{\left[2\right]}(t,\cdot )}{\mathrm{\partial }t}|{|}_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}+||\mathrm{\nabla }(\frac{\mathrm{\partial }{\mathbf{w}}_{\epsilon }}{\mathrm{\partial }t}-\frac{\mathrm{\partial }{\mathbf{w}}_{\epsilon }^{\left[2\right]}}{\mathrm{\partial }t})|{|}_{L^2(0,T;L^2({\mathrm{\Omega }}_{\epsilon }^{\alpha }\left)\right)}\le C{\epsilon }^{9/2},\\ & \parallel p_{\epsilon }-p_{\epsilon }^{\left[2\right]}{\parallel }_{L^2(0,T;L^2({\mathrm{\Omega }}_{\epsilon }^{\alpha }\left)\right)}\le C{\epsilon }^{5/2},\end{array}$(102) )₂ and (102(102) $\begin{array}{rl}& \underset{t\in [0,T]}{sup}\parallel {\mathbf{u}}_{\epsilon }(t,\cdot )-{\mathbf{u}}_{\epsilon }^{\left[2\right]}(t,\cdot ){\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}+\parallel \mathrm{\nabla }({\mathbf{u}}_{\epsilon }-{\mathbf{u}}_{\epsilon }^{\left[2\right]})|{|}_{L^2(0,T;L^2({\mathrm{\Omega }}_{\epsilon }^{\alpha }\left)\right)}\le C{\epsilon }^{7/2},\\ & \underset{t\in [0,T]}{sup}||\frac{\mathrm{\partial }{\mathbf{u}}_{\epsilon }(t,\cdot )}{\mathrm{\partial }t}-\frac{\mathrm{\partial }{\mathbf{u}}_{\epsilon }^{\left[2\right]}(t,\cdot )}{\mathrm{\partial }t}|{|}_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}+||\mathrm{\nabla }(\frac{\mathrm{\partial }{\mathbf{u}}_{\epsilon }}{\mathrm{\partial }t}-\frac{\mathrm{\partial }{\mathbf{u}}_{\epsilon }^{\left[2\right]}}{\mathrm{\partial }t})|{|}_{L^2(0,T;L^2({\mathrm{\Omega }}_{\epsilon }^{\alpha }\left)\right)}\le C{\epsilon }^{7/2},\\ & \underset{t\in [0,T]}{sup}\parallel {\mathbf{w}}_{\epsilon }(t,\cdot )-{\mathbf{w}}_{\epsilon }^{\left[2\right]}(t,\cdot ){\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}+\parallel \mathrm{\nabla }({\mathbf{w}}_{\epsilon }-{\mathbf{w}}_{\epsilon }^{\left[2\right]})|{|}_{L^2(0,T;L^2({\mathrm{\Omega }}_{\epsilon }^{\alpha }\left)\right)}\le C{\epsilon }^{9/2},\\ & \underset{t\in [0,T]}{sup}||\frac{\mathrm{\partial }{\mathbf{w}}_{\epsilon }(t,\cdot )}{\mathrm{\partial }t}-\frac{\mathrm{\partial }{\mathbf{w}}_{\epsilon }^{\left[2\right]}(t,\cdot )}{\mathrm{\partial }t}|{|}_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}+||\mathrm{\nabla }(\frac{\mathrm{\partial }{\mathbf{w}}_{\epsilon }}{\mathrm{\partial }t}-\frac{\mathrm{\partial }{\mathbf{w}}_{\epsilon }^{\left[2\right]}}{\mathrm{\partial }t})|{|}_{L^2(0,T;L^2({\mathrm{\Omega }}_{\epsilon }^{\alpha }\left)\right)}\le C{\epsilon }^{9/2},\\ & \parallel p_{\epsilon }-p_{\epsilon }^{\left[2\right]}{\parallel }_{L^2(0,T;L^2({\mathrm{\Omega }}_{\epsilon }^{\alpha }\left)\right)}\le C{\epsilon }^{5/2},\end{array}$(102) )₄.

Let now ${\mathbf{\text{d}}}_{\epsilon }$ be the solution of the problem (121) $\begin{array}{rl}& \text{div}{\mathbf{\text{d}}}_{\epsilon }=r_{\epsilon }\text{in}{\mathrm{\Omega }}_{\epsilon }^{\alpha },\\ & {\mathbf{\text{d}}}_{\epsilon }=0\text{on}\mathrm{\partial }{\mathrm{\Omega }}_{\epsilon }^{\alpha }.\end{array}$(121) Supposing that ${\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}{r}_{\epsilon }=0$, for all t, it follows from Lemma (5.2) that the problem (121(121) $\begin{array}{rl}& \text{div}{\mathbf{\text{d}}}_{\epsilon }=r_{\epsilon }\text{in}{\mathrm{\Omega }}_{\epsilon }^{\alpha },\\ & {\mathbf{\text{d}}}_{\epsilon }=0\text{on}\mathrm{\partial }{\mathrm{\Omega }}_{\epsilon }^{\alpha }.\end{array}$(121) ) has at least one solution satisfying the estimate (122) $\parallel \mathrm{\nabla }{\mathbf{\text{d}}}_{\epsilon }{\parallel }_{L^2(0,T;L^2({\mathrm{\Omega }}_{\epsilon }^{\alpha }\left)\right)}\le \frac{C}{\epsilon }\parallel r_{\epsilon }{\parallel }_{L^2(0,T;L^2({\mathrm{\Omega }}_{\epsilon }^{\alpha }\left)\right)}.$(122) Multiplying the equation (112(112) $\begin{array}{rl}& \frac{\mathrm{\partial }{\mathbf{\text{R}}}_{\epsilon }}{\mathrm{\partial }t}-\mu \mathrm{\Delta }{\mathbf{\text{R}}}_{\epsilon }+\mathrm{\nabla }{r}_{\epsilon }=a\text{rot}({\mathbf{\text{w}}}_{\epsilon }-{\mathbf{\text{w}}}_{\epsilon }^{\left[1\right]})-{\mathbf{\text{E}}}_{\epsilon }\text{in}{\mathrm{\Omega }}_{\epsilon }^{\alpha },\\ & \text{div}{\mathbf{\text{R}}}_{\epsilon }=-{\mathit{\pi }}_{\epsilon }\text{in}{\mathrm{\Omega }}_{\epsilon }^{\alpha },\\ & {\mathbf{\text{R}}}_{\epsilon }=\mathbf{\text{0}}\text{on}{\mathrm{\Gamma }}_{\epsilon }^{\alpha },\\ & {\mathbf{\text{R}}}_{\epsilon }=-{\mathbf{\text{r}}}_0\text{on}{\mathrm{\Sigma }}_{\epsilon }^0{\mathbf{\text{R}}}_{\epsilon }=-{\mathbf{\text{r}}}_l\text{on}{\mathrm{\Sigma }}_{\epsilon }^l,\\ & {\mathbf{\text{R}}}_{\epsilon }(0,\cdot )=\mathbf{\text{0}}.\end{array}$(112) )₁ by ${\mathbf{\text{d}}}_{\epsilon }$ and integrating over ${\mathrm{\Omega }}_{\epsilon }^{\alpha }$ yields (123) $\begin{array}{rl}\parallel r_{\epsilon }{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}^2& ={\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}\frac{\mathrm{\partial }{\mathbf{\text{R}}}_{\epsilon }^{\ast }}{\mathrm{\partial }t}{\mathbf{\text{d}}}_{\epsilon }+\mu {\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}\mathrm{\nabla }{\mathbf{\text{R}}}_{\epsilon }^{\ast }\mathrm{\nabla }{\mathbf{\text{d}}}_{\epsilon }-a{\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}\text{rot}({\mathbf{\text{w}}}_{\epsilon }-{\mathbf{\text{w}}}_{\epsilon }^{\left[1\right]}){\mathbf{\text{d}}}_{\epsilon }+{\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}{\mathbf{\text{E}}}_{\epsilon }{\mathbf{\text{d}}}_{\epsilon }\\ & +{\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}\frac{\mathrm{\partial }{\mathit{\zeta }}_{\epsilon }}{\mathrm{\partial }t}{\mathbf{\text{d}}}_{\epsilon }+\mu {\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}\mathrm{\nabla }{\mathit{\zeta }}_{\epsilon }\mathrm{\nabla }{\mathbf{\text{d}}}_{\epsilon }.\end{array}$(123) Estimating the terms on the right–hand of (123(123) $\begin{array}{rl}\parallel r_{\epsilon }{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}^2& ={\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}\frac{\mathrm{\partial }{\mathbf{\text{R}}}_{\epsilon }^{\ast }}{\mathrm{\partial }t}{\mathbf{\text{d}}}_{\epsilon }+\mu {\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}\mathrm{\nabla }{\mathbf{\text{R}}}_{\epsilon }^{\ast }\mathrm{\nabla }{\mathbf{\text{d}}}_{\epsilon }-a{\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}\text{rot}({\mathbf{\text{w}}}_{\epsilon }-{\mathbf{\text{w}}}_{\epsilon }^{\left[1\right]}){\mathbf{\text{d}}}_{\epsilon }+{\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}{\mathbf{\text{E}}}_{\epsilon }{\mathbf{\text{d}}}_{\epsilon }\\ & +{\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}\frac{\mathrm{\partial }{\mathit{\zeta }}_{\epsilon }}{\mathrm{\partial }t}{\mathbf{\text{d}}}_{\epsilon }+\mu {\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}\mathrm{\nabla }{\mathit{\zeta }}_{\epsilon }\mathrm{\nabla }{\mathbf{\text{d}}}_{\epsilon }.\end{array}$(123) ) and using Poincaré's inequality yields: (124) $\begin{array}{rl}& |{\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}\frac{\mathrm{\partial }{\mathbf{\text{R}}}_{\epsilon }^{\ast }}{\mathrm{\partial }t}{\mathbf{\text{d}}}_{\epsilon }|\le ||\frac{\mathrm{\partial }{\mathbf{\text{R}}}_{\epsilon }^{\ast }}{\mathrm{\partial }t}|{|}_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}\parallel {\mathbf{\text{d}}}_{\epsilon }{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}\le C{\epsilon }^2||\mathrm{\nabla }\frac{\mathrm{\partial }{\mathbf{\text{R}}}_{\epsilon }^{\ast }}{\mathrm{\partial }t}|{|}_{L^2\left({\mathrm{\Omega }}_{\epsilon }\right)}\parallel \mathrm{\nabla }{\mathbf{\text{d}}}_{\epsilon }{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)},\\ & |{\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}\mathrm{\nabla }{\mathbf{\text{R}}}_{\epsilon }^{\ast }\mathrm{\nabla }{\mathbf{\text{d}}}_{\epsilon }|\le \parallel \mathrm{\nabla }{\mathbf{\text{R}}}_{\epsilon }^{\ast }{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}\parallel \mathrm{\nabla }{\mathbf{\text{d}}}_{\epsilon }{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)},\\ & |{\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}\text{rot}({\mathbf{\text{w}}}_{\epsilon }-{\mathbf{\text{w}}}_{\epsilon }^{\left[1\right]}){\mathbf{\text{d}}}_{\epsilon }|\le \epsilon \parallel \mathrm{\nabla }({\mathbf{\text{w}}}_{\epsilon }-{\mathbf{\text{w}}}_{\epsilon }^{\left[1\right]}){\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}\parallel \mathrm{\nabla }{\mathbf{\text{d}}}_{\epsilon }{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}\\ & \le C\epsilon (\parallel \mathrm{\nabla }({\mathbf{\text{w}}}_{\epsilon }-{\mathbf{\text{w}}}_{\epsilon }^{\left[2\right]}){\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}+\parallel \mathrm{\nabla }({\mathbf{\text{w}}}_{\epsilon }^{\left[2\right]}-{\mathbf{\text{w}}}_{\epsilon }^{\left[1\right]}){\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)})\parallel \mathrm{\nabla }{\mathbf{\text{d}}}_{\epsilon }{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}\\ & \le C\epsilon \parallel \mathrm{\nabla }{\mathbf{\text{s}}}_{\epsilon }^{\ast }{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}\parallel \mathrm{\nabla }{\mathbf{\text{d}}}_{\epsilon }{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}+C{\epsilon }^5\parallel \mathrm{\nabla }{\mathbf{\text{d}}}_{\epsilon }{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)},\\ & |{\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}{\mathbf{\text{E}}}_{\epsilon }{\mathbf{\text{d}}}_{\epsilon }|\le \parallel {\mathbf{\text{E}}}_{\epsilon }{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}\parallel {\mathbf{\text{d}}}_{\epsilon }{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}\le C\epsilon \parallel {\mathbf{\text{E}}}_{\epsilon }{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}\parallel \mathrm{\nabla }{\mathbf{\text{d}}}_{\epsilon }{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)},\\ & |{\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}\frac{\mathrm{\partial }{\mathit{\zeta }}_{\epsilon }}{\mathrm{\partial }t}{\mathbf{\text{d}}}_{\epsilon }|\le ||\frac{\mathrm{\partial }{\mathit{\zeta }}_{\epsilon }}{\mathrm{\partial }t}|{|}_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}\parallel {\mathbf{\text{d}}}_{\epsilon }{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}\le C{\epsilon }^2||\frac{\mathrm{\partial \nabla }{\mathit{\zeta }}_{\epsilon }}{\mathrm{\partial }t}|{|}_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}\parallel \mathrm{\nabla }{\mathbf{\text{d}}}_{\epsilon }{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)},\\ & |{\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}\mathrm{\nabla }{\mathit{\zeta }}_{\epsilon }\mathrm{\nabla }{\mathbf{\text{d}}}_{\epsilon }|\le \parallel \mathrm{\nabla }{\mathit{\zeta }}_{\epsilon }{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}\parallel \mathrm{\nabla }\mathbf{\text{d}}{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}.\end{array}$(124) Applying (124(124) $\begin{array}{rl}& |{\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}\frac{\mathrm{\partial }{\mathbf{\text{R}}}_{\epsilon }^{\ast }}{\mathrm{\partial }t}{\mathbf{\text{d}}}_{\epsilon }|\le ||\frac{\mathrm{\partial }{\mathbf{\text{R}}}_{\epsilon }^{\ast }}{\mathrm{\partial }t}|{|}_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}\parallel {\mathbf{\text{d}}}_{\epsilon }{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}\le C{\epsilon }^2||\mathrm{\nabla }\frac{\mathrm{\partial }{\mathbf{\text{R}}}_{\epsilon }^{\ast }}{\mathrm{\partial }t}|{|}_{L^2\left({\mathrm{\Omega }}_{\epsilon }\right)}\parallel \mathrm{\nabla }{\mathbf{\text{d}}}_{\epsilon }{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)},\\ & |{\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}\mathrm{\nabla }{\mathbf{\text{R}}}_{\epsilon }^{\ast }\mathrm{\nabla }{\mathbf{\text{d}}}_{\epsilon }|\le \parallel \mathrm{\nabla }{\mathbf{\text{R}}}_{\epsilon }^{\ast }{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}\parallel \mathrm{\nabla }{\mathbf{\text{d}}}_{\epsilon }{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)},\\ & |{\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}\text{rot}({\mathbf{\text{w}}}_{\epsilon }-{\mathbf{\text{w}}}_{\epsilon }^{\left[1\right]}){\mathbf{\text{d}}}_{\epsilon }|\le \epsilon \parallel \mathrm{\nabla }({\mathbf{\text{w}}}_{\epsilon }-{\mathbf{\text{w}}}_{\epsilon }^{\left[1\right]}){\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}\parallel \mathrm{\nabla }{\mathbf{\text{d}}}_{\epsilon }{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}\\ & \le C\epsilon (\parallel \mathrm{\nabla }({\mathbf{\text{w}}}_{\epsilon }-{\mathbf{\text{w}}}_{\epsilon }^{\left[2\right]}){\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}+\parallel \mathrm{\nabla }({\mathbf{\text{w}}}_{\epsilon }^{\left[2\right]}-{\mathbf{\text{w}}}_{\epsilon }^{\left[1\right]}){\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)})\parallel \mathrm{\nabla }{\mathbf{\text{d}}}_{\epsilon }{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}\\ & \le C\epsilon \parallel \mathrm{\nabla }{\mathbf{\text{s}}}_{\epsilon }^{\ast }{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}\parallel \mathrm{\nabla }{\mathbf{\text{d}}}_{\epsilon }{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}+C{\epsilon }^5\parallel \mathrm{\nabla }{\mathbf{\text{d}}}_{\epsilon }{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)},\\ & |{\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}{\mathbf{\text{E}}}_{\epsilon }{\mathbf{\text{d}}}_{\epsilon }|\le \parallel {\mathbf{\text{E}}}_{\epsilon }{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}\parallel {\mathbf{\text{d}}}_{\epsilon }{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}\le C\epsilon \parallel {\mathbf{\text{E}}}_{\epsilon }{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}\parallel \mathrm{\nabla }{\mathbf{\text{d}}}_{\epsilon }{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)},\\ & |{\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}\frac{\mathrm{\partial }{\mathit{\zeta }}_{\epsilon }}{\mathrm{\partial }t}{\mathbf{\text{d}}}_{\epsilon }|\le ||\frac{\mathrm{\partial }{\mathit{\zeta }}_{\epsilon }}{\mathrm{\partial }t}|{|}_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}\parallel {\mathbf{\text{d}}}_{\epsilon }{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}\le C{\epsilon }^2||\frac{\mathrm{\partial \nabla }{\mathit{\zeta }}_{\epsilon }}{\mathrm{\partial }t}|{|}_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}\parallel \mathrm{\nabla }{\mathbf{\text{d}}}_{\epsilon }{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)},\\ & |{\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}\mathrm{\nabla }{\mathit{\zeta }}_{\epsilon }\mathrm{\nabla }{\mathbf{\text{d}}}_{\epsilon }|\le \parallel \mathrm{\nabla }{\mathit{\zeta }}_{\epsilon }{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}\parallel \mathrm{\nabla }\mathbf{\text{d}}{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}.\end{array}$(124) ) and Young's inequality on the right–hand side of (123(123) $\begin{array}{rl}\parallel r_{\epsilon }{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}^2& ={\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}\frac{\mathrm{\partial }{\mathbf{\text{R}}}_{\epsilon }^{\ast }}{\mathrm{\partial }t}{\mathbf{\text{d}}}_{\epsilon }+\mu {\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}\mathrm{\nabla }{\mathbf{\text{R}}}_{\epsilon }^{\ast }\mathrm{\nabla }{\mathbf{\text{d}}}_{\epsilon }-a{\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}\text{rot}({\mathbf{\text{w}}}_{\epsilon }-{\mathbf{\text{w}}}_{\epsilon }^{\left[1\right]}){\mathbf{\text{d}}}_{\epsilon }+{\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}{\mathbf{\text{E}}}_{\epsilon }{\mathbf{\text{d}}}_{\epsilon }\\ & +{\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}\frac{\mathrm{\partial }{\mathit{\zeta }}_{\epsilon }}{\mathrm{\partial }t}{\mathbf{\text{d}}}_{\epsilon }+\mu {\int }_{{\mathrm{\Omega }}_{\epsilon }^{\alpha }}\mathrm{\nabla }{\mathit{\zeta }}_{\epsilon }\mathrm{\nabla }{\mathbf{\text{d}}}_{\epsilon }.\end{array}$(123) ), integrating over t and using (113(113) $\parallel \mathrm{\nabla }{\mathit{\zeta }}_{\epsilon }{\parallel }_{L^2(0,T;L^2({\mathrm{\Omega }}_{\epsilon }^{\alpha }\left)\right)}+||\mathrm{\nabla }\frac{\mathrm{\partial }{\mathit{\zeta }}_{\epsilon }}{\mathrm{\partial }t}|{|}_{L^2(0,T;L^2({\mathrm{\Omega }}_{\epsilon }^{\alpha }\left)\right)}+||\mathrm{\nabla }\frac{{\mathrm{\partial }}^2{\mathit{\zeta }}_{\epsilon }}{\mathrm{\partial }{t}^2}|{|}_{L^2(0,T;L^2({\mathrm{\Omega }}_{\epsilon }^{\alpha }\left)\right)}\le C{\epsilon }^{7/2}.$(113) ), (116(116) $\underset{t\in [0,T]}{sup}\parallel {\mathbf{\text{R}}}_{\epsilon }^{\ast }{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}^2+{\int }_0^T\parallel \mathrm{\nabla }{\mathbf{\text{R}}}_{\epsilon }^{\ast }{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}^2\le C{\epsilon }^7,$(116) ), (117(117) $\underset{t\in [0,T]}{sup}\parallel {\mathbf{\text{s}}}_{\epsilon }^{\ast }{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}^2+{\int }_0^T\parallel \mathrm{\nabla }{\mathbf{\text{s}}}_{\epsilon }^{\ast }{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}^2\le C{\epsilon }^9,$(117) ), (119(119) $\underset{t\in [0,T]}{sup}||\frac{\mathrm{\partial }{\mathbf{\text{R}}}_{\epsilon }^{\ast }}{\mathrm{\partial }t}|{|}_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}^2+{\int }_0^T||\mathrm{\nabla }\frac{\mathrm{\partial }{\mathbf{\text{R}}}_{\epsilon }^{\ast }}{\mathrm{\partial }t}|{|}_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}^2\le C{\epsilon }^7.$(119) ) and (122(122) $\parallel \mathrm{\nabla }{\mathbf{\text{d}}}_{\epsilon }{\parallel }_{L^2(0,T;L^2({\mathrm{\Omega }}_{\epsilon }^{\alpha }\left)\right)}\le \frac{C}{\epsilon }\parallel r_{\epsilon }{\parallel }_{L^2(0,T;L^2({\mathrm{\Omega }}_{\epsilon }^{\alpha }\left)\right)}.$(122) ), we finally obtain: (125) $\parallel r_{\epsilon }{\parallel }_{L^2(0,T;L^2({\mathrm{\Omega }}_{\epsilon }^{\alpha }\left)\right)}\le C{\epsilon }^{5/2},$(125) completing the proof.

Remark 5.6

If we assume κ and α to be constant, due to consideration in Section 4.3 (see (73(73) ${\mathit{\pi }}_{\epsilon }={\mathit{\pi }}_{\epsilon ,3}+{\mathit{\pi }}_{\epsilon ,res}.$(73) )), following the proof of Theorem 5.5, we can improve the estimates (102(102) $\begin{array}{rl}& \underset{t\in [0,T]}{sup}\parallel {\mathbf{u}}_{\epsilon }(t,\cdot )-{\mathbf{u}}_{\epsilon }^{\left[2\right]}(t,\cdot ){\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}+\parallel \mathrm{\nabla }({\mathbf{u}}_{\epsilon }-{\mathbf{u}}_{\epsilon }^{\left[2\right]})|{|}_{L^2(0,T;L^2({\mathrm{\Omega }}_{\epsilon }^{\alpha }\left)\right)}\le C{\epsilon }^{7/2},\\ & \underset{t\in [0,T]}{sup}||\frac{\mathrm{\partial }{\mathbf{u}}_{\epsilon }(t,\cdot )}{\mathrm{\partial }t}-\frac{\mathrm{\partial }{\mathbf{u}}_{\epsilon }^{\left[2\right]}(t,\cdot )}{\mathrm{\partial }t}|{|}_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}+||\mathrm{\nabla }(\frac{\mathrm{\partial }{\mathbf{u}}_{\epsilon }}{\mathrm{\partial }t}-\frac{\mathrm{\partial }{\mathbf{u}}_{\epsilon }^{\left[2\right]}}{\mathrm{\partial }t})|{|}_{L^2(0,T;L^2({\mathrm{\Omega }}_{\epsilon }^{\alpha }\left)\right)}\le C{\epsilon }^{7/2},\\ & \underset{t\in [0,T]}{sup}\parallel {\mathbf{w}}_{\epsilon }(t,\cdot )-{\mathbf{w}}_{\epsilon }^{\left[2\right]}(t,\cdot ){\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}+\parallel \mathrm{\nabla }({\mathbf{w}}_{\epsilon }-{\mathbf{w}}_{\epsilon }^{\left[2\right]})|{|}_{L^2(0,T;L^2({\mathrm{\Omega }}_{\epsilon }^{\alpha }\left)\right)}\le C{\epsilon }^{9/2},\\ & \underset{t\in [0,T]}{sup}||\frac{\mathrm{\partial }{\mathbf{w}}_{\epsilon }(t,\cdot )}{\mathrm{\partial }t}-\frac{\mathrm{\partial }{\mathbf{w}}_{\epsilon }^{\left[2\right]}(t,\cdot )}{\mathrm{\partial }t}|{|}_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}+||\mathrm{\nabla }(\frac{\mathrm{\partial }{\mathbf{w}}_{\epsilon }}{\mathrm{\partial }t}-\frac{\mathrm{\partial }{\mathbf{w}}_{\epsilon }^{\left[2\right]}}{\mathrm{\partial }t})|{|}_{L^2(0,T;L^2({\mathrm{\Omega }}_{\epsilon }^{\alpha }\left)\right)}\le C{\epsilon }^{9/2},\\ & \parallel p_{\epsilon }-p_{\epsilon }^{\left[2\right]}{\parallel }_{L^2(0,T;L^2({\mathrm{\Omega }}_{\epsilon }^{\alpha }\left)\right)}\le C{\epsilon }^{5/2},\end{array}$(102) ) to obtain: $\begin{array}{rl}& \underset{t\in [0,T]}{sup}\parallel {\mathbf{\text{u}}}_{\epsilon }(t,\cdot )-{\mathbf{\text{u}}}_{\epsilon }^{\left[2\right]}(t,\cdot ){\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}+\parallel \mathrm{\nabla }({\mathbf{\text{u}}}_{\epsilon }-{\mathbf{\text{u}}}_{\epsilon }^{\left[2\right]})|{|}_{L^2(0,T;L^2({\mathrm{\Omega }}_{\epsilon }^{\alpha }\left)\right)}\le C{\epsilon }^4,\\ & \underset{t\in [0,T]}{sup}||\frac{\mathrm{\partial }{\mathbf{\text{u}}}_{\epsilon }(t,\cdot )}{\mathrm{\partial }t}-\frac{\mathrm{\partial }{\mathbf{\text{u}}}_{\epsilon }^{\left[2\right]}(t,\cdot )}{\mathrm{\partial }t}|{|}_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}+||\mathrm{\nabla }(\frac{\mathrm{\partial }{\mathbf{\text{u}}}_{\epsilon }}{\mathrm{\partial }t}-\frac{\mathrm{\partial }{\mathbf{\text{u}}}_{\epsilon }^{\left[2\right]}}{\mathrm{\partial }t})|{|}_{L^2(0,T;L^2({\mathrm{\Omega }}_{\epsilon }^{\alpha }\left)\right)}\le C{\epsilon }^4,\\ & \underset{t\in [0,T]}{sup}\parallel {\mathbf{\text{w}}}_{\epsilon }(t,\cdot )-{\mathbf{\text{w}}}_{\epsilon }^{\left[2\right]}(t,\cdot ){\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}+\parallel \mathrm{\nabla }({\mathbf{\text{w}}}_{\epsilon }-{\mathbf{\text{w}}}_{\epsilon }^{\left[2\right]})|{|}_{L^2(0,T;L^2({\mathrm{\Omega }}_{\epsilon }^{\alpha }\left)\right)}\le C{\epsilon }^5,\\ & \underset{t\in [0,T]}{sup}||\frac{\mathrm{\partial }{\mathbf{\text{w}}}_{\epsilon }(t,\cdot )}{\mathrm{\partial }t}-\frac{\mathrm{\partial }{\mathbf{\text{w}}}_{\epsilon }^{\left[2\right]}(t,\cdot )}{\mathrm{\partial }t}|{|}_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}+||\mathrm{\nabla }(\frac{\mathrm{\partial }{\mathbf{\text{w}}}_{\epsilon }}{\mathrm{\partial }t}-\frac{\mathrm{\partial }{\mathbf{\text{w}}}_{\epsilon }^{\left[2\right]}}{\mathrm{\partial }t})|{|}_{L^2(0,T;L^2({\mathrm{\Omega }}_{\epsilon }^{\alpha }\left)\right)}\le C{\epsilon }^5,\\ & \parallel p_{\epsilon }-p_{\epsilon }^{\left[2\right]}{\parallel }_{L^2(0,T;L^2({\mathrm{\Omega }}_{\epsilon }^{\alpha }\left)\right)}\le C{\epsilon }^3.\end{array}$ If we, additionally, assume that $\mathbf{\text{f}},\mathbf{\text{g}}$ vanish in the neighbourhood of $x_1=0$ and $x_1=l$, ${\int }_0^l\mathbf{\text{g}}=0$ and take $P_2(x_1,t)=x_1{p}_{bl}\left(t\right)+p_{div}(x_1,t)$, following the procedure given in Section 4.3 (see (75(75) $\begin{array}{rl}{\mathit{\pi }}_{\epsilon ,3}& =\frac{\mathrm{\partial }{V}_2^1}{\mathrm{\partial }{x}_1}-\kappa (V_2^2\cos \alpha -V_2^3\sin \alpha )+{\kappa }^2({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast })(\cos \alpha V_1^2-\sin \alpha V_1^3)\\ & +2\kappa ({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast })(\frac{\mathrm{\partial }{V}_1^1}{\mathrm{\partial }{x}_1}-\kappa (V_1^2\cos \alpha -V_1^3\sin \alpha )),\end{array}$(75) )–(79(79) $\text{div}{\widetilde{\mathbf{\text{u}}}}_{\epsilon }^{\left[2\right]}={\tilde{\mathit{\pi }}}_{\epsilon },\parallel {\tilde{\mathit{\pi }}}_{\epsilon }{\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}=\mathcal{O}\left({\epsilon }^{11/2}\right).$(79) )), we can even further improve the error estimates. Namely, we get: $\begin{array}{rl}& \underset{t\in [0,T]}{sup}\parallel {\mathbf{\text{u}}}_{\epsilon }(t,\cdot )-{\mathbf{\text{u}}}_{\epsilon }^{\left[2\right]}(t,\cdot ){\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}+\parallel \mathrm{\nabla }({\mathbf{\text{u}}}_{\epsilon }-{\mathbf{\text{u}}}_{\epsilon }^{\left[2\right]})|{|}_{L^2(0,T;L^2({\mathrm{\Omega }}_{\epsilon }^{\alpha }\left)\right)}\le C{\epsilon }^{9/2},\\ & \underset{t\in [0,T]}{sup}||\frac{\mathrm{\partial }{\mathbf{\text{u}}}_{\epsilon }(t,\cdot )}{\mathrm{\partial }t}-\frac{\mathrm{\partial }{\mathbf{\text{u}}}_{\epsilon }^{\left[2\right]}(t,\cdot )}{\mathrm{\partial }t}|{|}_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}+||\mathrm{\nabla }(\frac{\mathrm{\partial }{\mathbf{\text{u}}}_{\epsilon }}{\mathrm{\partial }t}-\frac{\mathrm{\partial }{\mathbf{\text{u}}}_{\epsilon }^{\left[2\right]}}{\mathrm{\partial }t})|{|}_{L^2(0,T;L^2({\mathrm{\Omega }}_{\epsilon }^{\alpha }\left)\right)}\le C{\epsilon }^{9/2},\\ & \underset{t\in [0,T]}{sup}\parallel {\mathbf{\text{w}}}_{\epsilon }(t,\cdot )-{\mathbf{\text{w}}}_{\epsilon }^{\left[2\right]}(t,\cdot ){\parallel }_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}+\parallel \mathrm{\nabla }({\mathbf{\text{w}}}_{\epsilon }-{\mathbf{\text{w}}}_{\epsilon }^{\left[2\right]})|{|}_{L^2(0,T;L^2({\mathrm{\Omega }}_{\epsilon }^{\alpha }\left)\right)}\le C{\epsilon }^5,\\ & \underset{t\in [0,T]}{sup}||\frac{\mathrm{\partial }{\mathbf{\text{w}}}_{\epsilon }(t,\cdot )}{\mathrm{\partial }t}-\frac{\mathrm{\partial }{\mathbf{\text{w}}}_{\epsilon }^{\left[2\right]}(t,\cdot )}{\mathrm{\partial }t}|{|}_{L^2\left({\mathrm{\Omega }}_{\epsilon }^{\alpha }\right)}+||\mathrm{\nabla }(\frac{\mathrm{\partial }{\mathbf{\text{w}}}_{\epsilon }}{\mathrm{\partial }t}-\frac{\mathrm{\partial }{\mathbf{\text{w}}}_{\epsilon }^{\left[2\right]}}{\mathrm{\partial }t})|{|}_{L^2(0,T;L^2({\mathrm{\Omega }}_{\epsilon }^{\alpha }\left)\right)}\le C{\epsilon }^5,\\ & \parallel p_{\epsilon }-p_{\epsilon }^{\left[2\right]}{\parallel }_{L^2(0,T;L^2({\mathrm{\Omega }}_{\epsilon }^{\alpha }\left)\right)}\le C{\epsilon }^{7/2}.\end{array}$

Acknowledgments

The authors of this work have been supported by the Croatian Science Foundation (scientific project: Asymptotic analysis of the boundary value problems in continuum mechanics). The authors are grateful to the Referees for their valuable remarks and comments that helped to correct and improve the paper.

Disclosure statement

No potential conflict of interest was reported by the authors.

Appendix

Regular part of the expansion

In the following, we write down the functions (depending on t and $x_1$) appearing in the computations for the first- and second-order correctors (see (26(26) $\begin{array}{rl}& -\mu {\mathrm{\Delta }}_{{\mathit{y}}_{\ast }}{V}_1^1=y_2{H}_1(t,x_1)+y_3{H}_2(t,x_2)\text{in}\mathrm{\Omega },\\ & V_1^1=0\text{on}\mathrm{\Gamma },\end{array}$(26) ), (32(32) $\begin{array}{rl}& -\delta {\mathrm{\Delta }}_{{\mathit{y}}_{\ast }}{W}_1^1=y_2{H}_3(t,x_1)+y_3{H}_4(t,x_1)\text{in}\mathrm{\Omega },\\ & W_1^1=0\text{on}\mathrm{\Gamma },\end{array}$(32) ), (35(35) $\begin{array}{rl}& -\delta {\mathrm{\Delta }}_{{\mathit{y}}_{\ast }}{W}_1^2-\beta (\frac{{\mathrm{\partial }}^2{W}_1^2}{\mathrm{\partial }{y}_2^2}+\frac{{\mathrm{\partial }}^2{W}_1^3}{\mathrm{\partial }{y}_2\mathrm{\partial }{y}_3})=y_2{H}_5+y_6{H}_6\text{in}\mathrm{\Omega },\\ & -\delta {\mathrm{\Delta }}_{{\mathit{y}}_{\ast }}{W}_1^3-\beta (\frac{\mathrm{\partial }{W}_1^3}{\mathrm{\partial }{y}_3^2}+\frac{\mathrm{\partial }{W}_1^2}{\mathrm{\partial }{y}_2\mathrm{\partial }{y}_3})=y_2{H}_7+y_3{H}_8\text{in}\mathrm{\Omega },\\ & W_1^2=W_1^3=0\text{on}\mathrm{\Gamma },\end{array}$(35) ), (36(36) $\begin{array}{rl}& W_1^2(t,x_1,{\mathit{y}}_{\ast })=(1-|{\mathit{y}}_{\ast }{|}^2\left)\right(y_2{H}_9(t,x_1)+y_3{H}_{10}(t,x_1)),\\ & W_1^3(t,x_1,{\mathit{y}}_{\ast })=(1-|{\mathit{y}}_{\ast }{|}^2\left)\right(y_2{H}_{11}(t,x_1)+y_3{H}_{12}(t,x_1)),\end{array}$(36) ), (38(38) $\begin{array}{rl}& {\mathrm{\Delta }}_{{\mathit{y}}_{\ast }}{V}_2^1=A_1{y}_2^2+A_2{y}_2{y}_3+A_3{y}_3^2+A_4\text{in}\mathrm{\Omega },\\ & V_2^1=0\text{on}\mathrm{\Gamma },\end{array}$(38) ), (40(40) $\begin{array}{rl}& -\mu {\mathrm{\Delta }}_{{\mathit{y}}_{\ast }}{V}_2^2+\frac{\mathrm{\partial }{P}_3}{\mathrm{\partial }{y}_2}=A_5{y}_2^2+A_6{y}_2{y}_3+A_7{y}_3^2+A_8,\\ & -\mu {\mathrm{\Delta }}_{{\mathit{y}}_{\ast }}{V}_2^3+\frac{\mathrm{\partial }{P}_3}{\mathrm{\partial }{y}_3}=A_9{y}_2^2+A_{10}{y}_2{y}_3+A_{11}{y}_3^2+A_{12},\\ & \frac{\mathrm{\partial }{V}_2^2}{\mathrm{\partial }{y}_2}+\frac{\mathrm{\partial }{V}_2^3}{\mathrm{\partial }{y}_3}=A_{13}{y}_2^3+A_{14}{y}_3^3+A_{13}{y}_2{y}_3^2+A_{14}{y}_2^2{y}_3-A_{13}{y}_2-A_{14}{y}_3,\\ & V_2^2=V_2^3=0\text{on}\mathrm{\Gamma },\end{array}$(40) ), (41(41) $\begin{array}{rl}& V_2^2(t,x_1,{\mathit{y}}_{\ast })=(1-|{\mathit{y}}_{\ast }{|}^2\left)\right(B_5{y}_2^2+B_6{y}_2{y}_3+B_7{y}_2^3+B_8),\\ & V_2^3(t,x_1,{\mathit{y}}_{\ast })=(1-|{\mathit{y}}_{\ast }{|}^2\left)\right(B_9{y}_2^2+B_{10}{y}_2{y}_3+B_{11}{y}_2^3+B_{12}),\\ & P_3(t,x_1,{\mathit{y}}_{\ast })=M_1{y}_2^3+M_2{y}_3^3+M_3{y}_2{y}_3^2+M_4{y}_2^2{y}_3+M_5{y}_2+M_6{y}_3.\end{array}$(41) ), (43(43) $\begin{array}{rl}& {\mathrm{\Delta }}_{{\mathit{y}}_{\ast }}{W}_2^1=C_1{y}_2^2+C_2{y}_2{y}_3+C_3{y}_3^2+C_4\text{in}\mathrm{\Omega },\\ & W_2^1=0\text{on}\mathrm{\Gamma },\end{array}$(43) ), (47(47) $\begin{array}{rl}& -\delta (\frac{{\mathrm{\partial }}^2{W}_2^2}{\mathrm{\partial }{y}_2^2}+\frac{\mathrm{\partial }{W}_2^2}{\mathrm{\partial }{y}_3^2})-\beta (\frac{{\mathrm{\partial }}^2{W}_2^2}{\mathrm{\partial }{y}_2^2}+\frac{\mathrm{\partial }{W}_2^3}{\mathrm{\partial }{y}_2\mathrm{\partial }{y}_3})=C_5{y}_2^2+C_6{y}_2{y}_3+C_7{y}_3^2+C_8\text{in}\mathrm{\Omega },\\ & -\delta (\frac{{\mathrm{\partial }}^2{W}_2^3}{\mathrm{\partial }{y}_2^2}+\frac{{\mathrm{\partial }}^2{W}_2^3}{\mathrm{\partial }{y}_3^2})-\beta (\frac{{\mathrm{\partial }}^2{W}_2^2}{\mathrm{\partial }{y}_2\mathrm{\partial }{y}_3}+\frac{{\mathrm{\partial }}^2{W}_2^3}{\mathrm{\partial }{y}_3^2})=C_9{y}_2^2+C_{10}{y}_2{y}_3+C_{11}{y}_3^2+C_{12}\text{in}\mathrm{\Omega },\\ W_2^2& =W_2^3=0\text{on}\mathrm{\Gamma }.\end{array}$(47) ) and (48(47) $\begin{array}{rl}& -\delta (\frac{{\mathrm{\partial }}^2{W}_2^2}{\mathrm{\partial }{y}_2^2}+\frac{\mathrm{\partial }{W}_2^2}{\mathrm{\partial }{y}_3^2})-\beta (\frac{{\mathrm{\partial }}^2{W}_2^2}{\mathrm{\partial }{y}_2^2}+\frac{\mathrm{\partial }{W}_2^3}{\mathrm{\partial }{y}_2\mathrm{\partial }{y}_3})=C_5{y}_2^2+C_6{y}_2{y}_3+C_7{y}_3^2+C_8\text{in}\mathrm{\Omega },\\ & -\delta (\frac{{\mathrm{\partial }}^2{W}_2^3}{\mathrm{\partial }{y}_2^2}+\frac{{\mathrm{\partial }}^2{W}_2^3}{\mathrm{\partial }{y}_3^2})-\beta (\frac{{\mathrm{\partial }}^2{W}_2^2}{\mathrm{\partial }{y}_2\mathrm{\partial }{y}_3}+\frac{{\mathrm{\partial }}^2{W}_2^3}{\mathrm{\partial }{y}_3^2})=C_9{y}_2^2+C_{10}{y}_2{y}_3+C_{11}{y}_3^2+C_{12}\text{in}\mathrm{\Omega },\\ W_2^2& =W_2^3=0\text{on}\mathrm{\Gamma }.\end{array}$(47) )).

First-order corrector

The functions $H_1(t,x_1)$ and $H_2(t,x_1)$ in the problem (26(26) $\begin{array}{rl}& -\mu {\mathrm{\Delta }}_{{\mathit{y}}_{\ast }}{V}_1^1=y_2{H}_1(t,x_1)+y_3{H}_2(t,x_2)\text{in}\mathrm{\Omega },\\ & V_1^1=0\text{on}\mathrm{\Gamma },\end{array}$(26) ) for the first component of the velocity first-order corrector $V_1^1$ are given by: (A1) $\begin{array}{rl}{H}_1(t,x_1)& =\cos \alpha (-\frac{4\mu \kappa }{\pi }{F}_0^{\ast }-\kappa f_1-\frac{\mathrm{\partial }{f}_2}{\mathrm{\partial }{x}_1}+\tau f_3-\frac{a}{2\delta +\beta }{g}_3)+\sin \alpha (-\tau f_2-\frac{\mathrm{\partial }{f}_3}{\mathrm{\partial }{x}_1}+\frac{a}{2\delta +\beta }{g}_2),\\ H_2(t,x_1)& =\sin \alpha (\frac{4\mu \kappa }{\pi }{F}_0^{\ast }+\kappa f_1+\frac{\mathrm{\partial }{f}_2}{\mathrm{\partial }{x}_1}-\tau f_3-\frac{a}{2\delta +\beta }{g}_3)-\cos \alpha (\tau f_2+\frac{\mathrm{\partial }{f}_3}{\mathrm{\partial }{x}_1}+\frac{a}{2\delta +\beta }{g}_2).\end{array}$(A1) The functions $H_3(t,x_1)$ and $H_4(t,x_1)$ in the problem (32(32) $\begin{array}{rl}& -\delta {\mathrm{\Delta }}_{{\mathit{y}}_{\ast }}{W}_1^1=y_2{H}_3(t,x_1)+y_3{H}_4(t,x_1)\text{in}\mathrm{\Omega },\\ & W_1^1=0\text{on}\mathrm{\Gamma },\end{array}$(32) ) for the first component of the microrotation first-order corrector $W_1^1$ are given by: (A2) $\begin{array}{rl}{H}_3(t,x_1)& =-\frac{3\kappa }{2}{g}_1\cos \alpha +\frac{\beta }{2\delta +\beta }((\tau g_3-\frac{\mathrm{\partial }{g}_2}{\mathrm{\partial }{x}_1})\cos \alpha -(\tau g_2+\frac{\mathrm{\partial }{g}_3}{\mathrm{\partial }{x}_1})\sin \alpha ),\\ H_4(t,x_1)& =\frac{3\kappa }{2}{g}_1\sin \alpha -\frac{\beta }{2\delta +\beta }((\tau g_2+\frac{\mathrm{\partial }{g}_3}{\mathrm{\partial }{x}_1})\cos \alpha +(\tau g_3-\frac{\mathrm{\partial }{g}_2}{\mathrm{\partial }{x}_1})\sin \alpha ).\end{array}$(A2) The functions $H_5(t,x_1),\dots ,H_8(t,x_1)$ in the problem (35(35) $\begin{array}{rl}& -\delta {\mathrm{\Delta }}_{{\mathit{y}}_{\ast }}{W}_1^2-\beta (\frac{{\mathrm{\partial }}^2{W}_1^2}{\mathrm{\partial }{y}_2^2}+\frac{{\mathrm{\partial }}^2{W}_1^3}{\mathrm{\partial }{y}_2\mathrm{\partial }{y}_3})=y_2{H}_5+y_6{H}_6\text{in}\mathrm{\Omega },\\ & -\delta {\mathrm{\Delta }}_{{\mathit{y}}_{\ast }}{W}_1^3-\beta (\frac{\mathrm{\partial }{W}_1^3}{\mathrm{\partial }{y}_3^2}+\frac{\mathrm{\partial }{W}_1^2}{\mathrm{\partial }{y}_2\mathrm{\partial }{y}_3})=y_2{H}_7+y_3{H}_8\text{in}\mathrm{\Omega },\\ & W_1^2=W_1^3=0\text{on}\mathrm{\Gamma },\end{array}$(35) ) for the second and third component of the microrotation first-order corrector $(W_1^2,W_1^3)$ are given by: (A3) $\begin{array}{rl}{H}_5(t,x_1)& =\frac{\delta \kappa \cos \alpha }{2\delta +\beta }(g_2(t,x_1)\cos \alpha +g_3(t,x_1)\sin \alpha )+\frac{\beta \kappa }{2\delta +\beta }{g}_2(t,x_1)-\frac{\beta }{2\delta }\frac{\mathrm{\partial }{g}_1(t,x_1)}{\mathrm{\partial }{x}_1},\\ H_6(t,x_1)& =-\frac{\delta \kappa \sin \alpha }{2\delta +\beta }(g_2\cos \alpha +g_3\sin \alpha )-\frac{4a}{\pi }{F}_0^{\ast }\left(t\right),\\ H_7(t,x_1)& =\frac{\kappa \delta \cos \alpha }{2\delta +\beta }(g_3\cos \alpha -g_2\sin \alpha )+\frac{4a}{\pi }{F}_0^{\ast }\left(t\right),\\ H_8(t,x_1)& =\frac{\delta \kappa \sin \alpha }{2\delta +\beta }(g_2(t,x_1)\sin \alpha -g_3(t,x_1)\cos \alpha \sin \alpha )+\frac{\beta \kappa }{2\delta +\beta }{g}_2(t,x_1)-\frac{\beta }{2\delta }\frac{\mathrm{\partial }{g}_1(t,x_1)}{\mathrm{\partial }{x}_1}.\end{array}$(A3) The functions $H_9(t,x_1),\dots ,H_{12}(t,x_1)$ in the expressions (36(36) $\begin{array}{rl}& W_1^2(t,x_1,{\mathit{y}}_{\ast })=(1-|{\mathit{y}}_{\ast }{|}^2\left)\right(y_2{H}_9(t,x_1)+y_3{H}_{10}(t,x_1)),\\ & W_1^3(t,x_1,{\mathit{y}}_{\ast })=(1-|{\mathit{y}}_{\ast }{|}^2\left)\right(y_2{H}_{11}(t,x_1)+y_3{H}_{12}(t,x_1)),\end{array}$(36) ) for the second and third component of the microrotation first-order corrector $(W_1^2,W_1^3)$ are given by: (A4) $\begin{array}{rl}& H_9(t,x_1)=\frac{\beta H_8-(4\delta +3\beta )H_5}{2[{\beta }^2-(4\delta +3\beta {)}^2]},H_{10}(t,x_1)=\frac{\beta H_7-(4\delta +\beta )H_6}{2[{\beta }^2-(4\delta +\beta {)}^2]},\\ & H_{11}(t,x_1)=\frac{1}{2\beta }{H}_6-\frac{4\delta +\beta }{\beta }\frac{\beta H_7-(4\delta +\beta )H_6}{2[{\beta }^2-(4\delta +\beta {)}^2]},H_{12}(t,x_1)=\frac{1}{2\beta }{H}_5-\frac{4\delta +\beta }{\beta }\frac{\beta H_8-(4\delta +\beta )H_5}{2[{\beta }^2-(4\delta +\beta {)}^2]}.\end{array}$(A4)

Second-order corrector

The functions $A_1(t,x_1),\dots ,A_4(t,x_1)$ in the problem (38(38) $\begin{array}{rl}& {\mathrm{\Delta }}_{{\mathit{y}}_{\ast }}{V}_2^1=A_1{y}_2^2+A_2{y}_2{y}_3+A_3{y}_3^2+A_4\text{in}\mathrm{\Omega },\\ & V_2^1=0\text{on}\mathrm{\Gamma },\end{array}$(38) ) for the first component of the velocity second-order corrector $V_2^1$ are given as follows: (A5) $\begin{array}{rl}& A_1(t,x_1)\\ & =-\frac{2}{\pi \mu }\frac{\mathrm{d}}{\mathrm{d}t}{F}_0^{\ast }+\frac{3\kappa \cos \alpha }{8\mu }{H}_1-\frac{\kappa \sin \alpha }{8\mu }{H}_2-\frac{2{\kappa }^2}{\pi }{F}_0^{\ast }+\frac{\kappa }{\mu }\cos \alpha H_1+\frac{4{\kappa }^2{\cos }^2\alpha }{\pi }{F}_0^{\ast }\\ & -\frac{{\kappa }^2}{\mu }{\cos }^2\alpha (\frac{8\mu }{\pi }{F}_0^{\ast }-f_1)+\frac{\kappa }{\mu }({\cos }^2\alpha \frac{\mathrm{\partial }{f}_2}{\mathrm{\partial }{x}_1}-\sin \alpha \cos \alpha f_2+\cos \alpha \sin \alpha \frac{\mathrm{\partial }{f}_3}{\mathrm{\partial }{x}_1}+{\cos }^2\alpha f_3)\\ & -\frac{a}{\mu }(-3H_{11}+H_{10}),\\ & A_2(t,x_1)\\ & =\frac{\kappa \cos \alpha }{4\mu }{H}_2-\frac{\kappa \sin \alpha }{4\mu }{H}_1+\frac{\kappa \cos \alpha }{\mu }{H}_2-\frac{\kappa \sin \alpha }{\mu }{H}_1-\frac{8{\kappa }^2\cos \alpha \sin \alpha }{\pi }{F}_0^{\ast }+\frac{2{\kappa }^2\sin \alpha \cos \alpha }{\mu }(\frac{8\mu }{\pi }{F}_0^{\ast }+f_1)\\ & -\frac{\kappa }{\mu }(-2\sin \alpha \cos \alpha \frac{\mathrm{\partial }{f}_2}{\mathrm{\partial }{x}_1}-({\cos }^2\alpha -{\sin }^2\alpha )f_2+({\cos }^2\alpha -{\sin }^2\alpha )\frac{\mathrm{\partial }{f}_3}{\mathrm{\partial }{x}_1}\\ & -2f_3\sin \alpha \cos \alpha )+\frac{a}{\mu }(2H_{12}-2H_9),\\ & A_3(t,x_1)\\ & =-\frac{2}{\pi \mu }\frac{\mathrm{d}}{\mathrm{d}t}{F}_0^{\ast }\left(t\right)+\frac{\kappa \cos \alpha }{8\mu }{H}_1-\frac{3\kappa \sin \alpha }{8\mu }{H}_2-\frac{2{\kappa }^2}{\pi }{F}_0^{\ast }\left(t\right)-\frac{\kappa \sin \alpha }{\mu }{H}_2+\frac{4{\kappa }^2{\sin }^2\alpha }{\pi }{F}_0^{\ast }\left(t\right)\\ & -\frac{{\kappa }^2{\sin }^2\alpha }{\mu }(\frac{8}{\pi }{F}_0^{\ast }-f_1)+\frac{\kappa }{\mu }(\frac{\mathrm{\partial }{f}_2}{\mathrm{\partial }{x}_1}{\sin }^2\alpha -\sin \alpha \cos \alpha f_2+\frac{\mathrm{\partial }{f}_3}{\mathrm{\partial }{x}_1}\sin \alpha \cos \alpha +f_3{\sin }^2\alpha )\\ & -\frac{a}{\mu }(-H_{11}+3H_{10}),\\ & A_4(t,x_1)\\ & =\frac{2}{\mu \pi }\frac{\mathrm{d}}{\mathrm{d}t}{F}_0^{\ast }\left(t\right)-\frac{\kappa \cos \alpha }{8\mu }{H}_1+\frac{\kappa \sin \alpha }{8\mu }{H}_2+\frac{2{\kappa }^2}{\pi }{F}_0^{\ast }\left(t\right)+\frac{1}{\mu }{p}_1\left(t\right)+\frac{1}{\mu }{x}_1(\frac{p_2\left(t\right)-p_1\left(t\right)}{l})-\frac{a}{\mu }(H_{11}-H_{10}).\end{array}$(A5) The functions $A_5(t,x_1),\dots ,A_{14}(t,x_1)$ in the problem (40(40) $\begin{array}{rl}& -\mu {\mathrm{\Delta }}_{{\mathit{y}}_{\ast }}{V}_2^2+\frac{\mathrm{\partial }{P}_3}{\mathrm{\partial }{y}_2}=A_5{y}_2^2+A_6{y}_2{y}_3+A_7{y}_3^2+A_8,\\ & -\mu {\mathrm{\Delta }}_{{\mathit{y}}_{\ast }}{V}_2^3+\frac{\mathrm{\partial }{P}_3}{\mathrm{\partial }{y}_3}=A_9{y}_2^2+A_{10}{y}_2{y}_3+A_{11}{y}_3^2+A_{12},\\ & \frac{\mathrm{\partial }{V}_2^2}{\mathrm{\partial }{y}_2}+\frac{\mathrm{\partial }{V}_2^3}{\mathrm{\partial }{y}_3}=A_{13}{y}_2^3+A_{14}{y}_3^3+A_{13}{y}_2{y}_3^2+A_{14}{y}_2^2{y}_3-A_{13}{y}_2-A_{14}{y}_3,\\ & V_2^2=V_2^3=0\text{on}\mathrm{\Gamma },\end{array}$(40) ) for the second and third component of the velocity second-order corrector and third-order pressure corrector $(V_2^2,V_2^3,P_3)$ are given as follows: $\begin{array}{rl}{A}_5(t,x_1)& =\frac{a{g}_1\mu \kappa \sin \alpha }{16\mu \delta }+\frac{2\mu ({\kappa }^{\prime }\cos \alpha +\kappa \tau \sin \alpha )F_0^{\ast }}{\pi }-a{H}_4\\ & +\frac{a}{2(2\delta +\beta )}(\frac{\mathrm{\partial }{g}_3}{\mathrm{\partial }{x}_1}\cos \alpha +g_3\tau \sin \alpha -\frac{\mathrm{\partial }{g}_2}{\mathrm{\partial }{x}_1}\sin \alpha +\tau g_2\cos \alpha ),\\ A_6(t,x_1)& =-\frac{a{g}_1\mu \kappa \cos \alpha }{8\mu \delta }-2a{H}_3-\frac{a\kappa g_1\cos \alpha }{2\delta },\\ A_7(t,x_1)& =-\frac{3a{g}_1\mu \kappa \sin \alpha }{16\mu \delta }-\frac{2\mu ({\kappa }^{\prime }\cos \alpha +\kappa \tau \sin \alpha )F_0^{\ast }}{\pi }-3a{H}_4+\frac{a\kappa \sin \alpha g_1}{2\delta }\\ & +\frac{a}{2(2\delta +\beta )}(\frac{\mathrm{\partial }{g}_3}{\mathrm{\partial }{x}_1}\cos \alpha +g_3\tau \sin \alpha -\frac{\mathrm{\partial }{g}_2}{\mathrm{\partial }{x}_1}\sin \alpha +\tau g_2\cos \alpha ),\\ A_8(t,x_1)& =\frac{a{g}_1\mu \kappa \sin \alpha }{16\mu \delta }+\frac{2\mu ({\kappa }^{\prime }\cos \alpha +\kappa \tau \sin \alpha )F_0^{\ast }}{\pi }\\ & +a{H}_4-\frac{a}{2(2\delta +\beta )}(\frac{\mathrm{\partial }{g}_3}{\mathrm{\partial }{x}_1}\cos \alpha +g_3\tau \sin \alpha -\frac{\mathrm{\partial }{g}_2}{\mathrm{\partial }{x}_1}\sin \alpha +\tau g_2\cos \alpha ),\\ A_9(t,x_1)& =\frac{3a\kappa \cos \alpha g_1}{16\mu \delta }-\frac{2\mu (\kappa \tau \cos \alpha -{\kappa }^{\prime }\sin \alpha )F_0^{\ast }}{\pi }\\ & -\frac{a}{2(2\delta +\beta )}(\frac{\mathrm{\partial }{g}_2}{\mathrm{\partial }{x}_1}\cos \alpha +g_2\tau \sin \alpha +\frac{\mathrm{\partial }{g}_3}{\mathrm{\partial }{x}_1}\sin \alpha -g_3\tau \sin \alpha )\\ & +3a{H}_3+\frac{a{g}_1\kappa \cos \alpha }{2\delta },\end{array}$ (A6) $\begin{array}{rl}{A}_{10}(t,x_1)& =-\frac{a{g}_1\mu \kappa \sin \alpha }{8\mu \delta }+2a{H}_4-\frac{a{g}_1\kappa \sin \alpha }{2\delta },\\ A_{11}(t,x_1)& =\frac{a\kappa \cos \alpha g_1}{16\mu \delta }-\frac{2\mu (\kappa \tau \cos \alpha -{\kappa }^{\prime }\sin \alpha )F_0^{\ast }}{\pi }\\ & -\frac{a}{2(2\delta +\beta )}(\frac{\mathrm{\partial }{g}_2}{\mathrm{\partial }{x}_1}\cos \alpha +g_2\tau \sin \alpha +\frac{\mathrm{\partial }{g}_3}{\mathrm{\partial }{x}_1}\sin \alpha -g_3\tau \sin \alpha )+a{H}_3,\\ A_{12}(t,x_1)& =-\frac{a{g}_1\mu \kappa \cos \alpha }{16\mu \delta }+\frac{2\mu (\kappa \tau \cos \alpha -{\kappa }^{\prime }\sin \alpha )F_0^{\ast }}{\pi }\\ & +\frac{a}{2(2\delta +\beta )}(\frac{\mathrm{\partial }{g}_2}{\mathrm{\partial }{x}_1}\cos \alpha +g_2\tau \sin \alpha +\frac{\mathrm{\partial }{g}_3}{\mathrm{\partial }{x}_1}\sin \alpha -g_3\tau \sin \alpha )-a{H}_3,\\ A_{13}(t,x_1)& =\frac{1}{8\mu }\frac{\mathrm{\partial }{H}_1}{\mathrm{\partial }{x}_1}+\frac{a\kappa g_1\sin \alpha }{16\mu \delta }+\frac{2{\kappa }^{\prime }\cos \alpha +2\kappa \tau \sin \alpha }{\pi }{F}_0^{\ast },\\ A_{14}(t,x_1)& =\frac{1}{8\mu }\frac{\mathrm{\partial }{H}_2}{\mathrm{\partial }{x}_1}+\frac{a\kappa g_1\cos \alpha }{16\mu \delta }+\frac{-2{\kappa }^{\prime }\sin \alpha +2\kappa \tau \cos \alpha }{\pi }{F}_0^{\ast }.\end{array}$(A6) The functions $M_1(t,x_1),\dots ,M_6(t,x_1)$ and $B_5(t,x_1),\dots ,B_{12}(t,x_1)$ in the expressions (41(41) $\begin{array}{rl}& V_2^2(t,x_1,{\mathit{y}}_{\ast })=(1-|{\mathit{y}}_{\ast }{|}^2\left)\right(B_5{y}_2^2+B_6{y}_2{y}_3+B_7{y}_2^3+B_8),\\ & V_2^3(t,x_1,{\mathit{y}}_{\ast })=(1-|{\mathit{y}}_{\ast }{|}^2\left)\right(B_9{y}_2^2+B_{10}{y}_2{y}_3+B_{11}{y}_2^3+B_{12}),\\ & P_3(t,x_1,{\mathit{y}}_{\ast })=M_1{y}_2^3+M_2{y}_3^3+M_3{y}_2{y}_3^2+M_4{y}_2^2{y}_3+M_5{y}_2+M_6{y}_3.\end{array}$(41) ) for the second and third component of the velocity second-order corrector and third-order pressure corrector $(V_2^2,V_2^3,P_3)$ are given by: (A7) $\begin{array}{rl}{M}_1(t,x_1)& =\mu A_{13}+\frac{1}{3}{A}_5-\frac{1}{12}{A}_7+\frac{1}{24}{A}_{10},M_2(t,x_1)=\frac{144}{147}\mu A_{14}+\frac{4}{147}{A}_6-\frac{8}{147}{A}_9+\frac{8}{147}{A}_{11},\\ M_3(t,x_1)& =\mu A_{13}+\frac{1}{4}{A}_7-\frac{3}{8}{A}_{10},M_4(t,x_1)=\frac{96}{105}\mu A_{14}-\frac{8}{21}{A}_6+\frac{5}{21}{A}_9+\frac{17}{105}{A}_{11},\\ M_5(t,x_1)& =-\frac{4}{3}\mu A_{13}+\frac{1}{6}{A}_7-A_8-\frac{5}{24}{A}_{10},M_6(t,x_1)=-\frac{998}{735}\mu A_{14}-\frac{191}{882}{A}_6\\ & +\frac{79}{441}{A}_9+\frac{5491}{311640}{A}_{11}+A_{12},\end{array}$(A7) and (A8) $\begin{array}{rl}{B}_5(t,x_1)& =-\frac{21}{96\mu }{M}_1+\frac{1}{96\mu }{M}_3+\frac{7}{96\mu }{A}_5-\frac{1}{96\mu }{A}_7,B_6(t,x_1)=-\frac{1}{6\mu }{M}_4+\frac{1}{12\mu }{A}_6,\\ B_7(t,x_1)& =\frac{3}{96\mu }{M}_1-\frac{7}{96\mu }{M}_3-\frac{1}{96\mu }{A}_5+\frac{7}{96\mu }{A}_7,\\ B_8(t,x_1)& =-\frac{9}{96\mu }{M}_1-\frac{3}{96\mu }{M}_3+\frac{3}{96\mu }{A}_5+\frac{3}{96\mu }{A}_7-\frac{1}{4\mu }{M}_5+\frac{1}{4\mu }{A}_8,\\ B_9(t,x_1)& =\frac{3}{96\mu }{M}_2-\frac{7}{96\mu }{M}_4+\frac{7}{96\mu }{A}_9-\frac{1}{96\mu }{A}_{11},B_{10}(t,x_1)=-\frac{1}{6\mu }{M}_3+\frac{1}{12\mu }{A}_{10},\\ B_{11}(t,x_1)& =-\frac{21}{96\mu }{M}_2+\frac{1}{96\mu }{M}_4+\frac{7}{96\mu }{A}_{11}-\frac{1}{96\mu }{A}_9,\\ B_{12}(t,x_1)& =-\frac{9}{96\mu }{M}_2-\frac{3}{96\mu }{M}_4+\frac{3}{96\mu }{A}_9+\frac{6}{96\mu }{A}_{11}-\frac{1}{4\mu }{M}_6+\frac{1}{4\mu }{A}_{12}.\end{array}$(A8) The functions $C_1(t,x_1),\dots ,C_4(t,x_1)$ in the problem (43(43) $\begin{array}{rl}& {\mathrm{\Delta }}_{{\mathit{y}}_{\ast }}{W}_2^1=C_1{y}_2^2+C_2{y}_2{y}_3+C_3{y}_3^2+C_4\text{in}\mathrm{\Omega },\\ & W_2^1=0\text{on}\mathrm{\Gamma },\end{array}$(43) ) for the first component of the microrotation second-order corrector $W_2^1$ are given as follows: $\begin{array}{rl}{C}_1(t,x_1)& =-\frac{1}{4{\delta }^2}\frac{\mathrm{\partial }{g}_1}{\mathrm{\partial }t}-\frac{1}{4\delta }\frac{{\mathrm{\partial }}^2{g}_1}{\mathrm{\partial }{x}_1^2}+\frac{3\kappa \cos \alpha }{8\mu }{H}_3-\frac{1}{2\delta (2\delta +\beta )}(\frac{\mathrm{\partial }{g}_2}{\mathrm{\partial }{x}_1}\cos \alpha +\tau g_2\sin \alpha +\frac{\mathrm{\partial }{g}_3}{\mathrm{\partial }{x}_1}\sin \alpha -\tau g_3\cos \alpha )\\ & +\frac{\kappa \sin \alpha }{2(2\delta +\beta )}(\frac{\mathrm{\partial }{g}_3}{\mathrm{\partial }{x}_1}\cos \alpha +\tau g_3\sin \alpha -\frac{\mathrm{\partial }{g}_2}{\mathrm{\partial }{x}_1}\sin \alpha +\tau g_2\cos \alpha )-\frac{\kappa \sin \alpha }{8\mu }{H}_4\\ & +\frac{1}{2(2\delta +\beta )}\frac{\mathrm{\partial }}{\mathrm{\partial }{x}_1}((\kappa -{\kappa }^{\prime })\cos \alpha (g_2\cos \alpha +g_3\sin \alpha )-(\kappa -{\kappa }^{\prime })\sin \alpha (g_3\cos \alpha -g_2\sin \alpha ))\\ & -\frac{{\kappa }^2}{4\delta }{g}_1+\frac{\kappa }{\mu }\cos \alpha H_3+\frac{{\kappa }^2}{2\delta }{\cos }^2\alpha g_1+\frac{\beta }{4{\delta }^2}\frac{{\mathrm{\partial }}^2{g}_1}{\mathrm{\partial }{x}_1^2}\\ & +\frac{\beta }{2\delta (2\delta +\beta )}(-(\kappa \cos \alpha {)}^{\prime }(g_2\cos \alpha +g_3\sin \alpha )-\kappa \cos \alpha (g_2\cos \alpha +g_3\sin \alpha {)}^{\prime }\\ & +(\kappa \sin \alpha {)}^{\prime }(g_3\cos \alpha -g_2\sin \alpha )+\kappa \sin \alpha (g_3\cos \alpha -g_2\sin \alpha {)}^{\prime })+\frac{3\beta }{\delta }\frac{\mathrm{\partial }{H}_9}{\mathrm{\partial }{x}_1}+\frac{\beta }{\delta }\frac{\mathrm{\partial }{H}_{12}}{\mathrm{\partial }{x}_1}\\ & +\frac{\beta \kappa }{\delta (2\delta +\beta )}\cos \alpha (\frac{\mathrm{\partial }{g}_2}{\mathrm{\partial }{x}_1}\cos \alpha +\tau g_2\sin \alpha +\frac{\mathrm{\partial }{g}_3}{\mathrm{\partial }{x}_1}\sin \alpha -\tau g_3\cos \alpha )+\frac{4a^2}{16\mu {\delta }^2}{g}_1-\frac{a}{2{\delta }^2}{g}_1,\\ C_2(t,x_1)& =\frac{\kappa \cos \alpha }{4\mu }{H}_4-\frac{\kappa \sin \alpha }{4\mu }{H}_3-\frac{\kappa \sin \alpha }{\mu }{H}_3+\frac{\kappa }{\mu }\cos \alpha H_4+\frac{{\kappa }^2\sin \alpha \cos \alpha }{\delta }{g}_1+\frac{2\beta }{\delta }\frac{\mathrm{\partial }{H}_{11}}{\mathrm{\partial }{x}_1}\\ & -\frac{\beta \kappa \sin \alpha }{\delta (2\delta +\beta )}(\frac{\mathrm{\partial }{g}_2}{\mathrm{\partial }{x}_1}\cos \alpha +\tau g_2\sin \alpha +\frac{\mathrm{\partial }{g}_3}{\mathrm{\partial }{x}_1}\sin \alpha -\tau g_3\cos \alpha )\\ & +\frac{\beta \kappa \cos \alpha }{\delta (2\delta +\beta )}(\frac{\mathrm{\partial }{g}_3}{\mathrm{\partial }{x}_1}\cos \alpha +\tau g_3\sin \alpha -\frac{\mathrm{\partial }{g}_2}{\mathrm{\partial }{x}_1}\sin \alpha -\tau g_2\cos \alpha ),\end{array}$ $\begin{array}{rl}{C}_3(t,x_1)& =-\frac{1}{4{\delta }^2}\frac{\mathrm{\partial }{g}_1}{\mathrm{\partial }t}-\frac{1}{4\delta }\frac{{\mathrm{\partial }}^2{g}_1}{\mathrm{\partial }{x}_1^2}+\frac{\kappa \cos \alpha }{8\mu }{H}_3-\frac{\kappa \cos \alpha }{2(2\delta +\beta )}(\frac{\mathrm{\partial }{g}_2}{\mathrm{\partial }{x}_1}\cos \alpha +\tau g_2\sin \alpha +\frac{\mathrm{\partial }{g}_3}{\mathrm{\partial }{x}_1}\sin \alpha -\tau g_3\sin \alpha )\\ & +\kappa \sin \alpha \frac{1}{2(2\delta +\beta )}(\frac{\mathrm{\partial }{g}_3}{\mathrm{\partial }{x}_1}\cos \alpha +\tau g_3\sin \alpha -\frac{\mathrm{\partial }{g}_2}{\mathrm{\partial }{x}_1}\sin \alpha +\tau g_2\cos \alpha )-\frac{3\kappa \sin \alpha }{8\mu }{H}_4\\ & +\frac{1}{2\delta (2\delta +\beta )}(\frac{\mathrm{\partial }}{\mathrm{\partial }{x}_1}((\kappa -{\kappa }^{\prime })\cos \alpha (g_2\cos \alpha +g_3\sin \alpha )-(\kappa -{\kappa }^{\prime })\sin \alpha (g_3\cos \alpha -g_2\sin \alpha )))\\ & -{\kappa }^2\frac{1}{4\delta }{g}_1\\ & -\frac{\kappa }{\mu }\sin \alpha H_4+\frac{{\kappa }^2{\sin }^2\alpha }{2\delta }{g}_1+\frac{\beta }{4{\delta }^2}\frac{{\mathrm{\partial }}^2{g}_1}{\mathrm{\partial }{x}_1^2}+\frac{\beta }{2\delta (2\delta +\beta )}((\kappa \cos \alpha {)}^{\prime }(g_2\cos \alpha +g_3\sin \alpha )\\ & -\kappa \cos \alpha (g_2\cos \alpha +g_3\sin \alpha {)}^{\prime }+(\kappa \sin \alpha {)}^{\prime }(g_3\cos \alpha -g_2\sin \alpha )+\kappa \sin \alpha (g_3\cos \alpha -g_2\sin \alpha {)}^{\prime })\\ & -\frac{\beta }{\delta }\frac{\mathrm{\partial }{H}_9}{\mathrm{\partial }{x}_1}\\ & +\frac{3\beta }{\delta }\frac{\mathrm{\partial }{H}_{12}}{\mathrm{\partial }{x}_1}-\frac{\beta \kappa \sin \alpha }{\delta (2\delta +\beta )}(\frac{\mathrm{\partial }{g}_3}{\mathrm{\partial }{x}_1}\cos \alpha +\tau g_3\sin \alpha -\frac{\mathrm{\partial }{g}_2}{\mathrm{\partial }{x}_1}\sin \alpha +\tau g_2\cos \alpha )+\frac{a^2{g}_1}{4\mu {\delta }^2}-\frac{a{g}_1}{2{\delta }^2},\end{array}$ (A9) $\begin{array}{rl}{C}_4(t,x_1)=& \frac{1}{4{\delta }^2}\frac{\mathrm{\partial }{g}_1}{\mathrm{\partial }t}+\frac{1}{4\delta }\frac{{\mathrm{\partial }}^2{g}_1}{\mathrm{\partial }{x}_1^2}-\frac{\kappa \cos \alpha }{8\mu }{H}_3+\frac{\kappa \cos \alpha }{2\delta (2\delta +\beta )}(\frac{\mathrm{\partial }{g}_2}{\mathrm{\partial }{x}_1}\cos \alpha +\tau g_2\sin \alpha +\frac{\mathrm{\partial }{g}_3}{\mathrm{\partial }{x}_1}\sin \alpha -\tau g_3\sin \alpha )\\ & -\frac{\kappa \sin \alpha }{2(2\delta +\beta )}(\frac{\mathrm{\partial }{g}_3}{\mathrm{\partial }{x}_1}\cos \alpha +\tau g_3\sin \alpha -\frac{\mathrm{\partial }{g}_2}{\mathrm{\partial }{x}_1}\sin \alpha +\tau g_2\cos \alpha )+\frac{\kappa \sin \alpha }{8\mu }{H}_4\\ & -\frac{1}{2(2\delta +\beta )}\frac{\mathrm{\partial }}{\mathrm{\partial }{x}_1}((\kappa -{\kappa }^{\prime })\cos \alpha (g_2\cos \alpha +g_3\sin \alpha )-(\kappa -{\kappa }^{\prime })\sin \alpha (g_3\cos \alpha -g_2\sin \alpha ))\\ & +\frac{{\kappa }^2}{4\delta }{g}_1-\frac{\beta }{4{\delta }^2}\frac{{\mathrm{\partial }}^2{g}_1}{\mathrm{\partial }{x}_1^2}-\frac{\beta }{2\delta (2\delta +\beta )}(-(\kappa \cos \alpha {)}^{\prime }(g_2\cos \alpha +g_3\sin \alpha )-\kappa \cos \alpha (g_2\cos \alpha +g_3\sin \alpha {)}^{\prime })\\ & +(\kappa \sin \alpha {)}^{\prime }(g_3\cos \alpha -g_2\sin \alpha )+\kappa \sin \alpha (g_3\cos \alpha -g_2\sin \alpha {)}^{\prime })\\ & +\frac{\beta }{\delta }\frac{\mathrm{\partial }{H}_9}{\mathrm{\partial }{x}_1}-\frac{\beta }{\delta }\frac{\mathrm{\partial }{H}_{12}}{\mathrm{\partial }{x}_1}-\frac{a^2}{8\mu {\delta }^2}{g}_1+\frac{a}{2{\delta }^2}{g}_1.\end{array}$(A9) The functions $C_5(t,x_1),\dots ,C_{12}(t,x_1)$ in the problem (47(47) $\begin{array}{rl}& -\delta (\frac{{\mathrm{\partial }}^2{W}_2^2}{\mathrm{\partial }{y}_2^2}+\frac{\mathrm{\partial }{W}_2^2}{\mathrm{\partial }{y}_3^2})-\beta (\frac{{\mathrm{\partial }}^2{W}_2^2}{\mathrm{\partial }{y}_2^2}+\frac{\mathrm{\partial }{W}_2^3}{\mathrm{\partial }{y}_2\mathrm{\partial }{y}_3})=C_5{y}_2^2+C_6{y}_2{y}_3+C_7{y}_3^2+C_8\text{in}\mathrm{\Omega },\\ & -\delta (\frac{{\mathrm{\partial }}^2{W}_2^3}{\mathrm{\partial }{y}_2^2}+\frac{{\mathrm{\partial }}^2{W}_2^3}{\mathrm{\partial }{y}_3^2})-\beta (\frac{{\mathrm{\partial }}^2{W}_2^2}{\mathrm{\partial }{y}_2\mathrm{\partial }{y}_3}+\frac{{\mathrm{\partial }}^2{W}_2^3}{\mathrm{\partial }{y}_3^2})=C_9{y}_2^2+C_{10}{y}_2{y}_3+C_{11}{y}_3^2+C_{12}\text{in}\mathrm{\Omega },\\ W_2^2& =W_2^3=0\text{on}\mathrm{\Gamma }.\end{array}$(47) ) for the second and third component of the microrotation second-order corrector $(W_2^2,W_2^3)$ read: $\begin{array}{rl}& C_5(t,x_1)\\ & =\frac{1}{2\delta +\beta }(\frac{\mathrm{\partial }{g}_2}{\mathrm{\partial }t}\cos \alpha +\frac{\mathrm{\partial }{g}_3}{\mathrm{\partial }t}\sin \alpha )-\frac{\delta }{2(2\delta +\beta )}(\frac{{\mathrm{\partial }}^2{g}_2}{\mathrm{\partial }{x}_1^2}\cos \alpha +\frac{\mathrm{\partial }{g}_2}{\mathrm{\partial }{x}_1}\tau \sin \alpha +({\tau }^{\prime }{g}_2+\tau \frac{\mathrm{\partial }{g}_2}{\mathrm{\partial }{x}_1})\sin \alpha -{\tau }^2{g}_2\cos \alpha \\ & +\frac{{\mathrm{\partial }}^2{g}_3}{\mathrm{\partial }{x}_1^2}\sin \alpha -\frac{\mathrm{\partial }{g}_3}{\mathrm{\partial }{x}_1}\tau \cos \alpha -({\tau }^{\prime }{g}_3+\tau \frac{\mathrm{\partial }{g}_3}{\mathrm{\partial }{x}_1})\cos \alpha -{\tau }^2{g}_3\sin \alpha )+3\delta \kappa \cos \alpha H_9-\delta \kappa \sin \alpha H_{10}\\ & +\frac{2\delta {\kappa }^2}{2\delta +\beta }{\cos }^2\alpha (g_2\cos \alpha +g_3\sin \alpha )-\frac{1}{4}((2\kappa \frac{\mathrm{\partial }{g}_1}{\mathrm{\partial }{x}_1}+{\kappa }^{\prime }{g}_1-\frac{2\delta {\kappa }^2}{2\delta +\beta }(g_2\cos \alpha +g_3\sin \alpha ))\cos \alpha +\kappa \tau g_1\sin \alpha )\\ & -{\cos }^2\alpha \frac{\delta {\kappa }^2}{2\delta +\beta }(g_2\cos \alpha +g_3\sin \alpha )-\frac{3\beta }{8\mu }\frac{\mathrm{\partial }{H}_3}{\mathrm{\partial }{x}_1}+3\beta \kappa \cos \alpha H_9-3\beta \kappa \sin \alpha H_{11}\\ & -\beta \cos \alpha (\frac{{\kappa }^{\prime }}{4\delta }{g}_1+\frac{{\kappa }^2\cos \alpha }{2(2\delta +\beta )}(g_2\cos \alpha +g_3\sin \alpha )-\frac{{\kappa }^2\sin \alpha }{2(2\delta +\beta )}(g_3\sin \alpha -g_2\cos \alpha )\\ & +2\kappa (\frac{1}{4\delta }\frac{\mathrm{\partial }{g}_1}{\mathrm{\partial }{x}_1}-\kappa (\cos \alpha \frac{1}{2(2\delta +\beta )}(g_2\cos \alpha +g_3\sin \alpha )-\sin \alpha \frac{1}{2(2\delta +\beta )}(g_3\cos \alpha -g_2\sin \alpha )))\\ & -\frac{\beta \kappa \tau }{4\delta }\sin \alpha g_1\\ & -\frac{\beta {\kappa }^{\prime }}{2\delta }\cos \alpha g_1-\frac{\beta \kappa \tau }{2\delta }\sin \alpha g_1-\beta {\kappa }^2{\cos }^2\alpha \frac{1}{2\delta +\beta }(g_2\cos \alpha +g_3\sin \alpha )\\ & -\beta \kappa \cos \alpha (\frac{1}{\delta }\frac{\mathrm{\partial }{g}_1}{\mathrm{\partial }{x}_1}-\kappa (\frac{1}{2\delta +\beta }\cos \alpha (g_2\cos \alpha +g_3\sin \alpha )+\frac{1}{2\delta +\beta }\sin \alpha (g_3\cos \alpha -g_2\sin \alpha )))\\ & -\frac{a}{8\mu }{H}_2+\frac{a}{2\delta +\beta }(g_2\cos \alpha +g_3\sin \alpha ),\end{array}$ $\begin{array}{rl}& C_6(t,x_1)\\ & =2\delta \kappa \cos \alpha H_{10}-2\delta \kappa \sin \alpha H_9-\frac{2\delta {\kappa }^2}{2\delta +\beta }\sin \alpha \cos \alpha (g_2\cos \alpha +g_3\sin \alpha )-\frac{\beta }{4\mu }\frac{\mathrm{\partial }{H}_4}{\mathrm{\partial }{x}_1}\\ & +2\beta \kappa \cos \alpha H_{10}-2\beta \kappa \sin \alpha H_{12}+\sin \alpha \frac{\beta {\kappa }^{\prime }{g}_1}{2\delta }-\frac{\beta \kappa \tau }{2\delta }\cos \alpha g_1+\beta {\kappa }^2\sin \alpha \cos \alpha \frac{1}{2\delta +\beta }(g_2\cos \alpha +g_3\sin \alpha )\\ & +\beta {\kappa }^2\sin \alpha \cos \alpha (g_3\cos \alpha -g_2\sin \alpha )-\beta \kappa \cos \alpha \frac{1}{\delta }\frac{\mathrm{\partial }{g}_1}{\mathrm{\partial }{x}_1}+2\beta {\kappa }^2\sin \alpha \cos \alpha \frac{1}{2\delta +\beta }(g_2\cos \alpha +g_3\sin \alpha )\\ & -2\beta {\kappa }^2{\sin }^2\alpha \frac{1}{2\delta +\beta }(g_3\cos \alpha -g_2\sin \alpha )-\frac{a}{4\mu }{H}_1-\frac{4a\kappa }{\pi }\cos \alpha F_0^{\ast },\end{array}$ $\begin{array}{rl}& C_7(t,x_1)\\ & =\frac{1}{2\delta +\beta }(\frac{\mathrm{\partial }{g}_2}{\mathrm{\partial }t}\cos \alpha +\frac{\mathrm{\partial }{g}_3}{\mathrm{\partial }t}\sin \alpha )-\frac{\delta }{2(2\delta +\beta )}(\frac{{\mathrm{\partial }}^2{g}_2}{\mathrm{\partial }{x}_1^2}\cos \alpha +\frac{\mathrm{\partial }{g}_2}{\mathrm{\partial }{x}_1}\tau \sin \alpha +({\tau }^{\prime }{g}_2+\tau \frac{\mathrm{\partial }{g}_2}{\mathrm{\partial }{x}_1})\sin \alpha \\ & -{\tau }^2{g}_2\cos \alpha +\frac{{\mathrm{\partial }}^2{g}_3}{\mathrm{\partial }{x}_1^2}\sin \alpha -\frac{\mathrm{\partial }{g}_3}{\mathrm{\partial }{x}_1}\tau \cos \alpha -({\tau }^{\prime }{g}_3+\tau \frac{\mathrm{\partial }{g}_3}{\mathrm{\partial }{x}_1})\cos \alpha -{\tau }^2{g}_3\sin \alpha )+\delta \kappa \cos \alpha H_9\\ & -3\delta \kappa \sin \alpha H_{10}+\frac{2\delta {\kappa }^2}{2\delta +\beta }{\sin }^2\alpha (g_2\cos \alpha +g_3\sin \alpha )-\frac{1}{4}((2\kappa \frac{\mathrm{\partial }{g}_1}{\mathrm{\partial }{x}_1}+{\kappa }^{\prime }{g}_1\\ & -\frac{2\delta {\kappa }^2}{2\delta +\beta }(g_2\cos \alpha +g_3\sin \alpha ))\cos \alpha )+\kappa \tau g_1\sin \alpha )+{\kappa }^2{\sin }^2\alpha \frac{\delta }{2\delta +\beta }(g_2\cos \alpha +g_3\sin \alpha )\\ & -\frac{\beta }{8\mu }\frac{\mathrm{\partial }{H}_3}{\mathrm{\partial }{x}_1}+\beta \kappa \cos \alpha H_9\\ & -\beta \kappa \sin \alpha H_{11}-\beta \cos \alpha (\frac{{\kappa }^{\prime }}{4\delta }{g}_1+\frac{{\kappa }^2\cos \alpha }{2(2\delta +\beta )}(g_2\cos \alpha +g_3\sin \alpha )-\frac{{\kappa }^2\sin \alpha }{2(2\delta +\beta )}(g_2\cos \alpha -g_3\sin \alpha )\\ & +2\kappa (\frac{1}{4\delta }\frac{\mathrm{\partial }{g}_1}{\mathrm{\partial }{x}_1}-\kappa (\cos \alpha \frac{1}{2(2\delta +\beta )}(g_2\cos \alpha +g_3\sin \alpha )-\sin \alpha \frac{1}{2(2\delta +\beta )}(g_3\cos \alpha -g_2\sin \alpha ))))\\ & -\sin \alpha \frac{\beta \kappa \tau }{4\delta }{g}_1\\ & -\beta {\kappa }^2{\sin }^2\alpha \frac{1}{2\delta +\beta }(g_3\cos \alpha -g_2\sin \alpha )-2\beta \kappa {\sin }^2\alpha \frac{1}{2\delta +\beta }(g_3\cos \alpha -g_2\sin \alpha )-\frac{3a}{8\mu }{H}_2+\frac{4a\kappa }{\pi }\sin \alpha F_0^{\ast }\\ & +\frac{a}{2\delta +\beta }(g_2\cos \alpha +g_3\sin \alpha ),\end{array}$ (A10) $\begin{array}{r}{C}_8(t,x_1)\\ & =-\frac{1}{2\delta +\beta }(\frac{\mathrm{\partial }{g}_2}{\mathrm{\partial }t}\cos \alpha +\frac{\mathrm{\partial }{g}_3}{\mathrm{\partial }t}\sin \alpha )+\frac{\delta }{2(2\delta +\beta )}(\frac{{\mathrm{\partial }}^2{g}_2}{\mathrm{\partial }{x}_1^2}\cos \alpha +\frac{\mathrm{\partial }{g}_2}{\mathrm{\partial }{x}_1}\tau \sin \alpha +({\tau }^{\prime }{g}_2+\tau \frac{\mathrm{\partial }{g}_2}{\mathrm{\partial }{x}_1})\sin \alpha \\ & -{\tau }^2{g}_2\cos \alpha +\frac{{\mathrm{\partial }}^2{g}_3}{\mathrm{\partial }{x}_1^2}\sin \alpha -\frac{\mathrm{\partial }{g}_3}{\mathrm{\partial }{x}_1}\tau \cos \alpha -({\tau }^{\prime }{g}_3+\tau \frac{\mathrm{\partial }{g}_3}{\mathrm{\partial }{x}_1})\cos \alpha -{\tau }^2{g}_3\sin \alpha )-\delta \kappa \cos \alpha H_9+\delta \kappa \sin \alpha H_{10}\\ & +\frac{1}{4}((2\kappa \frac{\mathrm{\partial }{g}_1}{\mathrm{\partial }{x}_1}+{\kappa }^{\prime }{g}_1-\frac{2\delta {\kappa }^2}{2\delta +\beta }(g_2\cos \alpha +g_3\sin \alpha ))\cos \alpha +\kappa \tau g_1\sin \alpha )+\frac{\beta }{8\mu }\frac{\mathrm{\partial }{H}_3}{\mathrm{\partial }{x}_1}-\beta \kappa \cos \alpha H_9\\ & +\beta \kappa \sin \alpha H_{11}+\beta \cos \alpha (\frac{{\kappa }^{\prime }}{4\delta }{g}_1+{\kappa }^2\cos \alpha \frac{1}{2(2\delta +\beta )}(g_2\cos \alpha +g_3\sin \alpha )\\ & -{\kappa }^2\sin \alpha \frac{1}{2(2\delta +\beta )}(g_2\cos \alpha +g_3\sin \alpha )\\ & +2\kappa (\frac{1}{4\alpha }\frac{\mathrm{\partial }{g}_1}{\mathrm{\partial }{x}_1}-\kappa (\cos \alpha \frac{1}{2(2\delta +\beta )}(g_2\cos \alpha +g_3\sin \alpha )-\sin \alpha \frac{1}{2(2\delta +\beta )}(g_3\cos \alpha -g_2\sin \alpha ))))\\ & +\beta \kappa \tau \sin \alpha \frac{g_1}{4\delta }\\ & +\frac{a}{8\mu }{H}_2-\frac{a}{2\delta +\beta }(g_2\cos \alpha +g_3\sin \alpha ),\end{array}$(A10) and $\begin{array}{rl}& C_9(t,x_1)\\ & =\frac{1}{2(2\delta +\beta )}(\frac{\mathrm{\partial }{g}_3}{\mathrm{\partial }t}\cos \alpha -\frac{\mathrm{\partial }{g}_2}{\mathrm{\partial }t}\sin \alpha )-\frac{\delta }{2(2\delta +\beta )}(\frac{{\mathrm{\partial }}^2{g}_3}{\mathrm{\partial }{x}_1^2}\cos \alpha +\tau \frac{\mathrm{\partial }{g}_3}{\mathrm{\partial }{x}_1}\sin \alpha +({\tau }^{\prime }{g}_3+\tau \frac{\mathrm{\partial }{g}_3}{\mathrm{\partial }{x}_1})\sin \alpha \\ & -{\tau }^2{g}_3\cos \alpha \\ & -\frac{{\mathrm{\partial }}^2{g}_2}{\mathrm{\partial }{x}_1^2}\sin \alpha +\tau \frac{\mathrm{\partial }{g}_2}{\mathrm{\partial }{x}_1}\cos \alpha +({\tau }^{\prime }{g}_2+\tau \frac{\mathrm{\partial }{g}_2}{\mathrm{\partial }{x}_1})\cos \alpha +{\tau }^2{g}_2\sin \alpha )-\delta \kappa \sin \alpha H_{12}+3\delta \kappa \cos \alpha H_{11}\\ & +\frac{2\delta {\kappa }^2{\cos }^2\alpha }{2\delta +\beta }(g_3\cos \alpha -g_2\sin \alpha )-(\frac{\kappa \tau \cos \alpha }{4}{g}_1-(\frac{\kappa }{2}\frac{\mathrm{\partial }{g}_1}{\mathrm{\partial }{x}_1}+\frac{{\kappa }^{\prime }}{4}{g}_1-\frac{{\kappa }^2}{2(2\delta +\beta )}(g_2\cos \alpha +g_3\sin \alpha ))\sin \alpha )\\ & -\frac{\delta {\kappa }^2{\cos }^2\alpha }{2\delta +\beta }(g_3\cos \alpha -g_2\sin \alpha )-\frac{\beta }{8\mu }\frac{\mathrm{\partial }{H}_4}{\mathrm{\partial }{x}_1}+\beta \kappa \cos \alpha H_{10}\\ & -\beta \kappa \sin \alpha H_{12}-\beta \kappa \tau \cos \alpha \frac{1}{4\delta }{g}_1+\beta \kappa ({\kappa }^{\prime }\frac{1}{4\delta }{g}_1+{\kappa }^2\cos \alpha \frac{1}{2(2\delta +\beta )}(g_2\cos \alpha +g_3\sin \alpha )\\ & -{\kappa }^2\sin \alpha \frac{1}{2(2\delta +\beta )}(g_3\cos \alpha -g_2\sin \alpha ))-2\beta \kappa (\frac{1}{4\delta }\frac{\mathrm{\partial }{g}_1}{\mathrm{\partial }{x}_1}-\kappa (\cos \alpha \frac{1}{2(2\delta +\beta )}(g_2\cos \alpha +g_3\sin \alpha )\\ & -\frac{1}{2(2\delta +\beta )}(g_3\cos \alpha -g_2\sin \alpha )\sin \alpha ))\sin \alpha +\frac{3a}{8\mu }{H}_1+\frac{4a\kappa }{\pi }{F}_0^{\ast }\cos \alpha +\frac{a}{2\delta +\beta }(g_3\cos \alpha -g_2\sin \alpha ),\end{array}$ $\begin{array}{rl}& C_{10}(t,x_1)\\ & =-2\delta \kappa \sin \alpha H_{11}+2\delta \kappa \cos \alpha H_{12}-\frac{4\delta {\kappa }^2}{2\delta +\beta }\sin \alpha \cos \alpha (g_3\cos \alpha -g_2\sin \alpha )\\ & +2\delta {\kappa }^2\cos \alpha \sin \alpha \frac{1}{2\delta +\beta }(g_3\cos \alpha -g_2\sin \alpha )\\ & -\frac{\beta }{4\mu }\frac{\mathrm{\partial }{H}_3}{\mathrm{\partial }{x}_1}+2\beta \kappa \cos \alpha H_9-\beta \kappa \sin \alpha H_{11}-\beta {\kappa }^{\prime }\cos \alpha \frac{1}{2\delta }{g}_1-\beta \kappa \tau \sin \alpha \frac{1}{2\delta }{g}_1\\ & -\beta {\kappa }^2{\cos }^2\alpha \frac{1}{2\delta +\beta }(g_2\cos \alpha +g_3\sin \alpha )+\beta {\kappa }^2\cos \alpha \sin \alpha \frac{1}{2\delta +\beta }(g_3\cos \alpha -g_2\sin \alpha )\\ & -\beta \kappa \cos \alpha \frac{1}{\delta }\frac{\mathrm{\partial }{g}_1}{\mathrm{\partial }{x}_1}+2\beta {\kappa }^2\cos \alpha \frac{1}{2\delta +\beta }(\cos \alpha (g_2\cos \alpha +g_3\sin \alpha )+\sin \alpha (g_3\cos \alpha -g_2\sin \alpha \left)\right)\\ & +\frac{a}{4\mu }{H}_2-\frac{4a\kappa }{\pi }\sin \alpha F_0^{\ast },\end{array}$ $\begin{array}{rl}& C_{11}(t,x_1)\\ & =\frac{1}{2(2\delta +\beta )}(\frac{\mathrm{\partial }{g}_3}{\mathrm{\partial }t}\cos \alpha -\frac{\mathrm{\partial }{g}_2}{\mathrm{\partial }t}\sin \alpha )-\frac{\delta }{2(2\delta +\beta )}(\frac{{\mathrm{\partial }}^2{g}_3}{\mathrm{\partial }{x}_1^2}\cos \alpha +\tau \frac{\mathrm{\partial }{g}_3}{\mathrm{\partial }{x}_1}\sin \alpha +({\tau }^{\prime }{g}_3\\ & +\tau \frac{\mathrm{\partial }{g}_3}{\mathrm{\partial }{x}_1})\sin \alpha -{\tau }^2{g}_3\cos \alpha \\ & -\frac{{\mathrm{\partial }}^2{g}_2}{\mathrm{\partial }{x}_1^2}\sin \alpha +\tau \frac{\mathrm{\partial }{g}_2}{\mathrm{\partial }{x}_1}\cos \alpha +({\tau }^{\prime }{g}_2+\tau \frac{\mathrm{\partial }{g}_2}{\mathrm{\partial }{x}_1})\cos \alpha +{\tau }^2{g}_2\sin \alpha )-3\delta \kappa \sin \alpha H_{12}+\delta \kappa \cos \alpha H_{11}\\ & +2\delta {\kappa }^2{\sin }^2\alpha \frac{1}{2\delta +\beta }(g_3\cos \alpha -g_2\sin \alpha )-(\cos \alpha \frac{\kappa \tau }{4}{g}_1-(\frac{\kappa }{2}\frac{\mathrm{\partial }{g}_1}{\mathrm{\partial }{x}_1}+\frac{{\kappa }^{\prime }}{4}{g}_1)\sin \alpha )\\ & +\frac{{\kappa }^2}{2(2\delta +\beta )}(g_2\cos \alpha +g_3\sin \alpha )\sin \alpha -\delta {\kappa }^2{\sin }^2\alpha \frac{1}{2\delta +\beta }(g_3\cos \alpha -g_2\sin \alpha )-\frac{3\beta }{8\mu }\frac{\mathrm{\partial }{H}_4}{\mathrm{\partial }{x}_1}+3\beta \kappa \cos \alpha H_{10}\\ & -3\beta \kappa \sin \alpha H_{12}-\beta \kappa \tau \cos \alpha \frac{1}{4\delta }{g}_1+\beta \kappa ({\kappa }^{\prime }\frac{1}{4\delta }{g}_1+{\kappa }^2\cos \alpha \frac{1}{2(2\delta +\beta )}(g_2\cos \alpha +g_3\sin \alpha )\\ & -{\kappa }^2\sin \alpha \frac{1}{2(2\delta +\beta )}(g_3\cos \alpha -g_2\sin \alpha ))-2\beta \kappa (\frac{1}{4\delta }\frac{\mathrm{\partial }{g}_1}{\mathrm{\partial }{x}_1}-\kappa (\cos \alpha \frac{1}{2(2\delta +\beta )}(g_2\cos \alpha +g_3\sin \alpha )\\ & -\frac{1}{2(2\delta +\beta )}(g_3\cos \alpha -g_2\sin \alpha )\sin \alpha ))\sin \alpha +\frac{\beta {\kappa }^{\prime }}{2\delta }\sin \alpha g_1-\beta \kappa \tau \cos \alpha \frac{1}{2\delta }{g}_1\\ & -\beta {\kappa }^2\sin \alpha (-\cos \alpha \frac{1}{2\delta +\beta }(g_2\cos \alpha +g_3\sin \alpha )+\sin \alpha \frac{1}{2\delta +\beta }(g_3\cos \alpha -g_2\sin \alpha ))\\ & -2\beta \kappa \sin \alpha (-\frac{1}{2\delta }\frac{\mathrm{\partial }{g}_1}{\mathrm{\partial }{x}_1}+\kappa \frac{1}{2\delta +\beta }(\cos \alpha (g_2\cos \alpha +g_3\sin \alpha )+\sin \alpha (g_3\cos \alpha -g_2\sin \alpha )))\\ & +\frac{a}{8\mu }{H}_1+\frac{a}{2\delta +\beta }(g_3\cos \alpha -g_2\sin \alpha ),\end{array}$ (A11) $\begin{array}{rl}& C_{12}(t,x_1)\\ & =-\frac{2}{2(2\delta +\beta )}(\frac{\mathrm{\partial }{g}_3}{\mathrm{\partial }t}\cos \alpha -\frac{\mathrm{\partial }{g}_2}{\mathrm{\partial }t}\sin \alpha )+\frac{\delta }{2(2\delta +\beta )}(\frac{{\mathrm{\partial }}^2{g}_3}{\mathrm{\partial }{x}_1^2}\cos \alpha +\tau \frac{\mathrm{\partial }{g}_3}{\mathrm{\partial }{x}_1}\sin \alpha +({\tau }^{\prime }{g}_3+\tau \frac{\mathrm{\partial }{g}_3}{\mathrm{\partial }{x}_1})\sin \alpha \\ & -{\tau }^2{g}_3\cos \alpha \\ & -\frac{{\mathrm{\partial }}^2{g}_2}{\mathrm{\partial }{x}_1^2}\sin \alpha +\tau \frac{\mathrm{\partial }{g}_2}{\mathrm{\partial }{x}_1}\cos \alpha +({\tau }^{\prime }{g}_2+\tau \frac{\mathrm{\partial }{g}_2}{\mathrm{\partial }{x}_1})\cos \alpha +{\tau }^2{g}_2\sin \alpha )+\delta \kappa \sin \alpha H_{12}-\delta \kappa \cos \alpha H_{11}\\ & +(\cos \alpha \frac{\kappa \tau }{4}{g}_1-(\frac{\kappa }{2}\frac{\mathrm{\partial }{g}_1}{\mathrm{\partial }{x}_1}+\frac{{\kappa }^{\prime }}{4}{g}_1-\frac{{\kappa }^2}{2(2\delta +\beta )}(g_2\cos \alpha +g_3\sin \alpha ))\sin \alpha )+\frac{\beta }{8\mu }\frac{\mathrm{\partial }{H}_4}{\mathrm{\partial }{x}_1}-\beta \kappa \cos \alpha H_{10}\\ & +\beta \kappa \sin \alpha H_{12}+\beta \kappa \tau \cos \alpha \frac{1}{4\delta }{g}_1-\beta \kappa ({\kappa }^{\prime }\frac{1}{4\delta }{g}_1+{\kappa }^2\cos \alpha \frac{1}{2(2\delta +\beta )}(g_2\cos \alpha +g_3\sin \alpha )\\ & -{\kappa }^2\sin \alpha \frac{1}{2(2\delta +\beta )}(g_3\cos \alpha -g_2\sin \alpha ))+2\beta \kappa (\frac{1}{4\delta }\frac{\mathrm{\partial }{g}_1}{\mathrm{\partial }{x}_1}-\kappa (\cos \alpha \frac{1}{2(2\delta +\beta )}(g_2\cos \alpha +g_3\sin \alpha )\\ & -\frac{1}{2(2\delta +\beta )}\sin \alpha (g_3\cos \alpha -g_2\sin \alpha )))\sin \alpha -\frac{a}{8\mu }{H}_1-\frac{a}{2\delta +\beta }(g_3\cos \alpha -g_2\sin \alpha ).\end{array}$(A11) The functions $D_5(t,x_1),\dots ,D_{12}(t,x_1)$ in the expressions (48) for the second and third component of the microrotation second-order corrector $(W_2^2,W_2^3)$ are given as follows: (A12) $\begin{array}{rl}{D}_5(t,x_1)& =\frac{(2\delta -\frac{12{\beta }^2}{12\delta +6\beta })D_7+C_5-\frac{3\beta C_{10}}{12\delta +6\beta }}{-14\delta -12\beta +\frac{12{\beta }^2}{12\delta +6\beta }},D_6(t,x_1)=\frac{-4\beta (D_9+D_{11})-C_6}{12\delta +6\beta },\\ D_7(t,x_1)& =\frac{(C_7-\frac{3\beta C_{10}}{12\delta +6\beta })(-14\delta -12\beta +\frac{12{\beta }^2}{12\delta +6\beta })-(-2\delta -2\beta +\frac{12{\beta }^2}{12\delta +6\beta })(C_5-\frac{3\beta C_{10}}{12\delta +6\beta })}{(-2\delta -2\beta +\frac{12{\beta }^2}{12\delta +6\beta })(2\delta -\frac{12{\beta }^2}{12\delta +6\beta })+(-14\delta -2\beta +\frac{12{\beta }^2}{12\delta +6\beta }{)}^2},\\ D_8(t,x_1)& =\frac{2\delta (D_5+D_7)+2\beta D_5+\beta D_{10}-C_8}{4\alpha +2\beta },\\ D_9(t,x_1)& =\frac{C_9-\frac{3\beta C_6}{12\delta +6\beta }+(2\delta +2\beta -\frac{12{\beta }^2}{12\delta +6\beta })D_{11}}{-14\delta -2\beta +\frac{12{\beta }^2}{12\delta +6\beta }},D_{10}(t,x_1)=\frac{-4\beta (D_5+D_7)-C_{10}}{12\delta +6\beta },\\ D_{11}(t,x_1)& =\frac{(C_{11}-\frac{3\beta C_6}{12\delta +6\beta })(-14\delta -2\beta +\frac{12{\beta }^2}{12\delta +6\beta })+(2\delta -\frac{12{\beta }^2}{12\delta +6\beta })(C_9-\frac{3\beta C_6}{12\delta +6\beta })}{(-2\delta +\frac{12{\beta }^2}{12\delta +6\beta })(2\delta +2\beta -\frac{12{\beta }^2}{12\delta +6\beta })+(-14\delta -12\beta +\frac{12{\beta }^2}{12\delta +6\beta })(-14\delta -2\beta +\frac{12{\beta }^2}{12\delta +6\beta })},\\ D_{12}(t,x_1)& =\frac{2\delta (D_9+D_{11})+2\beta D_{11}+\beta D_6-C_{12}}{4\delta +2\beta }.\end{array}$(A12)

Boundary layers

In the following, we write down the exponentially decreasing functions $\mathrm{\Sigma },\mathrm{\Pi },\mathrm{\Theta }$ and Λ appearing in the equations for the velocity, pressure and microrotation first- and second-order boundary layers correctors in Sections 4.2.1 and 4.2.2 (see (54(54) $\begin{array}{rl}& -\mu \mathrm{\Delta }{\mathcal{V}}_1+\mathrm{\nabla }{\mathcal{P}}_1=\mathrm{\Xi }(t,0,{\mathcal{V}}_0,{\mathcal{W}}_0,{\mathcal{P}}_0)\text{in}{\mathcal{G}}_0,\\ & \text{div}{\mathcal{V}}_1=\kappa \left(0\right)\cos \alpha \left(0\right){\mathcal{V}}_0^2-\kappa \left(0\right)\sin \alpha \left(0\right){\mathcal{V}}_0^3\\ & -(2\kappa \left(0\right)\cos \alpha \left(0\right)y_2\frac{\mathrm{\partial }{\mathcal{V}}_0^1}{\mathrm{\partial }{y}_1}-2\kappa \left(0\right)\sin \alpha \left(0\right)y_3\frac{\mathrm{\partial }{\mathcal{V}}_0^1}{\mathrm{\partial }{y}_1}),{\mathcal{V}}_1=0\text{on}\omega =⟨0,\mathrm{\infty }⟩\times \mathrm{\partial }B,\\ & {\mathcal{V}}_1(t,0,\cdot )=-\left(V_1^1\right(t,0,\cdot ),V_1^2(t,0,\cdot ),V_1^3(t,0,\cdot \left)\right)-\left(\kappa \right(0\left)\right({\mathbf{\text{e}}}_{\alpha }\left(0\right)\cdot {\mathit{y}}_{\ast }\left)V_0^1\right(t,0,\cdot ),0,0)\\ & -\left(\kappa \right(0\left)\right({\mathbf{\text{e}}}_{\alpha }\left(0\right)\cdot {\mathit{y}}_{\ast }\left){\mathcal{V}}_0^1\right(t,0,\cdot ),0,0),\end{array}$(54) ), (57(57) $\begin{array}{rl}& -\mu \mathrm{\Delta }{\mathcal{V}}_2+\mathrm{\nabla }{\mathcal{P}}_2=\mathrm{\Pi }(t,0,{\mathcal{V}}_0,{\mathcal{V}}_1,{\mathcal{W}}_0,{\mathcal{W}}_1,{\mathcal{P}}_0,{\mathcal{P}}_1)\text{in}{\mathcal{G}}_0,\\ & \text{div}{\mathcal{V}}_2=\kappa \left(0\right)\cos \alpha \left(0\right){\mathcal{V}}_1^2-\kappa \left(0\right)\sin \alpha \left(0\right){\mathcal{V}}_1^3-\left({\kappa }^{\prime }\right(0\left)\right({\mathbf{\text{e}}}_{\alpha }\left(0\right)\cdot {\mathit{y}}_{\ast })+\kappa (0\left)\tau \right(0\left)\right({\mathbf{\text{e}}}_{\alpha }^{\perp }\left(0\right)\cdot {\mathit{y}}_{\ast }\left)\right){\mathcal{V}}_0^1\\ & -{\kappa }^2\left(0\right)\left({\mathbf{\text{e}}}_{\alpha }\right(0)\cdot {\mathit{y}}_{\ast })\cos \alpha \left(0\right){\mathcal{V}}_0^2+{\kappa }^2\left(0\right)\left({\mathbf{\text{e}}}_{\alpha }\right(0)\cdot {\mathit{y}}_{\ast })\sin \alpha \left(0\right){\mathcal{V}}_0^3\\ & +2\kappa \left(0\right)\left({\mathbf{\text{e}}}_{\alpha }\right(0)\cdot {\mathit{y}}_{\ast })(\kappa \left(0\right)\cos \alpha \left(0\right){\mathcal{V}}_0^2-\kappa \left(0\right)\sin \alpha \left(0\right){\mathcal{V}}_0^3)\\ & -2\kappa \left(0\right)\left({\mathbf{\text{e}}}_{\alpha }\right(0)\cdot {\mathit{y}}_{\ast })\frac{\mathrm{\partial }{\mathcal{V}}_1^1}{\mathrm{\partial }{y}_1}-3{\kappa }^2\left(0\right)\left({\mathbf{\text{e}}}_{\alpha }\right(0)\cdot {\mathit{y}}_{\ast }{)}^2\frac{\mathrm{\partial }{\mathcal{V}}_0^1}{\mathrm{\partial }{y}_1}\text{in}{\mathcal{G}}_0,\\ & {\mathcal{V}}_2=0\text{in}\omega =⟨0,\mathrm{\infty }⟩\times \mathrm{\partial }B,\\ & {\mathcal{V}}_2(t,0,\cdot )=-V_2(t,0,\cdot )-\left(\kappa \right({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast }\left)V_1^1\right(t,0,\cdot ),0,0)-\left({\kappa }^2\right({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast }{)}^2{V}_0^1(t,0,\cdot ),0,0)\\ & -\left(\kappa \right({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast }\left){\mathcal{V}}_1^1\right(t,0,\cdot ),0,0)-\left({\kappa }^2\right({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast }{)}^2{\mathcal{V}}_0^1(t,0,\cdot ),0,0),\end{array}$(57) ), (61(61) $\begin{array}{rl}& -\delta {\mathcal{W}}_1-\beta \mathrm{\nabla }\text{div}{\mathcal{W}}_1=\mathrm{\Theta }(t,0,{\mathcal{V}}_0,{\mathcal{W}}_0)\text{in}{\mathcal{G}}_0,\\ & {\mathcal{W}}_1=0\text{on}\omega =⟨0,\mathrm{\infty }⟩\times \mathrm{\partial }B,\\ & {\mathcal{W}}_1(t,0,\cdot )=-W_1(t,0,\cdot )-\left(\kappa \right({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast }\left)W_0^1\right(t,0,\cdot ),0,0)-\left(\kappa \right({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast }\left){\mathcal{W}}_0^1\right(t,0,\cdot ),0,0),\end{array}$(61) ) and (62(62) $\begin{array}{rl}& -\delta {\mathcal{W}}_2-\beta \mathrm{\nabla }\text{div}{\mathcal{W}}_2=\mathrm{\Lambda }(t,0,{\mathcal{V}}_0,{\mathcal{V}}_1,{\mathcal{W}}_0,{\mathcal{W}}_1)\text{in}{\mathcal{G}}_0,\\ & {\mathcal{W}}_2=0\text{on}\omega =⟨0,\mathrm{\infty }⟩\times \mathrm{\partial }B,\\ & {\mathcal{W}}_2(t,0,\cdot )=-W_2(t,0,\cdot )-\left(\kappa \right({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast }\left)W_1^1\right(t,0,\cdot ),0,0)-\left({\kappa }^2\right({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast }{)}^2{W}_0^1(t,0,\cdot ),0,0)\\ & -\left(\kappa \right({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast }\left){\mathcal{W}}_1^1\right(t,0,\cdot ),0,0)-\left({\kappa }^2\right({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast }{)}^2{\mathcal{W}}_0^1(t,0,\cdot ),0,0),\end{array}$(62) )).

Boundary layers for the velocity

The function $\mathrm{\Xi }(t,x_1,\mathbf{\text{J}},\mathbf{\text{K}},j)$ appearing in the equation (54(54) $\begin{array}{rl}& -\mu \mathrm{\Delta }{\mathcal{V}}_1+\mathrm{\nabla }{\mathcal{P}}_1=\mathrm{\Xi }(t,0,{\mathcal{V}}_0,{\mathcal{W}}_0,{\mathcal{P}}_0)\text{in}{\mathcal{G}}_0,\\ & \text{div}{\mathcal{V}}_1=\kappa \left(0\right)\cos \alpha \left(0\right){\mathcal{V}}_0^2-\kappa \left(0\right)\sin \alpha \left(0\right){\mathcal{V}}_0^3\\ & -(2\kappa \left(0\right)\cos \alpha \left(0\right)y_2\frac{\mathrm{\partial }{\mathcal{V}}_0^1}{\mathrm{\partial }{y}_1}-2\kappa \left(0\right)\sin \alpha \left(0\right)y_3\frac{\mathrm{\partial }{\mathcal{V}}_0^1}{\mathrm{\partial }{y}_1}),{\mathcal{V}}_1=0\text{on}\omega =⟨0,\mathrm{\infty }⟩\times \mathrm{\partial }B,\\ & {\mathcal{V}}_1(t,0,\cdot )=-\left(V_1^1\right(t,0,\cdot ),V_1^2(t,0,\cdot ),V_1^3(t,0,\cdot \left)\right)-\left(\kappa \right(0\left)\right({\mathbf{\text{e}}}_{\alpha }\left(0\right)\cdot {\mathit{y}}_{\ast }\left)V_0^1\right(t,0,\cdot ),0,0)\\ & -\left(\kappa \right(0\left)\right({\mathbf{\text{e}}}_{\alpha }\left(0\right)\cdot {\mathit{y}}_{\ast }\left){\mathcal{V}}_0^1\right(t,0,\cdot ),0,0),\end{array}$(54) ) is given as (A13) $\begin{array}{rl}\mathrm{\Xi }(t,x_1,\mathbf{\text{J}},\mathbf{\text{K}},j)& =\mu (\kappa \cos \alpha (\frac{\mathrm{\partial }{J}^1}{\mathrm{\partial }{y}_2}-\frac{\mathrm{\partial }{J}^2}{\mathrm{\partial }{y}_1})+\kappa \sin \alpha (\frac{\mathrm{\partial }{J}^3}{\mathrm{\partial }{y}_1}-\frac{\mathrm{\partial }{V}^1}{\mathrm{\partial }{y}_3})-\kappa (\frac{\mathrm{\partial }{J}^2}{\mathrm{\partial }{y}_1}\cos \alpha -\frac{\mathrm{\partial }{J}^3}{\mathrm{\partial }{y}_1}\sin \alpha )+{\kappa }^{\prime }\frac{\mathrm{\partial }{J}^2}{\mathrm{\partial }{y}_1}\cos \alpha \\ & -{\kappa }^{\prime }\frac{\mathrm{\partial }{J}^3}{\mathrm{\partial }{y}_1}\sin \alpha +\kappa ({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast })\mathrm{\Delta }{J}^1,-\kappa \cos \alpha \frac{\mathrm{\partial }{J}^2}{\mathrm{\partial }{y}_2}+\kappa \sin \alpha \frac{\mathrm{\partial }{J}^2}{\mathrm{\partial }{y}_3}+2\kappa \cos \alpha \frac{\mathrm{\partial }{J}^1}{\mathrm{\partial }{y}_1},\kappa \sin \alpha \frac{\mathrm{\partial }{J}^3}{\mathrm{\partial }{y}_3}\\ & -\kappa \cos \alpha \frac{\mathrm{\partial }{J}^3}{\mathrm{\partial }{y}_2}-2\kappa \sin \alpha \frac{\mathrm{\partial }{J}^1}{\mathrm{\partial }{y}_1})-(\kappa ({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast })\frac{\mathrm{\partial }j}{\mathrm{\partial }{y}_1},0,0)\\ & +a(\frac{\mathrm{\partial }{K}^3}{\mathrm{\partial }{y}_2}-\frac{\mathrm{\partial }{K}^2}{\mathrm{\partial }{y}_3},\frac{\mathrm{\partial }{K}^1}{\mathrm{\partial }{y}_3}-\frac{\mathrm{\partial }{K}^3}{\mathrm{\partial }{y}_1},\frac{\mathrm{\partial }{K}^2}{\mathrm{\partial }{y}_1}-\frac{\mathrm{\partial }{K}^1}{\mathrm{\partial }{y}_2}),\end{array}$(A13) where $\mathbf{\text{J}}=(J^1,J^2,J^3),\mathbf{\text{K}}=(K^1,K^2,K^3)$ are vector functions, j is a scalar function, $x_1\in [0,l]$ and $t\in [0,T]$.

The function $\mathrm{\Pi }(t,x_1,{\mathbf{\text{J}}}_0,{\mathbf{\text{J}}}_1,{\mathbf{\text{K}}}_0,{\mathbf{\text{K}}}_1,j_0,j_1)$ from the equation (57(57) $\begin{array}{rl}& -\mu \mathrm{\Delta }{\mathcal{V}}_2+\mathrm{\nabla }{\mathcal{P}}_2=\mathrm{\Pi }(t,0,{\mathcal{V}}_0,{\mathcal{V}}_1,{\mathcal{W}}_0,{\mathcal{W}}_1,{\mathcal{P}}_0,{\mathcal{P}}_1)\text{in}{\mathcal{G}}_0,\\ & \text{div}{\mathcal{V}}_2=\kappa \left(0\right)\cos \alpha \left(0\right){\mathcal{V}}_1^2-\kappa \left(0\right)\sin \alpha \left(0\right){\mathcal{V}}_1^3-\left({\kappa }^{\prime }\right(0\left)\right({\mathbf{\text{e}}}_{\alpha }\left(0\right)\cdot {\mathit{y}}_{\ast })+\kappa (0\left)\tau \right(0\left)\right({\mathbf{\text{e}}}_{\alpha }^{\perp }\left(0\right)\cdot {\mathit{y}}_{\ast }\left)\right){\mathcal{V}}_0^1\\ & -{\kappa }^2\left(0\right)\left({\mathbf{\text{e}}}_{\alpha }\right(0)\cdot {\mathit{y}}_{\ast })\cos \alpha \left(0\right){\mathcal{V}}_0^2+{\kappa }^2\left(0\right)\left({\mathbf{\text{e}}}_{\alpha }\right(0)\cdot {\mathit{y}}_{\ast })\sin \alpha \left(0\right){\mathcal{V}}_0^3\\ & +2\kappa \left(0\right)\left({\mathbf{\text{e}}}_{\alpha }\right(0)\cdot {\mathit{y}}_{\ast })(\kappa \left(0\right)\cos \alpha \left(0\right){\mathcal{V}}_0^2-\kappa \left(0\right)\sin \alpha \left(0\right){\mathcal{V}}_0^3)\\ & -2\kappa \left(0\right)\left({\mathbf{\text{e}}}_{\alpha }\right(0)\cdot {\mathit{y}}_{\ast })\frac{\mathrm{\partial }{\mathcal{V}}_1^1}{\mathrm{\partial }{y}_1}-3{\kappa }^2\left(0\right)\left({\mathbf{\text{e}}}_{\alpha }\right(0)\cdot {\mathit{y}}_{\ast }{)}^2\frac{\mathrm{\partial }{\mathcal{V}}_0^1}{\mathrm{\partial }{y}_1}\text{in}{\mathcal{G}}_0,\\ & {\mathcal{V}}_2=0\text{in}\omega =⟨0,\mathrm{\infty }⟩\times \mathrm{\partial }B,\\ & {\mathcal{V}}_2(t,0,\cdot )=-V_2(t,0,\cdot )-\left(\kappa \right({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast }\left)V_1^1\right(t,0,\cdot ),0,0)-\left({\kappa }^2\right({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast }{)}^2{V}_0^1(t,0,\cdot ),0,0)\\ & -\left(\kappa \right({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast }\left){\mathcal{V}}_1^1\right(t,0,\cdot ),0,0)-\left({\kappa }^2\right({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast }{)}^2{\mathcal{V}}_0^1(t,0,\cdot ),0,0),\end{array}$(57) ) reads: (A14) $\begin{array}{rl}\mathrm{\Pi }(t,x_1,{\mathbf{\text{J}}}_0,{\mathbf{\text{J}}}_1,{\mathbf{\text{K}}}_0,{\mathbf{\text{K}}}_1,j_0,j_1)& =-(\frac{\mathrm{\partial }{J}_0^1}{\mathrm{\partial }t},\frac{\mathrm{\partial }{J}_0^2}{\mathrm{\partial }t},\frac{\mathrm{\partial }{J}_0^3}{\mathrm{\partial }t})+\mu (\kappa \cos \alpha (\frac{\mathrm{\partial }{J}_1^1}{\mathrm{\partial }{y}_2}-\frac{\mathrm{\partial }{J}_1^2}{\mathrm{\partial }{y}_1})+\kappa \sin \alpha (\frac{\mathrm{\partial }{J}_1^3}{\mathrm{\partial }{y}_1}-\frac{\mathrm{\partial }{J}_1^1}{\mathrm{\partial }{y}_3})\\ & -(\kappa \cos \alpha \frac{\mathrm{\partial }{J}_1^2}{\mathrm{\partial }{y}_1}-\kappa \sin \alpha \frac{\mathrm{\partial }{J}_1^3}{\mathrm{\partial }{y}_1})+{\kappa }^{\prime }(\frac{\mathrm{\partial }{J}_1^2}{\mathrm{\partial }{y}_1}\cos \alpha -\frac{\mathrm{\partial }{J}_1^3}{\mathrm{\partial }{y}_1}\sin \alpha )\\ & -(J_0^2(\kappa \cos \alpha {)}^{\prime }-J_0^3(\kappa \sin \alpha {)}^{\prime }-J_0^2({\kappa }^{\prime }\cos \alpha {)}^{\prime }+J_0^3({\kappa }^{\prime }\sin \alpha {)}^{\prime })\\ & -{\kappa }^2{J}_0^1+\kappa ({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast })(\mathrm{\Delta }{J}_1^1-\kappa \cos \alpha \frac{\mathrm{\partial }{J}_0^1}{\mathrm{\partial }{y}_2}+\kappa \sin \alpha \frac{\mathrm{\partial }{J}_0^1}{\mathrm{\partial }{y}_3})+{\kappa }^2({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast }{)}^2\mathrm{\Delta }{J}_0^1,\\ & -\kappa \cos \alpha \frac{\mathrm{\partial }{J}_1^2}{\mathrm{\partial }{y}_2}+\kappa \sin \alpha \frac{\mathrm{\partial }{J}_1^2}{\mathrm{\partial }{y}_3}+2\kappa ({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast })(-\kappa \cos \alpha \frac{\mathrm{\partial }{J}_0^2}{\mathrm{\partial }{y}_2}+\kappa \sin \alpha \frac{\mathrm{\partial }{J}_0^2}{\mathrm{\partial }{y}_3})\\ & +(2\kappa \frac{\mathrm{\partial }{J}_1^1}{\mathrm{\partial }{y}_1}+{\kappa }^{\prime }{J}_0^1-{\kappa }^2{J}_0^2)\cos \alpha +\kappa \tau J_0^1\sin \alpha +{\kappa }^2\cos \alpha ({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast })\frac{\mathrm{\partial }{J}_0^2}{\mathrm{\partial }{y}_2}\\ & -{\kappa }^2\sin \alpha ({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast })\frac{\mathrm{\partial }{J}_0^2}{\mathrm{\partial }{y}_3},\kappa \sin \alpha \frac{\mathrm{\partial }{J}_1^3}{\mathrm{\partial }{y}_3}-\kappa \cos \alpha \frac{\mathrm{\partial }{J}_1^3}{\mathrm{\partial }{y}_2}+2\kappa ({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast })\kappa \sin \alpha \frac{\mathrm{\partial }{J}_0^3}{\mathrm{\partial }{y}_3}\\ & -2\kappa ({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast })\kappa \cos \alpha \frac{\mathrm{\partial }{J}_0^3}{\mathrm{\partial }{y}_2}+\kappa \tau J_0^1\cos \alpha -(2\kappa \frac{\mathrm{\partial }{J}_1^1}{\mathrm{\partial }{y}_1}+{\kappa }^{\prime }{J}_0^1-{\kappa }^2{J}_0^2)\sin \alpha \\ & +{\kappa }^2\cos \alpha ({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast })\frac{\mathrm{\partial }{J}_0^3}{\mathrm{\partial }{y}_2}-{\kappa }^2\sin \alpha ({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast })\frac{\mathrm{\partial }{J}_0^3}{\mathrm{\partial }{y}_3})\\ & -(\kappa ({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast })\frac{\mathrm{\partial }{j}_1}{\mathrm{\partial }{y}_1}+{\kappa }^2({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast }{)}^2\frac{\mathrm{\partial }{j}_0}{\mathrm{\partial }{y}_1},0,0)\\ & +a(\frac{\mathrm{\partial }{K}_1^3}{\mathrm{\partial }{y}_2}-\frac{\mathrm{\partial }{K}_1^2}{\mathrm{\partial }{y}_3},\frac{\mathrm{\partial }{K}_1^1}{\mathrm{\partial }{y}_3}-\frac{\mathrm{\partial }{K}_1^3}{\mathrm{\partial }{y}_1}+\kappa ({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast })(\frac{\mathrm{\partial }{K}_0^1}{\mathrm{\partial }{y}_3}-\frac{\mathrm{\partial }{K}_0^3}{\mathrm{\partial }{y}_1}),\\ & \frac{\mathrm{\partial }{K}_1^2}{\mathrm{\partial }{y}_1}-\frac{\mathrm{\partial }{K}_1^1}{\mathrm{\partial }{y}_1}+\kappa ({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast })(\frac{\mathrm{\partial }{K}_0^2}{\mathrm{\partial }{y}_1}-\frac{\mathrm{\partial }{K}_0^1}{\mathrm{\partial }{y}_2}),\end{array}$(A14) where ${\mathbf{\text{J}}}_0=(J_0^1,J_0^2,J_0^3),{\mathbf{\text{J}}}_1=(J_1^2,J_1^2,J_1^3),{\mathbf{\text{K}}}_0=(K_0^1,K_0^2,K_0^3),{\mathbf{\text{K}}}_1=(K_1^1,K_1^2,K_1^3)$ are vector functions, $j_0,j_1$ are scalar functions, $x_1\in [0,l]$ and $t\in [0,T]$.

Boundary layers for the microrotation

The function $\mathrm{\Theta }(t,x_1,\mathbf{\text{J}},\mathbf{\text{K}})$ in the equation (61(61) $\begin{array}{rl}& -\delta {\mathcal{W}}_1-\beta \mathrm{\nabla }\text{div}{\mathcal{W}}_1=\mathrm{\Theta }(t,0,{\mathcal{V}}_0,{\mathcal{W}}_0)\text{in}{\mathcal{G}}_0,\\ & {\mathcal{W}}_1=0\text{on}\omega =⟨0,\mathrm{\infty }⟩\times \mathrm{\partial }B,\\ & {\mathcal{W}}_1(t,0,\cdot )=-W_1(t,0,\cdot )-\left(\kappa \right({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast }\left)W_0^1\right(t,0,\cdot ),0,0)-\left(\kappa \right({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast }\left){\mathcal{W}}_0^1\right(t,0,\cdot ),0,0),\end{array}$(61) ) takes the following form: (A15) $\begin{array}{rl}\mathrm{\Theta }(t,x_1,\mathbf{\text{J}},\mathbf{\text{K}})& =\delta (\kappa \cos \alpha (\frac{\mathrm{\partial }{K}^1}{\mathrm{\partial }{y}_2}-\frac{\mathrm{\partial }{K}^2}{\mathrm{\partial }{y}_1})+\kappa \sin \alpha (\frac{\mathrm{\partial }{K}^3}{\mathrm{\partial }{y}_1}-\frac{\mathrm{\partial }{K}^1}{\mathrm{\partial }{y}_3})-\kappa (\frac{\mathrm{\partial }{K}^2}{\mathrm{\partial }{y}_1}\cos \alpha -\frac{\mathrm{\partial }{K}^3}{\mathrm{\partial }{y}_1}\sin \alpha )\\ & +{\kappa }^{\prime }(\frac{\mathrm{\partial }{K}^2}{\mathrm{\partial }{y}_1}\cos \alpha -\frac{\mathrm{\partial }{K}^3}{\mathrm{\partial }{y}_1}\sin \alpha )+\kappa ({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast })\mathrm{\Delta }{K}^1,-\kappa \cos \alpha \frac{\mathrm{\partial }{K}^2}{\mathrm{\partial }{y}_2}+\kappa \sin \alpha \frac{\mathrm{\partial }{K}^2}{\mathrm{\partial }{y}_3}+2\kappa \cos \alpha \frac{\mathrm{\partial }{K}^1}{\mathrm{\partial }{y}_1},\\ & \kappa \sin \alpha \frac{\mathrm{\partial }{K}^3}{\mathrm{\partial }{y}_3}-\kappa \cos \alpha \frac{\mathrm{\partial }{K}^3}{\mathrm{\partial }{y}_2}-2\kappa \sin \alpha \frac{\mathrm{\partial }{K}^1}{\mathrm{\partial }{y}_1})+\beta (-\kappa \cos \alpha \frac{\mathrm{\partial }{K}^2}{\mathrm{\partial }{y}_1}+\kappa \sin \alpha \frac{\mathrm{\partial }{K}^3}{\mathrm{\partial }{y}_1},\\ & -\kappa (\frac{\mathrm{\partial }{K}^2}{\mathrm{\partial }{y}_2}\cos \alpha -\frac{\mathrm{\partial }{K}^3}{\mathrm{\partial }{y}_2}\sin \alpha )+2\kappa \cos \alpha \frac{\mathrm{\partial }{K}^1}{\mathrm{\partial }{y}_1},-\kappa (\frac{\mathrm{\partial }{K}^2}{\mathrm{\partial }{y}_3}\cos \alpha -\frac{\mathrm{\partial }{K}^3}{\mathrm{\partial }{y}_3}\sin \alpha )-2\kappa \sin \alpha \frac{\mathrm{\partial }{K}^1}{\mathrm{\partial }{y}_1})\\ & +a(\frac{\mathrm{\partial }{J}^3}{\mathrm{\partial }{y}_2}-\frac{\mathrm{\partial }{J}^2}{\mathrm{\partial }{y}_3},\frac{\mathrm{\partial }{J}^1}{\mathrm{\partial }{y}_3}-\frac{\mathrm{\partial }{J}^3}{\mathrm{\partial }{y}_1},\frac{\mathrm{\partial }{J}^2}{\mathrm{\partial }{y}_1}-\frac{\mathrm{\partial }{J}^1}{\mathrm{\partial }{y}_2}),\end{array}$(A15) where $\mathbf{\text{J}}=(J^1,J^2,J^3)$, $,\mathbf{\text{K}}=(K^1,K^2,K^3)$ are vector functions, $x_1\in [0,l]$ and $t\in [0,T]$.

The function $\mathrm{\Lambda }(t,x_1,{\mathbf{\text{J}}}_0,{\mathbf{\text{J}}}_1,{\mathbf{\text{K}}}_0,{\mathbf{\text{K}}}_1)$ from the equation (62(62) $\begin{array}{rl}& -\delta {\mathcal{W}}_2-\beta \mathrm{\nabla }\text{div}{\mathcal{W}}_2=\mathrm{\Lambda }(t,0,{\mathcal{V}}_0,{\mathcal{V}}_1,{\mathcal{W}}_0,{\mathcal{W}}_1)\text{in}{\mathcal{G}}_0,\\ & {\mathcal{W}}_2=0\text{on}\omega =⟨0,\mathrm{\infty }⟩\times \mathrm{\partial }B,\\ & {\mathcal{W}}_2(t,0,\cdot )=-W_2(t,0,\cdot )-\left(\kappa \right({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast }\left)W_1^1\right(t,0,\cdot ),0,0)-\left({\kappa }^2\right({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast }{)}^2{W}_0^1(t,0,\cdot ),0,0)\\ & -\left(\kappa \right({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast }\left){\mathcal{W}}_1^1\right(t,0,\cdot ),0,0)-\left({\kappa }^2\right({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast }{)}^2{\mathcal{W}}_0^1(t,0,\cdot ),0,0),\end{array}$(62) ) reads: $\begin{array}{rl}\mathrm{\Lambda }(t,x_1,{\mathbf{\text{J}}}_0,{\mathbf{\text{J}}}_1,{\mathbf{\text{K}}}_0,{\mathbf{\text{K}}}_1)& =-(\frac{\mathrm{\partial }{K}_0^1}{\mathrm{\partial }t},\frac{\mathrm{\partial }{K}_0^2}{\mathrm{\partial }t},\frac{\mathrm{\partial }{K}_0^3}{\mathrm{\partial }t})+\delta (\kappa \cos \alpha (\frac{\mathrm{\partial }{K}_1^1}{\mathrm{\partial }{y}_2}-\frac{\mathrm{\partial }{K}_1^2}{\mathrm{\partial }{y}_1})+\kappa \sin \alpha (\frac{\mathrm{\partial }{K}_1^3}{\mathrm{\partial }{y}_1}-\frac{\mathrm{\partial }{K}_1^1}{\mathrm{\partial }{y}_3})\\ & -(\kappa \cos \alpha \frac{\mathrm{\partial }{K}_1^2}{\mathrm{\partial }{y}_1}-\kappa \sin \alpha \frac{\mathrm{\partial }{K}_1^3}{\mathrm{\partial }{y}_1})+{\kappa }^{\prime }(\frac{\mathrm{\partial }{J}_1^2}{\mathrm{\partial }{y}_1}\cos \alpha -\frac{\mathrm{\partial }{J}_1^3}{\mathrm{\partial }{y}_1}\sin \alpha )\\ & -(K_0^2(\kappa \cos \alpha {)}^{\prime }-K_0^3(\kappa \sin \alpha {)}^{\prime }-J_0^2({\kappa }^{\prime }\cos \alpha {)}^{\prime }+J_0^3({\kappa }^{\prime }\sin \alpha {)}^{\prime })-{\kappa }^2{K}_0^2\\ & +\kappa ({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast })(\mathrm{\Delta }{K}_1^1-\kappa \cos \alpha \frac{\mathrm{\partial }{K}_0^1}{\mathrm{\partial }{y}_2}+\kappa \sin \alpha \frac{\mathrm{\partial }{K}_0^1}{\mathrm{\partial }{y}_3})+{\kappa }^2({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast }{)}^2\mathrm{\Delta }{K}_0^1,\\ & -\kappa \cos \alpha \frac{\mathrm{\partial }{K}_1^2}{\mathrm{\partial }{y}_2}+\kappa \sin \alpha \frac{\mathrm{\partial }{K}_1^2}{\mathrm{\partial }{y}_3}+2\kappa ({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast })(-\kappa \cos \alpha \frac{\mathrm{\partial }{K}_0^2}{\mathrm{\partial }{y}_2}+\kappa \sin \alpha \frac{\mathrm{\partial }{K}_0^2}{\mathrm{\partial }{y}_3})\\ & +(2\kappa \frac{\mathrm{\partial }{K}_1^1}{\mathrm{\partial }{y}_1}+{\kappa }^{\prime }{K}_0^1-{\kappa }^2{K}_0^2)\cos \alpha +\kappa \tau K_0^1\sin \alpha +{\kappa }^2\cos \alpha ({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast })\frac{\mathrm{\partial }{K}_0^2}{\mathrm{\partial }{y}_2}\\ & -{\kappa }^2\sin \alpha ({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast })\frac{\mathrm{\partial }{K}_0^2}{\mathrm{\partial }{y}_3},\kappa \sin \alpha \frac{\mathrm{\partial }{K}_1^3}{\mathrm{\partial }{y}_3}-\kappa \cos \alpha \frac{\mathrm{\partial }{K}_1^3}{\mathrm{\partial }{y}_2}+2\kappa ({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast })\kappa \sin \alpha \frac{\mathrm{\partial }{K}_0^3}{\mathrm{\partial }{y}_3}\\ & -2\kappa ({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast })\kappa \cos \alpha \frac{\mathrm{\partial }{K}_0^3}{\mathrm{\partial }{y}_2}+\kappa \tau K_0^1\cos \alpha -(2\kappa \frac{\mathrm{\partial }{K}_1^1}{\mathrm{\partial }{y}_1}+{\kappa }^{\prime }{K}_0^1-{\kappa }^2{K}_0^2)\sin \alpha \\ & +{\kappa }^2\cos \alpha ({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast })\frac{\mathrm{\partial }{K}_0^3}{\mathrm{\partial }{y}_2}-{\kappa }^2\sin \alpha ({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast })\frac{\mathrm{\partial }{K}_0^3}{\mathrm{\partial }{y}_3})\end{array}$ $\begin{array}{rl}& +\beta (-(\kappa \cos \alpha {)}^{\prime }{K}_0^2-\kappa \cos \alpha \frac{\mathrm{\partial }{K}_1^2}{\mathrm{\partial }{y}_1}+(\kappa \sin \alpha {)}^{\prime }{K}_0^3+\kappa \sin \alpha \frac{\mathrm{\partial }{K}_1^3}{\mathrm{\partial }{y}_1}\\ & +\kappa ({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast })(-\kappa \cos \alpha \frac{\mathrm{\partial }{K}_0^2}{\mathrm{\partial }{y}_1}+\kappa \sin \alpha \frac{\mathrm{\partial }{K}_0^3}{\mathrm{\partial }{y}_1}),-\kappa (\frac{\mathrm{\partial }{K}_1^2}{\mathrm{\partial }{y}_2}\cos \alpha -\frac{\mathrm{\partial }{K}_1^3}{\mathrm{\partial }{y}_2}\sin \alpha )\\ & +({\kappa }^{\prime }{K}_0^1+{\kappa }^2{K}_0^2-{\kappa }^2\sin \alpha K_0^3+2\kappa (\frac{\mathrm{\partial }{K}_1^1}{\mathrm{\partial }{y}_1}-\kappa (K_0^2\cos \alpha -K_0^3\sin \alpha )))\cos \alpha \\ & +\kappa \tau K_0^1\sin \alpha +{\kappa }^{\prime }({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast })\frac{\mathrm{\partial }{K}_1^1}{\mathrm{\partial }{y}_2}+\kappa \tau ({\mathbf{\text{e}}}_{\alpha }^{\perp }\cdot {\mathit{y}}_{\ast })\frac{\mathrm{\partial }{K}_1^1}{\mathrm{\partial }{y}_2}+{\kappa }^2({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast })\frac{\mathrm{\partial }{K}_0^2}{\mathrm{\partial }{y}_2}\cos \alpha \\ & -{\kappa }^2({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast })\frac{\mathrm{\partial }{K}_0^3}{\mathrm{\partial }{y}_2}\sin \alpha +2\kappa ({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast })(\frac{{\mathrm{\partial }}^2{K}_1^1}{\mathrm{\partial }{y}_1\mathrm{\partial }{y}_2}-\kappa (\frac{\mathrm{\partial }{K}_0^2}{\mathrm{\partial }{y}_2}\cos \alpha -\frac{\mathrm{\partial }{K}_0^3}{\mathrm{\partial }{y}_2}\sin \alpha )),\\ & -\kappa (\frac{\mathrm{\partial }{K}_1^2}{\mathrm{\partial }{y}_3}\cos \alpha -\frac{\mathrm{\partial }{K}_1^3}{\mathrm{\partial }{y}_3}\sin \alpha )+\kappa \tau K_0^1\cos \alpha -({\kappa }^{\prime }{K}_0^1+{\kappa }^2\cos \alpha K_0^2-{\kappa }^2\sin \alpha K_0^3\\ & +2\kappa (\frac{\mathrm{\partial }{K}_1^1}{\mathrm{\partial }{y}_1}-\kappa (K_0^2\cos \alpha -K_0^3\sin \alpha )))\sin \alpha +{\kappa }^{\prime }({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast })\frac{\mathrm{\partial }{K}_0^1}{\mathrm{\partial }{y}_3}+\kappa \tau ({\mathbf{\text{e}}}_{\alpha }^{\perp }\cdot {\mathit{y}}_{\ast })\frac{\mathrm{\partial }{K}_0^1}{\mathrm{\partial }{y}_3}\end{array}$ (A16) $\begin{array}{rl}& +{\kappa }^2({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast })(\cos \alpha \frac{\mathrm{\partial }{K}_0^2}{\mathrm{\partial }{y}_3}-\sin \alpha \frac{\mathrm{\partial }{K}_0^2}{\mathrm{\partial }{y}_3})+2\kappa ({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast })\frac{{\mathrm{\partial }}^2{K}_1^1}{\mathrm{\partial }{y}_1\mathrm{\partial }{y}_3}\\ & -2{\kappa }^2({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast })(\frac{\mathrm{\partial }{K}_0^2}{\mathrm{\partial }{y}_3}\cos \alpha -\frac{\mathrm{\partial }{K}_0^3}{\mathrm{\partial }{y}_3}\sin \alpha ))+2a(K_0^1,K_0^2,K_0^3)\\ & +a(\frac{\mathrm{\partial }{J}_1^3}{\mathrm{\partial }{y}_2}-\frac{\mathrm{\partial }{J}_1^2}{\mathrm{\partial }{y}_3},\frac{\mathrm{\partial }{J}_1^1}{\mathrm{\partial }{y}_3}-\frac{\mathrm{\partial }{J}_1^3}{\mathrm{\partial }{y}_1}+\kappa ({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast })(\frac{\mathrm{\partial }{J}_0^1}{\mathrm{\partial }{y}_3}-\frac{\mathrm{\partial }{J}_0^3}{\mathrm{\partial }{y}_1}),\frac{\mathrm{\partial }{J}_1^2}{\mathrm{\partial }{y}_1}-\frac{\mathrm{\partial }{J}_1^1}{\mathrm{\partial }{y}_2}\\ & +\kappa ({\mathbf{\text{e}}}_{\alpha }\cdot {\mathit{y}}_{\ast })(\frac{\mathrm{\partial }{J}_0^2}{\mathrm{\partial }{y}_1}-\frac{\mathrm{\partial }{J}_0^1}{\mathrm{\partial }{y}_2})),\end{array}$(A16) where ${\mathbf{\text{J}}}_0=(J_0^1,J_0^2,J_0^3),{\mathbf{\text{J}}}_1=(J_1^2,J_1^2,J_1^3),{\mathbf{\text{K}}}_0=(K_0^1,K_0^2,K_0^3),{\mathbf{\text{K}}}_1=(K_1^1,K_1^2,K_1^3)$ are vector functions, $x_1\in [0,l]$ and $t\in [0,T]$.