Intuitionist and Classical Dimensions of Hegel’s Hybrid Logic

Abstract

Hegel interpreters commonly reject attempts to situate Hegel’s logic in relation to modern movements. Appealing to his criticisms of the logic of Verstand or mere understanding with its fixed logical structure, Hegel’s logic, it is pointed out, was a logic of Vernunft or reason—a logic more at home in the thought of Plato and Aristotle than in modern mathematical forms. Contesting this implied dichotomy, it is here argued that the ancient roots of Hegel’s logic, especially as transmitted by late Neopythagorean/Neoplatonic thinkers such as Proclus, gave it many features similar to ones later found in the type of algebraic transformation of Aristotle, started first by Leibniz, reanimated by Boole in the mid-nineteenth century and then developed by others such as C. S. Peirce and Arend Heyting. In particular, the ancient mathematics upon which Hegel had drawn allowed him to anticipate an answer to the criticism that Frege would later aim at Boole, concerning his inability to unite opposed class and propositional calculi. Hegel’s logic would be a hybrid, incorporating features found later in intuitionist and classical logic, but it could be so because of the way he had called upon the mathematics of the ancient Platonist tradition.

KEYWORDS:

• Hegel
• Plato
• logic
• geometry
• intuitionism

1. Introduction

Does Hegel’s The Science of Logic (Hegel 2010) have any relation to or relevance for what is now known as ‘the science of logic’? Here a negative answer is as likely to be endorsed by many contemporary Hegel scholars as it is by mainstream analytic philosophers, but, of course, with different intent. The Hegel interpreters are likely to dismiss the relevance of the contemporary science of logic for philosophy, damning it as committed to what Hegel criticised as ‘the abstract understanding’, der blosse Verstand. Mainstream analysts, in contrast, will use this to dismiss Hegel’s philosophical claims, as analytic philosophy is widely seen as having revolutionised philosophy’s method in the wake of the modernisation of logic by Gottlob Frege and Bertrand Russell.

Russell first learnt of the work of Frege in 1900, and I suggest that it is wise to harbour suspicions about ‘revolutions’, like this one, that hinge on years that end in two zeroes. In both mathematics and logic, for example, the nineteenth century had been a century of immense progress, while in comparison, the mathematical advances on which Russell had drawn in elaborating Frege’s Begriffsschrift had been comparatively narrow. The logic prized by ‘analytic’ philosophy had, appropriately, grown out of the mathematical discipline of ‘analysis’ contributed to by mathematicians such as Cauchy, Weierstrass, Cantor and Dedekind in attempts to overcome problems in the foundations of calculus by giving rigorous definitions to intuitive notions like those of number, continuity and limit. However, analysis had been just one area of development in mathematics in the nineteenth century, with major developments having also taken place in both algebra and geometry, especially when combined in novel ways, as in disciplines such as projective geometry, a form of mathematics with roots deep within ancient Greek culture.

Moreover, one of the first significant expressions of the characteristically ‘abstract’ type of algebra in that century (Parshall 2008) had been the movement within mathematical logic that tends to be overlooked in the wake of the Frege–Russell ‘revolution’—the ‘algebra of logic’ initiated in mid-century by the British mathematicians George Boole and Augustus de Morgan, seemingly unaware of Leibniz’s earlier version in the seventeenth century. In this tradition, algebra, now ‘disinterpreted’ and so generalised beyond the domain of arithmetic,¹ had been applied to the Aristotelian syllogistic. While limited in its Boolean form, this tradition had been developed by others, especially Charles Sanders Peirce, in ways that, as has been argued by Hilary Putnam for example, paralleled the technical achievements of Frege.²

Significantly, Peirce’s approach to logical categories have been likened to Hegel’s (Stern 2013) and Peirce himself had sensed parallels between Hegel’s logical thought and the new geometry inspiring his own logic: ‘Most of what is true in Hegel is a darkling glimmer of a conception which the mathematicians had long before made pretty clear, and which recent researches have still further illustrated’ (Peirce 1992, 296). Significantly, neither Whitehead nor Russell themselves had sprung from the earth as fully fledged ‘analysts’. Whitehead’s first major book, A Treatise on Universal Algebra (Whitehead 1898), summed up the nineteenth-century developments of abstract algebra with a strong emphasis on geometry, while Russell, in his first work, An Essay on the Foundations of Geometry (Russell 1897), had drawn together Hegel’s treatment of space in his Philosophy of Nature with the philosophical attitude expressed within these new forms of geometric algebra.

While the Hegelian/geometric framework sketched in Russell’s early work was quickly abandoned, I want to examine the idea that Hegel’s ‘logic’ may indeed have had features that fitted these algebraic and geometric developments of the nineteenth century that had roots within areas of Greek mathematics with which Hegel had been familiar. Such features in turn bring it into relation with more recent logics, especially the logic associated with intuitionism, the mathematical movement started by the Dutch mathematician L. E. J. Brouwer, who was critical of the logicism of Whitehead and Russell.³ Hegel is, of course, known for his non-acknowledgement of the classical laws of logic, the laws of non-contradiction and excluded middle. I will be arguing that, like the intuitionistic variant of Boolean logic, Hegel rejected the law of excluded middle as a type of logical axiom but allowed it to apply, along with the law of non-contradiction, in certain restricted contexts. These would reflect the somewhat ‘hybrid’ nature of his logic that combined classical and intuitionistic features,⁴ but for Hegel the capacity to integrate these opposed logical forms would be related to the ways in which ancient mathematics had informed Plato’s conception in the Timaeus of the ‘beautiful bond’ responsible for the coherence of the cosmos (Plato 1997, 31b−33c)—a structure on which Hegel modelled his own logic.

2. The Pre-Analytic Russell, Hegel, and Geometry

Nicholas Griffin, in a study of early drafts of a paper published by Russell in 1901 (Griffin 2013), noted that for a very short period after the encounter with Guiseppe Peano in 1900 when he learnt of the work of Frege, Russell had flirted with a logic based on the algebraic theory of groups. In the 1830s, group theory, a form of abstract algebra, had arisen in relation to the theorisation of solutions to polynomial equations and in the 1870s Felix Klein in his ‘Erlangen Program’ had applied it to geometry so as to classify the various new forms of geometry that had developed during the nineteenth century. Griffin speculates (Griffin 2013, 378):

With the considerable advantages of hindsight, we can see now that group theory might have done as well as set theory. From the point of view of getting a clear conception of the basic ideas of mathematics, group theory has much to commend it, perhaps as much as set theory. […] To someone coming to the project, as Russell was, from geometry, it could well seem that group theory had the advantage, since it had already considerable successes to its credit, especially in Klein’s hands, whereas the effects of Cantorean set theory in geometry were problematic to say the least.

Group theory would be replaced by Cantor’s set theory in Russell’s logicist project of seeking the logical foundations of mathematics, an occurrence Griffin puts down to various factors. One was the idea of conceptual analysis that Russell had come to adopt following Moore, in which analysis was understood as the ‘decomposition of complex terms (propositions) into their simpler constituents’ (Griffin 2013, 384).

Such a ‘decompositional’ conception of analysis as described by Michael Beaney (Beaney 2007), had been starkly missing from both group theory and from Russell’s first venture into foundational issues in mathematics in An Essay on the Foundations of Geometry in 1897. The form of geometry in question in this work was projective geometry, a type of geometry that had been proposed in the seventeenth century by Girard Desargues and a few others as a counter to the ‘analytic geometry’ of Descartes (Field and Gray 1987). In analytic geometry, figures from Euclidean geometry could be reduced to variable-containing equations by the application of coordinates to the plane in which such figures were constructed.⁵ This meant that a line was essentially decomposable into an infinite set of points—Descartes’ ‘analytic’ geometry clearly being prototypical of later forms of ‘decompositional’ analysis (Beaney 2007, 2). In this way, Descartes had effectively reversed the approach found in Aristotle almost two thousand years before, in which a line was conceived as a fundamentally continuous magnitude, and a point was determined as the place where two lines intersected.

In contrast to Descartes’ analytic geometry, Desargues’ projective geometry was non-metrical and had developed from ancient Greek precursors as well as Renaissance theories of perspectival representation in painting (Andersen 2007, chs. 1–5). Harold Coxeter has characterised projective geometry and its relation to this background as follows: ‘Plane projective geometry may be described as the study of geometrical properties that are unchanged by ‘central projection', which is essentially what happens when an artist draws a picture of a tiled floor on a vertical canvas. The square tiles cease to be square, as their sides and angles are distorted by foreshortening; but the lines remain straight, since they are sections (by the picture-plane) of the planes that join them to the artist’s eye’ (Coxeter 1987, 3). In short, for projective geometry, the ‘invariance’ of crucial factors assumed within Euclidean geometry, such as the distance between two points, or the angle formed by two intersecting lines, no longer held. But if the geometer cannot rely on such invariances, what can be relied upon? Something needs to remain constant among projections for there to be geometrical truths. But while determinate distances or angles were not preserved, certain ‘second-order’ relations among such relations were. Crucial here was a peculiar double-ratio existing between two pairs of colinear points on a line called the ‘cross-ratio’. It turns out that if four concurrent lines meeting at some external point O are drawn through those four points, then that cross-ratio will be reproduced within any other line sectioning those four lines regardless of the angle at which it crosses them.⁶ The cross-ratio would thus turn out to have a central role within projective geometry because it is ‘projectively invariant’. We will return to this phenomenon as this double-ratio will have a peculiar significance for Hegel’s logic.

While Desargues’ geometry had been eclipsed by the success of Descartes’ analytic geometry during the seventeenth and eighteenth centuries, it had been rediscovered and developed in France in the first decades of the nineteenth (Gray 2007). It would be taken up by German mathematicians, including Klein, in the second half of the century, and from there would feed into the complex new forms of geometry utilised by Einstein, for example, in his general theory of relativity.

Within projective geometry, the relation of point and line came to be conceived in a new way that had elements of both Descartes’ analytic and Aristotle’s synthetic approaches. In projective geometry parallel lines could be conceived of as meeting at some infinitely distant point, as in a point on the horizon in a perspectival painting, and so any two lines (now including parallel ones) could be conceived as determining a single point by their intersection, just as any two points could be conceived as determining the single line joining them. This resulted in the idea of a type of primitive equivalence and interchangeability as holding between points and lines, captured by the notion of ‘duality’.⁷ This duality would come to play an important part in nineteenth-century algebraic logic, and Hegel, in his ‘subjective logic’ in the Science of Logic book 3, would employ a corresponding duality in which a type of equivalence is posited between two otherwise opposed judgment forms.

Russell’s 1897 essay had been written during a period in which he adhered to some kind of mixture of Kantian and Hegelian philosophical ideas common at Cambridge at the time. Thus, in the Preface of the Essay, while acknowledging his ‘chief obligation’ as being to Felix Klein,⁸ the idealist dimensions of his approach are signalled in his acknowledgement of the approach to logic of Bradley and Bosanquet and the dedication to McTaggart. While there is only one reference to Hegel (Russell 1897, 138 n.2), it is clear that in terms of ideas concerning the relation of geometry to space, his approach is distinctly Hegelian. This comes out especially in his criticism of Kant’s ultimately ‘subjectivist’ approach to the domain of geometry as that of ‘pure intuition’.

Calling upon the mathematician Hermann Grassmann’s ‘profound philosophical introduction’ to his Linear Extension Theory of 1844 (Grassmann 1995), a type of hybrid of algebra and geometry similar to that found in projective geometry, Russell notes that he had suggested that ‘Geometry, although improperly regarded as pure, was really a branch of applied mathematics, since it dealt with a subject-matter not created, like number, by the intellect, but given to it, and therefore not wholly subject to its laws alone’ (Russell 1897, 134). This ‘given’ was space itself, qua medium of extended objects arranged within it. Nevertheless, Grassmann had believed it possible ‘to construct a branch of pure mathematics, a science, that is, in which our object should be wholly a creature of the intellect, which should yet deal, as Geometry does, with extension—extension as conceived, however, not as empirically perceived in sensation or intuition’ (Russell 1897, 134). Grassmann had called this ‘Extension Theory’ (Ausdehnungslehre) and indeed others have believed that Grassmann, a student at the University of Berlin during Hegel’s period there, had been deeply influenced by Hegel’s Science of Logic.⁹ Russell does not mention Hegel at this point, but the Hegelian dimension of the move that this effects with respect to Kant’s conception of geometry is clear. Kant’s ‘distinction between the subjective and the objective’ (Russell 1897, 135) or intuition and concept had, with Grassmann, effectively been moved within the sphere of the intellect to enable a notion of pure extension to be explored entirely conceptually.¹⁰ Hegel had responded to Kant’s ‘subjective idealism’ in much the same way, incorporating the relation between what Kant took to be between concepts and the non-conceptual form of ‘intuitive’ cognition into the realm of concepts.

The point to be emphasised here, however, is the rejection of Descartes’ analytic reduction of the geometric continuum into discrete points that results from Russell’s embrace of the ‘qualitative’ nature of projective geometry. Externality, Russell points out, is an essentially relative conception: ‘nothing can be external to itself. To be external to something is to be another with some relation to that thing’ (Russell 1897, 136) and hence ‘a position can have no intrinsic quality’ (137). In extensive magnitudes what are summed are not points but relations: ‘our form contains neither elements nor totality, but only endless relations—the terms of these relations being excluded by our abstraction from the matter which fills our form’. But these are relations without prior existing relata. ‘One of the strangest properties’ of the form of externality is that the summing of those infinite ‘would-be elements’ of which it is made up involves the summation of relations. While ‘to speak of dividing or adding relations may well sound absurd’ it is nevertheless difficult ‘to find an expression which shall be less improper. The fact seems to be, that externality is not so much a relation as bare relativity, or the bare possibility of a relation’ (138). At this point Russell refers in a footnote to a paragraph from Hegel’s Philosophy of Nature describing space, qua ‘primary or immediate determination of nature’ as ‘the abstract universality of its self-externality, its unmediated indifference. […] space is simply continuous, and is devoid of any determinate difference’ (Hegel 1970, § 254). But this is only the immediate determination of space, and two paragraphs later Hegel notes how the concept of space must be differentiated so to be ‘determinate and quantitative’. While the point is ‘the negation of the immediate and undifferentiated self-externality of space itself’, the determining self-sublating of the point is the line (§ 256 and Remark). Russell is quite explicit to the effect that this is the way he will enlarge upon the subject in the final chapter of his book (Russell 1897, 138).

3. Hegel, Geometry and Logic

The projective geometry discussed by Russell had for the most part post-dated Hegel, but it is clear that Hegel was familiar with at least its general features. For example, he knew well the work of Johannes Kepler (Hegel 2002), who had helped introduce projective ideas into geometry in the seventeenth century (Field and Gray 1987), and that of the Swiss mathematician Johann Heinrich Lambert (Hegel 2010, 544), who had pursued a form of projective geometry in the eighteenth (Andersen 2007, ch. 12). Furthermore, Hegel had apparently become familiar with Greek mathematics via the histories transmitted by late Neopythagorean and Neoplatonic writers such as Nicomachus of Gerasa and Proclus.¹¹ Both of these Neoplatonists had linked the ‘beautiful bond’ of Plato’s Timaeus to the cross-ratio via a configuration of numbers called the musical tetractys.¹²

Hegel was aware, moreover, of the reintroduction of projective geometry in his own time, having in his library what was apparently the first book to reintroduce it in the nineteenth century (Mense 1993, 673). This was a German translation published in 1803 of De la corrélation des figures de géométrie by Lazare Carnot published two years before (Carnot 1801). Carnot, a mathematician and military engineer, had been a hero of the French Revolution and the subsequent revolutionary wars and in his book had re-introduced a specific and essentially prototypical instance of the cross-ratio called the harmonic cross-ratio as found earlier in Desargues and in late antiquity in the work of Pappus of Alexandria. While Hegel never mentions Carnot’s book on geometry, in The Science of Logic he relies heavily on an earlier work when discussing the problem of infinitesimals in the calculus of Leibniz and Newton (Hegel 2010, 218−219). This was Carnot’s Reflexions sur la metaphysique du calcul infinitesimal published in 1797 that Hegel also possessed (Mense 1993, 682). Carnot’s response to the problem of infinitesimals itself drew on ancient geometry, and Carnot himself had regarded his subsequent geometric work as a development of his solution to the problem of infinitesimals (Schubring 2005, 325).

Carnot’s approach to the problem of infinitesimals in Reflexions was much like that of Russell in Foundations to the analogous problem of points in which he treated the form of extensive magnitudes as containing ‘neither elements nor totality, but only endless relations—the terms of these relations being excluded by our abstraction from the matter which fills our form’ (Russell 1897, 137). According to Carnot, infinitesimal quantities are ‘introduced in the calculus solely to facilitate the expression of the conditions proposed. It is clear that it is absolutely necessary to eliminate them from the calculation to obtain the desired result, that is, the ratios or relations (rapports) sought’ (Carnot 1797, 30). These relations sought are what are symbolised in Leibniz’s notation by $\frac{dy}{dx}$, that Carnot interprets as a ratio or relation more fundamental than the supposedly infinitesimally small magnitudes being related.

With this Carnot had been drawing upon the ancient geometric approach to ratios started by Eudoxus of Cnidus, a mathematician within Plato’s early academy, thought to be responsible for the contents of Euclid’s Elements Book V (Heath 1921, 325−326). Eudoxus had addressed the problem of ‘incommensurable magnitudes’—the discovery of irrational numbers that had been a consequence of Pythagoras’ theorem—by asserting that ratios between continuous magnitudes such as line-segments could be determinate without numerical specification. One development of this Eudoxean approach had been the attempt to find three mutually defining ratios based on the idea of the unity of the three ‘means’ found in Pythagorean music theory.¹³ Hegel seems to have been aware of the basic shape of this history as revealed in his discussion of the category ‘ratio’ in the Science of Logic (Hegel 2010, Bk. I, Sect. I, Ch. 3). There he describes attempts to specify the nature of a ratio starting with ‘direct ratio’, the simple conception of a ratio as composed of independent discrete numbers. On this model, a ratio is dependent for its determinate value on other quanta (the natural numbers), but Hegel is after a determinate quantum that is self-sufficient, and the sequence concludes with the ‘ratio of powers’, a ratio ‘positing itself as self-identical in its otherness’ (Hegel 2010, 278). This idea of a quantum (here a ratio) that relates to another quantum (another ratio) as both itself and its otherness is an apt description of a ratio in harmonic cross-ratio relation to another ratio.

The harmonic cross-ratio posits an equivalence relation holding between two ratios, each being a ratio between two parts of a line segment, AB, divided by a point. One point P located between A and B is said to divide AB internally, but for every such P there will be another point, P′ that divides the distance between A and B externally in the same ratio. For example, in Figure 1 below, the ratio between the distances from A to P′ and from P′ to B (2:6) is the same as that between the distances from A to P and P to B (1:3)—or, at least the same when the absolute value of the distances are considered. When, following an idea introduced by Kant in a paper in his precritical period (Kant 1992) and developed later by Carnot and Grassmann, such line segments are considered in terms of their direction, the ratio AP′: P′B will be thought to be equivalent to −AP: PB because one of the line segments (here AP′) goes in the opposite direction to the three others.¹⁴ In short, the two ratios in the harmonic cross-ratio are equal when considered in terms of their absolute value, but are opposites when the ‘qualitative’ determination of direction is taken into consideration.

Figure 1. Equivalent internal and external divisions of an interval producing the harmonic cross ratio.

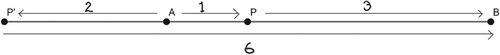
The cross-ratio is called ‘harmonic’ because the double ratio relates back to a sequence of four numbers found in the music theory of Pythagorean mathematicians around the time of Plato, the sequence 6, 8, 9, 12, called the ‘harmonia’ or ‘musical tetraktys’ (Barbera 1984). As can be appreciated from the figure above, the sequence 6, 8, 9, 12 instantiates the pattern represented by the sequence P′, A, P, B, such that the musical tetraktys is an instance of the harmonic cross ratio. The musical tetraktys had represented for the Pythagoreans how a musical octave, represented by the extremes 6 and 12, was divided into consonant intervals. While the octaves are determined by a geometric series (6, 12, 24, 48 …), within the octave the perfect fifth is determined by the arithmetic mean of the extremes (the arithmetic mean of 6 and 12 being 9), and the fourth by harmonic mean (the harmonic mean of 6 and 12 being 8).¹⁵ But a significance well beyond this musical one had been given to this configuration such that, according to the late neo-Pythagoreans, it had been intended by Plato as the ‘beautiful bond’ responsible for the unity of the world described in the Timaeus—a configuration that Hegel treats in his Lectures on the History of Philosophy, as appropriated but distorted by Aristotle in his syllogistic logic (Hegel 2006, 209–210).¹⁶

The cross-ratio posits an equivalence between internal and external ‘divisions’ of an interval in a way that, I suggest, parallels that in which Hegel describes similarly opposed internal and external divisions of the subjects and predicates of judgments, creating dual judgment-forms with opposed ‘inhering’ and ‘subsuming’ predicates, respectively.¹⁷ With his familiarity with Plato’s Pythagorean influences, Hegel would surely have been aware of a fact rediscovered in the 1930s (Einarson 1936), that Aristotle had taken his technical terminology for the syllogism from the music theory of contemporary Pythagorean mathematicians, Aristotle’s logical ‘means’ or ‘middle terms’ coming from the arithmetic and harmonic means dividing the octave.

Hegel’s dual judgment forms cohered with the logic taught at the Tübingen Stift while he was a student there. This was the logic of Gottfried Ploucquet, an important eighteenth-century follower of Leibniz in his attempt to modernise the Aristotelian syllogism by the use of algebra—the approach rediscovered independently in the nineteenth century by Boole. Both Leibniz and Boole had mixed elements from Aristotle’s term logic with a more propositional form of logic of the ancient Stoics, and both struggled to unify these different approaches to the logical form of judgment. Hegel had inherited this dual analysis of judgment structure, with judgments of inherence having a more Aristotelian term-logical form, and judgments of subsumption having a more ‘modern’ propositional content (Hegel 2010, 554−555). He distinguished these two judgment forms by the opposing ways that each handled negation.

Negation in Hegel’s judgments of inherence is ‘internal’ in the sense that it is restricted in scope to the predicate. In negating a judgment of inherence, such as ‘the rose is red’, the scope of negation extends only to the predicate ‘red’ in that while to deny that the rose is red is to imply that it is nevertheless a rose and that has some non-red colour (Hegel 2010, 565). This leaves the negated judgment (‘the rose is non-red’) open to a further, ‘second’ negation which extends to the entire content as with the ‘external negation’ found in the Stoics (Bobzien 2020) as well as modern logics like that of Frege.¹⁸ But in each of Hegel’s judgment-forms, negation simply marks the difference between what is being asserted. In the former judgments, a predicate is asserted of a subject, as when some particular rose is said to be red. In the latter, the assertion applies to the complete sentence: what is asserted is ‘that the rose is red’. That is, like denial (negation), assertion similarly comes in internal and external forms. The fundamental form of negation here is neither of the opposed internal and external forms but the inverse relation that holds between them.

In Hegel’s logic, these internal and external forms of predication would be ultimately shown to be implicit within a more complex form of judgment, the ‘judgment of the concept’,¹⁹ which is itself an implicit ‘syllogism’ made up of two judgments exhibiting each of these opposed logical forms. In this way, Hegel’s syllogism combines opposed internal and externally divided judgment forms echoing the cross-ratio configuration purportedly at the heart of Plato’s syllogism. It is just this aspect that in turn allows Hegel to anticipate and respond to Frege’s criticism of Boole.

George Boole would treat what Hegel had treated as subsumptive judgments as having a modified subject–predicate structure in which the subject term was now the complete primary judgment with the predicate predicating of it that it is true. Thus, he distinguished ‘primary propositions’ as in ‘the sun shines’, which classifies an object (the sun), within a class of objects (things that shine), from secondary propositions, such as ‘it is true that the sun shines’, which classifies the primary proposition, that the sun shines, with the class of true propositions (Boole 1854, 38). But Frege had criticised Boole’s system for the fact that these different logical forms ‘run alongside one another, so that one is like the mirror image of the other, but for that very reason stands in no organic relation to it’ (Frege 1979, 14).

While Boole’s duality of forms approximates Hegel’s, his primary−secondary distinction carries the suggestion that secondary propositions unilaterally presuppose independent primary ones in virtue of being constructed from them. In contrast, Hegel’s forms, characterised as immediate and mediated, are to be related to each other by the fact that each is shown to be an aspect of a further judgment (the judgment of the concept) that is properly a syllogism. In this syllogism, what is presented is some ultimate content that is expressed in partial and inverted ways by each of the component judgments with their opposed logical forms, this ultimate content being, as Michael Wolff points out,²⁰ a type of ‘absolute value’ underlying its opposed logically structured expressions.

It might be expected that the irreducibility of these two logically different judgment forms within the system will play havoc with the traditional laws of logic as usually conceived. Typically, the classical laws of non-contradiction (LNC) and excluded middle (LEM) are expressed in terms of propositional negation: LNC as the law ∼(p and ∼p) and LEM as p or ∼p. But in both Aristotle and Hegel, judgments can, reflecting the role of internal negation in term logics, be opposed to each other in terms of contrariety. For example, the simple judgment of inherence, ‘the rose is red’ will typically be denied by contrary assertions to the fact that the rose is another colour (Hegel 2010, 565). Moreover, one might suspect here that the meaning of ‘this rose is not red’, understood as a negation of the assertion of the predicate of the subject (‘this rose is non-red’), might itself be dependent on some more determinate predication, as in ‘this rose is yellow’ or ‘this rose is pink’ and so on. Here, logical relations cannot be simply abstracted from epistemological issues: it could not be known that a rose was not red without somebody knowing what colour it actually was. This idea has received its most explicit expression in modern intuitionistic logic.

4. Intuitionistic Logic’s Rejection of LEM as a Model for Hegel’s Logic.

Brouwer had conceived his intuitionism as a philosophical perspective on mathematics that was more in accord than its rivals—the logicism of Frege and Russell and the formalism of Hilbert and his school—with the implicit methodology of mathematics and the experience of the mathematician. As the name implies, ‘intuitionism’ had connections to Kant’s earlier philosophy of mathematics in which the truths of mathematics were seen as based upon ‘pure intuition’, with intuition itself characterised as a non-conceptual form of cognition. Brouwer, however, was working downstream of those nineteenth-century developments in geometry, such as projective geometry, that had challenged Kant’s assumptions about the universality of Euclidean geometry and his reaction to this was distinctive.

Rather than abandon the concept–intuition distinction along the lines of a generally conceptual approach as reflected in Frege’s break with Kantianism, Brouwer criticised the exclusivity of the ‘logico-linguistic method, which operated on words by means of logical rules, sometimes without any guidance from experience and sometimes even starting from axioms framed independently of experience’.²¹ Indeed, Whitehead and Russell had exemplified the ‘logico-linguistic’ approach to mathematics to which Brouwer was opposed.²² As Michael Detlefsen has summarised, Brouwer had stressed the need to account for ‘the seeming difference in the epistemic conditions of provers whose reasoning is based on genuine insight into the subject-matter being investigated, and would-be provers whose reasoning is not based on such insight’. The new epistemology would require a new conception of inference, ‘for in order for a truth to be proven it requires that it be “experienced” in a certain way’ (Detlefsen 1990, 501). ‘Mathematical knowledge becomes more than simply knowledge of a mathematical proposition, and is distinguished by a certain mode or kind of cognitive state as well’ (505–506). Hegel’s judgments of inherence would similarly require that the judgment’s content be ‘“experienced” in a certain way’.

Within intuitionism, one particular expression of this attitude would be the rejection of indirect proofs in mathematical reasoning, such as found in traditional ‘reductio ad absurdem’ arguments. Some geometric truth, for example, could not be simply established by showing that its negation was self-contradictory, because such a form of proof was not accompanied by any appropriate corresponding mathematical experience. This undermined the axiomatic status given to the Law of Excluded Middle which, stated in modern form, asserts that if a proposition p is false then its contradictory ∼p must be true.²³ This in turn is associated with two important features of Brouwer’s intuitionism. First, it allows a role for undecidable propositions—ones for which there exist neither proofs nor refutations and so cannot be considered either true or false. Next, it is linked to the role of the experience of some concrete ‘witness’ or ‘proof object’ required for the truth of any abstractly propositional claim (Bridges and Palmgren 2018, section III).

Extended to empirical knowledge, such intuitionistic claims clearly bear on modal issues. We might think that in relation to the determinateness of our claims about the actual world rather than about merely possible alternatives to it, the semantics of our sentences must go beyond a mere consideration of truth-values of abstract propositions. Within the Frege–Russell logical system, an existential statement about a red rose can be about some indefinite x existing in some or other indefinite time and place, declaring that x to be both a rose and red. On the model of intuitionism, however, to be meaningful this abstract existential statement would need to be accompanied by a singular ‘witness statement’ about some specific identifiable rose—‘this rose’—that, on the basis of experience of it, is known to be red.²⁴ In a similar way, Hegel’s abstract judgments of subsumption, in order to have determinate content, must in some way stand in relation to an equivalent judgment of inherence.

Hegel’s predicative duality clearly situates his logic more within the Boolean ‘algebra of logic’ tradition than within the classical logic of Frege and Russell, but within such approaches I have suggested that his position on the classical logical laws puts him closer to intuitionistic variants of that logic. Thus, rather than Boolean logic, which conforms to the laws of non-contradiction and excluded middle, Hegel’s might be better compared to the type of mathematical logic proposed in the 1920s by Arend Heyting, the student of Brouwer who developed an algebraic form of logic which, while similar to the approach of Boole, departed from it in important ways.

Crucially, negation does not function within the Heyting algebra underlying his intuitionistic logic as it does in Boolean logic. For Heyting, ‘true’ will effectively have to be understood as indicating proven by the experience of a witness and ‘false’ as similarly indicating refuted by some experience of a contrary witness. In Hegel, this will be reflected by the idea that a negative judgment, such as the ‘rose is not red’, presupposes an experience of the rose as having some other colour. For intuitionistic logic, this means that ‘true’ and ‘false’ are no longer complementary notions: ‘not p’ is not defined as the contradictory of ‘p’, such that ‘not p’ is false when ‘p’ is true and true when ‘p’ is false.²⁵ Rather, Heyting’s alternative was to define negation by utilising a consequence that had followed from Russell’s original definition of material implication—the fact that a false proposition implies any other proposition.²⁶ But if implication is to be used in the definition of negation, clearly the relation of implication cannot itself be defined in ways that employ the negation operator, as is done in classical logic. The relation of implication must be defined in a different way and the implication that results in a Heyting algebra is weaker than that found in classical logic.²⁷

This does not mean that intuitionistic logic is simply different to or incommensurable with classical logic, however. Rather, it means that the Heyting algebra underlying inituitionistic logic is able to be strengthened by the addition of certain other axioms that, in specific contexts, convert it to a Boolean one. This allows the judgments to feed into different aspects of inquiry resulting in an overall dynamic system, but this type of dynamism might also, I suggest, be seen as implicit within Hegel’s logic as well.

Consider, for example, Hegel’s singular judgments of inherence, considered as functioning as ‘witness statements’, and so as providing intuitionist-type proofs for corresponding general claims.²⁸ In verifying a theory, one will want to call upon specific witness statements rather than their abstract equivalents. But in other contexts, one will rely on the equivalent abstractly propositional claims to understand how such claims relate to other claims via the formal implication relations among their contents. Only abstract judgments will be needed, for example, in considering logical relations holding within some hypothetical model of reality that is yet to be subject to empirical verification. They will be sufficient for models of the way the world might be, but not for the way that it is known to be.

Such an idea of logic cannot conform to the requirement of constructing some ultimate logical language—a Leibnizian ‘characteristica universalis’ or Fregean ‘Begriffsschrift’—but Hegel was critical of the conception of any such ‘standard language in which each concept is presented as a connection of other concepts’ in such a way that ‘a content would still retain the same determinations that it has when fixed in isolation’ (Hegel 2010, 608). A similar rejection of any idea of a universal language would later be expressed by the Cambridge logician W. E. Johnson, who continued to hold to the algebraic approach of the Booleans after the appearance of Principia Mathematica (Johnson 1921–1924). Johnson treated logic as a mathematical device useful in specific contexts to aid human reasoning, but which itself depended upon human reasoning and human language for its interpretation. As he had put it in the early 1890s, a logical calculus ‘aims at exhibiting, in a non-intelligent form, those same intelligent principles that are actually required for working it’ (Johnson 1892, 3). That is, in order for these ‘disinterpreted’ strings of symbols to be grasped as logical, they must once more be reinterpreted which suggests two things: they must rely upon the resources of everyday language and thought for their reinterpretation, and that reinterpretation could in turn result in new meanings being given to those elements.²⁹ Hegel had suggested a picture of thought as similarly dependent upon, but not simply determined by, the historically changing resources of human language (Hegel 2010, 12−14).

5. Conclusion

Frege’s project of a ‘concept script’ [Begriffsschrift] might be seen as historically continuous with a conception of logic going back to William of Ockham, for whom logic was essentially an attempt to find the language implicit in the thoughts of the type of omniscient god found in the Old Testament. While the discipline of logic may have long abandoned such explicit theological assumptions, it can be argued that the presupposed idea of divine omniscience has continued into its modern classical form. In this way, logic is typically conceived as a logic fit for reasoning in a domain containing no undecidable propositions—that is, reasoning about a world within which there is nothing that would be unknowable to an omniscient god, were there to be one. Such a conception of divine omniscience was not, I have argued elsewhere, part of Hegel’s theology (Redding 2012) and neither should it be found as an implicit idea within his logic.

While Ockham’s god had conformed to Old Testament theology, Hegel’s was more influenced by the theos of Plato and Aristotle, as well as the Neoplatonic doctrines that later would feed into Christianity with its trinitarian conception of God becoming man—the same sources that had influenced his approach to mathematics and logic. It should not be surprising, then, that his logic would reflect features different from those of classical logic and that he would reject the axiomatic status of classical logical laws. I have argued that, in particular, his logic can be understood as sharing features similar to those found in the intuitionistic modification of Boole.

Notes

1 The idea of the ‘distinterpretation’ of algebra to a purely syntactic calculus had been proposed by the Cambridge mathematician Duncan Gregory in 1840 (Ewald 1996, 443).

2 See Putnam 1990. There are now numerous challenges to the conventional story of the definitive triumph of the modern classical logic initiated by Frege and Russell. The familiar ‘classical’ and ‘nonclassical’ distinction tends to fracture along many different fault lines, but two that are particularly relevant for Hegel’s logic concern, on the one hand, the different roles given to mathematics between the rival algebra of logic and logicist traditions of the late nineteenth century, and, on the other, the differences between logics focusing on actions (judgments and inferences) and intentional objects (propositions and relations of logical consequence). In relation to the former, see for example Peckhaus 2009 and Grattan Guinness 2004, and in relation to the latter, Prior 1949 and Sundholm 2009. For a wider coverage of the sorts of distinctions found among modern logics see the contributions to Stelzner and Stöckler 2001.

3 Intuitionistic logic would be first developed by Brouwer’s student Arend Heyting in the 1920s.

4 The idea of a hybrid logic of this sort was suggested by Arthur Prior (Blackburn 2006).

5 For example, plotted against the x and y coordinates, a circle of radius 1 unit with its centre at the origin of the co-ordinates could be represented by the equation x² + y² = 1.

6 See, for example, Struik 2011, chs. 2–7. This is because the same cross-ratio relation holds among the angles formed by the lines intersecting at point O. This equivalence of the cross-ratio holding among four collinear points on the one hand and four concurrent lines on the other is an example of the duality between point and lines in projective geometry.

7 See, for example, Gowers 2008. Thus, for any theorem in projective geometry that concerns some complex relation (or relation of relations) among some structured array of points, an equivalent theorem could be found that applied in the same way to an analogously structured array of lines.

8 Russell 1897, v. Russell also acknowledges Whitehead for his own becoming aware of the ‘philosophical importance of projective Geometry’ (Russell 1897, v).

9 See, for example, Wolff 1999 and Lawvere 1991. Grassmann seems to have been much more directly influenced by the idealist philosopher and hermeneutic theorist Friedrich Schleiermacher, and through him, by Friedrich Schlegel (Lewis 1977). Nevertheless, there clearly had been certain parallels in the approaches to logic common to Schleiermacher and Hegel, despite their differences.

10 Perception ‘presents us with various things, with discriminated and differentiate contents’ but ‘what we wish to study here […] is the bare possibility of such diversity’ which can be considered the ‘form of externality’. Russell then asks after the properties that ‘such a form, when studied in abstraction, [must] necessarily possess’ (Russell 1897, 136). He answers that such a form must be essentially relational: ‘In the first place, externality is an essentially relative conception—nothing can be external to itself. […] Hence, when we abstract a form of externality from all material content, and study it in isolation, position will appear, of necessity, as purely relative—a position can have no intrinsic quality’ (136–137).

11 Alan Paterson stresses the influence of Proclus (Paterson 2004/2005, 63), but besides Proclus’ Commentary on Euclid’s Elements, Hegel also possessed significant works by Iamblichus and Nicomachus of Gerasa (Mense 1993, 672).

12 See, in particular, Nicomachus of Gerasa 1926, 284–285 and Proclus 2009, 171, 10–15. The history of projective geometry and the cross-ratio would be reconstructed by the French geometer, Michel Chasles (Chasles 1837).

13 These three means were the geometric, the arithmetic, and the harmonic. The history of this phase of Greek mathematics had been preserved by Pappus of Alexandria, whose writings had been influential in the reintroduction of projective geometry in the seventeenth century.

14 Thus, the harmonic cross-ratio between two pairs of colinear points (A and B) and (P' and P) can be expressed by fractions and said to hold when the double-ratio $\frac{\frac{AP}{PB}}{\frac{A{P}^{\mathrm{\prime }}}{{\mathrm{P}}^{\mathrm{\prime }}\mathrm{B}}}=-1$.

15 Of two terms, a and b, the geometric mean = √ab, the arithmetic mean = $\frac{a+b}{2}$ and the harmonic mean $=\frac{2ab}{a+b}$. The three means are interrelated in complex ways.

16 I have presented the details of these links in Redding 2023.

17 Indeed, the German term for judgment, ‘Urteil’, already suggests such a division, ‘Teilung’, into parts, Teilen.

18 Following Frege, Russell had denied that everyday judgments like Hegel’s ‘the rose is red’ were in fact proper judgments. Without complete propositional content, Russell came to treat such judgments as incapable of truth or falsity. Thus, he treated in this way an example used by the early modal logician, Hugh MacColl, ‘Mrs Brown is not at home’ (Russell 1906). Such an expression was really a type of predicate that would only be capable of truth or falsity if said of a particular point in time, as in ‘Mrs Brown is not at home at time t₁’.

19 See Hegel 2010, 581−587. Such a judgment in Hegel’s account is an explicitly evaluative judgment about a human act or artifact. His examples are ‘this act is good’ and ‘this house is bad’ (Hegel 2010, 583).

20 ‘Hegel actually anticipates an important idea in the newer algebra and vector algebra [of Hermann Grassmann]. […] Hegel […] introduces the concept of absolute value as the concept of a substrate of logical reflection’ (Wolff 1999, 15).

21 Brouwer 1981, 2. At his most extreme, Brouwer seemed to suggest that concepts were relevant only to the linguistic expression of mathematical truths and not the truths themselves.

22 Thus, Whitehead had stated in his Treatise on Universal Algebra that ‘Mathematics in its widest signification is the development of all types of formal, necessary, deductive reasoning. […] The sole concern of mathematics is the inference of proposition from proposition’ (Whitehead 1898, vi), while Russell, in The Principles of Mathematics, would proclaim pure mathematics to be ‘the class of all propositions of the form ‘p implies q’, where p and q are propositions containing one or more variables, the same in the two propositions, and neither p nor q contains any constants except logical constants’ (Russell 1903, § 1).

23 C.f. ‘The belief in the universal validity of the principle of the excluded third in mathematics [i.e., LEM] is considered by the intuitionists as a phenomenon of the history of civilization of the same kind as the former belief in the rationality of π, or in the rotation of the firmament about the earth’ (Brouwer 1981, 7).

24 Without invoking intuitionism, Robert Stalnaker uses the idea of singular propositions playing to role of witnesses to existential propositions in this way in Stalnaker 2019, ch. 2.

25 Technically, Heyting algebra will have the structure of a ‘semi-lattice’ in contrast to the ‘lattice’ structure of Boolean algebra, this difference being reflected in their different conceptions of negation.

26 In Brouwer’s example, ∼p ⊃ (p ⊃ 1 = 2).

27 In the 1930s Gerhard Gentzen would come to provide a form of logical semantics appropriate to intuitionism. See, for example, Sundholm 2009, 292−8.

28 Such proofs must be of course fallible and be able to be revised under further discoveries about the conditions under which one experiences. Propositions that had previously been assumed and thus without proof or refutation, for example, may come to be shown to be false.

29 On the resistance of the logic of Frege and Russell to reinterpretation, see Goldfarb 1982, 694.