On the edge irregular reflexive labeling of corona product of graphs with path

Abstract

We define a total k-labeling $\phi$ of a graph G as a combination of an edge labeling ${\phi }_e:E(G)\to \{1,2,\dots ,k_e\}$ and a vertex labeling ${\phi }_v:V(G)\to \{0,2,\dots ,2k_v\},$ such that $\phi (x)={\phi }_v(x)$ if $x\in V(G)$ and $\phi (x)={\phi }_e(x)$ if $x\in E(G),$ where $k= \text{max} \{k_e,2k_v\}.$ The total k-labeling $\phi$ is called an edge irregular reflexive k-labeling of G if every two different edges has distinct edge weights, where the edge weight is defined as the summation of the edge label itself and its two vertex labels. Thus, the smallest value of k for which the graph G has the edge irregular reflexive k-labeling is called the reflexive edge strength of G. In this paper, we study the edge irregular reflexive labeling of corona product of two paths and corona product of a path with isolated vertices. We determine the reflexive edge strength for these graphs.

Keywords:

• Edge irregular reflexive labeling
• reflexive edge strength
• corona product
• path
• complete graph

2010 Mathematical Subject Classification:

• 05C78
• 05C38

1. Introduction

An edge irregular reflexive labeling is introduced by Ryan et al. [25] and is inspired by the problems of an irregular assignment and an edge irregular total labeling. Let us start with a brief review of the origins and some background information of these labelings.

Chartrand et al. [13] proposed a labeling problem in 1988, that is, determine the minimum value of parallel edges between every two vertices to ensure that a loopless multigraph has vertex irregularity. This problem is created as a consequence of Handshaking Lemma, i.e., no simple graph can have each distinct vertex degree, however, it is possible in multigraphs.

They defined this labeling problem as an edge k-labeling $\delta :E(G)\to \{1,2,\dots ,k\}$ of a graph G such that the vertex weight is $w_{\delta }(x)\ne w_{\delta }(y)$ for all vertices x, $y\in V(G)$ with $x\ne y,$ where $w_{\delta }(x)=\sum \delta (xy)$ the summation is over all vertices y adjacent to x. Such labeling is called irregular assignment and the irregularity strength of G, s(G) is known as the minimum k for which G has an irregular assignment using labels not greater than k. In other words, irregularity strength is interpreted as the minimum number of parallel edges, such that every vertex has a distinct degree in multigraph. For further results, see papers [6, 14, 17, 23, 24]. For comprehensive survey of graph labelings, please refer [15].

Bača et al. [10] introduced a total k-labeling $\rho :V(G)\cup E(G)\to \{1,2,\dots ,k\}$ to be an edge irregular total k-labeling of a graph G if for every two different edges xy and $x\prime y\prime$ of G one has $wt(xy)=\rho (x)+\rho (xy)+\rho (y)\ne wt(x\prime y\prime )=\rho (x\prime )+\rho (x\prime y\prime )+\rho (y\prime ).$ The total edge irregularity strength, denoted by tes(G) is defined as the minimum k for which G has an edge irregular total k-labeling. Some other results on the total edge irregularity strength can be referred to [2–5, 7, 11, 12, 21, 22, 26].

Therefore, Ryan et al. [25] were subsequently combined these two labeling problems by allowing for the vertex labels representing as loops. They noticed that: (a) the vertex labels are even non-negative integers, which also representing the fact that each loop added 2 to the vertex degree; and (b) vertex label 0 is permissible as representing a loopless vertex.

Thus, they defined the edge irregular reflexive k-labeling as a combination of an edge labeling ${\phi }_e:E(G)\to \{1,2,\dots ,k_e\}$ and a vertex labeling ${\phi }_v:V(G)\to \{0,2,\dots ,2k_v\},$ in which labeling $\phi$ is a total k-labeling of the graph G such that $\phi (x)={\phi }_v(x)$ if $x\in V(G)$ and $\phi (x)={\phi }_e(x)$ if $x\in E(G),$ where $k= \text{max} \{k_e,2k_v\}.$ The total k-labeling $\phi$ is called an edge irregular reflexive k-labeling of G if for every two different edges xy, $x\prime y\prime$ of G one has $wt(xy)={\phi }_v(x)+{\phi }_e(xy)+{\phi }_v(y)\ne wt(x\prime y\prime )={\phi }_v(x\prime )+{\phi }_e(x\prime y\prime )+{\phi }_v(y\prime ).$ The smallest value of k for which such labeling exists is called the reflexive edge strength of G and is denoted by res(G). For more results of reflexive edge strength of graphs, see [1, 8, 9, 16, 18–20, 27, 28].

This paper focuses on the edge irregular reflexive labeling of two classes of corona product of graphs, that is, corona product of two paths and corona product of a path with isolated vertices. All graphs considered here are simple, finite and undirected. At the end of this paper, we are able to determine the reflexive edge strength of these graphs with condition that they admit such labeling.

2. Significant lemma and conjecture

It is known that Lemma 1 is proved in [25].

Lemma 1.

For every graph G, $$\text{res}(G)\ge \mathrm{ }\{\begin{array}{ll}\lceil \frac{|E(G)|}{3}\rceil & \mathit{\text{if}} |E(G)|\cancel{\equiv }2,3 (\text{mod} 6),\\ \lceil \frac{|E(G)|}{3}\rceil +1& \mathit{\text{if}} |E(G)|\equiv 2,3 (\text{mod} 6).\end{array}\begin{array}{c}\\ \end{array}$$

The following conjecture is proved by Bača et al. [9].

Conjecture 1.

Any graph G with maximum degree $\Delta (G)$ satisfies: $$\mathit{res}(G)=\text{max} \{\lfloor \frac{\Delta +2}{2}\rfloor ,\lfloor \frac{|E(G)|}{3}\rfloor +r\}$$ where r = 1 for $|E(G)|\equiv 2,3 (\text{mod} 6),$ and zero otherwise.

3. Corona product of two paths

Suppose P_n is a path of order n and P_m is another path of order m. The corona product of two paths, denoted by $P_n\odot P_m$ is defined as a graph obtained by taking one copy of P_n (with n vertices) and n copies of P_m, and then joining the i-th vertex of P_n to every vertex of the i-th copy of P_m.

Therefore, the vertex set and edge set of $P_n\odot P_m$ are defined as $V(P_n\odot P_m)=\{x_i,y_i^j:1\le i\le n,1\le j\le m\}$ and $E(P_n\odot P_m)=\{x_i{y}_i^j:1\le i\le n,1\le j\le m\}\cup \{y_i^j{y}_i^{j+1}:1\le i\le n,1\le j\le m-1\}\cup \{x_i{x}_{i+1}:1\le i\le n-1\},$ respectively.

The following theorem shows the edge irregular reflexive labeling on $P_n\odot P_m$ and its reflexive edge strength, $\mathit{res}(P_n\odot P_m).$

Theorem 1.

For $n\ge 2$ and $m\ge 2,$ $$\mathit{res}(P_n\odot P_m)=\{\begin{array}{l}\lceil \frac{2nm-1}{3}\rceil ,\mathrm{ }\\ \lceil \frac{2nm-1}{3}\rceil +1,\end{array}\begin{array}{l}\mathit{\text{if}}\mathrm{ }nm\cancel{\equiv }2 (\mathrm{mod}\mathrm{ }3),\\ \mathit{\text{if}}\mathrm{ }nm\equiv 2 (\mathrm{mod}\mathrm{ }3).\end{array}\mathrm{ }\mathrm{ }$$

Proof.

Note that the graph $P_n\odot P_m$ has $2nm-1$ edges. By using Lemma 1, we obtain the following lower bound: $$\mathit{res}(P_n\odot P_m)\ge k=\{\begin{array}{ll}\lceil \frac{2nm-1}{3}\rceil ,& \text{if}\mathrm{ }nm\cancel{\equiv }2 (\mathrm{mod}\mathrm{ }3),\\ \lceil \frac{2nm-1}{3}\rceil +1,\mathrm{ }& \text{if}\mathrm{ }nm\equiv 2 (\mathrm{mod}\mathrm{ }3).\mathrm{ }\end{array}\mathrm{ }$$

It clearly shows that k is odd only when n, $m\equiv 1 (\text{mod} 3)$ or n, $m\equiv 2 (\text{mod} 3),$ otherwise, k is even.

Now, we prove that k is the upper bound for $\mathit{res}(P_n\odot P_m$), where n, $m\ge 2.$ First, we define a total k-labeling $\phi$ of $P_n\odot P_m$ by labeling the vertex set and edge set.

1. All vertices x_i and $y_i^j$ are labeled with the even integers in the following ways.

   1. $\phi (x_1)=0,\phi (x_2)=2\lceil \frac{m-1}{3}\rceil ,$ otherwise, $\phi (x_i)=2\lceil \frac{im-1}{3}\rceil$ if $i\ge 3.$

   2. For $1\le j\le m,\phi (y_1^j)=2\lceil \frac{j-2}{2}\rceil ,$ otherwise, $\phi (y_i^j)=2\lceil \frac{im-1}{3}\rceil$ if $i\ge 2.$

2. The edges $x_i{y}_i^j,y_i^j{y}_i^{j+1}$ and $x_i{x}_{i+1}$ are labeled as follows.

   1. $\phi (x_1{y}_1^j)=1$ if j is odd, whereas $\phi (x_1{y}_1^j)=2$ if j is even. For $1\le j\le m,\phi (x_2{y}_2^j)=j,$ otherwise, $\phi (x_i{y}_i^j)=2m(i-1)-4\lceil \frac{im-1}{3}\rceil +j$ if $i\ge 3.$

   2. For $1\le j\le m-1,\phi (y_1^j{y}_1^{j+1})=m+2-j,$ otherwise, $\phi (y_i^j{y}_i^{j+1})=m(2i-1)-4\lceil \frac{im-1}{3}\rceil +j$ if $i\ge 2.$

   3. The edges $x_i{x}_{i+1}$ are labeled as follows: $$\phi (x_i{x}_{i+1})=\{\begin{array}{ll}2m-2\lceil \frac{m-1}{3}\rceil ,\mathrm{ }& \text{if}\mathrm{ }i=1,2,\\ 2im-2\lceil \frac{im-1}{3}\rceil -2\lceil \frac{(i+1)m-1}{3}\rceil ,& \text{if}\mathrm{ }i\ge 3.\mathrm{ }\end{array}\mathrm{ }$$

Evidently, all vertex labels and edge labels are at most k under the labeling $\phi ,$ thus, labeling $\phi$ is a total k-labeling of $P_n\odot P_m.$ Next, we show the edge weights of all edges in $P_n\odot P_m$ are distinct under the total k-labeling $\phi .$

1. $w{t}_{\phi }(x_i{y}_i^j)=\phi (x_i)+\phi (x_i{y}_i^j)+\phi (y_i^j).$

   1. For j odd, $w{t}_{\phi }(x_1{y}_1^j)=0+1+2\lceil \frac{j-2}{2}\rceil =1+j-1=j,$ whereas for j even, $w{t}_{\phi }(x_1{y}_1^j)=0+2+2\lceil \frac{j-2}{2}\rceil =2+j-2=j.$

   2. For $1\le j\le m,w{t}_{\phi }(x_2{y}_2^j)=2\lceil \frac{m-1}{3}\rceil +j+2\lceil \frac{im-1}{3}\rceil =2(\lceil \frac{m-1}{3}\rceil +\lceil \frac{2m-1}{3}\rceil )+j=2m+j.$

   3. For $i\ge 3$ and $1\le j\le m,w{t}_{\phi }(x_i{y}_i^j)=2\lceil \frac{im-1}{3}\rceil +2m(i-1)-4\lceil \frac{im-1}{3}\rceil +j+2\lceil \frac{im-1}{3}\rceil =2m(i-1)+j.$

2. $w{t}_{\phi }(y_i^j{y}_i^{j+1})=\phi (y_i^j)+\phi (y_i^j{y}_i^{j+1})+\phi (y_i^{j+1}).$

   1. For $1\le j\le m-1,w{t}_{\phi }(y_1^j{y}_1^{j+1})=2\lceil \frac{j-2}{2}\rceil +m+2-j+2\lceil \frac{(j+1)-2}{2}\rceil =2(j-1)+m+2-j=m+j.$

   2. For $i\ge 2$ and $1\le j\le m-1,w{t}_{\phi }(y_i^j{y}_i^{j+1})=2\lceil \frac{im-1}{3}\rceil +m(2i-1)-4\lceil \frac{im-1}{3}\rceil +j+2\lceil \frac{im-1}{3}\rceil =m(2i-1)+j.$

3. $w{t}_{\phi }(x_i{x}_{i+1})=\phi (x_i)+\phi (x_i{x}_{i+1})+\phi (x_{i+1}).$

   1. $w{t}_{\phi }(x_1{x}_2)=0+2m-2\lceil \frac{m-1}{3}\rceil +2\lceil \frac{m-1}{3}\rceil =2m.$

   2. $w{t}_{\phi }(x_2{x}_3)=2\lceil \frac{m-1}{3}\rceil +2m-2\lceil \frac{m-1}{3}\rceil +2\lceil \frac{(i+1)m-1}{3}\rceil =2m+2\lceil \frac{3m-1}{3}\rceil =2m+2m=4m.$

   3. For $i\ge 3,w{t}_{\phi }(x_i{x}_{i+1})=2\lceil \frac{im-1}{3}\rceil +2im-2\lceil \frac{im-1}{3}\rceil -2\lceil \frac{(i+1)m-1}{3}\rceil +2\lceil \frac{(i+1)m-1}{3}\rceil =2im.$

We can see that the edge weights of all edges in $P_n\odot P_m$ are distinct integers from the set $\{1,2,\dots ,2nm-1\},$ in other words, every edge has a distinct weight. Thus, the total k-labeling $\phi$ is an edge irregular reflexive k-labeling of $P_n\odot P_m$ and k is the reflexive edge strength of $P_n\odot P_m.$ This completes the proof. □

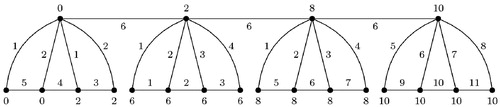
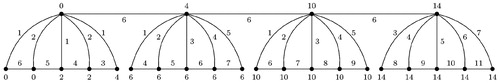
Figures 1 and 2 show the corresponding edge irregular reflexive k-labelings of $P_4\odot P_4$ and $P_4\odot P_5.$

Figure 1. The edge irregular reflexive 11-labeling of $P_4\odot P_4.$

Figure 2. The edge irregular reflexive 14-labeling of $P_4\odot P_5.$

4. Corona product of a path with isolated vertices

Assume P_n is a path of order n and mK₁ is a disjoint union of m copies of isolated vertex. The corona product of a path with m copies of isolated vertex, denoted by $P_n\odot m{K}_1$ is defined as a graph obtained by taking one copy of P_n (with n vertices) and n copies of mK₁ by joining the i-th vertex of P_n to every vertex of the i-th copy of mK₁. Note that $P_n\odot m{K}_1$ is also known as a subclass of caterpillars.

Therefore, the vertex set and edge set of $P_n\odot m{K}_1$ are $V(P_n\odot m{K}_1)=\{x_i,y_i^j:1\le i\le n,1\le j\le m\}$ and $E(P_n\odot m{K}_1)=\{x_i{x}_{i+1}:1\le i\le n-1\}\cup \{x_i{y}_i^j:1\le i\le n,1\le j\le m\},$ respectively. The number of edges of $P_n\odot m{K}_1,$ denoted by $|E(P_n\odot m{K}_1)|$ is $n(m+1)-1.$ Thus, according to Lemma 1, (1) $$\mathit{res}(P_n\odot m{K}_1)\ge k=\{\begin{array}{ll}\lceil \frac{n(m+1)-1}{3}\rceil ,& \text{if}\mathrm{ }n(m+1)-1\cancel{\equiv }2,3 (\mathrm{mod} 6),\\ \lceil \frac{n(m+1)-1}{3}\rceil +1,& \text{if}\mathrm{ }n(m+1)-1\equiv 2,3 (\text{mod}\mathrm{ }6).\mathrm{ }\end{array}\mathrm{ }$$(1)

We notice that k is odd when $n\equiv 1 (\text{mod} 3),m\equiv 1 (\text{mod} 6)$ or $n\equiv 2 (\text{mod} 3),m\equiv 3 (\text{mod} 6)$ or $n\equiv 2 (\text{mod} 6),m\equiv 0 (\text{mod} 6)$ or n, $m\equiv 4 (\text{mod} 6).$ Otherwise, k is even.

The following lemmas show the reflexive edge strength of $P_n\odot m{K}_1$ by distinguishing m into odd and even cases. First, we deal with $P_n\odot m{K}_1$ when m is odd.

Lemma 2.

For $n\ge 2$ and m odd, $$\mathit{res}(P_n\odot m{K}_1)=\{\begin{array}{ll}\lceil \frac{n(m+1)-1}{3}\rceil ,& \mathit{\text{if}}\mathrm{ }n(m+1)-1\cancel{\equiv }2,3 (\mathrm{mod} 6),\\ \lceil \frac{n(m+1)-1}{3}\rceil +1,& \mathit{\text{if}}\mathrm{ }n(m+1)-1\equiv 2,3 (\mathrm{mod} 6).\mathrm{ }\end{array}\mathrm{ }$$

Proof.

As a fact that $P_n\odot m{K}_1$ has $n(m+1)-1$ edges. According to Lemma 1, the lower bound for $\mathit{res}(P_n\odot m{K}_1)$ is shown as (1). Now, we prove that k is the upper bound for $\mathit{res}(P_n\odot m{K}_1)$ when m is odd. We first define a total k-labeling $\phi$ of $P_n\odot m{K}_1.$

1. All vertices x_i and $y_i^j$ are labeled with the even integers in the following ways.

   1. $\phi (x_1)=0.$ For $m\equiv 1 (\text{mod} 6),i\equiv 2 (\text{mod} 3)$ or $m\equiv 3 (\text{mod} 6),i\equiv 4 (\text{mod} 3),\phi (x_i)=\lceil \frac{i(m+1)+2}{3}\rceil .$ Otherwise, $\phi (x_i)=\lceil \frac{i(m+1)-2}{3}\rceil .$

   2. $$\phi (y_i^j)=\{\begin{array}{ll}0,& \text{if} i=1,m\equiv 1 (\mathrm{mod} 6),1\le j\le \lceil \frac{2(m+2)}{3}\rceil , \\ & \text{or} m\equiv 3,5 (\mathrm{mod} 6),1\le j\le \lceil \frac{2m}{3}\rceil ,\\ & \text{if} i=2,m\equiv 1 (\text{mod} 6),1\le j\le \lceil \frac{m+3}{3}\rceil , \\ & \text{or} m\equiv 3,5 (\mathrm{mod} 6),1\le j\le \lceil \frac{m}{3}\rceil ,\\ \lceil \frac{2(m+2)}{3}\rceil ,& \text{if} i=1,m\equiv 1 (\mathrm{mod} 6),\lceil \frac{2(m+2)}{3}\rceil +1\le j\le m, \\ & \text{or} i=2,m\equiv 1 (\text{mod} 6),\lceil \frac{m+3}{3}\rceil +1\le j\le m,\\ \lceil \frac{2m}{3}\rceil ,& \text{if} i=1,m\equiv 3,5 (\mathrm{mod}6),\lceil \frac{2m}{3}\rceil +1\le j\le m,\\ & \text{or} i=2,m\equiv 3,5 (\mathrm{mod} 6),\lceil \frac{m}{3}\rceil +1\le j\le m,\\ \phi (x_i),& i\ge 3,1\le j\le m.\end{array}$$

2. The edges $x_i{y}_i^j$ and $x_i{x}_{i+1}$ are labeled as follows.

   1. $\phi (x_1{y}_1^j)=j$ if $m\equiv 1 (\text{mod} 6),1\le j\le \mathrm{ }\lceil \frac{2(m+2)}{3}\rceil$ or $m\equiv 3,5 (\text{mod} 6),1\le j\le \mathrm{ }\lceil \frac{2m}{3}\rceil ,$ otherwise, $\phi (x_1{y}_1^j)=j-\phi (x_2).$ Next, $\phi (x_2{y}_2^j)=m+1-\phi (x_2)+j$ if $m\equiv 1 (\text{mod} 6),1\le j\le \mathrm{ }\lceil \frac{m+3}{3}\rceil$ or $m\equiv 3,5 (\text{mod} 6),1\le j\le \lceil \frac{m}{3}\rceil ,$ otherwise, $\phi (x_2{y}_2^j)=m+1-2\phi (x_2)+j.$ For $i\ge 3$ and $1\le j\le m,\phi (x_i{y}_i^j)=(i-1)(m+1)-2\phi (x_i)+j.$

   2. $\phi (x_1{x}_2)=\lceil \frac{m-1}{3}\rceil$ if $m\equiv 1 (\text{mod} 6),$ whereas $\phi (x_1{x}_2)=\lceil \frac{m+1}{3}\rceil$ if $m\equiv 3,5 (\text{mod} 6).$ Next, for $m\equiv 1 (\text{mod} 6),i\equiv 2,3 (\text{mod} 3)$ or $m\equiv 3 (\text{mod} 6),i\equiv 4 (\text{mod} 3),\phi (x_i{x}_{i+1})=i(m+1)-2\phi (x_i)-\lceil \frac{m-3}{3}\rceil .$ For $m\equiv 5 (\text{mod} 6),i\ge 2,\phi (x_i{x}_{i+1})=i(m+1)-2\phi (x_i)-\lceil \frac{m+1}{3}\rceil .$ Otherwise, $\phi (x_i{x}_{i+1})=i(m+1)-2\phi (x_i)-\lceil \frac{m+3}{3}\rceil .$

Evidently, all vertex labels and edge labels are at most k under the labeling $\phi ,$ thus, labeling $\phi$ is a total k-labeling of $P_n\odot m{K}_1.$ Next, we show the edge weights of all edges in $P_n\odot m{K}_1$ are distinct under the total k-labeling $\phi .$

1. $w{t}_{\phi }(x_i{y}_i^j)=\phi (x_i)+\phi (x_i{y}_i^j)+\phi (y_i^j).$

   1. For i = 1,

      1. $w{t}_{\phi }(x_1{y}_1^j)=0+j+0=j$ if $m\equiv 1 (\text{mod}6),1\le j\le \mathrm{ }\lceil \frac{2(m+2)}{3}\rceil$ or $m\equiv 3,5 (\text{mod}6)$ $1\le j\le \lceil \frac{2m}{3}\rceil .$

      2. $w{t}_{\phi }(x_1{y}_1^j)=0+j-\phi (x_2)+\lceil \frac{2(m+2)}{3}\rceil =j-\lceil \frac{2(m+2)}{3}\rceil +\lceil \frac{2(m+2)}{3}\rceil =j$ if $m\equiv 1 (\text{mod}6),\lceil \frac{2(m+2)}{3}\rceil +1\le j\le m.$

      3. $w{t}_{\phi }(x_1{y}_1^j)=0+j-\phi (x_2)+\mathrm{ }\lceil \frac{2m}{3}\rceil =j-\lceil \frac{2m}{3}\rceil +\lceil \frac{2m}{3}\rceil =j$ if $m\equiv 3,5 (\text{mod} 6),\lceil \frac{2m}{3}\rceil +1\le j\le m.$

   2. For i = 2,

      1. when $m\equiv 1 (\text{mod} 6),w{t}_{\phi }(x_2{y}_2^j)=\lceil \frac{i(m+1)+2}{3}\rceil +m+1-\phi (x_2)+j+0=\lceil \frac{i(m+1)+2}{3}\rceil +m+1-\lceil \frac{i(m+1)+2}{3}\rceil +j=m+1+j$ if $1\le j\le \mathrm{ }\lceil \frac{m+3}{3}\rceil ,$ otherwise, $w{t}_{\phi }(x_2{y}_2^j)=\lceil \frac{i(m+1)+2}{3}\rceil +m+1-2\phi (x_2)+j+\lceil \frac{2(m+2)}{3}\rceil =\lceil \frac{i(m+1)+2}{3}\rceil +m+1-2\lceil \frac{i(m+1)+2}{3}\rceil +j+\lceil \frac{i(m+1)+2}{3}\rceil =m+1+j$ if $\lceil \frac{m+3}{3}\rceil +1\le j\le m.$

      2. when $m\equiv 3,5 (\text{mod} 6),w{t}_{\phi }(x_2{y}_2^j)=\lceil \frac{i(m+1)-2}{3}\rceil +m+1-\phi (x_2)+j+0=\lceil \frac{i(m+1)-2}{3}\rceil +m+1-\lceil \frac{i(m+1)-2}{3}\rceil +j=m+1+j$ if $1\le j\le \lceil \frac{m}{3}\rceil ,$ otherwise, $w{t}_{\phi }(x_2{y}_2^j)=\lceil \frac{i(m+1)-2}{3}\rceil +m+1-2\phi (x_2)+j+\lceil \frac{2m}{3}\rceil =\lceil \frac{i(m+1)-2}{3}\rceil +m+1-2\lceil \frac{i(m+1)-2}{3}\rceil +j+\lceil \frac{i(m+1)-2}{3}\rceil =m+1+j$ if $\lceil \frac{m}{3}\rceil +1\le j\le m.$

   3. For $i\ge 3$ and $1\le j\le m,$

      1. $w{t}_{\phi }(x_i{y}_i^j)=\lceil \frac{i(m+1)-2}{3}\rceil +(i-1)(m+1)-2\phi (x_i)+j+\lceil \frac{i(m+1)-2}{3}\rceil =\lceil \frac{i(m+1)-2}{3}\rceil +(i-1)(m+1)-2\lceil \frac{i(m+1)-2}{3}\rceil +j+\lceil \frac{i(m+1)-2}{3}\rceil =(i-1)(m+1)+j$ if $m\equiv 1 (\text{mod} 6),i\equiv 0,1 (\text{mod} 3)$ or $m\equiv 3 (\text{mod} 6),i\equiv 0,2 (\text{mod} 3)$ or $m\equiv 5 (\text{mod} 6),i\ge 3.$

      2. $w{t}_{\phi }(x_i{y}_i^j)=\lceil \frac{i(m+1)+2}{3}\rceil +(i-1)(m+1)-2\phi (x_i)+j+\lceil \frac{i(m+1)+2}{3}\rceil =\lceil \frac{i(m+1)+2}{3}\rceil +(i-1)(m+1)-2\lceil \frac{i(m+1)+2}{3}\rceil +j+\lceil \frac{i(m+1)+2}{3}\rceil =(i-1)(m+1)+j$ if $m\equiv 1 (\text{mod} 6),i\equiv 2 (\text{mod} 3)$ or $m\equiv 3 (\text{mod} 6),i\equiv 1 (\text{mod} 3).$ Take note that $i\ne 1,2$ in (iii)(A) and (iii)(B).

2. $w{t}_{\phi }(x_i{x}_{i+1})=\phi (x_i)+\phi (x_i{x}_{i+1})+\phi (x_{i+1}).$

   1. For i = 1,

      1. $w{t}_{\phi }(x_1{x}_2)=0+\lceil \frac{m-1}{3}\rceil +\lceil \frac{(i+1)(m+1)+2}{3}\rceil =\lceil \frac{m+1}{3}\rceil +\lceil \frac{2m+4}{3}\rceil =\frac{1}{3}[(m-1)+2(m+2)]=m+1$ if $m\equiv 1 (\text{mod} 6).$

      2. $w{t}_{\phi }(x_1{x}_2)=0+\lceil \frac{m+1}{3}\rceil +\lceil \frac{(i+1)(m+1)-2}{3}\rceil =\lceil \frac{m+1}{3}\rceil +\lceil \frac{2m}{3}\rceil =\frac{1}{3}[(m+3)+2m]=m+1$ if $m\equiv 3 (\text{mod} 6).$

      3. $w{t}_{\phi }(x_1{x}_2)=0+\lceil \frac{m+1}{3}\rceil +\lceil \frac{(i+1)(m+1)-2}{3}\rceil =\lceil \frac{m+1}{3}\rceil +\lceil \frac{2m}{3}\rceil =\frac{1}{3}[(m+1)+(m+2)]=m+1$ if $m\equiv 5 (\text{mod} 6).$

   2. For $i\ge 2,$

      1. when $i\equiv 0 (\text{mod} 3),w{t}_{\phi }(x_i{x}_{i+1})=\lceil \frac{i(m+1)-2}{3}\rceil +i(m+1)-2\phi (x_i)-\lceil \frac{m-3}{3}\rceil +\lceil \frac{(i+1)(m+1)-2}{3}\rceil =\lceil \frac{i(m+1)-2}{3}\rceil +i(m+1)-2\lceil \frac{i(m+1)-2}{3}\rceil -\lceil \frac{m-3}{3}\rceil +\lceil \frac{(i+1)(m+1)-2}{3}\rceil =i(m+1)+\frac{1}{3}[-i(m+1)-(m-1)+i(m+1)+(m-1)]=i(m+1)$ if $m\equiv 1 (\text{mod} 6),$ otherwise, $w{t}_{\phi }(x_i{x}_{i+1})=\lceil \frac{i(m+1)-2}{3}\rceil +i(m+1)-2\phi (x_i)-\lceil \frac{m+3}{3}\rceil +\lceil \frac{(i+1)(m+1)+2}{3}\rceil =i(m+1)+\frac{1}{3}[-i(m+1)-(m+3)+i(m+1)+(m+3)]=i(m+1)$ if $m\equiv 3 (\text{mod} 6).$

      2. when $i\equiv 1 (\text{mod} 3),w{t}_{\phi }(x_i{x}_{i+1})=\lceil \frac{i(m+1)-2}{3}\rceil +i(m+1)-2\phi (x_i)-\lceil \frac{m+3}{3}\rceil +\lceil \frac{(i+1)(m+1)+2}{3}\rceil =i(m+1)+\frac{1}{3}\{-[i(m+1)-2]-(m+5)+[i(m+1)-2]+(m+5)\}=i(m+1)$ if $m\equiv 1 (\text{mod} 6),$ otherwise, $w{t}_{\phi }(x_i{x}_{i+1})=\lceil \frac{i(m+1)+2}{3}\rceil +i(m+1)-2\phi (x_i)-\lceil \frac{m-3}{3}\rceil +\lceil \frac{(i+1)(m+1)-2}{3}\rceil =i(m+1)+\frac{1}{3}\{-[i(m+1)+2]-(m-3)+[i(m+1)+2]+(m-3)\}=i(m+1)$ if $m\equiv 3 (\text{mod} 6).$

      3. when $i\equiv 2 (\text{mod} 3),w{t}_{\phi }(x_i{x}_{i+1})=\lceil \frac{i(m+1)+2}{3}\rceil +i(m+1)-2\phi (x_i)-\lceil \frac{m-3}{3}\rceil +\lceil \frac{(i+1)(m+1)-2}{3}\rceil =i(m+1)+\frac{1}{3}\{-[i(m+1)+2]-(m-1)+[i(m+1)+2]+(m-1)\}=i(m+1)$ if $m\equiv 1 (\text{mod} 6),$ otherwise, $w{t}_{\phi }(x_i{x}_{i+1})=\lceil \frac{i(m+1)-2}{3}\rceil +i(m+1)-2\phi (x_i)-\lceil \frac{m+3}{3}\rceil +\lceil \frac{(i+1)(m+1)-2}{3}\rceil =i(m+1)+\frac{1}{3}\{-[i(m+1)-2]-(m+3)+[i(m+1)-2]+(m+3)\}=i(m+1)$ if $m\equiv 3 (\text{mod} 6).$

      4. $w{t}_{\phi }(x_i{x}_{i+1})=\lceil \frac{i(m+1)-2}{3}\rceil +i(m+1)-2\phi (x_i)-\lceil \frac{m+1}{3}\rceil +\lceil \frac{(i+1)(m+1)-2}{3}\rceil =i(m+1)+\frac{1}{3}[-i(m+1)-(m+1)+i(m+1)+(m+1)]=i(m+1)$ if $m\equiv 5 (\text{mod} 6).$

It clearly shows that the edge weights of all edges in $P_n\odot m{K}_1$ are distinct integers from the set $\{1,2,\dots ,n(m+1)-1\},$ which means that all edges have distinct weights. Thus, the total k-labeling $\phi$ is an edge irregular reflexive k-labeling of $P_n\odot m{K}_1$ and k is the reflexive edge strength of $P_n\odot m{K}_1,$ where m is odd. This completes the proof. □

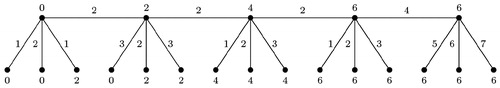
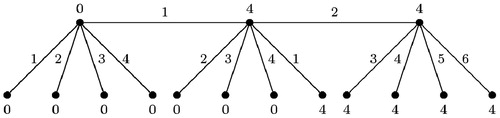
Figures 3 and 4 show the corresponding edge irregular reflexive 7-labeling of $P_5\odot 3K_1$ and edge irregular reflexive 8-labeling of $P_4\odot 5K_1,$ respectively.

Figure 3. An edge irregular reflexive 7-labeling for $P_5\odot 3K_1.$

Figure 4. An edge irregular reflexive 8-labeling for $P_4\odot 5K_1.$

In the next lemma, we deal with $P_n\odot m{K}_1$ when m is even.

Lemma 3.

For $n\ge 2$ and m even, $$\mathit{res}(P_n\odot m{K}_1)=\{\begin{array}{ll}\lceil \frac{n(m+1)-1}{3}\rceil ,& \mathrm{ }\text{if}\mathrm{ }n(m+1)-1\cancel{\equiv }2,3 (\mathrm{mod}\mathrm{ }6),\\ \lceil \frac{n(m+1)-1}{3}\rceil +1,& \mathrm{ }\text{if}\mathrm{ }n(m+1)-1\equiv 2,3 (\mathrm{mod}\mathrm{ }6).\end{array}\mathrm{ }$$

Proof.

Since the number of edges of $P_n\odot m{K}_1$ is $n(m+1)-1,$ by Lemma 1, we obtain the lower bound as shown in (1). Now, we prove that k is the upper bound for $\mathit{res}(P_n\odot m{K}_1)$ when m is even. We first define a total k-labeling $\phi$ of $P_n\odot m{K}_1.$

1. All vertices x_i and $y_i^j$ are labeled as follows.

   1. For $i\ne 3,$

      1. $\phi (x_1)=0.$ Then, $\phi (x_i)=\lceil \frac{i(m+1)+2}{3}\rceil$ if $m\equiv 0 (\text{mod} 6),i\equiv 3,4 (\text{mod} 6)$ or $m\equiv 2 (\text{mod} 6),i\equiv 1 (\text{mod} 6)$ or $m\equiv 4 (\text{mod} 6),i\equiv 2,3 (\text{mod} 6).$ Next, $\phi (x_i)=\lceil \frac{i(m+1)}{3}\rceil$ if $m\equiv 0 (\text{mod} 6),i\equiv 5 (\text{mod} 6)$ or $m\equiv 4 (\text{mod} 6),i\equiv 1 (\text{mod} 6).$ Otherwise, $\phi (x_i)=\lceil \frac{i(m+1)-2}{3}\rceil .$

      2. The vertices $\phi (y_i^j)$ are labeled as follows. $$\phi (y_i^j)=\{\begin{array}{l}\begin{array}{ll}0,& \text{if} i=1,m\equiv 0,2 (\mathrm{mod} 6),1\le j\le \lceil \frac{2m}{3}\rceil ,\\ & \text{or} m\equiv 4 (\mathrm{mod} 6),1\le j\le \lceil \frac{2(m+2)}{3}\rceil ,\\ & \text{if} i=2,m\equiv 0 (\mathrm{mod} 6),1\le j\le \lceil \frac{m-3}{3}\rceil ,\\ & \text{or} m\equiv 2 (\mathrm{mod} 6),1\le j\le \lceil \frac{m}{3}\rceil ,\\ & \text{or} m\equiv 4 (\mathrm{mod} 6),1\le j\le \lceil \frac{m+3}{3}\rceil ,\\ \lceil \frac{2m}{3}\rceil ,& \text{if} i=1,m\equiv 0,2 (\mathrm{mod} 6),\lceil \frac{2m}{3}\rceil +1\le j\le m,\\ & \text{or} i=2,m\equiv 0 (\mathrm{mod} 6),\lceil \frac{m-3}{3}\rceil +1\le j\le m,\\ & \text{or} m\equiv 2 (\mathrm{mod} 6),\lceil \frac{m}{3}\rceil +1\le j\le m,\\ \begin{array}{l}\lceil \frac{2(m+2)}{3}\rceil ,\\ \\ \phi (x_i),\end{array}& \begin{array}{l}\text{if} i=1,m\equiv 4 (\mathrm{mod} 6),\lceil \frac{2(m+2)}{3}\rceil +1\le j\le m,\\ \text{or} i=2,m\equiv 4 (\mathrm{mod} 6),\lceil \frac{m+3}{3}\rceil +1\le j\le m,\\ \text{otherwise}.\end{array}\end{array}\end{array}$$

   2. For i = 3 and $1\le j\le m,\phi (x_3)=\phi (y_3^j)=m.$

2. The edges $x_i{y}_i^j$ and $x_i{x}_{i+1}$ are labeled as follows. .

   1. For $i\ne 3,$

      1. $\phi (x_1{y}_1^j)=j$ if $m\equiv 0,2 (\text{mod} 6),1\le j\le \mathrm{ }\lceil \frac{2m}{3}\rceil$ or $m\equiv 4 (\text{mod} 6),1\le j\le \mathrm{ }\frac{2(m+2)}{3},$ otherwise, $\phi (x_1{y}_1^j)=j-\phi (x_2).$ Next, $\phi (x_2{y}_2^j)=m+1-\phi (x_2)+j$ if $m\equiv 0 (\text{mod}6),1\le j\le \lceil \frac{m-3}{3}\rceil$ or $m\equiv 2 (\text{mod} 6),1\le j\le \lceil \frac{m}{3}\rceil$ or $m\equiv 4 (\text{mod} 6),1\le j\le \mathrm{ }\lceil \frac{m+3}{3}\rceil ,$ otherwise, $\phi (x_2{y}_2^j)=m+1-2\phi (x_2)+j.$ For $i\ge 4,1\le j\le m,\phi (x_i{y}_i^j)=(i-1)(m+1)-2\phi (x_i)+j.$

      2. $\phi (x_1{x}_2)=\lceil \frac{m+1}{3}\rceil$ if $m\equiv 0,2 (\text{mod} 6),$ otherwise, $\phi (x_1{x}_2)=\lceil \frac{m-1}{3}\rceil .$ Next, $\phi (x_2{x}_3)=i(m+1)-2\phi (x_i)-\lceil \frac{m-2}{3}\rceil$ if $m\equiv 0,2 (\text{mod} 6),$ otherwise, $\phi (x_2{x}_3)=i(m+1)-2\phi (x_i)-\lceil \frac{m-4}{3}\rceil .$ For $i\ge 4,$ $$\phi (x_i{x}_{i+1})=\{\begin{array}{ll}i(m+1)-2\phi (x_i)-\lceil \frac{m-2}{3}\rceil ,& \text{if} m\equiv 0(\text{mod} 6),i\cancel{\equiv }2(\mathrm{mod} 6),\\ & \text{or} m\equiv 2(\mathrm{mod} 6),i\equiv 1(\mathrm{mod} 2),\\ i(m+1)-2\phi (x_i)-\lceil \frac{m-4}{3}\rceil ,& \text{if} m\equiv 4(\mathrm{mod} 6),i\equiv 3(\mathrm{mod} 6),\\ i(m+1)-2\phi (x_i)-\lceil \frac{m}{3}\rceil ,& \text{if} m\equiv 4(\mathrm{mod} 6),i\cancel{\equiv }3(\mathrm{mod} 6),\\ i(m+1)-2\phi (x_i)-\lceil \frac{m+4}{3}\rceil ,& \text{otherwise}. \end{array}$$

   2. For i = 3,

      1. $\phi (x_3{y}_3^j)=2+j$ if $1\le j\le m.$

      2. $\phi (x_3{x}_4)=i(m+1)-2\phi (x_i)-\lceil \frac{m+4}{3}\rceil$ if $m\equiv 0,2(\text{mod} 6),$ otherwise, $\phi (x_3{x}_4)=i(m+1)-2\phi (x_i)-\lceil \frac{m}{3}\rceil$ if $m\equiv 4(\text{mod} 6).$

Clearly, all vertex labels and edge labels are at most k under the labeling $\phi ,$ thus, labeling $\phi$ is a total k-labeling of $P_n\odot m{K}_1.$ Using similar approach as in the proof of Lemma 2, we are able to find the edge weights of all edges in $P_n\odot m{K}_1.$ $$w{t}_{\phi }(x_i{x}_{i+1})=\phi (x_i)+\phi (x_i{x}_{i+1})+\phi (x_{i+1})$$ and $$w{t}_{\phi }(x_i{y}_i^j)=\phi (x_i)+\phi (x_i{y}_i^j)+\phi (y_i^j).$$

Therefore, the results of edge weights are: (a) $w{t}_{\phi }(x_i{x}_{i+1})=m+1$ if i = 1, otherwise, $w{t}_{\phi }(x_i{x}_{i+1})=i(m+1)$ if $i\ge 2;$ and (b) $w{t}_{\phi }(x_i{y}_i^j)=j$ if i = 1, $w{t}_{\phi }(x_i{y}_i^j)=m+1+j$ if i = 2, $w{t}_{\phi }(x_i{y}_i^j)=2(m+1)+j$ if i = 3, otherwise, $w{t}_{\phi }(x_i{y}_i^j)=(i-1)(m+1)+j$ if $i\ge 4.$

We can see that the edge weights of all edges in $P_n\odot m{K}_1$ are distinct integers from the set $\{1,2,\dots ,n(m+1)-1\},$ in other words, every edge has a distinct weight. Thus, the total k-labeling $\phi$ is an edge irregular reflexive k-labeling of $P_n\odot m{K}_1$ and k is the reflexive edge strength of $P_n\odot m{K}_1,$ where m is even. This completes the proof. □

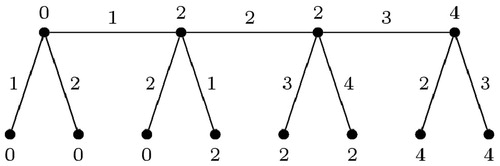
Figures 5 and 6 show the corresponding edge irregular reflexive 4-labeling of $P_4\odot 2K_1$ and edge irregular reflexive 6-labeling of $P_3\odot 4K_1,$ respectively.

Figure 5. An edge irregular reflexive 4-labeling for $P_4\odot 2K_1.$

Figure 6. An edge irregular reflexive 6-labeling for $P_3\odot 4K_1.$

Combining Lemmas 2 and 3, we obtain the concluding result for the reflexive edge strength of corona product of path with mK₁ as follows.

Theorem 2.

For $n\ge 2$ and all positive integers m, $$\mathit{res}(P_n\odot m{K}_1)=\{\begin{array}{ll}\lceil \frac{n(m+1)-1}{3}\rceil ,\mathrm{ }& \mathit{\text{if}}\mathrm{ }n(m+1)-1\cancel{\equiv }2,3 (\mathrm{mod}\mathrm{ }6),\\ \lceil \frac{n(m+1)-1}{3}\rceil +1,& \mathit{\text{if}}\mathrm{ }n(m+1)-1\equiv 2,3 (\mathrm{mod}\mathrm{ }6).\mathrm{ }\end{array}\mathrm{ }$$

5. Conclusion

This paper has successfully determined the reflexive edge strength of corona product of graphs, that is, corona product of two paths and corona product of a path with isolated vertices, where these graphs have also proven to admit the edge irregular reflexive labeling. Moreover, these generalized results are not only strengthened the Conjecture 1, but also thoroughly replaced the weak and restricted results of the previous paper [19]. Last but not least, this interesting study found a problem that worths for further investigation, that is:

Problem 1.

Determine the reflexive edge strength of corona product of a path P_n with m copies of complete graphs, i.e., $\mathit{res}(P_n\odot m{K}_t),$ where $n,m,t\ge 2$ and all positive integers m.

Acknowledgement

The authors would like to thank the referees for their valuable comments that improved the paper.

Disclosure statement

No potential competing interest was reported by the authors.

Additional information

Funding

This research is supported by the Fundamental Research Grant Scheme (FRGS), Phase 1/2020, Ministry of Higher Education, Malaysia with Reference Number FRGS/1/2020/STG06/UMT/02/1 (Grant Vot. 59609).