Developing ‘deep mathematical thinking’ in geometry with 3- and 4-year-olds: a collaborative study between early years teachers and university-based mathematicians

ABSTRACT

Mathematics in early years settings is often restricted to learning to count and identifying simple shapes. This is partly due to the narrow scope of many early years curricula and insufficient teacher training for exploring deeper mathematical concepts. We note that geometry is an area particularly neglected. In an innovative year-long project, a group of university-based mathematicians and early years teachers collaborated on a child-led exploration of ‘patterns in nature.’ The early years teachers ran the project within the setting, meeting regularly with the mathematicians to discuss potential areas of interest, and to highlight the children’s mathematical thinking. We found that, with the appropriate environment and guidance, the children naturally displayed deep levels of geometrical thinking and found enjoyment and satisfaction in the exploration of mathematical ideas. We define what we mean by the term ‘deep geometrical thinking’ and demonstrate this by looking at three excerpts through the lens of the van Hiele levels of geometric thought, finding that the children are capable of exhibiting thought at level 3 (abstraction), more advanced than previously thought of children of this age. Using a second taxonomy we also assess the range of skills across which they are demonstrating such geometric thought.

The mathematical development of young children has been the subject of much investigation over the last 20 or so years (MacDonald & Murphy, 2019), leading many to conclude that that they are capable of much deeper mathematical thinking than was previously thought (Reikerås et al., 2012). For example, Gelman (2006) and Zur and Gelman (2004) found that children aged 2½ to 4 years could demonstrate some arithmetic reasoning, and a grasp of the cardinality of numbers. Children as young as 3 years of age show themselves to be competent logical problem-solvers (Andrews & Trafton, 2002; Carpenter et al., 1993) and even 2-year-olds are able to grasp concepts such as one-one correspondence (Tirosh et al., 2020). Ginsburg et al. (2008) found children aged 3 to 5 years old to be capable of grasping and exploring a range of mathematical concepts, including abstract ones as well as ones relating to objects.

The Early Years Foundation Stage (EYFS) curriculum in England is prescribed by the government and covers the learning standards schools and childcare providers must meet for children up to age 5 (Department for Education, 2017, p. 8). According to this curriculum, educators must provide “ … children with opportunities to develop and improve their skills in counting, understanding and using numbers, calculating simple addition and subtraction problems; and to describe shapes, spaces, and measure..”¹ It is therefore unsurprising that preschool mathematics activities are often limited to numbers, for example, learning to count and understanding cardinality, and simple shape recognition (Clements & Sarama, 2018). This goal can lead to a prescriptive, algorithmic approach to mathematics teaching, even in an early years setting, as children are prepared to meet the requirements set out for their first school year.

Van Oers (2010) argues against training younger and younger children in mathematical operations, but instead for promoting mathematical thinking. Toddlers and young children are constantly making meaning of the world around them and the concepts they encounter, categorizing things and ideas, and updating their inbuilt ‘definitions’ when their own are at odds with those of others (Garvis & Nislev, 2017; Worthington et al., 2019). “Quality preschool mathematics is not elementary arithmetic pushed onto younger children. Instead, it invites children to experience mathematics as they play in, describe, and think about their world.” (Clements, 2001, p. 1). In a similar vein, Charles and Carmel (2005) propose anchoring a mathematical education around ‘Big Ideas’ rather than particular tasks (counting, arithmetic, shape recognition), since this will develop deeper understanding, and enable the children to make connections between seemingly disparate parts of mathematics and to apply the mathematical concepts to a diverse range of phenomena.

The importance of geometry

Mix and Cheng (2012) and Gunderson et al. (2012) found geometrical thinking to be a crucial basis for many parts of mathematics. Mamolo et al. (2015) demonstrated that approaching advanced mathematical concepts² using geometrical thinking made them much more widely accessible. Rittle-Johnson et al. (2019) found evidence that spatial reasoning in the early years is a strong predictor of future mathematics achievement. However, it has been noted that despite the clear importance of geometry and spatial reasoning for future mathematical progress, these areas are seldom explored in early years settings or related research (Clements & Sarama, 2011; National Council of Teachers of Mathematics, 2006), or in the education of early years teachers (Dindyal, 2015; Ginsburg et al., 2006; Moss et al., 2015).

In Piagetian terms, this makes complete sense; very young children are not expected to have a deep understanding of mathematics. His work stated that our cognitive abilities develop in sequential stages that are age-related and qualitatively different (Piaget & Inhelder, 1956). The ability to think logically is a later development (an element of the Concrete Operational stage which was said to develop around the age of 7 years). However, more recently the work of others has questioned Piaget’s theory of cognitive development, for example, some suggest he may have significantly underestimated what young children are capable of doing and understanding (see, Gelman et al., 1986; Spelke & Newport, 1998).

In a study of 3- to 6-year-olds, Clements and Samara (2000) found that young children’s concepts about geometric shapes have stabilized (though not necessarily accurately) by 6 years old. Given the relative performance of 3- and 6-year-olds, they conclude that to promote geometric learning and the posing and solving of interesting questions, it is important for teachers to provide good learning opportunities rather than to focus on the perceived “developmental level” of their students. . Many studies and summaries of “early years” geometry in fact focus on 4 – 8- year-olds, and on their ability to perform specific tasks such as shape recognition or classification (see for example, Dindyal, 2015; Gagatsis et al., 2006; Sinclair & Bruce, 2015). As well as being older, these children are likely to be learning within a much more structured and inflexible curriculum, making an open-ended child-led exploration of geometry less feasible. During our literature search, we have not found accounts of 3- and 4-year-old children who were actively engaged in explorations of geometry, and in this paper we aim to begin to address this gap.

In a geometrical context (distinguishing between and defining different shapes), Tsamir et al. (2015, p. 499) state that “Within the domain of mathematics, having precise definitions for concepts provides the foundation for building mathematical theories and ensures mathematical coherence.” This remains true throughout a mathematical education (Alcock & Simpson, 2009). It is therefore crucial that early years educators are comfortable not only with certain definitions, but with the idea of a definition; and how we use them to name or categorize objects. Interestingly, Harper and Daane (1998) found that geometry was the area associated with the highest level of anxiety in their cohort of preservice elementary teachers. What is clear from the literature is that the importance of geometry in early education has long been acknowledged, but that we are caught in a cycle where the future teachers of a given generation mostly receive a lackluster and formulaic mathematical education, do not receive adequate preparation for their own mathematical teaching, and are therefore ill-equipped to engage their pupils in deep geometrical thinking. “There is no doubt, early childhood teachers should be not only be well-qualified but more specifically have a strong background in geometry and a strong interest generally in mathematics education. The challenge will be to select, prepare and retain good teachers who can confidently teach geometry.” (Dindyal, 2015, p. 527).

Theoretical framework

By ‘deep geometrical thinking’ in this paper we mean noticing and considering the properties of shapes or figures, or the relationships between different shapes, and making related deductions. This is in contrast to working at a level where shapes are named but their properties are not considered. We will primarily use the van Hiele levels of geometric thought (Crowley, 1987; Van Hiele, 1986) to evidence the level at which the children in this study are thinking.

The van Hieles proposed that there were five levels of geometric thought, described as follows:

• Level 1 – Visualization: Figures are recognized by appearance alone, and often compared to some known object. The properties of the figure are not necessarily noticed.

• Level 2 – Analysis: Students see figures as collections of properties, and can recognize and name geometric properties, but they usually do not see the relationships between the properties, or appreciate which properties are necessary and sufficient.

• Level 3 – Abstraction: Students perceive relationships between properties and between figures. Students can create meaningful definitions of shapes, and can give informal arguments to justify their reasoning. Students can understand logical implications and inclusions, such as a square being a type of rectangle. Students do not yet understand formal deduction.

• Level 4 – Deduction: Students can construct proofs, understand the role of axioms and definitions and appreciate necessary and sufficient conditions.

• Level 5 – Rigor: Students understand the formal aspects of deduction, can understand various types of proof and can understand non-Euclidean systems.

These are sometimes given on a scale from 0–4, but we will use 1–5 throughout, as in (Mason, 1998).

A study by Fujita and Jones (2006) found that around two thirds of their cohort of primary teachers were at or below van Hieles’ Level 2 (analysis), unable to think analytically or descriptively about simple geometric shapes, for example, to mention right angles when asked to describe the necessary properties of a square. When geometry is so key to mathematical thought, this is a serious problem. Stephen Hawking said “Equations are just the boring part of mathematics. I attempt to see things in terms of geometry” (Larsen, 2005, p. 43), yet geometry was the least popular area of mathematics among teachers surveyed by Clements and Sarama (2011). The van Hieles argued that geometric thinking could be accelerated by good teaching (Mason, 1998), making this a pressing issue. “When teachers are not confident about their own geometrical knowledge then this can have a long-lasting negative impact on children’s learning” (Dindyal, 2015, p. 525).

A study of prospective teachers by Gutiérrez-Rubio et al. (2020) found that the main perceived learning goals when teaching geometry in school were procedural (instrumental in Skempian terms (Skemp, 1978)), focussing only on making further progress in geometry (identifying shapes, calculating areas etc.) rather than relational and general (improved mathematical reasoning, everyday problem solving etc.). Hoffer (1981) claimed that there were five important skills developed through the study of geometry: visual skills (recognition, observing properties etc.), verbal skills (using correct terminology, communicating spatial concepts), drawing skills (representing 2D and 3D figures, communicating through drawing), logical skills (classification, recognizing essential properties, discerning patterns, forming and testing hypotheses, using counter-examples) and applied skills (applying geometric skills to real life), giving examples of how these skills might be demonstrated at different van Hiele levels of geometric thought. These skills clearly have benefits well beyond the scope of mathematics, and we will use Hoffer’s taxonomy as a secondary framework in our study.

We give further details of how we worked with the teachers and the geometrical ideas we focused on below.

Research questions

This collaboration between the early years teachers and the University-based mathematicians began with the teachers’ desire to develop a broader understanding and appreciation of mathematics, and to expose the children to new, challenging and exciting ideas; essentially, to break the cycle mentioned earlier. We were conscious that the mathematical focus within early years is often predominantly around number, and so were particularly keen to introduce geometrical concepts. The initial theme was ‘patterns in nature,’ but the focus quickly became ‘circles’ after a particular child, Henry, showed interest in the concentric circles visible in a tree slice.

Because the project arose ‘organically’ from the felt need of the early years teachers, there were no formal research questions or objectives. However, informally, our questions were

1. Which areas within math (if any) proved to be engaging to these 3- and 4-year-olds?;

2. How did this ongoing input from mathematicians affect the teachers’ felt ability and confidence to explore mathematics within the setting? .

Methods

This project took place from September 2020 to July 2021, and therefore mostly under strict Covid-19 restrictions. The University-based mathematicians were unable to visit the setting, and interacted only with the teachers, via approximately half-termly (6-weekly) Zoom meetings. The meetings were unstructured. At first, the academic mathematicians mostly spoke about potential areas to explore under the heading ‘Patterns in nature.’ As the year progressed the early years teachers would relay the ideas that were particularly interesting to the children, and the group would discuss associated concepts. There were occasionally suggestions from the academics for future activities, or ways to demonstrate a particular mathematical concept, but largely the discussion took place at an adult level, and the early years teachers then went on to decide what to pursue with the children, and how. The child-led nature of the project meant that often the children would become fascinated with an aspect of an activity that we had not considered, and so Nicola (the teacher leading the project) would think of ways to explore this, sometimes seeking advice by e-mail.

Discussion was wide-ranging, encompassing both particular mathematical ideas or even theorems, and more general concepts such as ‘When are two things the same?’ Or ‘What is a theorem?’ Sometimes diagrams were drawn via the Zoom whiteboard, or further resources were suggested. Approximately once or twice per month, the teachers would provide updates in the form of written journals, showing the progress of the project in a similar form to the excerpts in our Results section. The teachers took time to be reflective following the discussions, and to ensure they understood these new theories and ideas. Further reading and research were often required. It is important to note that not every idea was acted upon. The University-based mathematicians were not familiar with the children or with early years setting and so ideas were taken and tweaked by the teachers with their children’s well-being and engagement in mind.

In many ways this project fits into the body of research on ‘collaborative lesson study’ (Takahashi & McDougal, 2016). Specifically, the focus is on the teachers building their own expertise and knowledge, there is significant preparation and reflection required on the part of the teachers, it is ongoing over a long period of time and there is input from ‘knowledgeable others.’ Moss et al. (2015) developed an adapted collaborative lesson study scheme, in which the emphasis was shifted from finding geometry activities that would fill gaps in the children’s knowledge to engaging groups of teachers in geometrical activities such that they were motivated to learn. This combination of group work and genuine engagement in the problem proved effective in engaging both the teachers and young students’ interest in sophisticated geometry.

The setting

The early years setting in which the project took place was a Local Authority maintained school (meaning that it is funded and controlled by the local education authority), situated in a socioeconomically diverse community and currently rated ‘Outstanding’ (the highest of four possible ratings, only awarded if all or most aspects are judged as ‘outstanding’ and none as ‘requiring improvement’ or ‘inadequate’) by Ofsted (the Office for Standards in Education in the UK, who conduct regular inspections of schools and early years settings). Almost 20% of the 3- and 4-year-olds were eligible for pupil premium funding (additional funding provided by the UK government to help support children from disadvantaged backgrounds), and there is a high proportion of children with special educational needs (SEN).

The setting uses the Reggio Emilia philosophy as a basis for its own pedagogy. The Reggio Emilia approach is based on the concept of the hundred languages of children (Edwards et al., 1998), presenting new ideas and concepts in multiple forms to ensure that all children have the chance to understand and connect with the concepts being explored.³ A key element of the rationale for this project was to incorporate mathematics into these creative experiences, to encourage the children’s flexibility in problem-solving and divergent thinking skills (see, Bolden et al., 2010). The teachers’ collective aim is to offer rich, hands-on learning experiences that promote creative critical thinking through collaboration. Decisions are made with the children’s interests and characters at heart, so that the direction of a project will be directed by the group, with the teacher having prepared for an array of possible ‘next steps.’

Projects in the setting

In a ‘project’ in this early years setting, a subject (for example, bees, light, homes and houses) is investigated in an open-ended fashion, with the direction and process evolving. Projects are a context for experimentation by groups of children and teachers alike. “It evokes the idea of a dynamic process that always arises in relationships with others. Project work grows in many directions, with no predefined progression, no outcomes decided before the journey begins. It means being sensitive to the unpredictable results of children’s investigations and research.” (Rinaldi, 2005, p. 19).

Projects are centered on a child (or a group of children’s) wonders, recognizing and responding to a question or a sense of fascination or sustained engagement with a material or an object. “Project work in the Reggio schools is a collective and collaborative effort to make sense of the children’s most salient questions.” (Linder et al., 2011). This inquiry approach leads the group (including teachers) to explore further as equal partners, with a level of respect for one another. The teachers are honest about not always having the answers, but make it their business to pursue these lines of inquiry and embark upon a journey with the children. They enable the interested cohort of children, through thought provoking questions, new tools and materials and collections of specific objects, to further develop their understanding. The aim is not to guide investigations toward a single solution but to allow for different journeys and to facilitate interaction and construction of group learning. Together the group can construct and verify hypotheses and theories. A project is a journey of research, dissemination and formative experiences whereby children and adults collectively become a community of learners.

Each week a teacher delivered a project session, in which the group would reflect on their prior learning and a new mathematical concept, or some new vocabulary might be introduced. Throughout the week, the children had continual access to the learning environment to explore, consolidate, revisit and build upon these ideas, and teachers would be looking for opportunities to extend the children’s thinking and support their enquiries. Another project, on ‘homes and houses,’ was run concurrently, and continued throughout the academic year because of the sustained interest of the children. The children did not have to commit to one single project through the year. As part of the daily routine, a ‘plan, do and review’ process is followed, where the children feed back anything they believe to be significant to the project to the whole group. This encourages the children to take ownership of their own learning and ensures that everyone knows how the project is progressing. A project can and does run for as long as the children are showing interest and a desire to explore further. The ‘circles’ project (to be discussed below) seemed to engage a cohort of children and together they pursued many avenues, supporting one another’s strengths and their own debates and wonders. These children quickly became known as the leaders of the project, driving the project and feeding the learning to others daily.

The environment

In the Reggio Emilia approach the environment is considered ‘the third teacher’, after the parent and the classroom teacher (Strong-Wilson & Ellis, 2007), and so the spaces within the setting are thoughtfully arranged to enrich a child’s experience. A big part of the provision throughout the environment in this particular setting is loose parts. The ‘theory of loose parts’ was initially proposed by Nicholson (1972), an architect who noted that children love to play with ‘building block’ items such as buttons, clothes pegs, cardboard boxes and so on, and that not to include such items in their environment was to stifle their innate creativity. Loose parts have been shown to be a stimulating and popular part of a preschool environment (Griebling et al., 2016), and there is some evidence that they are particularly effective for scientific and mathematical learning (Ruzzi et al., 2017). Loose parts can include a whole host of resources from a small basket of gems to cable drums in the garden. Crucially, they have no inherent ‘meaning’ within the project, but encourage free play and exploration, and for the children to assign their own significance to the parts. Loose parts can support and develop creative and critical thinking skills and encourage children to experiment freely with new ideas.

During the ‘Circles’ project loose parts were offered continuously, allowing the children to take time to test out ideas and share thoughts, work together or alone in making meaning of their own hypothesis or that of someone else, including the work of mathematical theorists shared through the academics. Some loose parts used in the ‘Circles’ project included string, sticks, objects that could be taken apart and put together to form a shape, objects that displayed the ‘concentric-ness’ of a set of circles, rope lights, projections and found objects within the garden.

The teachers also prepared collections. A collection is formed in response to the interests and questions of the children. The objects within a collection are meaningful, allowing for the consolidation of ideas. For example, at the start of this project, the teachers gathered a collection of logs and other tree parts, supporting Henry’s initial wonder with a tree slice. Later in the year, a collection of circles was also offered. Once the children had been offered these collections or loose parts they were never removed (although they were sometimes enhanced), giving children time to explore in their own way. These collections stimulate the children as they think over items, concepts and subjects.

Participants

Involved in the collaboration were five University-based mathematicians and three early years teachers. Most of the mathematicians had been involved in outreach projects with older children, but none had any experience of early years education. The early years staff were two teachers from different areas within the setting, and the headteacher. None of the teachers involved had a higher mathematics qualification than GCSE (General Certificate of Secondary Education, a qualification taken by most UK students at the end of compulsory education, around age 16), and some said openly that they had disliked or struggled with mathematics at school, with experiences mostly involving rote learning and memorizing.

In total there were twenty-three children involved in the study. Some took part only occasionally, whereas a core group of twelve would almost always choose this project and would access the resources daily in their own time. Of the core twelve, there was an equal split of male and female, two vulnerable children, three entitled to pupil premium funding and three with noted speech or behavioral challenges. At the start of the study, the children were all 3 years old, ranging from just having turned 3 (Eva), to turning 4 in the next few weeks (Henry, Rose). The mean age was 3 years 5.5 months, and the standard deviation was 92 days. In October 2020 the children were each assessed against the EYFS primary and specific areas of learning, and found to be at the expected developmental level for their age. The areas encompassed in this assessment are personal, social and emotional development; communication and language; physical development; literacy and mathematics.

All names of those involved have been changed, and parental permission has been given for photos and transcripts to be included, as per the guidelines set out by the British Educational Research Association (BERA, 2018) . The sample clearly involves those children who took an interest in the project, and it comes from a small and very particular environment. However, we believe the setting and the children represent broadly typical examples of each. Given this, we argue that many similar early years settings are likely to be interested in these findings.

Data collection and analysis

The conversations in the excerpts that follow were transcribed in almost real time by the teachers while in the room, then written up later. The teachers are careful to note down the exact words of each child, although it is likely that there will be some small inaccuracies. The teachers are practised in doing this, as such write ups are regularly given to parents. Given the ongoing and often spontaneous nature of the relevant discussions and activities, it would have been difficult and arguably inappropriate to have made video and/or audio recordings.

Throughout the project these (and many more) write-ups were used to inform the University-based mathematicians as to the directions of enquiry. They also enabled us to highlight the level of geometrical (or more general mathematical) thinking being exhibited. These particular excerpts were selected by the authors from the journals provided by Nicola and the other teachers, because they demonstrate the rich range of mathematical ground that can be covered from an apparently narrow focus.

Results and discussion: the circles project

Among the many geometrical areas explored by the children, circles became something of a recurring theme. This started when Henry found a tree slice (as a part of a collection relating to the ‘Patterns in Nature’ theme) and became fascinated by the concentric circles within. The following three excerpts provide snapshots of some of the exchanges that took place within the project, demonstrating the careful guidance given by Nicola (the teacher), the deep and ongoing learning taking place in the children, as well as the freedom the children have to explore their interests and questions within the environment. Nicola’s thoughts and rationale are in italics, to distinguish from her speech.

Excerpt 1: thinking about a definition

Following lots of interest in concentric circles, Nicola decided to provoke the children into thinking about things another way. What follows is a discussion, first with the teacher, Nicola, and then an exchange between Nicola and several of the children.

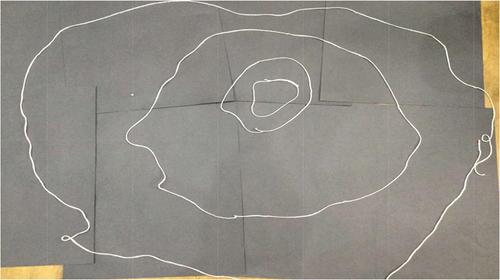
Nicola: The children all seem so certain that the “circles always get bigger and bigger” as they grow. This has been an ongoing inquiry and I can see why they are thinking that as originally we started looking at tree slices following Henry’s initial wonder. I am now wondering whether I should have a debate with the group and see what they think if I suggest the circles get smaller and smaller in size … Let’s see what their reactions to this theory are. I had already placed one large circle using the familiar material ‘string’ upon the black paper to allow the strong line of the circle to be seen clearly.

The string circles are shown in Figure 1.

Figure 1. The ‘circles’ created before and during the discussion. The outer ‘circle’ is the original ‘circle’ placed by Nicola, the inner ‘circles’ were placed during the discussion.

Nicola: What can you see?

Eva: It’s huge!

Rose: It’s like a footprint.

Harrison: It’s like a giant footprint.

Eva: It’s got a bottom and a top.

Rose: Like a concentric circle.

Roisin: Like a ball.

Everyone: Circle!

Harrison: Separate circles. It looks like a big turnip. (This is a connection to a previous story the children learned).

Rose: It’s a concentric circle because it’s big.

Harrison: No, only one circle.

Abbie: A big one!

Patrick: A huge circle!

Simon: It can’t (be a concentric circle) because we don’t have any more circles.

Patrick: We need more string to make it bigger.

Henry: You have to have a tiny one in the middle. (Retrieving earlier learning about the tree slice and the center point).

Rose: No, because there will only be one circle in the middle of that one. We need more! (Speaking with confidence and assertiveness).

Harrison: But one more is enough to make it a concentric circle.

In this exchange, the children display a range of deep geometrical thinking, as well as the ability to debate with one another and challenge ideas. The children are applying their knowledge of the properties of concentric circles to determine whether this first large circle is indeed a concentric circle. There is some misunderstanding of the crucial properties (‘It’s a concentric circle because it’s big’) but this is quickly challenged by Harrison and Simon, who remember that with concentric circles there are always multiple circles. Henry further adds that the new circles need to be in the middle. Whether or not it is clear in his mind that they share the same center is not obvious, but he is bringing in more information about how the circles must be arranged. Rose’s suggestion that more than one extra circle is needed is quickly challenged by Harrison, showing a deeper level of understanding of the necessary and sufficient properties of concentric circles: more than one extra circle would be permissible, but only one extra circle is strictly necessary. The children are debating the exact definition of ‘concentric circles’ in much the same way as the university-based mathematicians among us would do in our research.

The first few statements from the children demonstrate geometrical thinking at the van Hieles’ level 1 (visualization), naming the shape and comparing it to familiar objects (ball, footprint, turnip), but this is quickly superseded by thinking at levels 2 (analysis) and 3 (abstraction), naming geometric properties of the shape presented and of concentric circles. The children then move onto the idea of the exact definition and necessary and sufficient properties. Harrison, who initially compares the shape to a footprint and a turnip, is also the one to point out that only one extra circle is required to create concentric circles. Indeed, three different children (Harrison, Simon and Rose) directly challenge another child’s statement, demonstrating a high level of engagement and confidence.

In terms of the skills discussed by Hoffer (1981), the children are displaying visual skills at level 2 (analysis) by noticing the properties of a figure, verbal skills at level 3 (abstraction) by talking in terms of definitions and logical skills at level 3 by using properties of a figure to determine whether it belongs in a particular class.

A notable feature is the gentle but intentional way Nicola introduces the idea. Her opening question could lead to many different responses, and the children are given time to reflect on the knowledge they have acquired so far through the project, and to consider one another’s points of view. Nicola does have a learning agenda – to challenge the notion that concentric circles start in the center and get bigger and bigger – and she finds ways to guide the children into seeing concentric circles with a new level of thought.

Excerpt 2: spirals vs. circles

During the project, Henry brought a picture of a spiral to Nicola, as something that looked similar, but was somehow different. As Nicola introduced this new word ‘spiral,’ more conversation was sparked between the group.

Simon: I love spirals, them are my favorite. They go fast, round and round.

Abbie: I don’t love them.

Rose: The spiral gets bigger. The concentric circles is getting bigger, but they are separate.

Henry: The spiral is not separate – all one line.

(Thinking further about things)

Nicola: How do we know if we have concentric circles?

Simon: They are all inside each other.

Rose: They are all separate circles.

Hayley: The little ones sit in the big ones.

Nicola: What can you tell me about a spiral?

Henry: It curls around.

Hayley: It’s a curved line.

Patrick: It’s a long line.

In this brief exchange, the children have pinpointed one of the key points of difference between a set of concentric circles and a spiral: The spiral is made of one single line, whereas the concentric circles comprise two or more separate circles that are nested in this particular way.⁴ As the children focus together on drawing concentric circles and spirals, it is clear that unlike concentric circles, a spiral can be drawn in one fluid motion. This is an example of the phenomenon found by Thom and McGarvey (2015), that the act of drawing can cause children to become aware of geometric concepts. Explorations with string show that the spiral can be ‘unravelled’ and rearranged to form other, apparently very different, shapes, whereas the concentric circles are a fairly inflexible structure, remaining nested in their formation unless circles are broken or lines are crossed. Once again, the children have moved beyond the level 1 (visualization) act of naming the two figures, and are considering the properties that make them different. The children are demonstrating level 3 (abstraction) visual skills (Hoffer, 1981), in recognizing the similarities and differences between the concentric circles and spirals, level 3 verbal skills in speaking accurately about what distinguishes the two figures and level 3 logical skills in using the properties (in this case whether the figure is composed of a single line or several circles) to determine whether they belong to the same class.

Nicola then gave the group some time to put down on paper a mix of spirals and concentric circles (see, Figure 2):

Figure 2. Exploring spirals and concentric circles.

Nicola: A scene arose that was collaborative within the drawing process, but also within their own cognitive growth set, as children commented on one another’s drawings and talked through their own ideas. We had a mix of images, but one thing that was clear was that the spiral shape allowed for one continuous motion that got faster when the children were drawing, quickly becoming a cascade of overlapping lines. A graphic explosion of ‘curved lines.’ We then offered the children a sheet of acetate. We said to the children ‘It is up to you how you draw your circle and then we will project your image and see what the effect is. Does it look the same? Does it look different? What have you drawn’?

This touches on an idea that came up frequently in the discussions between the mathematicians and the teachers: ‘sameness’. Determining in which ways two objects, numbers, shapes, statements etc. are the same, or under what conditions they can be considered ‘the same’ (or ‘logically equivalent’), is an important area of mathematical thought that is often lacking in school aged children (Yopp, 2020). In Figure 2, the children are exploring this idea. Is a spiral drawn starting anti-clockwise ‘the same’ as one drawn starting clockwise, for example? Does it matter whether the shape is seen on paper, on acetate or on the wall? Here, the children are thinking about how a particular shape is defined, and what are its necessary and sufficient properties. Exploring whether, for example, two spirals drawn clockwise and anti-clockwise are the same by drawing them demonstrates Hoffer’s drawing skill at level 3 (abstraction).

Excerpt 3: measurements and theorems

The objective for the following session was to revisit some earlier concepts about circles, and to move on to thinking about the center point of the circle. Some of the objects in the collection presented by Nicola are shown in Figure 3. The collection included tree slices of varying diameters and depths, different sized circular mirrors, buttons, bottle tops, plates and jar lids.

Figure 3. Finding the center and measuring circles.

Nicola: The children entered the room with a collection of circular objects all ready to draw them in. They first sat down and looked at the resources in front of them. Then we began our usual exploratory conversation.

Everyone: Circles!

Henry: (Pointing) That’s bigger than all the rest. That medium and that the biggest.

Nicola: How do you know? How can you tell?

Henry: (Placing a tree slice on top of a large silver plate to compare) So when you put that on it bigger. Look, goes all around, see?

Nicola: Henry’s ability to visualize the difference in size was clear, but the other children remained a little unsure.

Nicola: What could we do to check they are bigger?

Jacob: That measuring tape there.

Simon: Measure things

Petra: It’s got numbers on.

Nicola: Henry then layered up the circles and measured straight across the top circle. I gave him time to play around with the tool, to see what he came back with in time. Everyone then had a little play with the measuring tools. This allowed for the words ‘diameter’ and ‘width’ to be used time and time again in the discussion. A natural flow of expressive language was being used between the group and through their explorations. We then brought our focus back together and explored how to correctly use the measuring tools, making sure the ‘0’ mark was at the edge (another word added to their vocabulary) of the circle, then placing the measurement tool straight through middle to the opposite edge of the circle. This led to more discussion as we noticed that we were not always placing the measurement tool across the widest part of the circle. I explained we need to make sure the ruler/tape is going across the widest part of the circle and to do that we need to find the exact center point. The point that we had been thinking about last week. How do we find the center point? Henry proceeded to point to instantly to the middle of the tree log slice and then I asked him how did he know this was the exact middle?

Henry: Because that’s the littlest circle in the middle.

Nicola: We proceeded with a demonstration of finding the middle by creating a square around the circle then using diagonal lines crossing straight through the middle. This tested out their problem-solving skills, with me just informing them of the next stage and allowing them to explore how this occurs. First of all discussing the properties of a square was interesting, (something again to come back to, perhaps test out the nesting of squares) then once this was established they each had a turn to create the square around the outside of the circle. The children are familiar with the string now and take their time to really maneuver it and place it with such care, demonstrating levels of dexterity. It was quite interesting to watch them figure out how the edges of the square needed to touch the edges of the circle. Eventually they succeeded in forming the square (Henry and Jacob being the ones to rectify it). Then we were able to bring in more language in the form of vertical, horizontal and diagonal lines, in order to complete the last part of finding that all important center of the circle.

Henry: Number one is vertical! A tree is straight when they fall down.

Nicola: Once they placed the lines corner to corner to the square then they were able to see the center of the circle. This was quite a discovery to them, and they seemed to love that the hard work had paid off to get to this point. As we now had the center mapped out the children all wanted to measure again to see what the diameter was using the widest part of the circle. More discussion arose as the children discovered that whichever point they go from on the edge of the circle, as long as the tape went through the middle they measured the same diameter. This occurred from them measuring the two diagonal pieces of string going right through middle of the circle. Henry was genuinely fascinated and in awe of this remarkable finding.

Henry: Can I stay in here and do this?

Nicola: This question was a powerful one in the sense that he was seeking permission to continue in his own discoveries, displaying real commitment. Henry wanted to measure all the circles in the set up.

In this exchange, there are several theorems encountered by the children. In mathematics, a theorem is a statement that, under some specified conditions, is always true. Firstly, Henry has his ‘theorem’ that if you put one circle on top of another, and you can still see the bottom circle all around the top one, the bottom one is the bigger of the two. Secondly, Nicola introduces a procedure to use a square to find the center of a circle. This is also a theorem: if you draw a square such that each side is tangent to a circle, its diagonals meet at the center of the circle. This is true for any circle. As Nicola notes, the children were thrilled with the results of their hard work, and that they now had this knowledge that applies to any circle. Using physical objects in this way demonstrates Hoffer’s Applied skill at level 2 (analysis). They quickly made another discovery, the third ‘theorem’: as long as you measure through the center of the circle, the distance from one edge to the other will be exactly the same. In this discovery, the children were touching upon exactly what distinguishes a circle from any other shape (a level 3 ‘abstraction’ concept).

This excerpt also demonstrates the value in ongoing and exploratory project work such as this. Henry is able to make the link to his existing knowledge that the smallest circle in a set of a concentric circles is at the center, and indeed the idea of the ‘centre’ of a circle is one that has been mentioned before to all. The children are also inspired to find measuring tapes, and therefore to use numbers in a way that is meaningful and useful to them.

General outcomes

What is clear from the above excerpts is that the children are capable of deep geometrical thinking (as we have defined it in the introduction), and of appreciating subtle concepts. They were interested and engaged, driving the discussion. For example, in the first two excerpts, many children gave their viewpoints, or assessed what another child has said. They did not always wait for Nicola to pose a question before voicing their thoughts or picking up on something new, and a large subset of the group were contributing. The discussions fed naturally into other aspects of mathematics, for example, measuring circles in Excerpt 3 led to discussion and comparison of numbers, but with the children arguably much more invested than if that had been the sole aim. The children are led to explore basic mathematical concepts in a flexible, open-ended, autonomous way. They regard these concepts as just as interesting, and just as much theirs to do what they like with, as anything else in their world. Sometimes they explore alone, but often in collaboration. The importance of the environment should not be underestimated here. Given various sets of ‘loose parts,’ mark-making materials and suitable stimuli the children are able to become engrossed in exploring mathematical ideas. We also see that the guidance of the teacher is an important catalyst for the children’s ideas and accomplishments, scaffolding and supporting these geometrical concepts.

The most striking outcome from the point of view of the University-based mathematicians was the similarity between the concepts and approaches taken by the children and themselves. For example, precise understanding of definitions (as in excerpt 1) is a crucial part of advanced mathematics, as is understanding the differences between apparently similar forms or concepts (as in excerpt 2). Furthermore, research-level mathematics is often very exploratory in nature, involving discussion with colleagues, much more like the approach these children take than a more traditional classroom setting. Without time as a barrier, and with loose parts and collections freely available, the children were able to freely explore their ideas and make connections. We also admired the respectful dialogue often heard as the children debated with one another and proposed alternative ways to approach a situation. We often remarked that this is exactly the sort of interaction we love to see in our undergraduate classes. There is a high value in playful learning through open discussions and discoveries, and the pleasure of creativity is often missing in conventional mathematics teaching at all levels. It has been refreshing to see the teachers and children exploring the richness of mathematics, going beyond narrow concepts, specific goals and achievements.

Because the nature of the collaboration between the University-based mathematicians and early years teachers had, from the start, been tailored to the child-led approach of the setting, it has been a sort of ‘teacher-led’ form of continual professional development (CPD). The mathematicians did no preparation beyond reading the latest write-ups provided by the teachers, and so the meetings were responsive and flexible. We often found ourselves discussing deep but simple ideas, such as ‘what does it mean for things to be the same?’ or ‘What is a theorem?’ The emphasis was more on revealing and exploring the mathematical thinking the children were already doing, as we have done here using the van Hiele and Hoffer taxonomies, rather than on imparting any new information or teaching any new methods. Indeed, one of the early years teachers commented: “The whole process of exploring these concepts and the thinking around them was invaluable to us as practitioners; it was almost like being given a set of keys to help us unlock children’s thinking into different spaces of deeper/higher level thinking.”

Conclusion and implications

We conclude by returning to the informal research questions posed in our Methods section.

Which areas within math (if any) proved to be engaging to these 3- and 4-year-olds?

The main striking point to arise from the excerpts in the Results section, and the project generally, was the capacity of the children to engage with geometrical concepts through their study of circles and some related ideas. We found that many of the children had a great appetite for geometrical ideas, making spontaneous links to geometry in ‘non-maths’ activities, spotting patterns in new environments and electing to spend more time carrying out their geometrical investigations. As demonstrated in the excerpts, the children are capable of displaying thinking well above the van Hieles’ level 1 (visualization) of simply recognizing and naming shapes. There are also examples of each of the five key skills associated with geometry by Hoffer (1981) at around level 3 (abstraction). Note that we are not claiming that these 3–4-year-old children are consistently operating at high van Hiele levels; rather, we use these levels as a way to present an ‘existence proof’ that children of this age can engage meaningfully with such thought. This taxonomy has been useful in helping the teachers in the study to appreciate and respond to the children’s ideas, words and strategies.

Adult emphasis on mathematics with small children often focusses on number (for example, in counting songs), but we would suggest that in fact numbers are simply not the most suitable or accessible aspect of mathematics for children of this age. The children in our project did introduce numbers, for example, in debating the necessary number of extra circles in Excerpt 1, and in measuring the diameters of circles in Excerpt 3, but they only appear because the children need them to answer a question with which they were engaged. By contrast, Lee (2012) uses a much broader definition of mathematics, and concludes (as we do) that toddlers are innate mathematicians. Children who chose not to take part in the ‘Patterns in Nature’ project would still be exposed to mathematical concepts, both in their chosen project and in the other sessions. For example, the ‘Homes and houses’ project running concurrently led to explorations of shape, tessellation, balance and scale.

Although in this paper we have focused on examples of deep and conceptual thinking that is beyond the scope of the EYFS curriculum, the setting also places an emphasis on exploring number and shape across the environment. In fact, this much more open-ended and discursive approach to mathematics enables the children to theorize and experiment together in a whole new branch of ways, and ones that, at times, may be much more accessible to children who perhaps are not confident with numbers. The use of ‘loose parts’ to form the circles and other shapes lends a generality to what is being learned. String, sticks, stones, tree slices, pen/paint drawings have all been used to create the geometric objects, and almost interchangeably, so that it is the form and essential properties that are the focus, rather than the particular material. This focus on the abstract ideal rather than the specific example is essential to mathematics (Clements & Samara, 2000).

How did this ongoing input from mathematicians affect the teachers’ felt ability and confidence to explore mathematics within the setting?

This style of teaching is demanding for the teacher (Björklund, 2012), perhaps especially in a mathematics context, as the teacher is committed to carrying out their own research as they consider possible future directions. As well as being aware of the children’s personalities, communication styles and goals, they must have a sufficient command of the mathematical content to guide the children appropriately, but the benefits are undeniable and, in our opinion well worth the effort. Nicola was able to place the large circle in Excerpt 1, putting forward the somewhat controversial notion that the circles can be seen as getting smaller and smaller. While this is not a complex idea in itself, it requires a confidence and way of thinking about mathematics that may not come naturally to all.

The teachers commented that this collaboration had empowered them to explore the mathematical aspects of activities that often arise in the setting. For example, in most years children notice patterns in the natural world (as Henry noticed concentric circles in the tree slice), but staff would invariably develop this into a project on trees (for example) rather than geometry.

Our use of the van Hiele levels and Hoffer skills has been invaluable in drawing attention to ‘higher level’ geometrical thinking, and for highlighting how such skills are demonstrated. The head teacher used the analogy of having various lenses through which staff see the children, according to the areas of the early years curriculum (communication and language, understanding the world, personal social and emotional development and so on). The project was precipitated by a general acknowledgment that the ‘mathematics’ lens was by far the least in focus, but the collaboration has sharpened it enormously.

The project also benefitted from the relative flexibility of the curriculum at this age; it is doubtful that, within the current curriculum, a teacher of older children would be able to run a child-led project in a similar way. This sparks a debate about the purpose of a mathematics education. Should teachers and teaching be less prescribed in order that this type of wonder for mathematics can be engendered in children? How can mathematics education escape the system of ‘instrumental’ understanding and nurture a genuine ‘relational’ understanding and appreciation for the subjects (Skemp, 1978). Certainly, if this is to happen, teachers need access to much more mathematical education themselves. As with Moss et al. (2015), we found evidence that a more teacher-led approach to CPD leads to increased confidence and mathematical ability. This style of collaboration is an aspect of the research we plan to explore further.

Limitations and future directions

This case study involves only a relatively small group of children and early years teachers, in a particular environment. We acknowledge that this makes the samples unique. However, we believe that both groups were quite typical in terms of their mathematical ability and their attitude to mathematics at the outset of the project. We feel that it is therefore very likely that other early years settings and the staff within will find resonance with the findings we have set out in this paper.

We conclude this section by identifying two key directions for further research. Firstly, we plan to conduct a much broader and less organic study into this form of collaborative, teacher-led CPD. We believe this project has had a positive effect on both the children and the teachers involved. Demonstrating its potential more generally will be achieved through links with a greater number of early years childcare providers, enlarging the scope of the collaboration. We will also be intentional in our use of evaluation and metrics, paying particular attention to any changes in attitude to mathematics among the educators and children, as well as assessing the children’s geometrical and/or mathematical thinking in a more systematic way.

The second avenue for future research would be to follow a number of children from this, or a similar, project through their education. Again, this would require a greater number of children, and the cooperation and input of follow-on schools.

Acknowledgments

First and foremost we are thankful to the children and parents for their cooperation. We would also like to thank Prof. John Parker, Dr. Karen Jones and Prof. Steve Higgins, for their help during the project, and the reviewers and editor for their time and insight.

Disclosure statement

No potential conflict of interest was reported by the author(s).

Additional information

Notes on contributors

Rachel Oughton

Rachel Oughton is an Assistant Professor of Statistics in the Department of Mathematical Sciences at Durham University and a member of the Mathematics Outreach Committee. Her main area of interest is Bayesian modelling for uncertainty quantification in complex systems. She is also interested in enabling children to explore and enjoy mathematics and tackling barriers to engagement.

Kathryn Nichols

Kathryn Nichols is an Early Years Educator who is passionate about Early Years education. Through her many years of working in Early Years she has developed a personal passion for children’s creative critical thinking. This project has enabled her to develop a real love and thirst for a broad and creative mathematical pedagogy, coinciding with her belief in allowing children to become curious and powerful individuals.

David S Bolden

David Bolden is an Associate Professor and Lecturer in Mathematics Education in the School of Education at Durham University. His research interests centre on early mathematics teaching and learning. He has particular interests in mathematical creativity, what it means to understand and the use of representations in developing understanding.

Sarah Dixon-Jones

Sarah Dixon-Jones is an Executive Head Teacher of two maintained nursery schools in the North East of England. Her main interests are in Early years education and the continuous professional development of staff.

Sam Fearn

Sam Fearn is an Assistant Professor (Education) in the Department of Mathematical Sciences, Durham University. His main area of interest is in the assessment of higher mathematics, and in particular automated assessment methods for higher mathematics.

Sophia Darwin

Sophia Darwin is an Assistant Professor (Education) in the Department of Mathematical Sciences at Durham University. She has a BA in English literature, a PGCE in secondary maths, and a PhD in maths (UCLA). Since 1986 she has taught at universities in England (Cambridge and Durham), the US (CUNY and UCLA) and Canada (University of Victoria). She is interested in how students manage the transition between school and university mathematics.

Mrita Mistry

Mrita Mistry is a qualified teacher with a PGCE specialising in the teaching of young children within the early years. She has been actively involved in this role for well over a decade and continues to be deeply passionate about how our young children learn and develop, particularly in the teaching and learning of mathematics as a language, which is often perceived as a challenging subject.

Norbert Peyerimhoff

Norbert Peyerimhoff is Professor of Mathematics at Durham University. His main areas of research are various topics related to geometry ranging from differential geometry to crystallography. He has been involved in a number of outreach activities at university, secondary and primary school level.

Adam Townsend

Adam Townsend in an Assistant Professor in the Department of Mathematical sciences at Durham University. His scientific research is in developing mathematical and computational techniques to investigate complex fluids; his public outreach has included work with the Ri, the BBC and the Science Museum. He co-founded the popular maths magazine Chalkdust.

Notes

1. In September 2021 ‘Shape, space and measure’ was replaced in the EYFS curriculum by ‘Numerical patterns’.

2. Optimization and rates of change, which they argue are conceptually interesting to young children.

3. There are examples of mathematical studies within the Reggio Emilia pedagogy, for example, Linder et al. (2011) and McLennan (2019), although these both focus on learning about number.

4. This difference links to a field of mathematics called topology. Topology is a field of geometry seldom encountered until undergraduate mathematics study, and often referred to as the “geometry of the rubber sheet” (Johnson & Glenn, 1960). In topology, objects are considered to be the same under deformations like stretching, crumpling and squeezing, as long as the objects are not torn or holes are inserted into them. In topology, distances and directions do not matter; the key property is connectedness. Piaget and Inhelder (1956) held that children think topologically before they begin to think about angles and distances, and Aksu et al. (2021) propose that topology should be encountered much earlier in a mathematical education.