Port based modelling of a multiscale adsorption column

Abstract

A port-based distributed parameter model of an adsorption column under isothermal conditions is presented. This model is made using bond graph terminology and basic axioms of irreversible thermodynamics. The particularity of this model is to split each phenomenon in basic elements with particular energetic behaviour, such as accumulation or dissipation. These basic elements are interconnected using the port variables and some interconnection power conserving structures, named Dirac structures. These energetic elements are presented in terms of the geometry of the different scales of the adsorption column. Moreover it is shown that each scale of the model has the same bond graph structure. The different scales of the model are also connected by a power conserving structure.

Keywords:

• bond graph
• distributed parameter systems
• power conserving structure
• transport phenomena

1. Introduction

The aim of this work is to present a port-based parameter model – also called bond graph model – of an adsorption column. This model takes into account the distributed nature and multi-scale aspect of the process.

Bond graph approach is a tool used for systematic modelling of complex systems 1. Systematic modelling in chemical engineering by using graphs or networks has already been described in the literature 2-4. Such an approach can be very efficient for model management. The model of a system can be manipulated as a set of interconnected and reusable sub-models, provided that softwares are available to support such a manipulation. The Bond Graph language is one of these tools 5. It is based on the energetic behaviour of the submodels and their interconnection using power conjugate variables.

Generalized bond graphs consist of energy-storing elements, resistive elements and power-continuous elements linked together by bonds, each carrying a pair of flow and effort variables, also named port variables, whose product equals the power through the bond.

The main advantage of the bond graph approach over other port based approaches 2-4 is that it is acausal. It assembles relations without imposing any computational structure. So any external port, either an effort variable or a flow variable may serve as an input and the model will respond with the complementary power conjugate variable. Finally submodels and phenomenological laws can be changed without taking into account problems linked to the interconnection since the interconnection is made thanks to the port variables.

The bond graph methodology is mainly issued from the modelling of electrical or mechanical systems 6. Their use in chemical engineering has only recently been developed. Historically, diffusion through a homogeneous membrane situated between two reservoirs was one of the first processes to be studied 7 for biological systems. Then the terminology used for bond graph modelling was network thermodynamics. This modelling assumed to be closed to the thermodynamics equilibrium – using the formalism of irreversible thermodynamics in Onsager's formulation – and so the use of linearized phenomenological laws. Such lumped models were extensively used and improved afterwards 8-12 for the representation of more complex systems – the distributed aspect being represented by a cascade of such lumped elemental models.

Finally in 13,14, the authors propose pseudo bond graph models – pseudo referring to bond graph models where the pairs of variables associated to the bonds are not power conjugate – for transport through planar membranes using a Fick's law for diffusion.

Distributed parameter systems modelling leads to bond graphs where the bond spaces are infinite dimensional vector spaces as recently proposed in 15,16. To this end, special elements are necessary such as the so called Stoke – Dirac structure presented in 15,16. It is an element of the junction structure of the model – the power conserving subgraph of a bond graph – permitting to characterize energy exchanges within the system and through its boundaries. In this study, these ideas are applied and generalized to thermodynamical systems.

In Section 2, the principle of the adsorption process are briefly recalled and the main assumptions, which underlie the modelling of this column are presented. In Section 3, the basic concepts of thermodynamics as well as the general conservation law for mass transfer are discussed in a general case. In Section 4, the port based model of the column is developed. First the general setting presented before is specialized to the assumptions given in Section 2. First the adsorption scale is described: it is shown that the port-based model of this scale can be decomposed into a Stoke – Dirac structure associated with the balance equations and some closure relations applied to some ports of the Stoke – Dirac structure. These closure equations characterize the constitutive laws that represent the energetic phenomena identified in the process at this scale, such as storage or dissipation of energy. Then it is emphasized that this model for the adsorption scale can be reused for the other scales but with different closure equations. Next the macroporous scale is presented and finally the extragranular scale. Finally, the complete model is presented with the coupling of the different scales.

2 The adsorption phenomena and the adsorption column

In this section, the adsorption phenomena is presented as well as the column of adsorption. Finally, the main hypotheses for the modelling of the different scales are given.

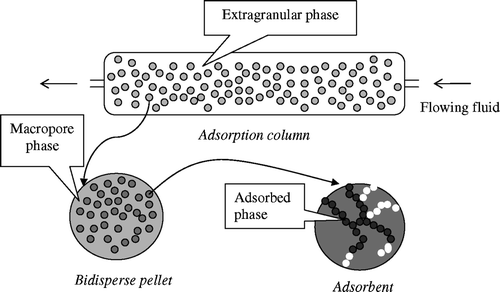
Adsorption is a separation process in which the molecules of a fluid phase are transferred to a solid surface. Therefore, the composition of the system is heterogeneous consisting of two or more fluid phases and the solid adsorbent. Molecules that have been adsorbed onto solid surfaces are referred to as adsorbates, and the surfaces to which they are adsorbed are referred to as the adsorbent.

The adsorption process is based on the ability of a solid to preferentially adsorb constituents present in a fluid phase mixture in order to separate them. This separation is essentially based on the difference of properties that rules the behaviour of each constituent in the fluid mixture – thermodynamic equilibrium between solid and fluid phases on the one hand, and mass transfer kinetics on the other hand 29,30. For instance, consider a binary gas mixture where one constituent, say A, is adsorbed faster than the other one, say B. Then, when a pulse of this mixture is inserted into the inert gas stream at the inlet of the column, component A is adsorbed and the outlet gas is enriched with component B during some transition time as shown on Figure 1.

Figure 1. Outlet concentration in species A and B versus time.

The extent of adsorption depends on physical parameters, such as temperature, pressure and concentration in the bulk phase, and the surface area of the adsorbent, as well as on chemical parameters such as the elemental nature of the adsorbate and the adsorbent.

As depicted in Figure 2, the adsorption column under consideration is composed of a fixed bed of particles having a bidisperse porous structure. These particles are themselves constituted by the agglomeration of crystals of solid – the adsorbent – and by pores between these crystals. These pores form the macropore fluid phase. The fluid phase in the column will be named the extragranular phase.

Figure 2. Description of the different scales of the adsorption column.

In this study, we will deal with the modelling of these three scales. At each scale, we will obtain a partial differential equation representing the material balance.

The following assumptions will be made throughout the study:

	For simplicity, we consider a binary mixture constituted of an inert gas and one component that can be adsorbed. This mixture of gases is supposed to be ideal.
	The adsorption column is supposed to be at constant temperature and pressure. The velocity v of the flowing fluid is supposed to be constant.
	The diffusion onto the surface of the crystal and the diffusion into the macropore volume are represented by using the Maxwell – Stefan formulation 18.
	In the extragranular phase, a dispersion phenomena is taken into account. It is represented with a constant axial dispersion coefficient.
	The Langmuir model for the adsorption equilibrium is used.
	The column is supposed to be cylindrical. The particles and the crystals are supposed to be spherical.
	Cross section of the column is constant and uniform properties of the adsorbent bed throughout the column are considered.
	Negligible concentration gradient are assumed in the radial direction in the column.
	Spherical symmetry are supposed in the macropore phase and for the adsorbent.

3 The basic concepts of thermodynamic and conservation laws

As far as port-based modelling is concerned, its extension to modelling in chemical engineering is based on Irreversible Thermodynamic since all the phenomena that are considered are dissipative. In this section, after a description of the classical approach of modelling in chemical engineering, the basis for the application of the port-based approach are given. Moreover the fundaments for transport phenomena – limited to mass transfer – are recalled through the analysis of the basic material balance equation.

In thermodynamics, in the context of lumped systems, the system variables are classified into extensive and intensive variables, depending on whether their values depend or not on the “amount of matter” of the system under consideration. In analogy with mechanical systems, the increment in the internal energy of a system can be expressed as the product of a thermodynamic generalized force – an intensive variable – and of a generalized displacement, which is always an extensive variable. The internal energy of a system is then expressed in terms of products of pairs of conjugate variables, such as pressure P/ volume V, temperature T/ entropy S and chemical potential μ _i / mole number n _i (for species i).

In fact all thermodynamic potentials are expressed in terms of pairings of conjugate variables 17. In the framework of non-equilibrium thermodynamics, let us consider the simple open one phase system with n _c species. The internal energy U of this system is a thermodynamic potential function of V, S and n _i . The coupling of the energy with these extensive variables and the expression of the intensive one are given by the local form of the Gibbs equation:

where the intensive variables are defined as . d denotes the differential. The internal energy corresponds to the total energy of the physical system under consideration and is subject to a conservation law.

When systems are considered at constant pressure and temperature, it is common to deal with the thermodynamical potential called Gibbs free energy G = U + PV − TS, which is function of mole numbers, T and P. This potential is no more a conserved quantity. For the sake of brevity, in the present work, we will omit the thermal and spatial domain. So we will only consider the pair of power conjugate variables of the material domain.

In the context of distributed parameter systems with 3-dimensional spatial domain, the Gibbs free energy over a sub-volume Ω of the volume of matter is then defined as

where again μ _i and are power conjugated variables.

Now in order to define all the pairing of variables necessary to represent the bond graph model, we deal with the material balance equation of material transfer. The classical PDEs state equations may be split into conservation laws (or balance equations) and closure equations (see for instance 18 or 19). The latter usually allow to compute thermodynamic fluxes from generalized driving forces or the reverse. In classical thermodynamics, it is assumed that generalized forces derive from potentials and that this relation between potential gradients and related fluxes are semi-linear. Examples of such closure equations will be examined in the sequel but we will first focus on material balance equation.

The general material balance equations for species i of a multicomponent system within a given 3-dimensional spatial domain Ω has the familiar form:

where n _c denotes the number of components, c _i the volume density or concentration, N _i the molar flux of the species i going through the boundary of the spatial domain ∂Ω and σ _i is a distributed source term representing the molar rate of production of species i per unit volume. Let us point out that this integral form of the balance equations defines the appearing variables as objects of various natures. The variable N _i is a molar flux, which can be integrated on a 2-dimensional boundary surface (in the general 3-dimensional case). It is called a differential 2-form and write N _i ∈ Λ²(Ω). The variables c _i and σ _i are densities, which are supposed to be integrated on spatial 3-dimensional domains. They are in that sense thermodynamic extensive variables and we call them differential 3-forms. We write thus c _i , σ _i ∈ Λ³(Ω). Now we introduce the exterior derivative d, which is coordinate free and independent from the dimension of Ω. It is in some sense the unique canonical extension of the differentiation of functions, which linearly maps k-differential forms on (k + 1)-differential forms. For instance, in a 3-dimensional domain Ω, the exterior derivative of a function is its gradient, the exterior derivative of a 1-form is a curl and the exterior derivative of a 2-form is its divergence 27,28.

Let us note that the previous material balance equation may be written in the local form:

We will now derive from the material balance equation (4) the energy balance written in some suitable canonical form. Let g denote the Gibbs free energy density. Then the time variation of the total Gibbs free energy in a domain Ω may be written:

where μ denotes the vector of chemical potential and ∧ is the exterior product between differential forms of different orders. This product is such that the product ω _k ∧ ω _l of two differential forms of order k and l, respectively, is a differential form of order k + l. For instance, in a 3-dimensional domain and in cartesian coordinates, one has for the 3-form “element of volume” dx ∧ dy ∧ dz. From Equation (4) – without the source term – and the well known Stokes theorem the total variation of Gibbs free energy within the domain Ω given in Equation (5) is decomposed in the following way:

Note that the wedge product μ ^T ∧ N is a 2-differential form and may naturally be integrated on ∂Ω in the case of a 3-dimensional domain. Equation (6) makes pairs of power conjugate variables appear that we will use throughout this study. Let us denote by the differential symbol d – the exterior derivative – which represents in coordinate free representation the gradient as well as the divergence symbol.

These are the pairs of distributed power conjugate variables

whose wedge product are differential 3-forms defined on Ω and the pair of boundary power conjugate variables

whose wedge product is a differential 2-form defined on ∂Ω and may be integrated on the boundary to get the external supplied power.

These pairing of variables (7) and (8) gives rise to the power preserving structure: the Stoke – Dirac structure whose constitutive relations associated to (6) are given by:

where (d) represents the n _c square n _c by n _c matrix with d on the diagonal terms. Details are given in 20. Its graphical bond graph representation is given in Figure 3.

Figure 3. Bond Graph representation of the Stoke – Dirac structure.

4 The port based model of the adsorption column

An adsorption column is a complex system, which can be mathematically described at each scale by partial differential balance equations as Equation (4).

The partial differential equations that govern the processes of mass transfer in the isothermal adiabatic adsorption column packed with porous adsorbent are the conservation equations of mass of the chemical species in the gas and solid phases coupled with distributed source terms – the term σ _i of Equation (4) – due to mass transfer between the scales.

In this section, the port based model of the adsorption column is presented.

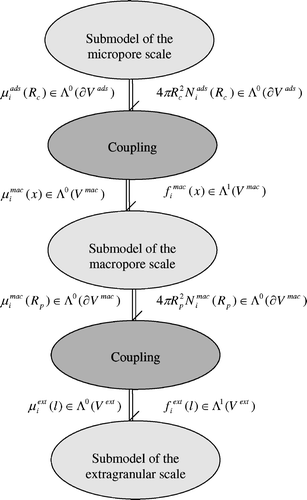
We shall propose the port-based model describing the mass transfer in the three scales identified in the adsorption column. We shall show that the port-based model at each scale of the adsorption process may be decomposed into a Dirac structure associated with the conservation laws and some constitutive equations coupled to some ports of the Dirac structure. Each one of these constitutive equations represents an energetic phenomenon and the Stokes – Dirac structure represents the coupling between these energetic phenomena and also with the external environment (the boundaries of each scale). These port-based models shall also be represented in the bond graph language 6,17 admitting a slight extension as their port variables are now differential forms. Also the interconnections between the microporous – macroporous and the macroporous – extragranular scales are formulated as power conserving interconnection structures.

First Section 4.1 is devoted to a coordinate free model. Section 4.2 is concerned with the model in the adsorbed phase with its characteristic phenomenological laws for mass transfer and thermodynamics. In Sections 4.3 and 4.4 the modelling of the macroporous scale and the extragranular one are discussed, respectively. Molar fluxes coming from other scales are considered as source terms. Their computation will be postponed at Section 4.5 where the complete model is given.

4.1 The independent geometry general submodel

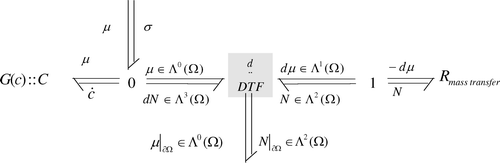
For a general material transfer model whose material balance is given by Equation (4) in a physical 3D domain Ω, we have to specify what is the driving force for the mass transfer and what is the thermodynamic properties of the medium in which the mass transfer occurs. Since we use the thermodynamical approach, it is natural to use the gradient of chemical potential as the driving force for the computation of the molar flux due to mass transfer 21. This will constitute the first phenomenological law: N = R _{mass transfer}(dμ). Mass transfer is clearly a dissipative phenomena: it creates an irreversible entropy production, so it is represented in the bond graph by a R element. The thermodynamic property of the medium is such that we will obtain μ as a function of c. This computation is made in the C element. Finally the distributed source term may represent a molar flow coming from a lower scale. The boundary terms of the DTF element correspond to molar flux flowing out of this scale to an upper one.

Let us associate a bond graph model to (4) independently of the geometry of the scale. The bond graph is given by Figure 4. This general structure will be the same for any scale of the adsorption column. Moreover this representation is independent of the coordinate system by the use of the differential notation. In the next section, we will specify the geometry and phenomenological laws for all scales of the adsorption column under consideration.

Figure 4. Bond Graph representation of the mass transfer submodel.

4.2 The adsorbed scale

The multiscale assumption means that any point of the microporous scale is indexed by a point of the macroporous one. For simplicity these indexes were and will be omitted. The dynamic model in the microporous medium is then simply given by the material balance equation:

Following the assumptions, crystals are spheres and spherical symmetry is supposed at the scale. Let us call V ^ads the geometrical domain under consideration and z the radial coordinate. Symmetry hypotheses permits to reduce the order of forms under consideration. With this geometry, in the coordinate system, Equation (6), representing the energy conservation in the DTF element, can be expressed as:

with the imposed boundary conditions. The variables and variables . The material balance (10) may be written

4.2.1 The adsorption phenomena

The Maxwell – Stefan formulation 21, which expresses the diffusion of p species by setting that the driving force is the chemical potential gradient, is used to model the diffusion in the crystal scale. This relation comes from the force balance between the driving force exerted by the species on each other and the friction force exerted by the wall of crystal and species. For this study, we suppose that only the friction forces are exerted. Moreover, the diffusion onto the surface of the crystal play a leading role in the adsorption. It is assumed that each molecule, which lies in the microporous scale is adsorbed. This means that in the adsorbed phase, there is no possibility of two different molecules undergoing counter-exchange at an adsorption site 21. In the coordinate system, the representation of the Maxwell – Stefan's equation is given by:

The n _c  + 1^th species represent the pseudo species of the vacant sites onto the surface of the crystal. At this species is associated the pseudo molar flux  – this flux is such that . If is the fraction of occupied site by species i, it is clear that . Let us call q _j the concentration of species j onto the crystal and is the saturation concentration onto the crystal then . Moreover, is equal to the total concentration of sites in the crystal. D _i is the Maxwell – Stefan diffusivity between species i and the wall, R is the perfect gas constant and T the temperature.

To obtain the expression of molar flux of the n _c species with respect to gradient, we have to inverse Equation (13) with the help of the additional constraint given above. In the case of only one species – say species I – is adsorbed, the result is straightforward:

This equation will constitute the constitutive relation for the R element of the bond graph.

To complete the model in this scale we shall add the constitutive equations defining the thermodynamic properties of the mixture. These closure properties define the energy storing elements C. This leads to express intensive variables (the chemical potential) as functions of the extensive variables.

4.2.2 The thermodynamic property of the crystal scale

The objective of this section is to give an expression of the chemical potential of the adsorbed species. There is no general formula to compute these chemical potentials. What is well known is the computation of chemical potentials for ideal gas mixture. The following reasoning – classical for chemical engineers – is used to obtain chemical potentials for adsorbed species:

Whenever a gas is in contact with a solid there will be an equilibrium established between the molecules in the gas phase and the corresponding adsorbed species which are bound onto the surface of the solid. In this study, the Langmuir isotherm is used to model this equilibrium:

where the b _j 's are the Langmuir equilibrium constant and c _j is the concentration of species j in gas phase. So in this gas phase it is known that .

Then we assume that locally the thermodynamical equilibrium is reached between the two phases, so for each species, the chemical potentials in the two phases are equal. So the chemical potential in the adsorbed phase is equal to:

Replacing the c's with their expression with respect to q's and c _tot with in the formula, we obtain the following closure equation defining the thermodynamic properties of the mixture in the microporous medium.

This closure equation expresses the chemical potential , in the adsorbed phase, of the components i at some temperature T and pressure P. denotes the chemical potential of pure component i at standard state and .

4.2.3 The bond graph

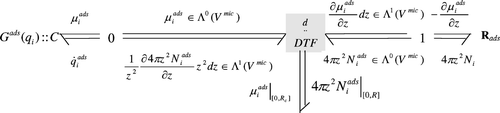
The bond graph model is represented in Figure 5.

Figure 5. Bond Graph representation of the adsorption process at the microporous scale.

The R _ads element is the diffusion model that represents the dissipative phenomenon. Its constitutive equation is given in Equation (14). The C element represents the storage phenomenon, its constitutive equation is given by Equation (17).

4.3 The macroporous scale

The multiscale assumption means that any point of the macroporous scale is indexed by a point of the extragranular one. For simplicity these indexes were and will be omitted. The spatial domain at this scale will be called V ^mac and spherical symmetry assumptions are made. Let us call x the radial coordinate at this scale and R _p the radius of the spherical domain.

4.3.1 Submodel of the macropororous medium

The mass transfer model in the pellet, the macroporous scale, is similar to the model of the adsorption process in the crystal scale. The dynamic model of diffusion process in the pellet is then given by the balance equation:

where is the molar flux given by the constitutive equation for diffusion (19) and is the flow of species i per unit of domain. This term is a distributed source accounting for the molar flow coming out of a microporous medium at a point x in the spatial domain V ^mac. The expression of the molar flow will be presented in Section 4.5.

Before presenting the bond graph of this scale, let us provide the constitutive laws for this scales.

4.3.2 The diffusion phenomena

We also use Maxwell – Stefan's law for modelling the diffusion in the macroporous scale. In the pellet, we consider only the friction between the gas molecules, the Maxwell – Stefan constitutive relations of diffusion are written as:

where is the molar fraction of species i in the macroporous scale, is the total concentration, is the molar flux of species i. is the molar concentration of species i (mol/m ⁻³). D _i,j is the Maxwell – Stefan diffusivity between i and j (m ²/s ⁻¹).

This is the classical diffusion equation 18,21. It is important to notice that, contrary to the case in the microporous medium, this equation does not express explicitly the molar flux as a function of the chemical potential gradient. Moreover this molar flux is not independent: . We obtain an implicit relation to describe the dissipative element. The numerical inversion is possible for n _c  – 1 species subject to constraints: .

4.3.3 The thermodynamical properties

In the macroporous media, the mixture is a gaseous phase assumed to be an ideal gas. The constitutive equations defining the thermodynamical properties is the classical expression of the chemical potential for an ideal gas. So in the macroporous medium, we have:

4.3.4 The bond Graph

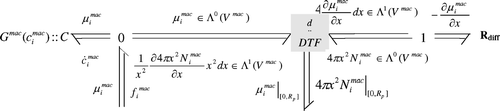
The bond graph model is given in Figure 6 where the dissipative element R _diff is the diffusion model. Its constitutive equation is given in Equation (19). The C element represents the storage phenomenon, its constitutive equation is given in the first part of Equation (20). The DTF is the Stokes – Dirac structure associated with this scale. This DTF element representing the interconnection structure between storage and dissipative part of this scale and has the same structure as the one presented in Section 4.2.

Figure 6. Bond Graph model of the diffusion at the macroporous scale.

4.4 The extragranular scale

In the column scale, the model variables are defined on the spatial domain V ^ext. With symmetry assumption, only the axial coordinate l is used, l ∈ ℝ = [0, L]. The dynamic model in the column scale is then given by the material balance equation including a distributed source term 18.

where is the flow of species i. This is a distributed source accounting for the molar flow coming out of a macroporous medium at a point l of the spatial domain V ^mac. More details about will be provided in Section 4.5.

Equation (6), representing the energy conservation in the DTF element, can be expressed as:

where L is the length of the column, R _cl the radius of the column.

4.4.1 The mass transfer phenomena

Dispersion is an important solute transport mechanism which arises from an interplay between non-uniform convection due to local heterogeneities and molecular diffusion. The model used hereafter is represented by means of an axial effective dispersion parameter D _ax and its corresponding flux expression is analogous to the Fick's relation 18.

The mass transfer phenomenon is slightly different from the first two scales. The mass transfer in this scale is governed by convection and dispersion. The convective flux is given by:

where v is the mean fluid velocity, is the molar density of species i (mol/m ⁻³).

The dispersion is due to flow inhomogeneity. It is represented by means of an axial dispersion parameter D _ax and its corresponding flux expression is analogous to the Fick's relation 18. The constitutive relation that gives the dispersive flux as a function of the gradient of the chemical potential, at constant temperature and pressure, is given by:

where is the reference chemical potential and the total linear molar density.

4.4.2 The thermodynamical properties of the extragranular scale

In the extragranular media the mixture is again a gaseous phase assumed to be an ideal gas. The constitutive equations defining the thermodynamical properties are:

4.4.3 The bond graph

The R _disp element represents the dispersion phenomenon with the constitutive relation (24). In the bond graph, the material balance is represented by the 0 junction connected to the energy storing element C.

The interconnection between the part representing the energy storage, and the other part, representing the convection and the dispersion, and also the boundary conditions is modelled by the element DTF that represent the Dirac structure. The bond graph model is then given in Figure 7. We note that as we consider a fluid moving with a constant velocity, so the S _f represents an energy source coming from another energetic domain.

Figure 7. Bond Graph model in the Extragranular scale.

4.5 The model of the multiscale process

In Sections 4.4 and 4.3, we have noticed the presence of distributed source terms in material balance equations (21) and (18). These source terms result from the dispersed heterogeneous nature of the process and correspond to matter flowing between the scales. Based on physical considerations, we will define an interconnection structure that will represent either the interconnection between the macroporous and the extragranular scales or the one between the adsorbant and the macroporous scales. First, we present the coupling between the macroporous and the extragranular scales.

4.5.1 The coupling between the macroporous scale and the extragranular scale

The hypothesis of separation of the two scales amounts to the assumption that in a slice of the extragranular fluid there is a sufficient number of pellets of much smaller size so that a pellet is abstracted to a point. The concentration of pellets in the extragranular fluid is denoted by C _pellet(l) where l is in the spatial domain of the extragranular scale. At a point l ₀ ∈ L, is attached a spatial domain isomorphic to some domain V ^mac and indexed by l ₀. Thus the domain of the set of pellets in the fluid is V ^ext × V ^mac.

Furthermore we use two assumptions, to couple the macroporous and the extragranular scales by relating firstly the intensive variables consisting of the chemical potential of the macroporous scale restricted to the boundary x = R _p of its domain, and the chemical potential at the extragranular scale at the point l ∈ L. Secondly a coupling relation is defined on the conjugated extensive variables, the volumetric density flux variable at the extragranular scale and the flux variable of macroporous scale restricted to the boundary of its domain.

These two conjugated relations actually involve on the one hand port boundary variables of the macropore scale with distributed port variables of the extragranular scale. It can be shown that these relations define a power continuous interconnection structure between the two scales which is a novel Dirac structure and called Coupling in this study. For further details on this Dirac structure, refer to 20.

The relation defining the coupling is:

where is the molar flow issued from the boundary of one pellet, c _pellet represents the density of pellets present at coordinate l in the extra granular phase and the differential volume of the considered slice of column .

4.5.2 The coupling between the microporous scale and the macroporous scale

In a same way, the relation defining this coupling is:

where is the molar flow issued from the boundary of one crystal, c _crystal represents the density of crystals present at coordinate x in the pellet phase and the differential volume of the considered slice of pellet .

4.5.3 The complete model

The complete Bond graph model is represented in Figure 8. The models of the three scales and the interconnection structure between the scales are represented in this model.

Figure 8. Complete bond graph of the adsorption column.

5 Conclusion

In this study, we discussed the port-based modelling of a distributed parameter system. To illustrate this approach, a model of an adsorption column has been derived directly from its thermodynamical description. The modelling methodology presented in this paper exhibits some interesting features:

	The modelling may be coordinate free 20. The model can be stated independently of the particular geometries of the adsorption column, pellets and crystal. These geometric specifications may be addressed at the end of the physical modelling process as the derived equations and interconnection structure are “geometry-independent”. In this study, for simplicity, we choose to present the model with coordinates.
	The model is a network model where each element represents a specific phenomenon which can be identified from a thermodynamics point of view. The constitutive equations of each element can be changed without modifying the structure of the model.
	The instantaneous power conservation and the description of the power transfers within the system and through its boundaries are explicitly represented with the help of the power-conserving geometric structure (called Dirac structure). This is the case for the adsorption and diffusion phenomena, the dispersion as well as for the scale interconnections. The two interconnection structures dedicated to distributed parameter systems are very general and can be used for any model in order to describe the instantaneous power exchange between boundaries and scale coupling models of mass transfer phenomena for heterogeneous systems.

These properties of the model have several important consequences:

	The model is acausal, hence postpones the choice of boundary conditions and is thus clearly reusable. One can choose to set either an effort or a flux at the boundary of the system; these boundaries being the port variables of the model as a submodel.
	This choice of state variables naturally leads to low index differential algebraic systems for heterogeneous processes 22.
	The central geometric Dirac structure is a direct generalization of Poisson structure in Hamiltonian systems. It is stable under interconnection. This suggests the use of control techniques based on energy shaping i.e. feedback laws issued from hamiltonian control systems 23.
	When the thermal domain is taken into account, the presented approach can be generalized using the entropy as the energy conjugate variable associated to the temperature. In this case, the use of passivity techniques 24 seems a natural approach for control purposes.

Finally these considerations strongly encourage the development of a discretization method which preserves both the nature of the interconnection structures and the physical properties of the connected elements. Such a method has been presented in 25,26.

Acknowledgements

This work has been done in the context of the European sponsored project GeoPlex with reference code IST-2001-34166. Further information is available at http://www.geoplex.cc.