Abundant new computational wave solutions of the GM-DP-CH equation via two modified recent computational schemes

Abstract

This work employs two new modified computational schemes to find the exact travelling and solitary wave solutions of the general modified Degasperis-Procesi Camassa-Holm (DP CH) equation that is used to illustrate the dynamical behaviour of shallow water. These schemes are the modified exponential expansion and the modified Khater methods that are used to formulate the computational solutions in various formulas such as trigonometric, hyperbolic, exponential functions. The obtained solutions are explained and figured out their substantial contributions in three different types of sketches (three, two-dimensional, and contour plots).

KEYWORDS:

• The general modified Degasperis-Procesi-Camassa-Holm (DP CH) equation
• computational recent modified schemes
• solitary solutions

1. Introduction

The nonlinear partial differential equations (NPDEs) have so many essential applications in various fields of engineering and science, such as heat transfer, fluid mechanics, chemistry, thermodynamic, physics, micro electro-mechanic system, etc. These equations have been being employed to describe many complex phenomena [1–10]. So, finding the exact and numerical solutions of these model have been being attracted the attention of many researchers in various branches of science. Consequently, a variety of practical, accurate solution methods have been being developed to be used for solving LPDEs, such as the auxiliary equation method [11,12], the Jacobi elliptic function expansion method [13,14], the $\left(G^{\mathrm{\prime }}/G\right)$ – expansion method [15,16], the modified Kudryashov method [17,18], the first integral method [19,20], the new extended direct algebraic method [21,22], the sine-Gordon expansion method [23,24], the Khater method [25,26], the sub-equation method [27,28], the extended sinh-Gordon equation expansion method [29,30] and so on.

In this sense, we are going to employ two modified recent computational schemes (the modified exponential expansion and modified Khater methods) to find the exact travelling and solitary wave solutions of the dimensionless form of the general modified Degasperis-Procesi Camassa-Holm (GM-DP-CH) equation that is given by [31,32] (1) $\begin{array}{rl}& {\mathcal{W}}_t-{\mathcal{W}}_{xxt}+\left(\mathrm{\wp }+1\right){\mathcal{W}}^2{\mathcal{W}}_x\\ & -\left(\mathrm{\wp }{\mathcal{W}}_x{\mathcal{W}}_{xx}+\mathcal{W}{\mathcal{W}}_{xxx}\right)=0,\end{array}$(1) where $\mathrm{\wp }$ is any real number, including $\mathrm{\wp }=2,3,$ also $\mathcal{W}=\mathcal{W}\left(x,t\right)$ is the function of displacement and time and used to explain the dynamical behaviour of the shallow water. Equation (1) is a member of a great mathematical integrable equation family that studies the shallow water physical properties. This family is given by (2) $\begin{array}{r}{\mathcal{W}}_t-{\mathcal{W}}_{xxt}+\left(\mathrm{\wp }+1\right)\mathcal{W}{\mathcal{W}}_x=\mathrm{\wp }{\mathcal{W}}_x{\mathcal{W}}_{xx}+\mathcal{W}{\mathcal{W}}_{xxx},\end{array}$(2) Equation (2) gives the Camassa-Holm (CH) equation [33] when $\mathrm{\wp }=2$, and provides the Degasperis-Procesi with (DP) equation [34] when $\mathrm{\wp }=3.$ Both of CH and DP equations are bi-Hamiltonian and have an associated isospectral problem. Additionally, they admit peaked solitary wave solutions. Equation (2) is just an integrable family of the equation in case $\mathrm{\wp }=2,3,$ but it is not for any other values of $\mathrm{\wp }.$ Using the next wave transformation $\mathcal{W}=\mathcal{W}\left(x,t\right)=\mathcal{U}\left(\mathfrak{E}\right),\mathfrak{E}=x+ct,$ where $c$ is an arbitrary constant on Equation (1) leads to (3) $c{\mathcal{U}}^{\mathrm{\prime }}-c{\mathcal{U}}^{\mathrm{\prime }\mathrm{\prime }\mathrm{\prime }}+\left(\mathrm{\wp }+1\right){\mathcal{U}}^2{\mathcal{U}}^{\mathrm{\prime }}-\mathrm{\wp }{\mathcal{U}}^{\mathrm{\prime }}{\mathcal{U}}^{\mathrm{\prime }\mathrm{\prime }}-\mathcal{U}{\mathcal{U}}^{\mathrm{\prime }\mathrm{\prime }\mathrm{\prime }}=0.$(3)

Balancing the highest order derivative term $,\mathcal{U}{\mathcal{U}}^{\mathrm{\prime }\mathrm{\prime }\mathrm{\prime }}$and nonlinear term ${\mathcal{U}}^2{\mathcal{U}}^{\mathrm{\prime }}$ in Equation (3) leads to $n+n+3=2n+n+1\Rightarrow n=2.$

The rest sections in this paper are organized as follows. Section 2 studies the performance of the modified exponential expansion method [35,36], and the modified Khater method [37–40] on the GM-DP-CH equation. Section 3 gives the conclusion.

2. Application

In this section, the modified exponential expansion method and the modified Khater method are used to find novel solitary wave solutions of the GM-DP-CH equation. These solutions have a significant role in discovering more physical properties of the dynamics of the shallow water waves.

The modified exponential expansion method.

Applying this scheme to Equation (3) leads to the following general solution (4) $\begin{array}{rl}\mathcal{U}\left(\mathfrak{E}\right)& =\sum _{i=-n}^n{a}_i{\text{e}}^{-i\varphi \left(\mathfrak{E}\right)}\\ & ={\text{e}}^{2\varphi \left(\mathfrak{E}\right)}{a}_{-2}+{\text{e}}^{\varphi \left(\mathfrak{E}\right)}{a}_{-1}+a_0\\ & +{\text{e}}^{-\varphi \left(\mathfrak{E}\right)}{a}_1+{\text{e}}^{-2\varphi \left(\mathfrak{E}\right)}{a}_2,\end{array}$(4) where $a_i,\left(i=-2,-1,0,1,2\right)$ are arbitrary constants. Also, $\varphi \left(\mathfrak{E}\right)$ is the solution function of the next ODE; (5) ${\varphi }^{\mathrm{\prime }}\left(\mathfrak{E}\right)=\varrho +\delta {\text{e}}^{\left(\mathfrak{E}\right)}+{{\text{e}}^{\left(\mathfrak{E}\right)}}^{-1},$(5) where $\varrho ,\delta$ are arbitrary constants. Substituting Equation (4) along (5) into Equation (3) and collecting all terms with the same power of ${\text{e}}^{j\varphi \left(\mathfrak{E}\right)},\left(j=-7,-6,\dots ,6,7\right)$ lead to a system of algebraic equations. Solving this system yields

Family I: $\begin{array}{rl}{a}_{-2}& =a_{-1}=0,a_0\\ & =-\frac{\begin{array}{c}6{\varrho }^2-\varrho \left(8\delta +{\varrho }^2\right)a_1+\\ \sqrt{36{\varrho }^4+{\left(-4\delta \varrho +{\varrho }^3\right)}^2{a}_1\left(-12\varrho +a_1\right)}\end{array}}{12{\varrho }^2},\\ & a_2=\frac{a_1}{\varrho },\\ c& =\frac{6{\varrho }^2+\sqrt{36{\varrho }^4+{\left(-4\delta \varrho +{\varrho }^3\right)}^2{a}_1\left(-12\varrho +a_1\right)}}{2\varrho \left(6\varrho -a_1\right)},\\ \mathrm{\wp }& =\frac{-12\varrho +a_1}{6\varrho -a_1}.\end{array}$Family II $\begin{array}{rl}{a}_{-2}& =\frac{\delta a_{-1}}{\varrho },a_0\\ & =-\frac{\begin{array}{c}6\delta {\varrho }^2-\varrho \left(8\delta +{\varrho }^2\right)a_{-1}+\\ \sqrt{36{\delta }^2{\varrho }^4+{\left(-4\delta \varrho +{\varrho }^3\right)}^2{a}_{-1}\left(-12\delta \varrho +a_{-1}\right)}\end{array}}{12\delta {\varrho }^2},\\ & a_1=a_2=0,\\ c& =\frac{\begin{array}{c}6\delta {\varrho }^2+\\ \sqrt{36{\delta }^2{\varrho }^4+{\left(-4\delta \varrho +{\varrho }^3\right)}^2{a}_{-1}\left(-12\delta \varrho +a_{-1}\right)}\end{array}}{2\varrho \left(6\delta \varrho -a_{-1}\right)},\\ \mathrm{\wp }& =\frac{-12\delta \varrho +a_{-1}}{6\delta \varrho -a_{-1}}.\end{array}$Thus, the solitary wave solutions of the GM-DP-CH are given based on family I by

For ${\varrho }^2-4\delta >0,\delta \ne \text{ 0;}$ (6) $\begin{array}{rl}& {\mathcal{W}}_{1,1}\left(x,t\right)\\ & =\frac{1}{12}[-6-\frac{\sqrt{\begin{array}{c}36{\varrho }^4+{\left(-4\delta \varrho +{\varrho }^3\right)}^2\\ a_1\left(-12\varrho +a_1\right)\end{array}}}{{\varrho }^2}\\ & +a_1(\varrho +\frac{48{\delta }^2}{\begin{array}{c}\varrho (\varrho +\sqrt{-4\delta +{\varrho }^2}\text{Tanh}\\ {\left[\frac{1}{2}\sqrt{-4\delta +{\varrho }^2}\left(ct+x+\text{℧}\right)\right])}^2\end{array}}\\ & +8\delta \left(\frac{1}{\varrho }-\frac{3}{\begin{array}{c}\varrho +\sqrt{-4\delta +{\varrho }^2}\text{Tanh}\\ \left[\frac{1}{2}\sqrt{-4\delta +{\varrho }^2}\left(ct+x+\text{℧}\right)\right]\end{array}}\right))],\end{array}$(6) (7) $\begin{array}{rl}& {\mathcal{W}}_{1,2}\left(x,t\right)\\ & =\frac{1}{12}[-6+(\varrho +\frac{48{\delta }^2}{\begin{array}{c}\varrho (\varrho +\sqrt{-4\delta +{\varrho }^2}\text{Coth}\\ {\left[\frac{1}{2}\sqrt{-4\delta +{\varrho }^2}\left(ct+x+\text{℧}\right)\right])}^2\end{array}}\\ & +8\delta (\frac{1}{\varrho }-\frac{3}{\begin{array}{c}\varrho +\sqrt{-4\delta +{\varrho }^2}\text{Coth}\\ \left[\frac{1}{2}\sqrt{-4\delta +{\varrho }^2}\left(ct+x+\text{℧}\right)\right]\end{array}}))a_1\\ & -\frac{\sqrt{36{\varrho }^4+{\left(-4\delta \varrho +{\varrho }^3\right)}^2{a}_1\left(-12\varrho +a_1\right)}}{{\varrho }^2}\phantom{\frac{48{\delta }^2}{\begin{array}{c}\varrho (\varrho +\sqrt{-4\delta +{\varrho }^2}\text{Coth}\\ {\left[\frac{1}{2}\sqrt{-4\delta +{\varrho }^2}\left(ct+x+\text{℧}\right)\right])}^2\end{array}}}].\end{array}$(7)

For ${\varrho }^2-4\delta >0,\delta =\text{0;}$ (8) $\begin{array}{rl}& {\mathcal{W}}_{1,3}\left(x,t\right)\\ & =\frac{1}{12}(-6+\left(\varrho +3\varrho \text{Csch}{\left[\frac{1}{2}\varrho \left(ct+x+\text{℧}\right)\right]}^2\right)a_1\\ & -\frac{\sqrt{36{\varrho }^4+{\varrho }^6{a}_1\left(-12\varrho +a_1\right)}}{{\varrho }^2}).\end{array}$(8) For ${\varrho }^2-4\delta =0,\delta \ne \text{ 0, }\varrho \ne \text{ 0;}$ (9) $\begin{array}{rl}& {\mathcal{W}}_{1,4}\left(x,t\right)\\ & =-\frac{\begin{array}{c}6{\varrho }^2+2\varrho \left(-4\delta +{\varrho }^2\left(1-\frac{6}{{\left(2+\varrho \left(ct+x+\text{℧}\right)\right)}^2}\right)\right)\\ a_1+\sqrt{36{\varrho }^4+{\left(-4\delta \varrho +{\varrho }^3\right)}^2{a}_1\left(-12\varrho +a_1\right)}\end{array}}{12{\varrho }^2}.\end{array}$(9) For ${\varrho }^2-4\delta <0,\delta \ne \text{ 0}$ (10) $\begin{array}{rl}& {\mathcal{W}}_{1,5}\left(x,t\right)\\ & =-\frac{1}{12{\varrho }^2}[6{\varrho }^2-\varrho (12{\text{e}}^{\frac{4\delta }{\varrho -\sqrt{4\delta -{\varrho }^2}\text{Tan}\left[\frac{1}{2}\sqrt{4\delta -{\varrho }^2}\left(ct+x+\text{℧}\right)\right]}}\\ & +8\delta +12{\text{e}}^{\frac{2\delta }{\varrho -\sqrt{4\delta -{\varrho }^2}\text{Tan}\left[\frac{1}{2}\sqrt{4\delta -{\varrho }^2}\left(ct+x+\text{℧}\right)\right]}}\varrho +{\varrho }^2)a_1\\ & +\sqrt{36{\varrho }^4+{\left(-4\delta \varrho +{\varrho }^3\right)}^2{a}_1\left(-12\varrho +a_1\right)}\phantom{12{\text{e}}^{\frac{4\delta }{\varrho -\sqrt{4\delta -{\varrho }^2}\text{Cot}\left[\frac{1}{2}\sqrt{4\delta -{\varrho }^2}\left(ct+x+\text{℧}\right)\right]}}}],\end{array}$(10) (11) $\begin{array}{rl}& {\mathcal{W}}_{1,6}\left(x,t\right)\\ & =-\frac{1}{12{\varrho }^2}[6{\varrho }^2-\varrho (12{\text{e}}^{\frac{4\delta }{\varrho -\sqrt{4\delta -{\varrho }^2}\text{Cot}\left[\frac{1}{2}\sqrt{4\delta -{\varrho }^2}\left(ct+x+\text{℧}\right)\right]}}\\ & +8\delta +12{\text{e}}^{\frac{2\delta }{\varrho -\sqrt{4\delta -{\varrho }^2}\text{Cot}\left[\frac{1}{2}\sqrt{4\delta -{\varrho }^2}\left(ct+x+\text{℧}\right)\right]}}\varrho +{\varrho }^2)a_1\\ & +\sqrt{36{\varrho }^4+{\left(-4\delta \varrho +{\varrho }^3\right)}^2{a}_1\left(-12\varrho +a_1\right)}\phantom{12{\text{e}}^{\frac{4\delta }{\varrho -\sqrt{4\delta -{\varrho }^2}\text{Cot}\left[\frac{1}{2}\sqrt{4\delta -{\varrho }^2}\left(ct+x+\text{℧}\right)\right]}}}].\end{array}$(11)

While, the solitary wave solutions of the GM-DP-CH are given based on family II by

For ${\varrho }^2-4\delta >0,\delta \ne \text{ 0;}$ (12) $\begin{array}{rl}& {\mathcal{W}}_{2,1}\left(x,t\right)\\ & =\frac{-1}{12\delta {\varrho }^2}[\phantom{\left(-1+3\text{Sech}{\left[\frac{1}{2}\sqrt{-4\delta +{\varrho }^2}\left(ct+x+\text{℧}\right)\right]}^2\right)}6\delta {\varrho }^2+\varrho (-4\delta +{\varrho }^2)\\ & \times \left(-1+3\text{Sech}{\left[\frac{1}{2}\sqrt{-4\delta +{\varrho }^2}\left(ct+x+\text{℧}\right)\right]}^2\right)\\ & \times a_{-1}\\ & +\sqrt{36{\delta }^2{\varrho }^4+{\left(-4\delta \varrho +{\varrho }^3\right)}^2{a}_{-1}\left(-12\delta \varrho +a_{-1}\right)}\phantom{\left(-1+3\text{Sech}{\left[\frac{1}{2}\sqrt{-4\delta +{\varrho }^2}\left(ct+x+\text{℧}\right)\right]}^2\right)}],\end{array}$(12) (13) $\begin{array}{rl}& {\mathcal{W}}_{2,2}\left(x,t\right)\\ & =\frac{-1}{12\delta {\varrho }^2}[\phantom{\left(-1+3\text{Sech}{\left[\frac{1}{2}\sqrt{-4\delta +{\varrho }^2}\left(ct+x+\text{℧}\right)\right]}^2\right)}6\delta {\varrho }^2-\varrho (-4\delta +{\varrho }^2)\\ & \times \left(1+3\text{Csch}{\left[\frac{1}{2}\sqrt{-4\delta +{\varrho }^2}\left(ct+x+\text{℧}\right)\right]}^2\right)a_{-1}\\ & +\sqrt{36{\delta }^2{\varrho }^4+{\left(-4\delta \varrho +{\varrho }^3\right)}^2{a}_{-1}\left(-12\delta \varrho +a_{-1}\right)}\phantom{\left(-1+3\text{Sech}{\left[\frac{1}{2}\sqrt{-4\delta +{\varrho }^2}\left(ct+x+\text{℧}\right)\right]}^2\right)}].\end{array}$(13)

For ${\varrho }^2-4\delta =0,\delta \ne \text{ 0, }\varrho \ne \text{ 0;}$ (14) $\begin{array}{rl}& {\mathcal{W}}_{2,3}\left(x,t\right)\\ & =\frac{1}{12}[-6+\frac{\begin{array}{c}(-16{\varrho }^4+\frac{{\varrho }^6}{\delta }-\frac{48{\varrho }^3}{ct+x+\text{℧}}+\\ \frac{48\delta {\left(2+\varrho \left(ct+x+\text{℧}\right)\right)}^2}{{\left(ct+x+\text{℧}\right)}^2})a_{-1}\end{array}}{{\varrho }^5}\\ & -\frac{\sqrt{36{\delta }^2{\varrho }^4-{\left(-4\delta \varrho +{\varrho }^3\right)}^2\left(12\delta \varrho -a_{-1}\right)a_{-1}}}{\delta {\varrho }^2}\phantom{\frac{\begin{array}{c}(-16{\varrho }^4+\frac{{\varrho }^6}{\delta }-\frac{48{\varrho }^3}{ct+x+\text{℧}}+\\ \frac{48\delta {\left(2+\varrho \left(ct+x+\text{℧}\right)\right)}^2}{{\left(ct+x+\text{℧}\right)}^2})a_{-1}\end{array}}{{\varrho }^5}}].\end{array}$(14)

For ${\varrho }^2-4\delta <0,\delta \ne \text{ 0;}$ Figures 1–3 (15) $\begin{array}{rl}& {\mathcal{W}}_{2,4}\left(x,t\right)\\ & =\frac{1}{12}[\phantom{12{\text{e}}^{-\frac{4\delta }{\varrho -\sqrt{4\delta -{\varrho }^2}\text{Cot}\left[\frac{1}{2}\sqrt{4\delta -{\varrho }^2}\left(ct+x+\text{℧}\right)\right]}}}-6\\ & +\frac{1}{\delta {\varrho }^2}(\varrho (12{\text{e}}^{-\frac{4\delta }{\varrho -\sqrt{4\delta -{\varrho }^2}\text{Tan}\left[\frac{1}{2}\sqrt{4\delta -{\varrho }^2}\left(ct+x+\text{℧}\right)\right]}}{\delta }^2+{\varrho }^2\\ & +\delta \left(8+12{\text{e}}^{-\frac{2\delta }{\varrho -\sqrt{4\delta -{\varrho }^2}\text{Tan}\left[\frac{1}{2}\sqrt{4\delta -{\varrho }^2}\left(ct+x+\text{℧}\right)\right]}}\varrho \right))a_{-1}\\ & -\sqrt{36{\delta }^2{\varrho }^4-{\left(-4\delta \varrho +{\varrho }^3\right)}^2\left(12\delta \varrho -a_{-1}\right)a_{-1}})\phantom{12{\text{e}}^{-\frac{4\delta }{\varrho -\sqrt{4\delta -{\varrho }^2}\text{Cot}\left[\frac{1}{2}\sqrt{4\delta -{\varrho }^2}\left(ct+x+\text{℧}\right)\right]}}}],\end{array}$(15) (16) $\begin{array}{rl}& {\mathcal{W}}_{2,5}\left(x,t\right)\\ & =\frac{1}{12}[\phantom{\sqrt{36{\delta }^2{\varrho }^4-{\left(-4\delta \varrho +{\varrho }^3\right)}^2\left(12\delta \varrho -a_{-1}\right)a_{-1}}}-6\\ & +\frac{1}{\delta {\varrho }^2}(\varrho (12{\text{e}}^{-\frac{4\delta }{\varrho -\sqrt{4\delta -{\varrho }^2}\text{Cot}\left[\frac{1}{2}\sqrt{4\delta -{\varrho }^2}\left(ct+x+\text{℧}\right)\right]}}{\delta }^2+{\varrho }^2\\ & +\delta \left(8+12{\text{e}}^{-\frac{2\delta }{\varrho -\sqrt{4\delta -{\varrho }^2}\text{Cot}\left[\frac{1}{2}\sqrt{4\delta -{\varrho }^2}\left(ct+x+\text{℧}\right)\right]}}\varrho \right))a_{-1}\\ & -\sqrt{36{\delta }^2{\varrho }^4-{\left(-4\delta \varrho +{\varrho }^3\right)}^2\left(12\delta \varrho -a_{-1}\right)a_{-1}})].\end{array}$(16)

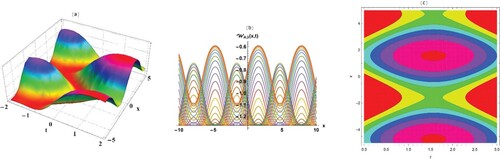
Figure 1. Dark-wave solutions of Equation (6) when $\varrho =5,\mathit{\delta }=1,\varrho =6,{\mathit{a}}_1=100,\text{℧}=5$ in three, two-dimensional, and contour plot in two-dimensional plots.

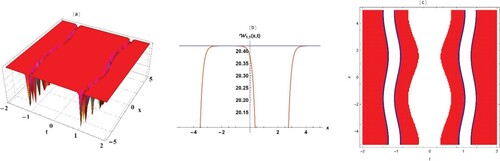
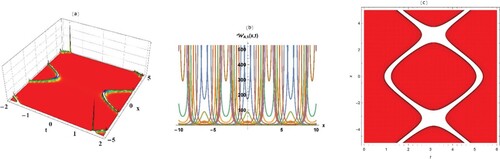
Figure 2. Bright wave solutions of Equation (7) when $\varrho =5,\mathit{\delta }=0,\varrho =6,{\mathit{a}}_1=100,\text{℧}=5$ in three, two-dimensional, and contour plot in two-dimensional plots.

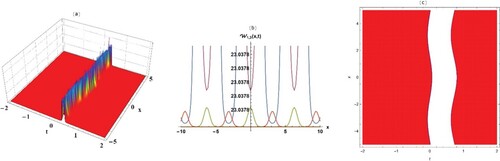
Figure 3. Dark-wave solutions of Equation (12) when $\varrho =5,\mathit{\delta }=1,\varrho =6,{\mathit{a}}_{-1}=100,\text{℧}=5$ in three, two-dimensional, and contour plot in two-dimensional plots.

2.2. Modified Khater method

Applying this scheme to Equation (3) leads to the following general solution (17) $\begin{array}{rl}\mathcal{U}\left(\mathfrak{E}\right)& =a_0+\sum _{i=1}^n{a}_i{k}^{if\left(\mathfrak{E}\right)}+\sum _{i=1}^n{b}_i\ast k^{-if\left(\mathfrak{E}\right)}\\ & =a_0+k^{f\left(\mathfrak{E}\right)}{a}_1+k^{2f\left(\mathfrak{E}\right)}{a}_2\\ & +k^{-f\left(\mathfrak{E}\right)}{b}_1+k^{-2f\left(\mathfrak{E}\right)}{b}_2,\end{array}$(17) where $a_i{b}_j,\left(i=0,1,2,j=1,2\right)$ are arbitrary constants. Also, $f\left(\mathfrak{E}\right)$ satisfies (18) $f^{\mathrm{\prime }}\left[\xi \right]=\frac{1}{\text{Log}\left(k\right)}\left[\text{ϟ}{k}^{-f\left(\mathfrak{E}\right)}+\text{ϡ}+\mathcal{r}{k}^{f\left(\mathfrak{E}\right)}\right],$(18) where $\text{ϟ},\text{ϡ},\mathcal{r}$ are arbitrary constants. Substituting Equa-tion (17) along (18) into Equation (3) and collecting all terms with the same power of $k^{if\left(\mathfrak{E}\right)},(j=-7,-6,\dots ,6,7)$ lead to a system of algebraic equations. Solving this system yields

Family A $\begin{array}{rl}{a}_0& =-\frac{\begin{array}{c}6\text{ϟ}{\text{ϡ}}^2-\text{ϡ}\left(8\mathcal{r}\text{ϟ}+{\text{ϡ}}^2\right)b_1+\\ \sqrt{36{\text{ϟ}}^2{\text{ϡ}}^4+{\left(-4\mathcal{r}\text{ϟ}\text{ϡ}+{\text{ϡ}}^3\right)}^2{b}_1\left(-12\text{ϟ}\text{ϡ}+b_1\right)}\end{array}}{12\text{ϟ}{\text{ϡ}}^2},\\ & a_1=a_2=0,b_2=\frac{\text{ϟ}{b}_1}{\text{ϡ}},\end{array}$ $\begin{array}{rl}c& =\frac{\begin{array}{c}6\text{ϟ}{\text{ϡ}}^2+\\ \sqrt{36{\text{ϟ}}^2{\text{ϡ}}^4+{\left(-4\mathcal{r}\text{ϟ}\text{ϡ}+{\text{ϡ}}^3\right)}^2{b}_1\left(-12\text{ϟ}\text{ϡ}+b_1\right)}\end{array}}{2\text{ϡ}\left(6\text{ϟ}\text{ϡ}-b_1\right)},\\ & \mathrm{\wp }=\frac{-12\text{ϟ}\text{ϡ}+b_1}{6\text{ϟ}\text{ϡ}-b_1}.\end{array}$

Family B $\begin{array}{rl}{a}_0& =-\frac{\begin{array}{c}6\mathcal{r}{\text{ϡ}}^2-\text{ϡ}\left(8\mathcal{r}\text{ϟ}+{\text{ϡ}}^2\right)a_1+\\ \sqrt{36{\mathcal{r}}^2{\text{ϡ}}^4+{\left(-4\mathcal{r}\text{ϟ}\text{ϡ}+{\text{ϡ}}^3\right)}^2{a}_1\left(-12\mathcal{r}\text{ϡ}+a_1\right)}\end{array}}{12\mathcal{r}{\text{ϡ}}^2},\\ & a_2\to \frac{\mathcal{r}{a}_1}{\text{ϡ}},b_1=b_2=0,\end{array}$ $\begin{array}{rl}c& =\frac{\begin{array}{c}6\mathcal{r}{\text{ϡ}}^2+\\ \sqrt{36{\mathcal{r}}^2{\text{ϡ}}^4+{\left(-4\mathcal{r}\text{ϟ}\text{ϡ}+{\text{ϡ}}^3\right)}^2{a}_1\left(-12\mathcal{r}\text{ϡ}+a_1\right)}\end{array}}{2\text{ϡ}\left(6\mathcal{r}\text{ϡ}-a_1\right)},\\ & \mathrm{\wp }=\frac{-12\mathcal{r}\text{ϡ}+a_1}{6\mathcal{r}\text{ϡ}-a_1}.\end{array}$

Consequently, the solitary wave solutions of the GM-DP-CH are given based on family A by

For ${\text{ϡ}}^2-4\text{ϟ}\mathcal{r}<0,\mathcal{r}\ne 0;$ (19) $\begin{array}{rl}& {\mathcal{W}}_{A,1}\left(x,t\right)\\ & =\frac{1}{12}[\phantom{\frac{3}{\begin{array}{c}\mathrm{\wp }-\sqrt{4\mathrm{\wp }\mathrm{\wp }-{\mathrm{\wp }}^2}\text{Tan}\\ \left[1/2\left(ct+x\right)\sqrt{4\mathrm{\wp }\mathrm{\wp }-{\mathrm{\wp }}^2}\right]\end{array}}}-6\\ & -\frac{\sqrt{36{\text{ϟ}}^2{\text{ϡ}}^4+{\left(-4\mathcal{r}\text{ϟ}\text{ϡ}+{\text{ϡ}}^3\right)}^2{b}_1\left(-12\text{ϟ}\text{ϡ}+b_1\right)}}{\text{ϟ}{\text{ϡ}}^2}\\ & +b_1(\frac{\text{ϡ}}{\text{ϟ}}+\frac{48{\mathcal{r}}^2\text{ϟ}}{\begin{array}{c}\text{ϡ}(\text{ϡ}-\sqrt{4\mathcal{r}\text{ϟ}-{\text{ϡ}}^2}\text{Tan}\\ {\left[1/2\left(ct+x\right)\sqrt{4\mathcal{r}\text{ϟ}-{\text{ϡ}}^2}\right])}^2\end{array}}\\ & +8\mathcal{r}\left(\frac{1}{\text{ϡ}}-\frac{3}{\begin{array}{c}\text{ϡ}-\sqrt{4\mathcal{r}\text{ϟ}-{\text{ϡ}}^2}\text{Tan}\\ \left[1/2\left(ct+x\right)\sqrt{4\mathcal{r}\text{ϟ}-{\text{ϡ}}^2}\right]\end{array}}\right))],\end{array}$(19) (20) $\begin{array}{rl}& {\mathcal{W}}_{A,2}\left(x,t\right)\\ & =\frac{1}{12}[-6+(\frac{\text{ϡ}}{\text{ϟ}}+\frac{48{\mathcal{r}}^2\text{ϟ}}{\begin{array}{c}\text{ϡ}(\text{ϡ}-\sqrt{4\mathcal{r}\text{ϟ}-{\text{ϡ}}^2}\text{Cot}\\ {\left[1/2\left(ct+x\right)\sqrt{4\mathcal{r}\text{ϟ}-{\text{ϡ}}^2}\right])}^2\end{array}}\\ & +8\mathcal{r}(\frac{1}{\text{ϡ}}-\frac{3}{\begin{array}{c}\text{ϡ}-\sqrt{4\mathcal{r}\text{ϟ}-{\text{ϡ}}^2}\text{Cot}\\ \left[1/2\left(ct+x\right)\sqrt{4\mathcal{r}\text{ϟ}-{\text{ϡ}}^2}\right]\end{array}}))b_1\\ & -\frac{\sqrt{36{\text{ϟ}}^2{\text{ϡ}}^4+{\left(-4\mathcal{r}\text{ϟ}\text{ϡ}+{\text{ϡ}}^3\right)}^2{b}_1\left(-12\text{ϟ}\text{ϡ}+b_1\right)}}{\text{ϟ}{\text{ϡ}}^2}].\end{array}$(20)

For ${\text{ϡ}}^2-4\text{ϟ}\mathcal{r}>0,\mathcal{r}\ne 0;$ (21) $\begin{array}{rl}& {\mathcal{W}}_{A,3}\left(x,t\right)\\ & =\frac{1}{12}[\phantom{\frac{3}{\begin{array}{c}\mathrm{\wp }+\sqrt{-4\mathrm{\wp }\mathrm{\wp }+{\mathrm{\wp }}^2}\text{Tanh}\\ \left[1/2\left(ct+x\right)\sqrt{-4\mathrm{\wp }\mathrm{\wp }+{\mathrm{\wp }}^2}\right]\end{array}}}-6\\ & -\frac{\sqrt{36{\text{ϟ}}^2{\text{ϡ}}^4+{\left(-4\mathcal{r}\text{ϟ}\text{ϡ}+{\text{ϡ}}^3\right)}^2{b}_1\left(-12\text{ϟ}\text{ϡ}+b_1\right)}}{\text{ϟ}{\text{ϡ}}^2}\\ & +b_1(\frac{\text{ϡ}}{\text{ϟ}}+\frac{48{\mathcal{r}}^2\text{ϟ}}{\begin{array}{c}\text{ϡ}(\text{ϡ}+\sqrt{-4\mathcal{r}\text{ϟ}+{\text{ϡ}}^2}\text{Tanh}\\ {\left[1/2\left(ct+x\right)\sqrt{-4\mathcal{r}\text{ϟ}+{\text{ϡ}}^2}\right])}^2\end{array}}\\ & +8\mathcal{r}\left((\frac{1}{\text{ϡ}}-\frac{3}{\begin{array}{c}\text{ϡ}+\sqrt{-4\mathcal{r}\text{ϟ}+{\text{ϡ}}^2}\text{Tanh}\\ \left[1/2\left(ct+x\right)\sqrt{-4\mathcal{r}\text{ϟ}+{\text{ϡ}}^2}\right]\end{array}})\right)],\end{array}$(21) (22) $\begin{array}{rl}& {\mathcal{W}}_{A,4}\left(x,t\right)\\ & =\frac{1}{12}[-6+[\frac{\text{ϡ}}{\text{ϟ}}+\frac{48{\mathcal{r}}^2\text{ϟ}}{\begin{array}{c}\text{ϡ}(\text{ϡ}+\sqrt{-4\mathcal{r}\text{ϟ}+{\text{ϡ}}^2}\text{Coth}\\ {\left[1/2\left(ct+x\right)\sqrt{-4\mathcal{r}\text{ϟ}+{\text{ϡ}}^2}\right])}^2\end{array}}\\ & +8\mathcal{r}\left((\frac{1}{\text{ϡ}}-\frac{3}{\begin{array}{c}\text{ϡ}+\sqrt{-4\mathcal{r}\text{ϟ}+{\text{ϡ}}^2}\text{Coth}\\ \left[1/2\left(ct+x\right)\sqrt{-4\mathcal{r}\text{ϟ}+{\text{ϡ}}^2}\right]\end{array}})\right]b_1\\ & -\frac{\sqrt{36{\text{ϟ}}^2{\text{ϡ}}^4+{\left(-4\mathcal{r}\text{ϟ}\text{ϡ}+{\text{ϡ}}^3\right)}^2{b}_1\left(-12\text{ϟ}\text{ϡ}+b_1\right)}}{\text{ϟ}{\text{ϡ}}^2}\phantom{\frac{48{\mathrm{\wp }}^2\mathrm{\wp }}{\begin{array}{c}\mathrm{\wp }(\mathrm{\wp }+\sqrt{-4\mathrm{\wp }\mathrm{\wp }+{\mathrm{\wp }}^2}\text{Coth}\\ {\left[1/2\left(ct+x\right)\sqrt{-4\mathrm{\wp }\mathrm{\wp }+{\mathrm{\wp }}^2}\right])}^2\end{array}}}].\end{array}$(22)

For $\text{ϡ}=\frac{\text{ϟ}}{2}=\kappa ,\mathcal{r}=0;$ (23) $\begin{array}{rl}{\mathcal{W}}_{A,5}\left(x,t\right)& =-\frac{1}{2}+(\frac{1}{24}+\frac{2}{{\left(-2+{\text{e}}^{\left(ct+x\right)\kappa }\right)}^2}\\ & +\frac{1}{-2+{\text{e}}^{\left(ct+x\right)\kappa }})b_1\\ & -\frac{\sqrt{{\kappa }^6\left(144-24{\kappa }^2{b}_1+b_1^2\right)}}{24{\kappa }^3}.\end{array}$(23)

For $\mathcal{r}=0,\text{ϡ}\ne 0,\text{ϟ}\ne 0;$ (24) $\begin{array}{rl}{\mathcal{W}}_{A,6}\left(x,t\right)& =-\frac{1}{2}+(\frac{\text{ϡ}}{12\text{ϟ}}+\frac{\text{ϟ}\text{ϡ}}{{\left(\text{ϟ}-{\text{e}}^{\left(ct+x\right)\text{ϡ}}\text{ϡ}\right)}^2}\\ & +\frac{\text{ϡ}}{-\text{ϟ}+{\text{e}}^{\left(ct+x\right)\text{ϡ}}\text{ϡ}})b_1\\ & -\frac{\sqrt{36{\text{ϟ}}^2{\text{ϡ}}^4+{\text{ϡ}}^6{b}_1\left(-12\text{ϟ}\text{ϡ}+b_1\right)}}{12\text{ϟ}{\text{ϡ}}^2}.\end{array}$(24)

For ${\text{ϡ}}^2-4\text{ϟ}\mathcal{r}=0;$ (25) $\begin{array}{rl}& {\mathcal{W}}_{A,7}\left(x,t\right)\\ & =\frac{-1}{12\text{ϟ}{\text{ϡ}}^2}[\phantom{\frac{\begin{array}{c}{\mathrm{\wp }}^2(-2+(ct+x)\mathrm{\wp }\\ \left(4+ct\mathrm{\wp }+x\mathrm{\wp }\right))\end{array}}{{\left(2+ct\mathrm{\wp }+x\mathrm{\wp }\right)}^2}}6(\text{ϟ}{\text{ϡ}}^2+\sqrt{{\text{ϟ}}^2{\text{ϡ}}^4})\\ & +2\text{ϡ}(-4\mathcal{r}\text{ϟ}+\frac{\begin{array}{c}{\text{ϡ}}^2(-2+(ct+x)\text{ϡ}\\ \left(4+ct\text{ϡ}+x\text{ϡ}\right))\end{array}}{{\left(2+ct\text{ϡ}+x\text{ϡ}\right)}^2})b_1]\end{array}$(25)

Consequently, the solitary wave solutions of the GM-DP-CH are given based on family B by

For ${\text{ϡ}}^2-4\text{ϟ}\mathcal{r}<0,\mathcal{r}\ne 0;$ (26) $\begin{array}{rl}& {\mathcal{W}}_{B,1}\left(x,t\right)\\ & =\frac{-1}{12\mathcal{r}{\text{ϡ}}^2}[\phantom{\frac{1}{2}}6\mathcal{r}{\text{ϡ}}^2+\text{ϡ}(-4\mathcal{r}\text{ϟ}+{\text{ϡ}}^2)\\ & \times (-1+3\text{Sec}{\left[\frac{1}{2}\left(ct+x\right)\sqrt{4\mathcal{r}\text{ϟ}-{\text{ϡ}}^2}\right]}^2)a_1\\ & +\sqrt{\begin{array}{c}36{\mathcal{r}}^2{\text{ϡ}}^4+{\left(-4\mathcal{r}\text{ϟ}\text{ϡ}+{\text{ϡ}}^3\right)}^2\\ a_1\left(-12\mathcal{r}\text{ϡ}+a_1\right)\end{array}}\phantom{\frac{1}{2}}],\end{array}$(26) (27) $\begin{array}{rl}& {\mathcal{W}}_{B,2}\left(x,t\right)\\ & =\frac{-1}{12\mathcal{r}{\text{ϡ}}^2}[\phantom{\frac{1}{2}}6\mathcal{r}{\text{ϡ}}^2+\text{ϡ}(-4\mathcal{r}\text{ϟ}+{\text{ϡ}}^2)\\ & \times (-1+3\text{Sec}{\left[\frac{1}{2}\left(ct+x\right)\sqrt{4\mathcal{r}\text{ϟ}-{\text{ϡ}}^2}\right]}^2)a_1\\ & +\sqrt{36{\mathcal{r}}^2{\text{ϡ}}^4+{(-4\mathcal{r}\text{ϟ}\text{ϡ}+{\text{ϡ}}^3)}^2{a}_1\left(-12\mathcal{r}\text{ϡ}+a_1\right)}\phantom{\frac{1}{2}}].\end{array}$(27)

For ${\text{ϡ}}^2-4\text{ϟ}\mathcal{r}>0,\mathcal{r}\ne 0;$ (28) $\begin{array}{rl}& {\mathcal{W}}_{B,3}\left(x,t\right)\\ & =\frac{-1}{12\mathcal{r}{\text{ϡ}}^2}[\phantom{\frac{1}{2}}6\mathcal{r}{\text{ϡ}}^2+\text{ϡ}(-4\mathcal{r}\text{ϟ}+{\text{ϡ}}^2)\\ & \times \left(-1+3\text{Sech}{\left[\frac{1}{2}\left(ct+x\right)\sqrt{-4\mathcal{r}\text{ϟ}+{\text{ϡ}}^2}\right]}^2\right)a_1\\ & +\sqrt{\begin{array}{c}36{\mathcal{r}}^2{\text{ϡ}}^4+{\left(-4\mathcal{r}\text{ϟ}\text{ϡ}+{\text{ϡ}}^3\right)}^2\\ a_1\left(-12\mathcal{r}\text{ϡ}+a_1\right)\end{array}}\phantom{\frac{1}{2}}],\end{array}$(28) (29) $\begin{array}{rl}& {\mathcal{W}}_{B,4}\left(x,t\right)\\ & =\frac{-1}{12\mathcal{r}{\text{ϡ}}^2}[\phantom{\frac{1}{2}}6\mathcal{r}{\text{ϡ}}^2-\text{ϡ}(-4\mathcal{r}\text{ϟ}+{\text{ϡ}}^2)\\ & \times \left(1+3\text{Csch}{\left[\frac{1}{2}\left(ct+x\right)\sqrt{-4\mathcal{r}\text{ϟ}+{\text{ϡ}}^2}\right]}^2\right)a_1\\ & +\sqrt{\begin{array}{c}36{\mathcal{r}}^2{\text{ϡ}}^4+{\left(-4\mathcal{r}\text{ϟ}\text{ϡ}+{\text{ϡ}}^3\right)}^2\\ a_1\left(-12\mathcal{r}\text{ϡ}+a_1\right)\end{array}}\phantom{\frac{1}{2}}].\end{array}$(29)

For $\text{ϡ}=\mathcal{r}=\kappa ,\text{ϟ}=0;$ (30) $\begin{array}{rl}& {\mathcal{W}}_{B,5}\left(x,t\right)\\ & =\frac{1}{12}[\phantom{\frac{\sqrt{{\kappa }^6\left(36-12{\kappa }^2{a}_1+a_1^2\right)}}{{\kappa }^3}}-6+\left(1+3\text{Csch}{\left[\frac{1}{2}\left(ct+x\right)\kappa \right]}^2\right)a_1\\ & -\frac{\sqrt{{\kappa }^6\left(36-12{\kappa }^2{a}_1+a_1^2\right)}}{{\kappa }^3}].\end{array}$(30)

For $\text{ϟ}=0,\text{ϡ}\ne 0,\mathcal{r}\ne 0;$ (31) $\begin{array}{rl}& {\mathcal{W}}_{B,6}\left(x,t\right)\\ & =\frac{1}{12}[-6+\frac{\left(4+{\text{e}}^{\left(ct+x\right)\text{ϡ}}\mathcal{r}\left(20+{\text{e}}^{\left(ct+x\right)\text{ϡ}}\mathcal{r}\right)\right)\text{ϡ}{a}_1}{\mathcal{r}{\left(-2+{\text{e}}^{\left(ct+x\right)\text{ϡ}}\mathcal{r}\right)}^2}\\ & -\frac{\sqrt{36{\mathcal{r}}^2{\text{ϡ}}^4+{\text{ϡ}}^6{a}_1\left(-12\mathcal{r}\text{ϡ}+a_1\right)}}{\mathcal{r}{\text{ϡ}}^2}].\end{array}$(31)

For ${\text{ϡ}}^2-4\text{ϟ}\mathcal{r}=0;$ Figures 4 and 5 (32) $\begin{array}{rl}& {\mathcal{W}}_{B,7}\left(x,t\right)\\ & =\frac{1}{12}[\phantom{\frac{\begin{array}{c}\left(\right({\mathrm{\wp }}^6/\mathrm{\wp })+(48\mathrm{\wp }{\mathrm{\wp }}^2{\left(2+ct\mathrm{\wp }+x\mathrm{\wp }\right)}^2/\\ \left(ct+x{)}^2\right)-\left(16\mathrm{\wp }{\mathrm{\wp }}^3\right(3+ct\mathrm{\wp }+x\mathrm{\wp })/\\ ct+x\left)\right)a_1\end{array}}{{\mathrm{\wp }}^5}}-6-\frac{6\mathcal{r}{\text{ϡ}}^2}{\sqrt{{\mathcal{r}}^2{\text{ϡ}}^4}}\\ & +\frac{\begin{array}{c}\left(\right({\text{ϡ}}^6/\mathcal{r})+(48\mathcal{r}{\text{ϟ}}^2{\left(2+ct\text{ϡ}+x\text{ϡ}\right)}^2/\\ \left(ct+x{)}^2\right)-\left(16\text{ϟ}{\text{ϡ}}^3\right(3+ct\text{ϡ}+x\text{ϡ})/ct+x))a_1\end{array}}{{\text{ϡ}}^5}].\end{array}$(32)

Figure 4. Periodic cone wave solutions of Equation (19) when $\text{ϡ}=5,\mathcal{r}=1,\text{ϟ}=6,{\mathit{b}}_1=100$ in three, two-dimensional, and contour plot in two-dimensional plots.

Figure 5. Periodic cone wave solutions of Equation (21) when $\text{ϡ}=2,\mathcal{r}=0,\text{ϟ}=4,\mathit{\kappa }=2,{\mathit{b}}_1=100$ in three, two-dimensional, and contour plot in two-dimensional plots.

3. Results and discussion

In this section, we discuss our obtained results of the GM-DP-CH equation through two used employed analytical schemes (The modified exponential expansion method and the modified Khater method). Firstly, we show the difference between our two used schemes and then we go for the coincide and difference between the obtained solutions by each one of the above-mentioned schemes. Finally, we show the novelty and originality of our paper:

1. Used schemes:

   The modified exponential expansion method and the modified Khater method have been employed to evaluate the exact travelling wave solutions of the GM-DP-CH equation. Both of these methods depend on auxiliary equation (5), (19) that play an essential role in finding the exact solutions that we have found however, Equations (5), (19) are equal when $\text{e}=k,\varrho =\text{ϡ},\text{ϟ}=1$. This equivalence makes the obtained solutions of both methods are also equal.

2. Obtained results:

   We can find the equivalence between the solutions that have been obtained via the above-mentioned schemes. We shall show the equivalence solutions as follows:

   Equations (6), (7) are equal to Equations (17), (18) when $a_1=b_1,\text{ϟ}=1$. However the discussed equivalence between the used methods but the modified Khater method has obtained more solutions than modified exponential expansion method that explains the superiority of the modified Khater method over the modified exponential expansion method.

3. Previous obtained solutions:

   Comparing our obtained results with that have been obtained Linares, F., Ponce, G., & Sideris, T. C (2019), shows the novelty of our solutions where the studied the property of for the solutions of properties of solutions to the IVP associated to the Camassa-Holm equation on the line related to the regularity and the decay of solutions but all our solutions in this paper is completely different from that have been obtained in their paper.

4. Conclusion

We succeed in constructing many distinct formulas of exact travelling and solitary wave solutions of the GM-DP-CH equation via the modified exponential expansion method and the modified Khater method. These obtained computational solutions explain the dynamical behaviour of shallow-water waves. Moreover, for further explanation of our obtained solutions, some distinct types of sketches were given in two, three-dimensional, and contour plots. The powerful and capable of both methods have been verified. The ability of both methods for applying to other nonlinear partial differential equation with an-integer and fractional order have been illustrated. Our future papers will aim to show the accuracy of the obtained solutions in this paper by applying numerical scheme to this model to illustrate the absolute error between exact and numerical solutions.

Acknowledgements

B. Almohsen is Supported by Researchers Supporting Project number (RSP-2020/158), King Saud University, Riyadh, Saudi Arabia.

Data Availability

All data used for the findings in this research are available publicly in manuscript.

Disclosure statement

No potential conflict of interest was reported by the author(s).

Additional information

Funding

B. Almohsen is Supported by Researchers Supporting Project number (RSP-2020/158), King Saud University, Riyadh, Saudi Arabia.