Traces of Sturm-Liouville operators with discontinuities

Abstract

This paper deals with the eigenvalue problems for the second-order differential operators with discontinuities inside a finite interval. We will obtain some formulas for the regularized traces of the second-order differential operators with discontinuities.

Keywords:

• second-order differential operator
• discontinuous condition
• regularized trace formula

AMS Subject Classifications:

• 34B24
• 34L20
• 47E05

Introduction

The operator under consideration is defined by the differential expression on the interval $(0,1/2)\bigcup (1/2,1)$,(1) $$\begin{array}{c}\hfill l(y):=-y^{\prime \prime }(x)+q(x)y(x)={\mathit{\lambda }}^{2}y(x),\end{array}$$(1) subject to the general boundary conditions(2) $$\begin{array}{c}\hfill y^{\prime }(0)-h_{1}y(0)=0,y^{\prime }(1)+h_{2}y(1)=0\end{array}$$(2) and the interface condition(3) $$\begin{array}{c}\hfill y(1/2+0)=a_{1}y(1/2-0),y^{\prime }(1/2+0)=a_1^{-1}{y}^{\prime }(1/2-0)+a_{2}y(1/2-0).\end{array}$$(3) Here, the real-valued function $q\in W_1^1((0,1/2)\bigcup (1/2,1))$, ${\mathit{\lambda }}^2$ is the spectral parameter, $h_1,h_2,a_1$ and $a_2$ are real parameters, and $a_1>0$. Moreover, $h_1$ and/or $h_2$ are allowed to be $\infty$, and then the corresponding boundary condition is interpreted as a Dirichlet one, so that all possible separated boundary conditions are considered. We denote the boundary value problem (1(1) $$\begin{array}{c}\hfill l(y):=-y^{\prime \prime }(x)+q(x)y(x)={\mathit{\lambda }}^{2}y(x),\end{array}$$(1) ), (2(2) $$\begin{array}{c}\hfill y^{\prime }(0)-h_{1}y(0)=0,y^{\prime }(1)+h_{2}y(1)=0\end{array}$$(2) ) and (3(3) $$\begin{array}{c}\hfill y(1/2+0)=a_{1}y(1/2-0),y^{\prime }(1/2+0)=a_1^{-1}{y}^{\prime }(1/2-0)+a_{2}y(1/2-0).\end{array}$$(3) ) by $B=B(q,h_1,h_2,a_1,a_2)$. In all cases, the corresponding operator $B$ is self-adjoint, and possesses a real and simple discrete spectrum.

Boundary value problems with a discontinuity condition inside the interval frequently arise in mathematics, mechanics, radio electronics, geophysics and other fields of science and technology. As a rule, such problems are related to discontinuous and non-smooth properties of a medium (e.g. see [1, 3, 4, 14, 20, 23, 24]). Most of these are motivated by geophysical models for oscillations of the Earth.

The presence of discontinuous conditions makes the analysis quite different. Some aspects of direct and inverse problems for differential operators with discontinuous conditions were considered. In particular, it was shown in [24] that if $q(x)$ is a priori known for $x\in (0,1/2)$, then the function $q(x)$ on the interval $(1/2,1)$ is uniquely determined by the spectrum. The case of a half-line was considered in [20]. In [7, 9], the author proved that for recovering $q(x)$ on the whole interval, it is necessary to specify two spectra of boundary value problems with different boundary conditions. In [10], authors studied some inverse problems of recovering $B$ from its spectral characteristics: Weyl function, eigenvalues and norming constants, and two spectra, respectively. In [23], authors assumed the coefficients $a_1$ and $a_2$ from (3(3) $$\begin{array}{c}\hfill y(1/2+0)=a_{1}y(1/2-0),y^{\prime }(1/2+0)=a_1^{-1}{y}^{\prime }(1/2-0)+a_{2}y(1/2-0).\end{array}$$(3) ) to be known a priori and fixed, and obtained some results on inverse nodal and inverse spectral problems, and established connections between them.

Gelfand and Levitan [11], assuming the continuous differentiability of the function $q(x)$, obtained the following remarkable formula for the regularized trace:(4) $$\begin{array}{c}\hfill \sum _{n=1}^{\infty }({\mathit{\lambda }}_n^2-n^2{\mathit{\pi }}^2)=-\frac{q(0)+q(1)}{4}.\end{array}$$(4) Here, the ${\mathit{\lambda }}_n^2$ are eigenvalues of the operator$$\begin{array}{c}\hfill -y^{\prime \prime }+q(x)y={\mathit{\lambda }}^{2}y,y(0)=0=y(1),\end{array}$$and it is assumed that the mean value of the potential $q(x)$ is zero on the interval $[0,1]$. This work was continued by many authors. Here, we refer e.g. to the papers [2, 5, 6, 11–13, 15–19, 21]. The trace formulas for the scalar differential operators have been found by Gelfand and Levitan [11], Dikii [8], Halberg and Kramer [22] and many other works. The list of the works on this subject is given in [17–19]. The trace identity of a differential operator deeply reveals spectral structure of the differential operator and has important applications in the numerical calculation of eigenvalues, inverse problem, theory of solitons, and theory of integrable system.[25]

The trace problems attract our attention in connection with the study of the second-order differential operator with discontinuity inside a finite interval. Although the interface conditions of the above form were suggested by Hald as back as in 1984, the problems considered in this paper have not been discussed before. The paper extends aspects of the spectral theory for Sturm-Liouville operators on a finite interval to the case where special interface conditions at the midpoint are imposed. Namely, we treat one forward problem for the eigenvalue problem and the results may produce some results for the inverse eigenvalue problem; then, we establishes the trace formula for such operators and thus generalize the classical result of Gelfand and Levitan of 1953. Since such interface conditions arise in several physical problems, the results of the paper are related to some classical geophysical problem; peculiar asymptotic behaviour, called solo-tone effect (see e.g. [14]) of eigenvalues of the Sturm-Liouville problem with elastic coefficient possessing one discontinuity point, which is a classical model of the Earth’s crust.

Gelfand-Levitan’s trace

The trace formulas depend on the boundary conditions. Namely, there are four cases: (I) RR-type: $h_1,h_2\in \mathbb{R}$; (II) DD-type: $h_1=h_2=\infty$; (III) RD-type: $h_1\in \mathbb{R},h_2=\infty$; (IV) DR-type: $h_2\in \mathbb{R},h_1=\infty$.

Denote the eigenvalues of the problem B by ${\mathit{\lambda }}_n^2$, then the following holds.

Theorem 2.1

The spectrum $\{{\mathit{\lambda }}_n^2\}$ of the problem B has the following asymptotic distribution: for sufficiently large $n$$$\begin{array}{c}\hfill {\mathit{\lambda }}_n=\{\begin{array}{cc}{\mathit{\mu }}_n+\frac{{(-1)}^{n}a+b}{{\mathit{\mu }}_n}+O\left(\frac{1}{n^2}\right)\hfill & \mathit{bad}\mathit{hboxbad}\mathit{hbox},\hfill \\ {\mathit{\mu }}_n+\frac{b}{{\mathit{\mu }}_n}+O\left(\frac{1}{n^2}\right)\hfill & \mathit{bad}\mathit{hboxbad}\mathit{hbox},\hfill \end{array}\end{array}$$where$$\begin{array}{c}\hfill {\mathit{\mu }}_n=\{\begin{array}{cc}n\mathit{\pi }\hfill & \mathit{bad}\mathit{hboxbad}\mathit{hbox},\hfill \\ (n+\frac{1}{2})\mathit{\pi }+{(-1)}^{n-1}\arcsin a_0\hfill & \mathit{bad}\mathit{hbox}\mathit{bad}\mathit{hbox}\hfill \\ (n+\frac{1}{2})\mathit{\pi }+{(-1)}^n\arcsin a_0\hfill & \mathit{bad}\mathit{hbox}\text{for}\text{DR-type},\hfill \end{array}\end{array}$$$$\begin{array}{c}\hfill a_0=\frac{a_1^{-1}-a_1}{a_1+a_1^{-1}},\end{array}$$and$$\begin{array}{c}\hfill a=\{\begin{array}{cc}\frac{a_2}{a_1+a_1^{-1}}+\frac{a_1-a_1^{-1}}{a_1+a_1^{-1}}(\frac{1}{2}{\int }_{\frac{1}{2}}^{1}q(t)\mathit{dt}+h_2-\frac{1}{2}{\int }_0^{\frac{1}{2}}q(t)\mathit{dt}-h_1)\hfill & \mathit{bad}\mathit{hbox}\text{for}\text{RR-type},\hfill \\ -\frac{a_2}{a_1+a_1^{-1}}-\frac{a_1-a_1^{-1}}{a_1+a_1^{-1}}(\frac{1}{2}{\int }_{\frac{1}{2}}^{1}q(t)\mathit{dt}-\frac{1}{2}{\int }_0^{\frac{1}{2}}q(t)\mathit{dt})\hfill & \mathit{bad}\mathit{hbox}\text{for}\text{DD-type},\hfill \end{array}\end{array}$$$$\begin{array}{c}\hfill b=\{\begin{array}{cc}\frac{a_2}{a_1+a_1^{-1}}+\frac{1}{2}{\int }_0^{1}q(t)\mathit{dt}+h_1+h_2\hfill & \mathit{bad}\mathit{hbox}\text{for}\text{RR-type},\hfill \\ \frac{a_2}{a_1+a_1^{-1}}+\frac{1}{2}{\int }_0^{1}q(t)\mathit{dt}\hfill & \mathit{bad}\mathit{hbox}\text{for}\text{DD-type},\hfill \\ \frac{a_2}{a_1+a_1^{-1}}+\frac{1}{2}{\int }_0^{1}q(t)\mathit{dt}+h_1\hfill & \mathit{bad}\mathit{hbox}\text{for}\text{RD-type},\hfill \\ \frac{a_2}{a_1+a_1^{-1}}+\frac{1}{2}{\int }_0^{1}q(t)\mathit{dt}+h_2\hfill & \mathit{bad}\mathit{hbox}\text{for}\text{DR-type}.\hfill \end{array}\end{array}$$

Now we can present formulas for the sums of eigenvalues ${\mathit{\lambda }}_n^2$, $n\in A$, which are so-called regularized traces. Here$$\begin{array}{c}\hfill A=\{\begin{array}{cc}\{\pm 0,\pm 1,\pm 2,\cdots \}\hfill & \mathit{bad}\mathit{hbox}\text{for}\text{RR-type},\hfill \\ \{\pm 1,\pm 2,\cdots \}\hfill & \mathit{bad}\mathit{hbox}\text{for}\text{DD-type},\hfill \\ \{0,\pm 1,\pm 2,\cdots \}\hfill & \mathit{bad}\mathit{hbox}\text{for}\mathit{bad}\mathit{hbox}.\hfill \end{array}\end{array}$$

Theorem 2.2

We have the following formulas of the regularized trace for the problem B.

1. For RR-type$$\begin{array}{cc}\hfill & \sum _{n\in A}[{\mathit{\lambda }}_n^2-{\mathit{\mu }}_n^2-{(-1)}^{n}2a-2b]\hfill \\ \hfill & =-\frac{4a_2}{a_1+a_1^{-1}}-\frac{a_1-a_1^{-1}}{a_1+a_1^{-1}}({\int }_{\frac{1}{2}}^{1}q(t)\mathit{dt}+2h_2-{\int }_0^{\frac{1}{2}}q(t)\mathit{dt}-2h_1)\hfill \\ \hfill \\ \hfill & -\frac{a_2^2}{{(a_1+a_1^{-1})}^2}+\frac{q(0)+q(1)}{2}-{\int }_0^{1}q(t)\mathit{dt}-2(h_1+h_2)-(h_1^2+h_2^2).\hfill \end{array}$$

2. For DD-type$$\begin{array}{cc}\hfill & \sum _{n\in A}[{\mathit{\lambda }}_n^2-{\mathit{\mu }}_n^2-{(-1)}^{n+1}2a-2b]\hfill \\ \hfill & =-\frac{a_1-a_1^{-1}}{a_1+a_1^{-1}}({\int }_{\frac{1}{2}}^{1}q(t)\mathit{dt}-{\int }_0^{\frac{1}{2}}q(t)\mathit{dt})-\frac{a_2^2}{{(a_1+a_1^{-1})}^2}-\frac{q(0)+q(1)}{2}+{\int }_0^{1}q(t)\mathit{dt}.\hfill \end{array}$$

3. For RD-type$$\begin{array}{cc}\hfill & \sum _{n\in A}({\mathit{\lambda }}_n^2-{\mathit{\mu }}_n^2-2b)\hfill \\ \hfill & =\frac{4(a_0{c}_2-c_1)a_0}{a_0-1}-\frac{a_2^2}{{(a_1+a_1^{-1})}^2}+\frac{q(0)-q(1)}{2}-h_1^2.\hfill \end{array}$$

4. For DR-type$$\begin{array}{cc}\hfill & \sum _{n\in A}({\mathit{\lambda }}_n^2-{\mathit{\mu }}_n^2-2b)\hfill \\ \hfill & =-\frac{4(a_0{c}_2+c_1)a_0}{a_0+1}-\frac{a_2^2}{{(a_1+a_1^{-1})}^2}+\frac{q(1)-q(0)}{2}-h_2^2.\hfill \end{array}$$

Remark 2.3

In particular, from the formula (2) with $a_1=1$ and $a_2=0$, we can obtain Gelfand and Levitan’s trace formula (4(4) $$\begin{array}{c}\hfill \sum _{n=1}^{\infty }({\mathit{\lambda }}_n^2-n^2{\mathit{\pi }}^2)=-\frac{q(0)+q(1)}{4}.\end{array}$$(4) ).

Proofs of Theorems 2.1 and 2.2    We only give the proofs for RR-type in Theorems 2.1 and 2.2. Analogously, we can also prove the other cases in Theorems 2.1 and 2.2.

Step 1 To establish the characteristic function$\mathbf{\Delta }(\mathit{\lambda })$

If we let$$\begin{array}{c}\hfill y_1(x)=y(x),y_2(x)=y(1-x),q_1(x)=q(x),q_2(x)=q(1-x)\end{array}$$for $0\le x\le 1/2$, then the problem $B$ can be transformed to$$\begin{array}{c}\hfill {\overrightarrow{y}}^{\prime }+({\mathit{\lambda }}^2{I}_2-Q(x))\overrightarrow{y}=0,0<x<1/2,\end{array}$$with boundary conditions$$\begin{array}{c}\hfill {\overrightarrow{y}}^{\prime }(0)-H\overrightarrow{y}(0)=0,A_1{\overrightarrow{y}}^{\prime }(1/2)+B_1\overrightarrow{y}(1/2)=0,\end{array}$$where $\overrightarrow{y}(x)=\left(\begin{array}{c}\hfill y_1(x)\hfill \\ \hfill y_2(x)\hfill \end{array}\right)$, $H=\text{diag}(h_1,h_2)$, and$$\begin{array}{c}\hfill Q(x)=\left(\begin{array}{cc}\hfill q_1(x)\hfill & \hfill 0\hfill \\ \hfill 0\hfill & \hfill q_2(x)\hfill \end{array}\right),A_1=\left(\begin{array}{cc}\hfill 0\hfill & \hfill 0\hfill \\ \hfill a_1^{-1}\hfill & \hfill 1\hfill \end{array}\right),B_1=\left(\begin{array}{cc}\hfill a_1\hfill & \hfill -1\hfill \\ \hfill a_2\hfill & \hfill 0\hfill \end{array}\right).\end{array}$$Let ${\mathit{\phi }}_i(x,\mathit{\lambda })$ denote the solutions of $y^{\prime \prime }+({\mathit{\lambda }}^2-q_i(x))y=0,0<x<1/2$ with ${\mathit{\phi }}_i(0,\mathit{\lambda })=1$ and ${\mathit{\phi }}_i^{\prime }(0,\mathit{\lambda })=h_i$ for $i=1,2$ and$$Y(x,\mathit{\lambda })=\left(\begin{array}{cc}\hfill {\mathit{\phi }}_1(x,\mathit{\lambda })\hfill & \hfill 0\hfill \\ \hfill 0\hfill & \hfill {\mathit{\phi }}_2(x,\mathit{\lambda })\hfill \end{array}\right).$$Then, the characteristic function $\mathrm{\Delta }(\mathit{\lambda })$ for the problem $B$ is(5) $$\begin{array}{cc}\hfill & \mathrm{\Delta }(\mathit{\lambda })\hfill \\ \hfill & =\text{det}[A_1{Y}^{\prime }(1/2,\mathit{\lambda })+B_{1}Y(1/2,\mathit{\lambda })]\hfill \\ \hfill & =\left|\begin{array}{cc}\hfill a_1{\mathit{\phi }}_1(1/2,\mathit{\lambda })\hfill & \hfill -{\mathit{\phi }}_2(1/2,\mathit{\lambda })\hfill \\ \hfill a_1^{-1}{\mathit{\phi }}_1^{\prime }(1/2,\mathit{\lambda })\hfill & \hfill a_2{\mathit{\phi }}_2(1/2,\mathit{\lambda })+{\mathit{\phi }}_2^{\prime }(1/2,\mathit{\lambda })\hfill \end{array}\right|\hfill \\ \hfill & ={\mathit{\phi }}_1(1/2,\mathit{\lambda })[a_2{\mathit{\phi }}_2(1/2,\mathit{\lambda })+a_1{\mathit{\phi }}_2^{\prime }(1/2,\mathit{\lambda })]+a_1^{-1}{\mathit{\phi }}_1^{\prime }(1/2,\mathit{\lambda }){\mathit{\phi }}_2(1/2,\mathit{\lambda }).\hfill \end{array}$$(5) From asymptotic expressions of solutions in [7] and [9], it yields$${\mathit{\phi }}_i(x,\mathit{\lambda })=\cos (\mathit{\lambda }x)+{\mathit{\omega }}_{i,1}(x)\frac{\sin (\mathit{\lambda }x)}{\mathit{\lambda }}+{\mathit{\omega }}_{i,2}(x)\frac{\cos (\mathit{\lambda }x)}{{\mathit{\lambda }}^2}+o\left(\frac{e^{\mathit{\tau }x}}{{\mathit{\lambda }}^2}\right)$$and$${\mathit{\phi }}_i^{\prime }(x,\mathit{\lambda })=-\mathit{\lambda }\sin (\mathit{\lambda }x)+{\mathit{\omega }}_{i,1}(x)\cos (\mathit{\lambda }x)+{\mathit{\omega }}_{i,3}(x)\frac{\sin (\mathit{\lambda }x)}{\mathit{\lambda }}+o\left(\frac{e^{\mathit{\tau }x}}{\mathit{\lambda }}\right),$$where $\mathit{\tau }=\mathfrak{I}\mathit{\lambda }$, and ${\mathit{\omega }}_{i,1}(x)=h_i+\frac{1}{2}{\int }_0^x{q}_i(t)\mathit{dt},$$${\mathit{\omega }}_{i,2}(x)=\frac{q_i(x)-q_i(0)}{4}-\frac{1}{8}{({\int }_0^x{q}_i(t)\mathit{dt})}^2-\frac{h_i}{2}{\int }_0^x{q}_i(t)\mathit{dt}$$and$${\mathit{\omega }}_{i,3}(x)=\frac{q_i(x)+q_i(0)}{4}+\frac{1}{8}{({\int }_0^x{q}_i(t)\mathit{dt})}^2+\frac{h_i}{2}{\int }_0^x{q}_i(t)\mathit{dt}.$$Substituting the expressions ${\mathit{\phi }}_i(x,\mathit{\lambda })$ and ${\mathit{\phi }}_i^{\prime }(x,\mathit{\lambda })$ into (5(5) $$\begin{array}{cc}\hfill & \mathrm{\Delta }(\mathit{\lambda })\hfill \\ \hfill & =\text{det}[A_1{Y}^{\prime }(1/2,\mathit{\lambda })+B_{1}Y(1/2,\mathit{\lambda })]\hfill \\ \hfill & =\left|\begin{array}{cc}\hfill a_1{\mathit{\phi }}_1(1/2,\mathit{\lambda })\hfill & \hfill -{\mathit{\phi }}_2(1/2,\mathit{\lambda })\hfill \\ \hfill a_1^{-1}{\mathit{\phi }}_1^{\prime }(1/2,\mathit{\lambda })\hfill & \hfill a_2{\mathit{\phi }}_2(1/2,\mathit{\lambda })+{\mathit{\phi }}_2^{\prime }(1/2,\mathit{\lambda })\hfill \end{array}\right|\hfill \\ \hfill & ={\mathit{\phi }}_1(1/2,\mathit{\lambda })[a_2{\mathit{\phi }}_2(1/2,\mathit{\lambda })+a_1{\mathit{\phi }}_2^{\prime }(1/2,\mathit{\lambda })]+a_1^{-1}{\mathit{\phi }}_1^{\prime }(1/2,\mathit{\lambda }){\mathit{\phi }}_2(1/2,\mathit{\lambda }).\hfill \end{array}$$(5) ), we obtain(6) $$\begin{array}{cc}\hfill ▵(\mathit{\lambda })& ={\mathit{\phi }}_1(\frac{1}{2},\mathit{\lambda })[a_2{\mathit{\phi }}_2(\frac{1}{2},\mathit{\lambda })+a_1{\mathit{\phi }}_2^{\prime }(\frac{1}{2},\mathit{\lambda })]+a_1^{-1}{\mathit{\phi }}_1^{\prime }(\frac{1}{2},\mathit{\lambda }){\mathit{\phi }}_2(\frac{1}{2},\mathit{\lambda })\hfill \\ \hfill \\ \hfill & =a_2[\cos \frac{\mathit{\lambda }}{2}+{\mathit{\omega }}_{1,1}\left(\frac{1}{2}\right)\frac{\sin \frac{\mathit{\lambda }}{2}}{\mathit{\lambda }}+{\mathit{\omega }}_{1,2}\left(\frac{1}{2}\right)\frac{\cos \frac{\mathit{\lambda }}{2}}{{\mathit{\lambda }}^2}+o\left(\frac{e^{\frac{\mathit{\tau }}{2}}}{{\mathit{\lambda }}^2}\right)]\hfill \\ \hfill \\ \hfill & \times [\cos \frac{\mathit{\lambda }}{2}+{\mathit{\omega }}_{2,1}\left(\frac{1}{2}\right)\frac{\sin \frac{\mathit{\lambda }}{2}}{\mathit{\lambda }}+{\mathit{\omega }}_{2,2}\left(\frac{1}{2}\right)\frac{\cos \frac{\mathit{\lambda }}{2}}{{\mathit{\lambda }}^2}+o\left(\frac{e^{\frac{\mathit{\tau }}{2}}}{{\mathit{\lambda }}^2}\right)]\hfill \\ \hfill \\ \hfill & +a_1[\cos \frac{\mathit{\lambda }}{2}+{\mathit{\omega }}_{1,1}\left(\frac{1}{2}\right)\frac{\sin \frac{\mathit{\lambda }}{2}}{\mathit{\lambda }}+{\mathit{\omega }}_{1,2}\left(\frac{1}{2}\right)\frac{\cos \frac{\mathit{\lambda }}{2}}{{\mathit{\lambda }}^2}+o\left(\frac{e^{\frac{\mathit{\tau }}{2}}}{{\mathit{\lambda }}^2}\right)]\hfill \\ \hfill \\ \hfill & \times [-\mathit{\lambda }\sin \frac{\mathit{\lambda }}{2}+{\mathit{\omega }}_{2,1}\left(\frac{1}{2}\right)\cos \frac{\mathit{\lambda }}{2}+{\mathit{\omega }}_{2,3}\left(\frac{1}{2}\right)\frac{\sin \frac{\mathit{\lambda }}{2}}{\mathit{\lambda }}+o\left(\frac{e^{\frac{\mathit{\tau }}{2}}}{\mathit{\lambda }}\right)]\hfill \\ \hfill \\ \hfill & +a_1^{-1}[-\mathit{\lambda }\sin \frac{\mathit{\lambda }}{2}+{\mathit{\omega }}_{1,1}\left(\frac{1}{2}\right)\cos \frac{\mathit{\lambda }}{2}+{\mathit{\omega }}_{1,3}\left(\frac{1}{2}\right)\frac{\sin \frac{\mathit{\lambda }}{2}}{\mathit{\lambda }}+o\left(\frac{e^{\frac{\mathit{\tau }}{2}}}{\mathit{\lambda }}\right)]\hfill \\ \hfill \\ \hfill & \times [\cos \frac{\mathit{\lambda }}{2}+{\mathit{\omega }}_{2,1}\left(\frac{1}{2}\right)\frac{\sin \frac{\mathit{\lambda }}{2}}{\mathit{\lambda }}+{\mathit{\omega }}_{2,2}\left(\frac{1}{2}\right)\frac{\cos \frac{\mathit{\lambda }}{2}}{{\mathit{\lambda }}^2}+o\left(\frac{e^{\frac{\mathit{\tau }}{2}}}{{\mathit{\lambda }}^2}\right)]\hfill \\ \hfill \\ \hfill & =-\frac{a_1+a_1^{-1}}{2}\mathit{\lambda }\sin \mathit{\lambda }+\frac{1}{2}[a_2+(a_1-a_1^{-1})(\frac{1}{2}{\int }_{\frac{1}{2}}^{1}q(t)\mathit{dt}+h_2\hfill \\ \hfill \\ \hfill & -\frac{1}{2}{\int }_0^{\frac{1}{2}}q(t)\mathit{dt}-h_1\left)\right]+[a_2+(a_1+a_1^{-1})(\frac{1}{2}{\int }_0^{1}q(t)\mathit{dt}+h_1+h_2\left)\right]\hfill \\ \hfill & \times \frac{\cos \mathit{\lambda }}{2}+\left[a_2\right(\frac{1}{2}{\int }_0^{1}q(t)\mathit{dt}+h_1+h_2)+(a_1+a_1^{-1})(\frac{q(0)+q(1)}{4}\hfill \\ \hfill & +\frac{1}{8}{({\int }_0^{1}q(t)\mathit{dt})}^2+\frac{h_1+h_2}{2}{\int }_0^{1}q(t)\mathit{dt}+h_1{h}_2\left)\right]\frac{\sin \mathit{\lambda }}{2\mathit{\lambda }}+o\left(\frac{e^{\mathit{\tau }}}{\mathit{\lambda }}\right).\hfill \end{array}$$(6) Step 2    Asymptotics of spectrum of the problem B

Define$$\begin{array}{c}\hfill {▵}_0(\mathit{\lambda })=-\frac{a_1+a_1^{-1}}{2}\mathit{\lambda }\sin \mathit{\lambda },\end{array}$$and denote by ${\mathit{\mu }}_n,n\in A$, zeros of the function ${▵}_0(\mathit{\lambda })$, then$$\begin{array}{c}\hfill {\mathit{\mu }}_n=n\mathit{\pi },n\in A=\{\pm 0,\pm 1,\pm 2,\cdots \}.\end{array}$$Define$$A_{N_0}=\{\pm 0,\pm 1,\cdots ,\pm N_0\}.$$Denote by $C_n$, the circle of radius $\mathit{\epsilon },0<\mathit{\epsilon }<\frac{1}{2}$, centered at the origin ${\mathit{\mu }}_n$, $n\in A$ and let ${\mathrm{\Gamma }}_{N_0}$ 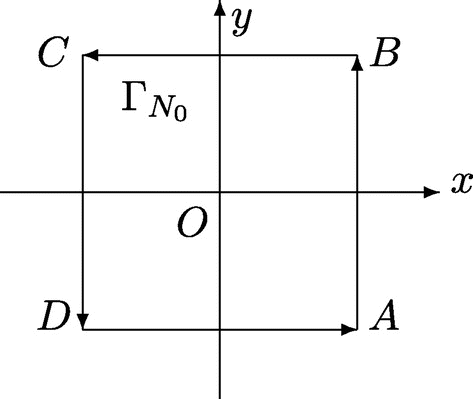be the counterclockwise square contour $\mathit{ABCD}$ as in Figure 1, integer $N_0=0,1,2,\cdots \to \infty$, with$$\begin{array}{cc}\hfill A& =(N_0+\frac{1}{2})\mathit{\pi }(1-i),B=(N_0+\frac{1}{2})\mathit{\pi }(1+i),\hfill \end{array}$$$$\begin{array}{cc}\hfill C& =(N_0+\frac{1}{2})\mathit{\pi }(-1+i),D=(N_0+\frac{1}{2})\mathit{\pi }(-1-i).\hfill \end{array}$$

Fig. 1 contour ${\mathrm{\Gamma }}_{N_0}$ in $\mathit{\lambda }$-complex plane.

To obtain trace formulas, we need the following lemma.

Lemma 2.4

For $N_0$ large enough, on the contour ${\mathrm{\Gamma }}_{N_0}$, there holds(7) $$\begin{array}{c}\hfill \left|\frac{\exp (|\mathfrak{I}\mathit{\lambda }|)}{\sin \mathit{\lambda }}\right|\le 4.\end{array}$$(7)

Proof

For $\mathit{\lambda }$ on the side $\mathit{AB}$, let $\mathit{\lambda }=(N_0+\frac{1}{2})\mathit{\pi }+i\mathit{\tau },-(N_0+\frac{1}{2})\mathit{\pi }\le \mathit{\tau }\le (N_0+\frac{1}{2})\mathit{\pi }$. We have,$$\begin{array}{c}\hfill \begin{array}{cc}\hfill \left|\frac{\exp (|\mathfrak{I}\mathit{\lambda }|)}{\sin \mathit{\lambda }}\right|& =\left|\frac{\exp (|\mathfrak{I}\mathit{\lambda }|)}{\sin ((N_0+\frac{1}{2})\mathit{\pi }+i\mathit{\tau })}\right|=\left|\frac{2\exp (|\mathfrak{I}\mathit{\lambda }|)}{e^{-\mathit{\tau }+i(N_0+\frac{1}{2})\mathit{\pi }}-e^{\mathit{\tau }-i(N_0+\frac{1}{2})\mathit{\pi }}}\right|\hfill \\ \hfill & \le \frac{2\exp (|\mathfrak{I}\mathit{\lambda }|)}{\exp (|\mathfrak{I}\mathit{\lambda }|)+\exp (-|\mathfrak{I}\mathit{\lambda }|)}\le 2<4.\hfill \end{array}\end{array}$$As $\mathit{\lambda }$ locates on the side $\mathit{BC}$, let $\mathit{\lambda }=\mathit{\sigma }+i(N_0+\frac{1}{2})\mathit{\pi },-(N_0+\frac{1}{2})\mathit{\pi }\le \mathit{\sigma }\le (N_0+\frac{1}{2})\mathit{\pi }$. We have,$$\begin{array}{c}\hfill \begin{array}{cc}\hfill \left|\frac{\exp (|\mathfrak{I}\mathit{\lambda }|)}{\sin \mathit{\lambda }}\right|& =\left|\frac{\exp (|\mathfrak{I}\mathit{\lambda }|)}{\sin (\mathit{\sigma }+i(N_0+\frac{1}{2})\mathit{\pi })}\right|=\left|\frac{2\exp (|\mathfrak{I}\mathit{\lambda }|)}{e^{-(N_0+\frac{1}{2})\mathit{\pi }+i\mathit{\sigma }}-e^{(N_0+\frac{1}{2})\mathit{\pi }-i\mathit{\sigma }}}\right|\hfill \\ \hfill & \le \frac{2\exp (|\mathfrak{I}\mathit{\lambda }|)}{e^{(N_0+\frac{1}{2})\mathit{\pi }}-e^{-(N_0+\frac{1}{2})\mathit{\pi }}}=\frac{2}{1-e^{-(2N_0+1)\mathit{\pi }}}\le 4.\hfill \end{array}\end{array}$$The last inequality follows from the fact that ${\lim }_{N_0\to \infty }{e}^{-(2N_0+1)\mathit{\pi }}=0$, and for $N_0$ large enough there holds $e^{-(2N_0+1)\mathit{\pi }}<\frac{1}{2}$.

For $\mathit{\lambda }$ on the side $\mathit{CD}$ and the side $\mathit{DA}$, the same conclusions are true. Thus, for $N_0$ that is large enough, on the contour ${\mathrm{\Gamma }}_{N_0}$, we obtain $\left|\frac{\exp (|\mathfrak{I}\mathit{\lambda }|)}{\sin \mathit{\lambda }}\right|\le 4$.

Using Lemma 2.4, combining (6(6) $$\begin{array}{cc}\hfill ▵(\mathit{\lambda })& ={\mathit{\phi }}_1(\frac{1}{2},\mathit{\lambda })[a_2{\mathit{\phi }}_2(\frac{1}{2},\mathit{\lambda })+a_1{\mathit{\phi }}_2^{\prime }(\frac{1}{2},\mathit{\lambda })]+a_1^{-1}{\mathit{\phi }}_1^{\prime }(\frac{1}{2},\mathit{\lambda }){\mathit{\phi }}_2(\frac{1}{2},\mathit{\lambda })\hfill \\ \hfill \\ \hfill & =a_2[\cos \frac{\mathit{\lambda }}{2}+{\mathit{\omega }}_{1,1}\left(\frac{1}{2}\right)\frac{\sin \frac{\mathit{\lambda }}{2}}{\mathit{\lambda }}+{\mathit{\omega }}_{1,2}\left(\frac{1}{2}\right)\frac{\cos \frac{\mathit{\lambda }}{2}}{{\mathit{\lambda }}^2}+o\left(\frac{e^{\frac{\mathit{\tau }}{2}}}{{\mathit{\lambda }}^2}\right)]\hfill \\ \hfill \\ \hfill & \times [\cos \frac{\mathit{\lambda }}{2}+{\mathit{\omega }}_{2,1}\left(\frac{1}{2}\right)\frac{\sin \frac{\mathit{\lambda }}{2}}{\mathit{\lambda }}+{\mathit{\omega }}_{2,2}\left(\frac{1}{2}\right)\frac{\cos \frac{\mathit{\lambda }}{2}}{{\mathit{\lambda }}^2}+o\left(\frac{e^{\frac{\mathit{\tau }}{2}}}{{\mathit{\lambda }}^2}\right)]\hfill \\ \hfill \\ \hfill & +a_1[\cos \frac{\mathit{\lambda }}{2}+{\mathit{\omega }}_{1,1}\left(\frac{1}{2}\right)\frac{\sin \frac{\mathit{\lambda }}{2}}{\mathit{\lambda }}+{\mathit{\omega }}_{1,2}\left(\frac{1}{2}\right)\frac{\cos \frac{\mathit{\lambda }}{2}}{{\mathit{\lambda }}^2}+o\left(\frac{e^{\frac{\mathit{\tau }}{2}}}{{\mathit{\lambda }}^2}\right)]\hfill \\ \hfill \\ \hfill & \times [-\mathit{\lambda }\sin \frac{\mathit{\lambda }}{2}+{\mathit{\omega }}_{2,1}\left(\frac{1}{2}\right)\cos \frac{\mathit{\lambda }}{2}+{\mathit{\omega }}_{2,3}\left(\frac{1}{2}\right)\frac{\sin \frac{\mathit{\lambda }}{2}}{\mathit{\lambda }}+o\left(\frac{e^{\frac{\mathit{\tau }}{2}}}{\mathit{\lambda }}\right)]\hfill \\ \hfill \\ \hfill & +a_1^{-1}[-\mathit{\lambda }\sin \frac{\mathit{\lambda }}{2}+{\mathit{\omega }}_{1,1}\left(\frac{1}{2}\right)\cos \frac{\mathit{\lambda }}{2}+{\mathit{\omega }}_{1,3}\left(\frac{1}{2}\right)\frac{\sin \frac{\mathit{\lambda }}{2}}{\mathit{\lambda }}+o\left(\frac{e^{\frac{\mathit{\tau }}{2}}}{\mathit{\lambda }}\right)]\hfill \\ \hfill \\ \hfill & \times [\cos \frac{\mathit{\lambda }}{2}+{\mathit{\omega }}_{2,1}\left(\frac{1}{2}\right)\frac{\sin \frac{\mathit{\lambda }}{2}}{\mathit{\lambda }}+{\mathit{\omega }}_{2,2}\left(\frac{1}{2}\right)\frac{\cos \frac{\mathit{\lambda }}{2}}{{\mathit{\lambda }}^2}+o\left(\frac{e^{\frac{\mathit{\tau }}{2}}}{{\mathit{\lambda }}^2}\right)]\hfill \\ \hfill \\ \hfill & =-\frac{a_1+a_1^{-1}}{2}\mathit{\lambda }\sin \mathit{\lambda }+\frac{1}{2}[a_2+(a_1-a_1^{-1})(\frac{1}{2}{\int }_{\frac{1}{2}}^{1}q(t)\mathit{dt}+h_2\hfill \\ \hfill \\ \hfill & -\frac{1}{2}{\int }_0^{\frac{1}{2}}q(t)\mathit{dt}-h_1\left)\right]+[a_2+(a_1+a_1^{-1})(\frac{1}{2}{\int }_0^{1}q(t)\mathit{dt}+h_1+h_2\left)\right]\hfill \\ \hfill & \times \frac{\cos \mathit{\lambda }}{2}+\left[a_2\right(\frac{1}{2}{\int }_0^{1}q(t)\mathit{dt}+h_1+h_2)+(a_1+a_1^{-1})(\frac{q(0)+q(1)}{4}\hfill \\ \hfill & +\frac{1}{8}{({\int }_0^{1}q(t)\mathit{dt})}^2+\frac{h_1+h_2}{2}{\int }_0^{1}q(t)\mathit{dt}+h_1{h}_2\left)\right]\frac{\sin \mathit{\lambda }}{2\mathit{\lambda }}+o\left(\frac{e^{\mathit{\tau }}}{\mathit{\lambda }}\right).\hfill \end{array}$$(6) ) and arranging the terms on the right-hand side in decreasing order of powers of $\mathit{\lambda }$ gives for the contour ${\mathrm{\Gamma }}_{N_0}$(8) $$\begin{array}{c}\hfill \frac{▵(\mathit{\lambda })}{{▵}_0(\mathit{\lambda })}=1-\frac{a}{\mathit{\lambda }\sin \mathit{\lambda }}-\frac{b}{\mathit{\lambda }}\cot \mathit{\lambda }-\frac{c}{{\mathit{\lambda }}^2}+o\left(\frac{1}{{\mathit{\lambda }}^2}\right),\end{array}$$(8) where$$\begin{array}{cc}\hfill c& =\frac{a_2}{a_1+a_1^{-1}}(\frac{1}{2}{\int }_0^{1}q(t)\mathit{dt}+h_1+h_2)+\frac{q(0)+q(1)}{4}\hfill \\ \hfill & +\frac{1}{8}{({\int }_0^{1}q(t)\mathit{dt})}^2+\frac{h_1+h_2}{2}{\int }_0^{1}q(t)\mathit{dt}+h_1{h}_2.\hfill \end{array}$$Expanding $\ln \frac{▵(\mathit{\lambda })}{{▵}_0(\mathit{\lambda })}$ by the Maclaurin formula, we find that on ${\mathrm{\Gamma }}_{N_0}$$$\begin{array}{c}\hfill \ln \frac{▵(\mathit{\lambda })}{{▵}_0(\mathit{\lambda })}=-\frac{a}{\mathit{\lambda }\sin \mathit{\lambda }}-\frac{b}{\mathit{\lambda }}\cot \mathit{\lambda }-\frac{c}{{\mathit{\lambda }}^2}-\frac{a^2}{2{\mathit{\lambda }}^2{\sin }^2\mathit{\lambda }}-\frac{b^2{\cot }^2\mathit{\lambda }}{2{\mathit{\lambda }}^2}-\frac{\mathit{ab}\cos \mathit{\lambda }}{{\mathit{\lambda }}^2{\sin }^2\mathit{\lambda }}+o\left(\frac{1}{{\mathit{\lambda }}^2}\right).\end{array}$$Using the residue theorem we have$$\begin{array}{cc}\hfill & {\mathit{\lambda }}_n-{\mathit{\mu }}_n\hfill \\ \hfill \\ \hfill & =-\frac{1}{2\mathit{\pi }i}{\oint }_{C_n}\ln \frac{▵(\mathit{\lambda })}{{▵}_0(\mathit{\lambda })}d\mathit{\lambda }\hfill \\ \hfill \\ \hfill & =\frac{1}{2\mathit{\pi }i}{\oint }_{C_n}[\frac{a}{\mathit{\lambda }\sin \mathit{\lambda }}+\frac{b}{\mathit{\lambda }}\cot \mathit{\lambda }+\frac{c}{{\mathit{\lambda }}^2}+\frac{a^2}{2{\mathit{\lambda }}^2{\sin }^2\mathit{\lambda }}+\frac{b^2}{2{\mathit{\lambda }}^2}{\cot }^2\mathit{\lambda }\hfill \\ \hfill \\ \hfill & +\frac{\mathit{ab}}{{\mathit{\lambda }}^2{\sin }^2\mathit{\lambda }}\cos \mathit{\lambda }+o\left(\frac{1}{{\mathit{\lambda }}^2}\right)]d\mathit{\lambda }\hfill \\ \hfill \\ \hfill & =\frac{1}{2\mathit{\pi }i}{\oint }_{C_n}[\frac{a}{\mathit{\lambda }\sin \mathit{\lambda }}+\frac{b}{\mathit{\lambda }}\cot \mathit{\lambda }]d\mathit{\lambda }+O\left(\frac{1}{n^2}\right)\hfill \\ \hfill \\ \hfill & =\frac{{(-1)}^{n}a+b}{n\mathit{\pi }}+O\left(\frac{1}{n^2}\right),\hfill \end{array}$$thus,$${\mathit{\lambda }}_n=n\mathit{\pi }+\frac{{(-1)}^{n}a+b}{n\mathit{\pi }}+O\left(\frac{1}{n^2}\right).$$

Step 3    Trace of the problem B

Asymptotic formula of ${\mathit{\lambda }}_n$ implies that for all sufficiently large $N_0$, the numbers ${\mathit{\lambda }}_n$ which are the zeros of the function $▵(\mathit{\lambda })$, with $n\le N_0$, are inside ${\mathrm{\Gamma }}_{N_0}$ and the number ${\mathit{\lambda }}_n$, with $n>N_0$, are outside ${\mathrm{\Gamma }}_{N_0}$.

Obviously, ${\mathit{\mu }}_n=n\mathit{\pi }$, which are the zeros of the function ${▵}_0(\mathit{\lambda })$, do not lie on the contour ${\mathrm{\Gamma }}_{N_0}$.

It follows that(9) $$\begin{array}{cc}\hfill & \sum _{n\in A_{N_0}}({\mathit{\lambda }}_n^2-{\mathit{\mu }}_n^2)\hfill \\ \hfill & =-\frac{1}{2\mathit{\pi }i}{\oint }_{{\mathrm{\Gamma }}_{N_0}}2\mathit{\lambda }\ln \frac{▵(\mathit{\lambda })}{{▵}_0(\mathit{\lambda })}d\mathit{\lambda }\hfill \\ \hfill \\ \hfill & =\frac{1}{2\mathit{\pi }i}{\oint }_{{\mathrm{\Gamma }}_{N_0}}[\frac{2a}{\sin \mathit{\lambda }}+2b\cot \mathit{\lambda }+\frac{2c}{\mathit{\lambda }}+\frac{a^2}{\mathit{\lambda }{\sin }^2\mathit{\lambda }}+\frac{b^2}{\mathit{\lambda }}{\cot }^2\mathit{\lambda }\hfill \\ \hfill & +\frac{2\mathit{ab}}{\mathit{\lambda }{\sin }^2\mathit{\lambda }}\cos \mathit{\lambda }+o\left(\frac{1}{\mathit{\lambda }}\right)]d\mathit{\lambda }.\hfill \end{array}$$(9) Standard residue calculations show that$$\begin{array}{c}\hfill \frac{1}{2\mathit{\pi }i}{\oint }_{{\mathrm{\Gamma }}_{N_0}}\frac{1}{\sin \mathit{\lambda }}d\mathit{\lambda }=1+\sum _{n\in A_{N_0}}{(-1)}^n,\frac{1}{2\mathit{\pi }i}{\oint }_{{\mathrm{\Gamma }}_{N_0}}\cot \mathit{\lambda }d\mathit{\lambda }=2N_0+1,\\ \hfill \frac{1}{2\mathit{\pi }i}{\oint }_{{\mathrm{\Gamma }}_{N_0}}\frac{1}{\mathit{\lambda }{\sin }^2\mathit{\lambda }}d\mathit{\lambda }=O\left(\frac{1}{N_0}\right),\frac{1}{2\mathit{\pi }i}{\oint }_{{\mathrm{\Gamma }}_{N_0}}\frac{{\cot }^2\mathit{\lambda }}{\mathit{\lambda }}d\mathit{\lambda }=-1+O\left(\frac{1}{N_0}\right),\end{array}$$and$$\begin{array}{cc}\hfill \frac{1}{2\mathit{\pi }i}{\oint }_{{\mathrm{\Gamma }}_{N_0}}\frac{\cos \mathit{\lambda }}{\mathit{\lambda }{\sin }^2\mathit{\lambda }}d\mathit{\lambda }& =-\frac{1}{2\mathit{\pi }i}{\oint }_{{\mathrm{\Gamma }}_{N_0}}\frac{1}{\mathit{\lambda }}d\left(\frac{1}{\sin \mathit{\lambda }}\right)\hfill \\ \hfill & =-\frac{1}{2\mathit{\pi }i}{\oint }_{{\mathrm{\Gamma }}_{N_0}}\frac{1}{{\mathit{\lambda }}^2\sin \mathit{\lambda }}d\mathit{\lambda }=O\left(\frac{1}{N_0}\right).\hfill \end{array}$$Substituting these expressions into (9(9) $$\begin{array}{cc}\hfill & \sum _{n\in A_{N_0}}({\mathit{\lambda }}_n^2-{\mathit{\mu }}_n^2)\hfill \\ \hfill & =-\frac{1}{2\mathit{\pi }i}{\oint }_{{\mathrm{\Gamma }}_{N_0}}2\mathit{\lambda }\ln \frac{▵(\mathit{\lambda })}{{▵}_0(\mathit{\lambda })}d\mathit{\lambda }\hfill \\ \hfill \\ \hfill & =\frac{1}{2\mathit{\pi }i}{\oint }_{{\mathrm{\Gamma }}_{N_0}}[\frac{2a}{\sin \mathit{\lambda }}+2b\cot \mathit{\lambda }+\frac{2c}{\mathit{\lambda }}+\frac{a^2}{\mathit{\lambda }{\sin }^2\mathit{\lambda }}+\frac{b^2}{\mathit{\lambda }}{\cot }^2\mathit{\lambda }\hfill \\ \hfill & +\frac{2\mathit{ab}}{\mathit{\lambda }{\sin }^2\mathit{\lambda }}\cos \mathit{\lambda }+o\left(\frac{1}{\mathit{\lambda }}\right)]d\mathit{\lambda }.\hfill \end{array}$$(9) ) we have$$\begin{array}{cc}\hfill & \sum _{n\in A_{N_0}}({\mathit{\lambda }}_n^2-{\mathit{\mu }}_n^2)\hfill \\ \hfill & =2a\sum _{n\in A_{N_0}}{(-1)}^n-2a+2b(2N_0+1)+2c-b^2+o(1)\hfill \\ \hfill & =2a\sum _{n\in A_{N_0}}{(-1)}^n+2b\sum _{n\in A_{N_0}}1-2a-2b+2c-b^2+o(1),\hfill \end{array}$$which implies that$$\sum _{n\in A_{N_0}}[{\mathit{\lambda }}_n^2-{\mathit{\mu }}_n^2-{(-1)}^{n}2a-2b]=-2a-2b+2c-b^2+o(1).$$Passing to the limit as $N_0\to \infty$ in the above equation, we find that$$\sum _{n\in A}[{\mathit{\lambda }}_n^2-{\mathit{\mu }}_n^2-{(-1)}^{n}2a-2b]=-2a-2b+2c-b^2,$$where, by a direct calculation,$$\begin{array}{cc}\hfill & -2a-2b+2c-b^2\hfill \\ \hfill & =-\frac{4a_2}{a_1+a_1^{-1}}-\frac{a_1-a_1^{-1}}{a_1+a_1^{-1}}({\int }_{\frac{1}{2}}^{1}q(t)\mathit{dt}+2h_2-{\int }_0^{\frac{1}{2}}q(t)\mathit{dt}-2h_1)\hfill \end{array}$$$$\begin{array}{cc}\hfill & -\frac{a_2^2}{{(a_1+a_1^{-1})}^2}+\frac{q(0)+q(1)}{2}-{\int }_0^{1}q(t)\mathit{dt}-2(h_1+h_2)-(h_1^2+h_2^2).\hfill \end{array}$$Then, the proof of the theorem is finished.

Acknowledgments

The author acknowledges helpful comments from the referees. The author is indebted to Professor G. Dulikravich for reading this paper. The author is very grateful to Professor V. N. Pivovarchik and Professor V. A. Yurko for many useful discussions related to some topics of spectral analysis of differential operators. This work was supported by the National Natural Science Foundation of China (11171152), Natural Science Foundation of Jiangsu Province of China (BK 2010489) and the Outstanding Plan-Zijin Star Foundation of NUST (AB 41366).