Valid and valuable: lower attaining pupils’ contributions to mixed attainment mathematics in primary schools

ABSTRACT

Existing research establishes that lower attaining pupils derive mathematical learning benefit from working in mixed attainment groupings. However, gains for lower attaining pupils are seen to derive from the contributions of higher attaining peers; evidence of the contributions that lower attaining pupils make to mixed attainment activity is currently lacking. This study contributes evidence of the merits of mixed attainment working through its focus on the mathematical contributions of lower attaining primary pupils to mixed attainment pair activity. It draws on the construct of mathematical noticing and focuses on the development of pupils’ noticing of mathematical pattern, structure and property. Close video analysis of pupils’ speech and action during paired activity establishes that primary school mixed attainment working can produce bi-directional benefits, with lower attaining pupils making important contributions to task progress and contributing mathematical insights in advance of and beyond those of their higher attaining partners.

KEYWORDS:

• Lower attaining pupils
• mixed attainment
• mathematical noticing
• primary education

Introduction

This paper analyses mathematical contributions made by lower attaining (LA) primary school pupils, and the impact of these contributions on task progress for the mixed attainment pairs in which they worked. It presents data from one mathematical task taught as a single lesson across three primary school classrooms; this lesson was part of, and data presented representative of, a wider study focusing on the mathematical activity and interactions of LA pupils and their higher attaining (HA) partners.

Despite numerous cautions regarding its negative impact, the use of either within-class or between-class arrangements that group primary pupils with peers of similar prior attainment predominate in English primary school mathematics lessons (Bradbury, 2019). Cautions centre on the conflation of measures of current attainment with notions of predetermined ability, exemplified in the nomenclature of “ability” groups and its contingent practices (Marks, 2013). These practices, frequently purporting to be neutral though drawing considerably on subjective judgement (McGillicuddy & Devine, 2018) result in disproportionate assignment of minority ethnic and lower socio-economic status pupils to lower groups (Archer et al., 2018), an inequity compounded by pedagogical approaches which see lower group pupils offered a restricted mathematical diet characterised by high levels of repetition and few opportunities for developing independence (McGillicuddy & Devine, 2018). Negative impact on self-confidence and learner identity arising from lower group allocation is well documented (Francis et al., 2020; Hargreaves, Quick, & Buchanan, 2021; McGillicuddy & Devine, 2020); moreover and perhaps most concerning is that pupils, including those most disadvantaged by ability grouping, rarely challenge its legitimacy, coming to see group allocation as deserved and reflective of natural ability (Archer et al., 2018).

Given the above social justice concerns, inevitable questions arise regarding the continued pervasiveness of ability grouping, and the merits of alternative arrangements. In relation to the former, barriers to changing existing practice remain significant (Taylor et al., 2017). Amongst these barriers, researchers identify low teacher expectation of those in lower groups to engage mathematically (Anthony & Hunter, 2017; Marks, 2014; McGillicuddy & Devine, 2018); the pressure of accountability regimes (Alderton & Gifford, 2018; Bradbury, 2019; McGillicuddy & Devine, 2018) and the need for practice change to be supported by significant professional development (Anthony & Hunter, 2017; Bradbury, 2019). Reluctance to abandon existing ability grouping also reflects concerns expressed by teachers (McGillicuddy & Devine, 2018) and pupils (Esmonde, Brodie, Dookie, & Takeuchi, 2009) that higher attainers will be “held back” by mixed attainment working. Moreover, as Marks (2013) cautions, change to practice must be accompanied by change to thinking; removal of ability grouping structures does not immediately change pre-existing expectations of performance or beliefs about pre-determined ability.

The current paucity of research into the merits of mixed attainment working is part of a vicious cycle identified by Taylor et al. (2017) in which limited use of mixed attainment practice leads to limited research opportunities and thus a restricted evidence base for decision making about its use. Nevertheless, outcomes from a small number of primary phase studies indicate that mixed attainment groupings have a positive impact on mathematics learning for previously LA pupils, importantly, without negative impact on the progress of HA peers. For example, in a US class-based study, Leonard (2001) found that all those working in mixed attainment groups fared better than those working in same attainment groups, with the effect being most pronounced for previously middle and lower attainers. In a non-class-based study, Fawcett and Garton (2005) compared performance on attribute based sorting activities for pupils working in either same attainment or mixed attainment pairs. The largest performance gains were made by LA pupils when collaborating with HA partners; for HA pupils, the only situation in which gains were made was when collaborating with a LA partner, with non-significant performance declines being recorded when HA pupils were paired together.

Further, countering the teacher expectations reported above, classroom-based studies confirm the capacity of LA pupils to independently think and act mathematically. For example, Houssart’s (2004) naturalistic observation in primary settings highlighted a range of spontaneous mathematical contributions by LA pupils, such as the noticing of generality, identification of possible extension to mathematical ideas under discussion and awareness of mathematical inaccuracy voiced by others. Importantly, the naturalistic nature of these observations confirms that such mathematical behaviours are part of how these LA pupils operate independently.

Despite reported gains from mixed attainment working, it is of particular note in the context of this paper that benefits accrued tend to be couched in terms of what the LA pupil gains from working with a more knowledgeable other. Benefits for the LA pupil are, for example, attributed to their exposure to the more sophisticated thinking and reasoning of HA peers (Fawcett & Garton, 2005), or their access to higher quality interaction (Leonard, 2001). Whilst these are not to be disregarded, recent research in a secondary context identifies that LA pupils believe they can also make contributions to mixed groups, that they can provide help to other pupils as well as benefitting from the support of others (Tereshchenko et al., 2019). However, evidence of the specific nature and impact of primary school LA pupils’ contributions, what they “give” as well as “take” from mixed attainment mathematical activity, is currently lacking.

In response and through its specific focus on the nature and impact of LA pupil contributions to mixed pair activity, this paper seeks to provide evidence of whether, and in what ways, LA pupils contribute to task progress in mixed attainment pairs. In particular the paper examines the validity of the assertion that where mixed attainment working is productive, benefits derive from HA pupils’ contributions. The study focuses on the construct of mathematical noticing, employing a pedagogical intervention designed to promote mathematical noticing, in all pupils, of the features and outcomes of their tasks.

Mathematical noticing

In mathematics classrooms, as well as in everyday life, we are often faced with multiple sensory and conceptual stimuli to which we cannot simultaneously attend; Gattegno (2010, p. 16) argues that we respond to this challenge through a process of “stressing and ignoring”, filtering out some stimuli in order to focus attention on others. Without use of this process, Gattegno asserts that “we can not see anything” (2010, p. 16). Yet this sifting, whether conscious or otherwise, has consequences; what we notice, and equally what we do not notice, is significant. Mason comments that “what you do not notice you cannot act upon” (2002, p. 7) and in relation specifically to classroom mathematical activity, Lobato, Hohensee, and Rhodehamel (2013, p. 844) conclude that “what students notice mathematically has consequences for their subsequent reasoning”.

Lobato et al. (2013) find that the specific nature of teacher pedagogies, such as the implicit or explicit focus of, and vocabulary used in, questions and prompts, as well as the nature of board annotations and gestures, impact on what is noticed at the time, and on pupils’ subsequent noticing behaviour. Importantly, Hohensee (2016) further suggests that pedagogies aimed at promoting noticing appear to have particular potential to support pupils identified as lower attaining. In his study, these pedagogies included focusing pupil attention on key mathematical features through questions and prompts and promoting the examination between pupils of the features of their representational diagrams. The strong gains made by previously LA pupils compared to those of their HA peers in their noticing and use of the mathematical relations on which the study was based, leads to the hypothesis that noticing “may be a mechanism that could be leveraged to help students who are low achieving to catch up with students who are achieving at more proficient levels” (Hohensee, 2016, p. 89). This differential impact on lower attainers may be partly accounted for in Mulligan’s (2011) finding that prior to specific intervention lower attainers were more likely to focus on non-mathematical features of tasks and were less independently predisposed to look for, recognise and productively use underlying mathematical features such as patterns and relationships.

Arising from the above, in this paper, mathematical noticing is viewed as central to the learning process, mediating learners’ activity and influencing their decision making. I define mathematical noticing as “the detection of mathematical features, relations, or processes”, and focus on noticing as an essential precursor to mathematical reasoning. Whilst noticing could be considered to be part of the reasoning process rather than a precursor to it, following Lobato et al. (2013), I separate the perceptual or analytic discernment of features, specifically in this study, mathematical pattern, structure and property, from the use of them in constructing reasoning and position noticing as a necessary but insufficient condition for mathematical reasoning.

The construct of noticing is related to that of awareness; the two ideas are not easy to tease apart. Mason’s separation of the two, “what gets through into awareness (conscious or subconscious) is what we notice, at whatever level” (2002, p. 32), suggests awareness as an enduring state and “what we notice” as the elements of the current environment that have been discriminated or marked out from the range of available stimuli. However, the phrase “become aware” (e.g. Hewitt, 2001, p. 39) suggests that the term awareness might also encompass the moment of perceptual discrimination, or change in understanding. As is the case with awareness, Mason (2002) argues that noticing is not always consciously driven, that our ability to use what we have perceived depends on the level at which it has been noticed. He draws, for example, a distinction between features that we have “barely noticed” and that we are unable to recall independently and those that are “marked” and which we can report on (Mason, 2011, p. 42). In this paper, I build particularly on Mason’s notion of “marking” and use the term noticing to refer to an event, a moment in time when a “movement or shift of attention” (Mason, 2011, p. 45) either intentional or otherwise, enables an individual to detect a mathematical idea or feature.

Analytic framework for mathematical reasoning

For the researcher, attempts to identify what pupils notice are complicated by the partially internal nature of the construct: what a pupil notices is not an observable event and the researcher has access only to the pupil’s response to their noticing through speech, gesture or action. Two key implications arise, first that some of what a pupil notices will remain beyond the reach of the observer, and second, a careful process of inference is required to move from the speech, gesture or action observed to the noticing that stimulated it.

This study focuses on the noticing of features that support the development of mathematical reasoning; in particular, pupils’ noticing of the related ideas of mathematical pattern, structure and property. Mulligan and Mitchelmore (2009, p. 34) define mathematical pattern as a “predictable regularity” and structure as “the way a mathematical pattern is organised”, noting that structure is expressed in the form of a generalisation, a relationship that is consistent within a defined domain. The term “mathematical property” refers to the classes to which a mathematical object belongs. To illustrate, the numbers 9, 15 and 21 share a property in that they all belong to the class of numbers that are multiples of three. Numbers in this class follow a pattern in that there is a difference of three between successive pairs of numbers; they share a structure in that each number in this class can be constructed as a group of threes. It is clear therefore, that while each term has its own definition, the three are interconnected.

The focus on pupils’ noticing of property incorporates both the identification of the properties of individual numbers and the identification that a given set of numbers may have a particular property in common. This latter aspect, the identification of commonality, is significant for the move to generalisation (Towers & Martin, 2014) and for the understanding of structure (Mason, 2003). Mason (2003) connects properties and structure in identifying that structure emerges when a property can be separated from its particular example and considered independently. The focus on structure draws on the emphasis placed on awareness of structure for mathematics achievement identified by Mulligan and Mitchelmore (2009), together with the essential role of generality in mathematical activity (Mason, Burton, & Stacey, 2010).

Research methods

This study focused on the research question: How do LA pupils’ mathematical noticings contribute to task progress in a mixed attainment pupil pair? Characteristic of the Education Design Research approach adopted, the study involved a collaboration between class teachers and a researcher to develop and explore a classroom-based pedagogical intervention through iterative cycles of planning, action and review (Plomp & Nieveen, 2013). Each of the three cycles comprised a workshop meeting between the researcher and class teachers, and a mathematics research lesson during which the intervention was enacted. A preliminary lesson preceded the research cycles and informed the design of the intervention as detailed below.

Workshop meetings served two key functions. First, triangulation of the sole researcher’s interpretations of video sequences in order to evaluate the impact of the intervention. Second, planning of subsequent research lesson activity, including discussion of the use of resources to promote noticing, and lines of teacher questioning. Lesson plans were subsequently drawn up by the researcher and ongoing email served to provide further lesson support as required.

Research lessons were led by the class teacher. The researcher adopted a balanced participation approach (Savin-Baden & Howell Major, 2013); chiefly engaged with video data collection but engaging on occasion both with the teacher to respond to queries and discuss issues regarding lesson progress, and with pupils in response to their questions and in line with the agreed structure of the lesson.

Participants

The study took place in the mixed attainment mathematics classrooms of three primary mathematics specialist teachers in three urban primary schools in the South East of England. Whilst normal grouping practices in the three classrooms varied depending on topic and task, the use of mixed attainment pairs specifically was not a commonly established practice. The use of problem-solving tasks and a focus on reasoning was a feature of all classrooms. Pupils were aged either 8–9 (year 4) or 10–11 (year 6).

All pupils took part in the mathematics lesson activities. Data collection in each class focused on two LA pupils who were operating at below national age-related expectations who were each paired with a HA pupil who was exceeding age-related expectations. Selection of the focus pupils was made by class teachers based on the most recent summative attainment data together with current teacher assessments and ensured a genuine and clear attainment gap between the two pupils in the pair.

Intervention

Analysis of the preliminary lesson revealed limited communication between pupil pairs of, and about, what either pupil had noticed in the course of their search for solutions; potentially valuable insights were generally not built upon in constructing reasoning. The intervention sought to raise the profile of the sharing and discussing of noticing as an important part of mathematical activity and a route to reasoning with and about solutions.

The intervention had four key features and remained consistent throughout the study. First, tasks were selected on the basis of their affordances for mathematical noticing and their potential for collaborative paired activity. All presented an investigative or problem-solving challenge which provided an accessible starting point for all levels of prior attainment whilst affording opportunities for collaboration and participation (Boaler, 2016; Taylor et al., 2017). Second, teachers promoted pupils’ use of manipulatives that represented both the numbers involved in the task and the relationships between them. Such manipulatives have been shown to provide support for articulating relationships (Foote & Lambert, 2011), and noticing generality (Houssart, 2004).

Third, building on Hewitt (2001), specific lines of teacher questioning aimed to maintain focus on, and to value, pupil noticing of task-related mathematical features. Teacher questioning throughout all parts of the lesson consistently addressed what pupils had noticed, what might be the same or different in the outcomes obtained, whether any pattern was emerging, and what they thought their noticing meant. Lastly, pupils’ recording sheets used sentence starters, such as “I’ve noticed that … ” to promote the recording of features noticed.

Data collection

Activity of the focus LA pupils and their HA partners was video recorded during the hour-long lessons. Cameras attached to tall tripods were positioned to capture pupils’ faces together with their verbal and non-verbal interaction and their tabletop activity involving task resources and artefacts. Additional still images captured pupils’ inscriptions on recording sheets.

Data analysis process

Cleaning of data resulted, for the lesson reported here, in data from five focus LA pupils: Mia, Joe, Charley, April and Rose and their respective HA partners Jade, Ash, Morgan, Jacob and Hannah being subjected to detailed analysis (all names are pseudonyms).

Analysis began with the construction of a detailed description of the activity contained within each video recording. This description separated video content into thematic sections, each representing a period in which a phase of activity was underway. Sections were separated by interruption to, or change in, activity. For example, a teacher approaching the table to talk, a mid-lesson plenary, pupils moving from exploration to recording or beginning a new trial, all stimulated the separation of one section from another. Sections were thus not of equal length, ranging from less than one minute to five minutes long, but typically were of two to three minutes duration.

Subsequently, critical events, defined as a sequence of action and speech which demonstrates a change from a pupil’s previous understanding (Powell, Francisco, & Maher, 2003), were identified in each video. In order to establish a starting point, the first critical event of each video was the first thematic section of pair activity in which both pupils demonstrated understanding of task requirements. Examination of the content of speech and focus of activity within this early section enabled the identification of the understandings and ideas that informed their initial response to the task. Beyond this, the selection built on identification of changed or new aspects of the LA pupil’s mathematical activity through the examination of thematic sections containing:

• direct speech which included new reference to mathematical facts, connections, patterns, or properties;

• pupils’ use of manipulatives to represent mathematical ideas or relationships;

• gesture (such as pointing) and inscriptions where these indicated that a pupil had noted a relevant feature.

Transcripts of the speech within each section identified as critical events were made and used alongside repeated viewing of the video data to support examination and interpretation of the features above. Previous and subsequent video activity was then examined in order to trace the development of new ideas or activity and, where interpretation was unclear, to establish corroborative examples.

Findings

In the lesson reported in this paper, pupils worked on a task entitled “What numbers can we make?” (Nrich, 2015). The task required pupils to select and sum any combination of any three numbers from the set 1, 4, 7, 10, (duplicates permitted, e.g. 4 + 4+7) and to say what they noticed. The key noticing arising from this part of the task is that all totals achieved will be multiples of three; once this was established, pupils were tasked with explaining this finding. It was within this latter part of the task that four of the five LA pupils contributed important noticings; data presentation thus focuses on this part of the task. In the transcripts below, pupils’ utterances and any particular features of it, such as tone or emphasis, is noted; a set of dots indicates a silent pause before the pupil resumes speech.

Moving on from a stuck position through structural noticing

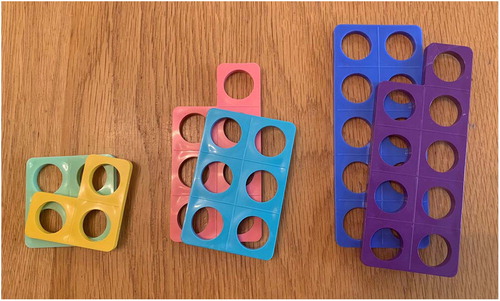
This section details findings relating to two focus LA pupils: first Mia (working with HA partner Jade) and subsequently Rose (working with HA partner Hannah) both of whom contributed pivotal noticings that resolved a state of being stuck in the task. In both classrooms teachers had encouraged the use of Numicon®, a resource presenting an image of number values from 1-10. Figures 1–5 show Numicon used across classrooms in this activity.

Figure 1. 1 + 4 + 7 shown as a multiple of 3.

Figure 2. “They all have one left over”.

Figure 3. “It makes another 3”.

Figure 4. Remainders.

Figure 5. Jacob’s use of Numicon.

Mia and Jade had used this resource to confirm that when summed, the total of any three of the available numbers was a multiple of three by placing a Numicon representation of a total on the table and covering it with Numicon 3 pieces (reconstructed in Figure 1). Representations such as this linked property and structure through demonstrating that a number had the property of being in the three times table because it could be constructed from a set of threes.

At this stage both Mia and Jade were engaging with each of the individual numbers as single entities (e.g. a “4”, a “7”); whilst they had engaged in consideration of the properties of totals obtained when three numbers were summed, they did not appear to have contemplated individual or shared properties of the numbers 1, 4, 7, or 10. An important noticing arose following the class teacher’s intervention to address their evident state of being stuck. A space was cleared on the table and Numicon 1, 4, 7 and 10 pieces placed in the space. The class teacher asked the pair to refocus on these four numbers and look at them again. Very shortly afterwards the following interaction took place:

Mia:

inhales sharply, smiles. “Ah”. She points at the Numicon; “in all three numbers, oh, um, they have like, one left over”. She picks up the Numicon 4, putting it on the table in front of her and places a 3 on top of it. “If you like take 3 away from that you have one left.” She touches the uncovered space on the Numicon 4. Indicating the 7 she continues, “you get the same with this one”. She puts two 3s on the 7.

Jade:

leans back, smiles and releases a long “ahhhh” sigh.

Mia:

“and you would get the same with 10 as well”. She puts three 3s on the 10 showing the one left over. (reconstructed in Figure 2)

Mia’s sharp inhalation and utterance “Ah” is an “Aha” moment (Mason et al., 2010) of new clarity regarding the problem scenario. It arises from a “shift of attention” (Mason, 2011, p. 45) in which she moves from seeing each of the three numbers as a single entity to seeing each as a multiple of 3 plus 1. This essential noticing foreruns the explanation of why adding three such numbers together results in a total which is a multiple of 3. Mia’s modelling with the Numicon, positioning Numicon 3s on top of each of the pieces in turn and highlighting the “one left” each time evidences her noticing of the commonality of structure and that this commonality relates to the three times table. Although four numbers are in the set, Mia’s first utterance refers to “all three numbers”; her actions make apparent her focus is on 4, 7 and 10. She did not return to the issue of whether and how 1 could be included in the commonality she had noted. Jade’s smile and sigh indicate a realisation dawning as she observes Mia’s action and listens to her speech.

Mia’s modelling with Numicon and her particular focus on the “one left over” supports a further important noticing. Pointing to the “left over” spaces on each of three separate Numicon models in front of her Mia says:

Mia:

“one, two, three, it makes another three”

This final noticing constituted Mia’s initial explanation of why totals of any three of the numbers were always multiples of three. Following a later prompt from the class teacher, Mia arranged the Numicon pieces to show how the three “left over” spaces could be physically arranged to enable a further 3 piece to be added (reconstructed in Figure 3), providing the pair with confidence to articulate their reasoning in the end of lesson plenary.

As with that of Mia above, Rose’s noticing of structure emerged following an intervention from the teacher itself prompted by awareness that pupils were struggling to explain the multiple of 3 finding. The teacher paused the whole class to refocus pupils on the individual numbers asking, “are any of the numbers you started with multiples of 3?”

This question provoked a productive shift of attention to the properties and structure of the individual numbers. Rose, with a Numicon 7 on the table in front of her used her hand to partition the 7 to show it as 6 and 1. Whilst the specific noticing about the properties of the number 7 that accompanied this action cannot be conclusively inferred from this alone, the action does signal a noticing that 7 could be considered as other than a single entity, i.e. as having component parts. A few moments later, Hannah speaks:

Hannah:

“yeah cos four is, one less is three and seven, one less is six”. Picking up a Numicon 1 piece and holding it up, she says in impassioned tones: “And the ‘one’ I have no idea about  …  the one is just, a one!”

Here Hannah (HA) notices that numbers one less than 4 and 7 are both multiples of 3. However, identification that the “1” shared this commonality eluded her. Rose (LA) was able to contribute the key noticing:

Rose:

taking the “one” piece from Hannah: “no, no, no, if it was one less it would be zero and there would be nothing there. And that would be a multiple of 3. Zero, three, six  … ” she waves her hand right and left as she begins a rhythmic times table count.

Hannah:

excitedly: “Oh yeah!  …  Oh yeah!”

Rose:

smiles broadly.

Exposure to the noticing of her higher attaining partner, arguably supported Rose in shifting her attention to considering one less than the number in question, stimulating the noticing demonstrated above. However, in doing so she demonstrates that she can follow and extend the higher attainers’ line of thinking. Her noticing that 1 has an equivalent relationship to multiples of 3 as do the other numbers in the set (i.e. that all are one more than a multiple of 3) is particularly important, not to mention being a feature that eluded both HA and LA pupils in two of the other focus pupil pairs. Specifically, the identification of shared commonality across all four of the numbers supported the articulation of generality that ensued and the pairs’ confidence in it. The response from both girls to this noticing; excitement from Hannah and delight from Rose, served to give the task further momentum.

This identified commonality led directly to the model in Figure 4, in which three Numicon 4 pieces have been set out with Numicon 3 and 1 pieces placed on top of each one. A small piece of paper labelled R is placed over each Numicon 1 piece. This model reflected their understanding of these as remainders and supported later reasoning about the result of adding three such remainders together.

For both Mia (LA) and Rose (LA), in their respective lessons, the making of important contributions to the progress of the task raised their status in the partnerships and afforded their continued confident engagement in the task. For Mia, surprise, reflecting perhaps her expectations of herself (Archer et al., 2018), as well as pride in her achievement are both evident in a comment made at the end of the lesson:

Mia:

“I thought Jade would be the first one to figure out like, find out like there’s one left over, but I did.”

Rose, on hearing another pupil say that they “kind of got it”, simply shows her pleasure:

Rose:

“I didn’t kind of get it, I got it.’ She puts both thumbs up and smiles.

Challenging a conjecture through property noticing

Joe (LA) and Ash (HA) were at the point in the task where they had noticed that all totals are multiples of 3 and were beginning to consider why this is the case:

Ash:

“‘cos you can only pick three numbers, can’t you?”

Joe:

nods

Ash:

“and they’re in the three times tables”

Ash’s conjecture, that the total is a multiple of three because three numbers have been summed, whilst incorrect, was common across all classes and represented a connection noted between a property of the outcomes and the problem scenario. As Ash began to record this conjecture on the shared recording sheet, Joe looks hard at the board before speaking:

Joe:

“Ah but Ash if you were only allowed to choose 2 it would be in the one times table only”

In making this challenge Joe continues Ash’s line of reasoning and shows that he has noticed that the conjecture does not hold true if only two numbers are summed; the total is not in the two times table as would be expected. In response, Ash looks towards the board with a puzzled expression and selects two numbers. In the extract below, Joe takes these two numbers on as an example to demonstrate the validity of his argument:

Ash:

“4 + 7”

Joe:

“4 + 7 = 11  …  and what calculation goes into 11, only 1 and 11”

Joe’s noticing is of property, and specifically of 11 as a prime number, to reason that since its only factors are 1 and 11, it cannot be in the two times table. Whilst the set of numbers from which they are selecting does include those which could result in a total in the two times table (eg 10 + 4; 7 + 1) Joe is correct that this will not always be so. Thus, Ash’s reasoning that the earlier totals are multiples of three because they are adding three of them does not hold true if two numbers are summed. This exchange and Joe’s challenge to Ash’s conjecture impact greatly on the work of this pair; indeed, this theme productively dominates their thinking for a large part of the remaining activity time as they contemplate how the set of numbers could be manipulated to render Ash’s conjecture true for summing 2, 3 and 4 numbers. It is the topic that they feed back during the mid-lesson plenary, in which the class teacher explicitly notes the merit of Joe’s reasoning. As with Mia and Jade above, Joe’s pride in his contribution is evident both in his immediate response and in his keenness to share it with the class.

Laying the groundwork through pattern and property noticing

Following the instruction to explain why totals were multiples of three, both April (LA) and Jacob (HA) stared hard at the board on which the numbers 1, 4, 7, 10 were displayed:

April:

“none of them are in the three times tables  …  no,,,wait  …  she pauses then points at the board: if you notice that 1 to get to 4 is 3 and 4 to get to 7” she looks at Jacob who looks at the board. “In between, in like the middle is 3”

Jacob:

“wait so”

April:

“so like 1 to get to 4 is 3”

Jacob:

“yeah then 4 to get to 7  …  is 3, um”. He pauses, then turns to April “it’s all in the three  …   I think it’s always” he drums his fingers on the table and pauses again, “I know  …  it’s the three times table add one”

Unlike the situation for LA pupils Mia and Rose, in which the key noticing had arisen when representation of the numbers and their relationship with multiples of three was being explored using manipulatives, in this exchange engagement is purely with the symbolic representation of the numerals on the whiteboard. Here, April’s noticing of pattern and property served to lay the groundwork for Jacob’s eventual noticing in the final utterance above. First she notices that none of the initial four numbers has the property of being in the three times table, and second that there is pattern of a difference of three between each successive pair. Jacob’s attention to his partner’s contribution is evident in his continuation of her line of thinking as he begins to speak providing evidence of its contribution to his own thinking. Noteworthy also is his valuing of it through his subsequent decision to record it, as “there’s a difference of three between all of the numbers” and his contribution in re-phrasing it in so doing, into a more mathematically sophisticated form.

Challenges in the use of manipulatives

Findings above have noted the role of manipulatives in supporting the noticing of structure for Mia and Rose. It is thus important to note findings which reveal complexity in this aspect of the intervention design. This section addresses two LA pupils, April and Charley and their respective HA partners Jacob and Morgan.

Following the noticing reported above, April’s HA partner Jacob led the construction, using Numicon, of a representation of 4, 7 and 10 to show that each is one more than a multiple of 3. However, rather than constructing the 7 as 3 + 3+1 and the 10 as 3+3+3+1, (as had been the case in Figure 2), Jacob constructs them as 6 + 1, and 9 + 1 respectively (reconstructed in Figure 5). This meant that the multiple of 3 was represented rather than the number of groups of three that were part of this multiple; this subtle difference rendered the representation less useful in communicating structure and relationship for April:

April:

“I don’t get why we are doing this”

Whilst Jacob’s explanation satisfied her and she was able to articulate later that adding two of the numbers was not sufficient, three were needed to get a multiple of three, her pathway to this was arguably made more challenging by the way in which the relationship was constructed.

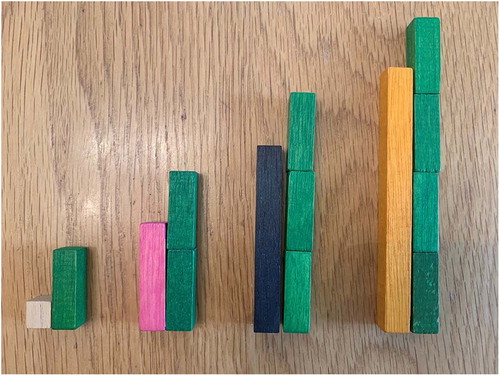
Charley’s participation in the task was also hampered by a particular representation, constructed by her HA partner Morgan, that did not sufficiently communicate, for her, the essential mathematical relationships. In addition to Numicon this classroom also contained Cuisenaire, a set of cuboid shaped rods representing values from 1–10 (shown in Figure 6). Since both Numicon and Cuisenaire could represent the essential structure and were familiar to pupils, both were available for use. Figure 6 reconstructs how Cuisenaire was used by Morgan. The left-hand rod of each pair represents the 1,4,7,10 respectively and the right-hand rod the related multiple of three. However, rather than presenting an image of, for example, 7 as equivalent to 3 + 3+1 which would have rendered the height of both bars in each pair equal, the construction presents 7 as equivalent to 3 + 3+3-2, with a height difference between adjoining bars equivalent to 2. The challenges arising from this particular arrangement proved a barrier for Charley and her subsequent participation in the task suffered as a result.

Figure 6. Morgan’s use of Cuisenaire.

Discussion

Data presented above was taken from one mathematical task taught across three primary school classrooms, and has shown lower attaining pupils collectively contributing noticing of pattern, property and structure. In so doing these pupils’ noticings have laid the groundwork for the development of ideas and been used to challenge conjecture, or to move thinking along when their HA partner was stuck. Material progress in the task was made through a process in which the LA pupils played an integral part. Across the wider study from which this lesson was drawn, lower attainers contributed mathematical noticings in the context of the tasks presented that mirror the findings presented above. These noticings were frequent across all classrooms (typically between 3 and 6 critical events for each LA pupil in each lesson) and documented for all lower attainers, including Charley who did not contribute strongly in the lesson reported here. Whilst this frequency represents an important outcome in itself, more important is the impact of LA pupils’ noticings on task progress. As was the case with Mia, Rose and Joe, reported here, lower attainers’ contributions were, on several occasions, pivotal to task progress, contributed at moments where both pupils were stuck, or providing an insight that changed the course of subsequent activity.

This paper does not seek to deny the benefits identified in earlier research that LA pupils accrue from working with HA partners (Fawcett & Garton, 2005; Leonard, 2001). Indeed, data presented here demonstrate the role of the HA pupil in stimulating a conjecture later challenged by the LA pupil, or in beginning a line of thinking subsequently further developed by the LA pupil. However, this paper does argue that the HA pupil’s contributions are not the only source of benefit for either pupil; furthermore, data does not support concerns voiced by teachers and pupils (Esmonde et al., 2009; McGillicuddy & Devine, 2018) that HA pupils will be held back by working with LA peers. LA pupils in Tereshchenko et al.’s (2019) study believed that they could contribute to mixed group mathematics working and this paper shows that in the primary classroom, this is indeed the case. Evidence from this study is that in mixed attainment partnerships, gains for the LA pupil do not arise purely through exposure to the activities and dialogue of their HA partners. LA pupils can also gain through their own mathematical contributions and from the reasoning that they build from these. HA pupils similarly can gain from exposure to and use of the contributions that their LA pupil partners make. In short, mixed attainment working can produce bi-directional benefits.

Whilst it is not possible to know what would have happened without teachers’ questions and refocusing prompts, it is evident that prompts, e.g. to represent numbers using resources, or to refocus on properties of the numbers concerned, immediately preceded the development of important noticings on several occasions. Thus, data from this study supports the earlier finding that the teacher has a pivotal role in directing pupil attention towards salient relationships (Lobato et al., 2013). However, the partially internal nature of the construct of noticing means that appropriate teacher intervention is not easy to achieve. Indeed, Mason (2020) notes that what pupils articulate will only be a fraction of their experience and that listening carefully and responding with appropriate action requires significant mathematical experience and sophistication; skilful questioning from the three class teachers was certainly evident in this study. Further, Lobato et al. (2013) suggest that teacher pedagogy can influence pupils’ subsequent noticing behaviour, stimulating noticing in future situations. In order for this to happen, Mason (2020) argues that whilst the activity of noticing should be valued, equally important is the probing and exploring of the pupil’s thinking such that learning about noticing, and more productive noticing, can emerge.

The use of manipulatives as a key intervention component also merits closer attention. When Mia and Rose were developing and demonstrating important structural noticing, physical representation of the relevant ideas was intrinsically involved. Such examples mirror the findings of earlier research (Foote & Lambert, 2011; Houssart, 2004). However, manipulative use by HA pupils was less successful in provoking productive noticing in the case of two further LA pupils, April and Charley. In these instances, the mathematical relationships at issue were not effectively communicated, and the lower attainer was left trying to follow their partner’s reasoning. Whilst in both cases the LA pupil was able to do so, in neither case was this the equitable participation seen with other pairs. When the differences in representation are examined, they are revealed to be slight; Jacob (HA) used multiples of 3 rather than multiple 3 pieces to represent the relationship at stake and Morgan (HA) drew on an associated idea that a number that is one more than one multiple of 3 can also be considered to be two less than the next multiple of three. Whilst Morgan’s use of Cuisenaire rather than Numicon may be considered to have contributed to the difficulty for Charley, arguably the challenge lies more in Morgan’s use of “two less than” rather than “one more than” a multiple. Nevertheless, in both cases the particular representations constructed rendered April and Charley respectively as followers in this section of the activity, despite, particularly in April’s case, having been an active participator and contributor to that point. Whilst research cautions that manipulative use is no guarantee of the development of understanding (Clements, 2000; Marley & Carbonneau, 2014), the subtleties of difference in the manner of use in this study highlight the critical role of the teacher in the careful selection of, and guidance and support for, manipulative use.

Conclusions

In order to break the vicious cycle identified by Taylor et al. (2017), generation of examples of successful mixed attainment working are needed; this paper provides one such example. However, this paper contributes more than this. Hewitt (2009) argues that building lesson experiences on pupils’ ability to notice and observe renders content accessible to all because engagement does not rely purely on recall of previous learning. Data presented here support this argument; in this study, LA pupils frequently contributed key noticings in advance of their HA peers evidencing a more equitable access to the mathematical ideas embedded in the task. This was achieved through a pedagogical approach which promoted and valued a process which Gattegno (2010) argued to be a natural facet of human activity. It may be, therefore, that the use of a clear pedagogical focus on noticing combined with the task structures employed in this study, is one way for pupils to engage in mathematics together without the attainment labels that carry with them such weight of teacher and pupil expectation. In order for mathematics education to achieve greater equity in the opportunities provided to all pupils, avenues such as this are surely important to pursue.

A strong recommendation arises therefore for the use of a pedagogical focus on noticing alongside the use of mixed attainment pairs and groups. However, this paper has also revealed complexity arising from the representation of numbers and relationships through the use of manipulatives and recommends that teacher promotion of this important component of a focus on noticing must include support to enable pupils to use manipulatives to construct and communicate the significant aspects of the relationships at issue. Finally, this paper has illustrated the pivotal role of the teacher in provoking productive noticing; further development of pedagogies which promote pupil noticing requires parallel support for teachers to reflect on and develop their own skill and confidence in listening and responding productively.

Disclosure statement

No potential conflict of interest was reported by the author(s).